|
Pentagon Jr.
In geometry, a pentagon (from the Greek πέντε ''pente'' meaning ''five'' and γωνία ''gonia'' meaning ''angle'') is any five-sided polygon or 5-gon. The sum of the internal angles in a simple pentagon is 540°. A pentagon may be simple or self-intersecting. A self-intersecting ''regular pentagon'' (or ''star pentagon'') is called a pentagram. Regular pentagons A ''regular pentagon'' has Schläfli symbol and interior angles of 108°. A ''regular pentagon'' has five lines of reflectional symmetry, and rotational symmetry of order 5 (through 72°, 144°, 216° and 288°). The diagonals of a convex regular pentagon are in the golden ratio to its sides. Given its side length t, its height H (distance from one side to the opposite vertex), width W (distance between two farthest separated points, which equals the diagonal length D) and circumradius R are given by: :\begin H &= \frac~t \approx 1.539~t, \\ W= D &= \frac~t\approx 1.618~t, \\ W &= \sqrt \ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cyclic Polygon
In geometry, the circumscribed circle or circumcircle of a polygon is a circle that passes through all the vertices of the polygon. The center of this circle is called the circumcenter and its radius is called the circumradius. Not every polygon has a circumscribed circle. A polygon that does have one is called a cyclic polygon, or sometimes a concyclic polygon because its vertices are concyclic. All triangles, all regular simple polygons, all rectangles, all isosceles trapezoids, and all right kites are cyclic. A related notion is the one of a minimum bounding circle, which is the smallest circle that completely contains the polygon within it, if the circle's center is within the polygon. Every polygon has a unique minimum bounding circle, which may be constructed by a linear time algorithm. Even if a polygon has a circumscribed circle, it may be different from its minimum bounding circle. For example, for an obtuse triangle, the minimum bounding circle has the longest ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Golden Ratio
In mathematics, two quantities are in the golden ratio if their ratio is the same as the ratio of their sum to the larger of the two quantities. Expressed algebraically, for quantities a and b with a > b > 0, where the Greek letter phi ( or \phi) denotes the golden ratio. The constant \varphi satisfies the quadratic equation \varphi^2 = \varphi + 1 and is an irrational number with a value of The golden ratio was called the extreme and mean ratio by Euclid, and the divine proportion by Luca Pacioli, and also goes by several other names. Mathematicians have studied the golden ratio's properties since antiquity. It is the ratio of a regular pentagon's diagonal to its side and thus appears in the construction of the dodecahedron and icosahedron. A golden rectangle—that is, a rectangle with an aspect ratio of \varphi—may be cut into a square and a smaller rectangle with the same aspect ratio. The golden ratio has been used to analyze the proportions of natural o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Regular Pentagon Using Carlyle Circle
The term regular can mean normal or in accordance with rules. It may refer to: People * Moses Regular (born 1971), America football player Arts, entertainment, and media Music * "Regular" (Badfinger song) * Regular tunings of stringed instruments, tunings with equal intervals between the paired notes of successive open strings Other uses in arts, entertainment, and media * Regular character, a main character who appears more frequently and/or prominently than a recurring character * Regular division of the plane, a series of drawings by the Dutch artist M. C. Escher which began in 1936 * ''Regular Show'', an animated television sitcom * '' The Regular Guys'', a radio morning show Language * Regular inflection, the formation of derived forms such as plurals in ways that are typical for the language ** Regular verb * Regular script, the newest of the Chinese script styles Mathematics There are an extremely large number of unrelated notions of "regularity" in mathematics. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Exact Trigonometric Values
In mathematics, the values of the trigonometric functions can be expressed approximately, as in \cos (\pi/4) \approx 0.707, or exactly, as in \cos (\pi/ 4)= \sqrt 2 /2. While trigonometric tables contain many approximate values, the exact values for certain angles can be expressed by a combination of arithmetic operations and square roots. Common angles The trigonometric functions of angles that are multiples of 15°, 18°, or 22.5° have simple algebraic values. These values are listed in the following table for angles from 0° to 90°.Abramowitz, Milton and Irene A. Stegun, p. 74 For angles outside of this range, trigonometric values can be found by applying the reflection and shift identities. In the table below, \infty stands for the ratio 1:0. These values can also be considered to be undefined (see division by zero). : Expressibility with square roots Some exact trigonometric values, such as \sin(60^\circ)=\sqrt/2, can be expressed in terms of a combination of arith ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Double Angle Formula
In trigonometry, trigonometric identities are equalities that involve trigonometric functions and are true for every value of the occurring variables for which both sides of the equality are defined. Geometrically, these are identities involving certain functions of one or more angles. They are distinct from triangle identities, which are identities potentially involving angles but also involving side lengths or other lengths of a triangle. These identities are useful whenever expressions involving trigonometric functions need to be simplified. An important application is the integration of non-trigonometric functions: a common technique involves first using the substitution rule with a trigonometric function, and then simplifying the resulting integral with a trigonometric identity. Pythagorean identities The basic relationship between the sine and cosine is given by the Pythagorean identity: :\sin^2\theta + \cos^2\theta = 1, where \sin^2 \theta means (\sin \theta)^2 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Half-angle Formula
In trigonometry, trigonometric identities are equalities that involve trigonometric functions and are true for every value of the occurring variables for which both sides of the equality are defined. Geometrically, these are identities involving certain functions of one or more angles. They are distinct from triangle identities, which are identities potentially involving angles but also involving side lengths or other lengths of a triangle. These identities are useful whenever expressions involving trigonometric functions need to be simplified. An important application is the integration of non-trigonometric functions: a common technique involves first using the substitution rule with a trigonometric function, and then simplifying the resulting integral with a trigonometric identity. Pythagorean identities The basic relationship between the sine and cosine is given by the Pythagorean identity: :\sin^2\theta + \cos^2\theta = 1, where \sin^2 \theta means (\sin \theta)^2 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
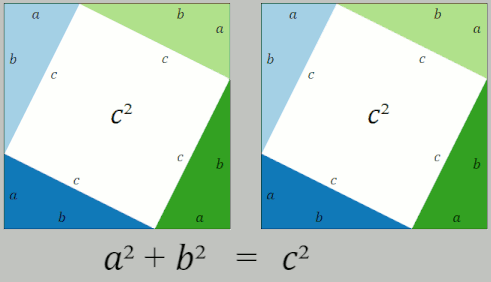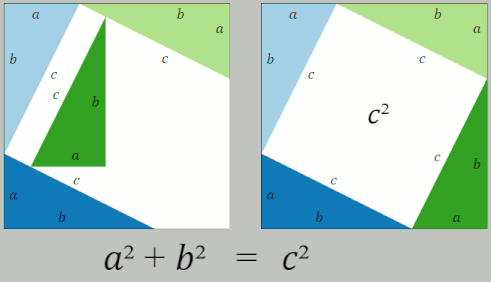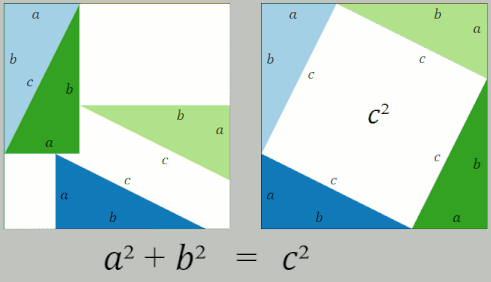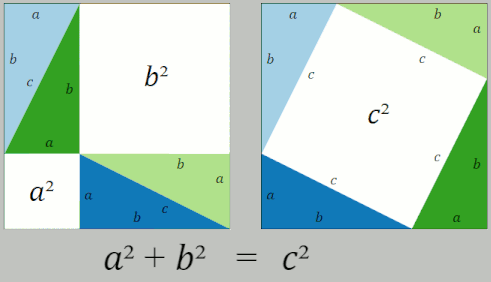
Pythagoras' Theorem
In mathematics, the Pythagorean theorem or Pythagoras' theorem is a fundamental relation in Euclidean geometry between the three sides of a right triangle. It states that the area of the square whose side is the hypotenuse (the side opposite the right angle) is equal to the sum of the areas of the squares on the other two sides. This theorem can be written as an equation relating the lengths of the sides ''a'', ''b'' and the hypotenuse ''c'', often called the Pythagorean equation: :a^2 + b^2 = c^2 , The theorem is named for the Greek philosopher Pythagoras, born around 570 BC. The theorem has been proven numerous times by many different methods – possibly the most for any mathematical theorem. The proofs are diverse, including both geometric proofs and algebraic proofs, with some dating back thousands of years. When Euclidean space is represented by a Cartesian coordinate system in analytic geometry, Euclidean distance satisfies the Pythagorean relation: the squared dis ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Polyhedra (book)
''Polyhedra'' is a book on polyhedra, by Peter T. Cromwell. It was published by in 1997 by the Cambridge University Press, with an unrevised paperback edition in 1999. Topics The book covers both the mathematics of polyhedra and its historical development, limiting itself only to three-dimensional geometry. The notion of what it means to be a polyhedron has varied over the history of the subject, as have other related definitions, an issue that the book handles largely by keeping definitions informal and flexible, and by pointing out problematic examples for these intuitive definitions. Many digressions help make the material readable, and the book includes many illustrations, including historical reproductions, line diagrams, and photographs of models of polyhedra. ''Polyhedra'' has ten chapters, the first four of which are primarily historical, with the remaining six more technical. The first chapter outlines the history of polyhedra from the ancient world up to Hilbert's third ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Richmond Pentagon 1
Richmond most often refers to: * Richmond, Virginia, the capital of Virginia, United States * Richmond, London, a part of London * Richmond, North Yorkshire, a town in England * Richmond, British Columbia, a city in Canada * Richmond, California, a city in California, United States Richmond may also refer to: People * Richmond (surname) * Earl of Richmond * Duke of Richmond * Richmond C. Beatty (1905–1961), American academic, biographer and critic * Richmond Avenal, character in British sitcom The IT Crowd Places Australia * Richmond, New South Wales ** RAAF Base Richmond ** Richmond Woodlands Important Bird Area * Richmond River, New South Wales **Division of Richmond ** Electoral district of Richmond (New South Wales) * Richmond, Queensland * Richmond, South Australia * Richmond, Tasmania * Richmond, Victoria ** Electoral district of Richmond (Victoria) ** City of Richmond Canada * Richmond, British Columbia, a city in Metro Vancouver ** Richmond (British Columbia provinc ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fermat Prime
In mathematics, a Fermat number, named after Pierre de Fermat, who first studied them, is a positive integer of the form :F_ = 2^ + 1, where ''n'' is a non-negative integer. The first few Fermat numbers are: : 3, 5, 17, 257, 65537, 4294967297, 18446744073709551617, ... . If 2''k'' + 1 is prime and ''k'' > 0, then ''k'' must be a power of 2, so 2''k'' + 1 is a Fermat number; such primes are called Fermat primes. , the only known Fermat primes are ''F''0 = 3, ''F''1 = 5, ''F''2 = 17, ''F''3 = 257, and ''F''4 = 65537 ; heuristics suggest that there are no more. Basic properties The Fermat numbers satisfy the following recurrence relations: : F_ = (F_-1)^+1 : F_ = F_ \cdots F_ + 2 for ''n'' ≥ 1, : F_ = F_ + 2^F_ \cdots F_ : F_ = F_^2 - 2(F_-1)^2 for ''n'' ≥ 2. Each of these relations can be proved by mathematical induction. From the second equation, we can deduce Goldbach's theorem (named after Christian Goldbach): no two Fermat numbers share a common integer factor ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Compass And Straightedge
In geometry, straightedge-and-compass construction – also known as ruler-and-compass construction, Euclidean construction, or classical construction – is the construction of lengths, angles, and other geometric figures using only an idealized ruler and a pair of compasses. The idealized ruler, known as a straightedge, is assumed to be infinite in length, have only one edge, and no markings on it. The compass is assumed to have no maximum or minimum radius, and is assumed to "collapse" when lifted from the page, so may not be directly used to transfer distances. (This is an unimportant restriction since, using a multi-step procedure, a distance can be transferred even with a collapsing compass; see compass equivalence theorem. Note however that whilst a non-collapsing compass held against a straightedge might seem to be equivalent to marking it, the neusis construction is still impermissible and this is what unmarked really means: see Markable rulers below.) More formall ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Circumscribed Circle
In geometry, the circumscribed circle or circumcircle of a polygon is a circle that passes through all the vertices of the polygon. The center of this circle is called the circumcenter and its radius is called the circumradius. Not every polygon has a circumscribed circle. A polygon that does have one is called a cyclic polygon, or sometimes a concyclic polygon because its vertices are concyclic. All triangles, all regular simple polygons, all rectangles, all isosceles trapezoids, and all right kites are cyclic. A related notion is the one of a minimum bounding circle, which is the smallest circle that completely contains the polygon within it, if the circle's center is within the polygon. Every polygon has a unique minimum bounding circle, which may be constructed by a linear time algorithm. Even if a polygon has a circumscribed circle, it may be different from its minimum bounding circle. For example, for an obtuse triangle, the minimum bounding circle has the longes ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |