|
Orthogonal Representation
In mathematics, orthogonality is the generalization of the geometric notion of '' perpendicularity''. By extension, orthogonality is also used to refer to the separation of specific features of a system. The term also has specialized meanings in other fields including art and chemistry. Etymology The word comes from the Ancient Greek ('), meaning "upright", and ('), meaning "angle". The Ancient Greek (') and Classical Latin ' originally denoted a rectangle. Later, they came to mean a right triangle. In the 12th century, the post-classical Latin word ''orthogonalis'' came to mean a right angle or something related to a right angle. Mathematics Physics * In optics, polarization states are said to be orthogonal when they propagate independently of each other, as in vertical and horizontal linear polarization or right- and left-handed circular polarization. * In special relativity, a time axis determined by a rapidity of motion is hyperbolic-orthogonal to a space axis ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Relativity Of Simultaneity
In physics, the relativity of simultaneity is the concept that ''distant simultaneity'' – whether two spatially separated events occur at the same time – is not absolute, but depends on the observer's reference frame. This possibility was raised by mathematician Henri Poincaré in 1900, and thereafter became a central idea in the special theory of relativity. Description According to the special theory of relativity introduced by Albert Einstein, it is impossible to say in an ''absolute'' sense that two distinct events occur at the same time if those events are separated in space. If one reference frame assigns precisely the same time to two events that are at different points in space, a reference frame that is moving relative to the first will generally assign different times to the two events (the only exception being when motion is exactly perpendicular to the line connecting the locations of both events). For example, a car crash in London and another in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Motorola 68000
The Motorola 68000 (sometimes shortened to Motorola 68k or m68k and usually pronounced "sixty-eight-thousand") is a 16/32-bit complex instruction set computer (CISC) microprocessor, introduced in 1979 by Motorola Semiconductor Products Sector. The design implements a 32-bit instruction set, with 32-bit registers and a 16-bit internal data bus. The address bus is 24 bits and does not use memory segmentation, which made it easier to program for. Internally, it uses a 16-bit data arithmetic logic unit (ALU) and two more 16-bit ALUs used mostly for addresses, and has a 16-bit external data bus. For this reason, Motorola termed it a 16/32-bit processor. As one of the first widely available processors with a 32-bit instruction set, and running at relatively high speeds for the era, the 68k was a popular design through the 1980s. It was widely used in a new generation of personal computers with graphical user interfaces, including the Macintosh 128K, Amiga, Atari ST, and X68000. Th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Thyssen-Bornemisza Museum
The Thyssen-Bornemisza National Museum (in Spanish, the Museo Nacional Thyssen-Bornemisza (), named after its founder), or simply the Thyssen, is an art museum in Madrid, Spain, located near the Prado Museum on one of the city's main boulevards. It is known as part of the "Golden Triangle of Art", which also includes the Prado and the Reina Sofía national galleries. The Thyssen-Bornemisza fills the historical gaps in its counterparts' collections: in the Prado's case this includes Italian primitives and works from the English, Dutch and German schools, while in the case of the Reina Sofia it concerns Impressionists, Expressionists, and European and American paintings from the 20th century. With over 1,600 paintings, it was once the second largest private collection in the world after the British Royal Collection.Jonathan Kandell"Baron Thyssen-Bornemisza, Industrialist Who Built Fabled Art Collection, Dies at 81,"New York ''Times'', 28 April 2002. A competition was held to house t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Website
A website (also written as a web site) is a collection of web pages and related content that is identified by a common domain name and published on at least one web server. Examples of notable websites are Google Search, Google, Facebook, Amazon (website), Amazon, and Wikipedia. All publicly accessible websites collectively constitute the World Wide Web. There are also private websites that can only be accessed on a intranet, private network, such as a company's internal website for its employees. Websites are typically dedicated to a particular topic or purpose, such as news, education, commerce, entertainment or social networking. Hyperlinking between web pages guides the navigation of the site, which often starts with a home page. User (computing), Users can access websites on a range of devices, including desktop computer, desktops, laptops, tablet computer, tablets, and smartphones. The application software, app used on these devices is called a Web browser. History ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Burgoyne Diller
Burgoyne A. Diller (January 13, 1906 – January 30, 1965) was an American abstract painter. Many of his best-known works are characterized by orthogonal geometric forms that reflect his strong interest in the De Stijl movement and the work of Piet Mondrian in particular. Overall, his Geometric abstraction and non-objective style also owe much to his study with Hans Hofmann at the Art Students League of New York. He was a founding member of the American Abstract Artists.Larsen, Susan C. “The American Abstract Artists: A Documentary History 1936-1941”, ''Archives of American Art Journal'', Vol. 14, No. 1 (1974), p 2. Diller's abstract work has sometimes been termed " constructivist". He also did figurative and representational works early in his career working as a muralist for the New York City Federal Arts Project. Life Diller was born in The Bronx, New York in 1906 to Andrew Diller, a violinist and conductor, and Mary Burgoyne. His father died in 1908, while Diller was ju ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Piet Mondrian
Pieter Cornelis Mondriaan (), after 1906 known as Piet Mondrian (, also , ; 7 March 1872 – 1 February 1944), was a Dutch painter and art theoretician who is regarded as one of the greatest artists of the 20th century. He is known for being one of the pioneers of 20th-century abstract art, as he changed his artistic direction from figurative painting to an increasingly abstract style, until he reached a point where his artistic vocabulary was reduced to simple geometric elements. Mondrian's art was highly utopian and was concerned with a search for universal values and aesthetics. He proclaimed in 1914: "Art is higher than reality and has no direct relation to reality. To approach the spiritual in art, one will make as little use as possible of reality, because reality is opposed to the spiritual. We find ourselves in the presence of an abstract art. Art should be above reality, otherwise it would have no value for man." His art, however, always remained rooted in nature. H ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
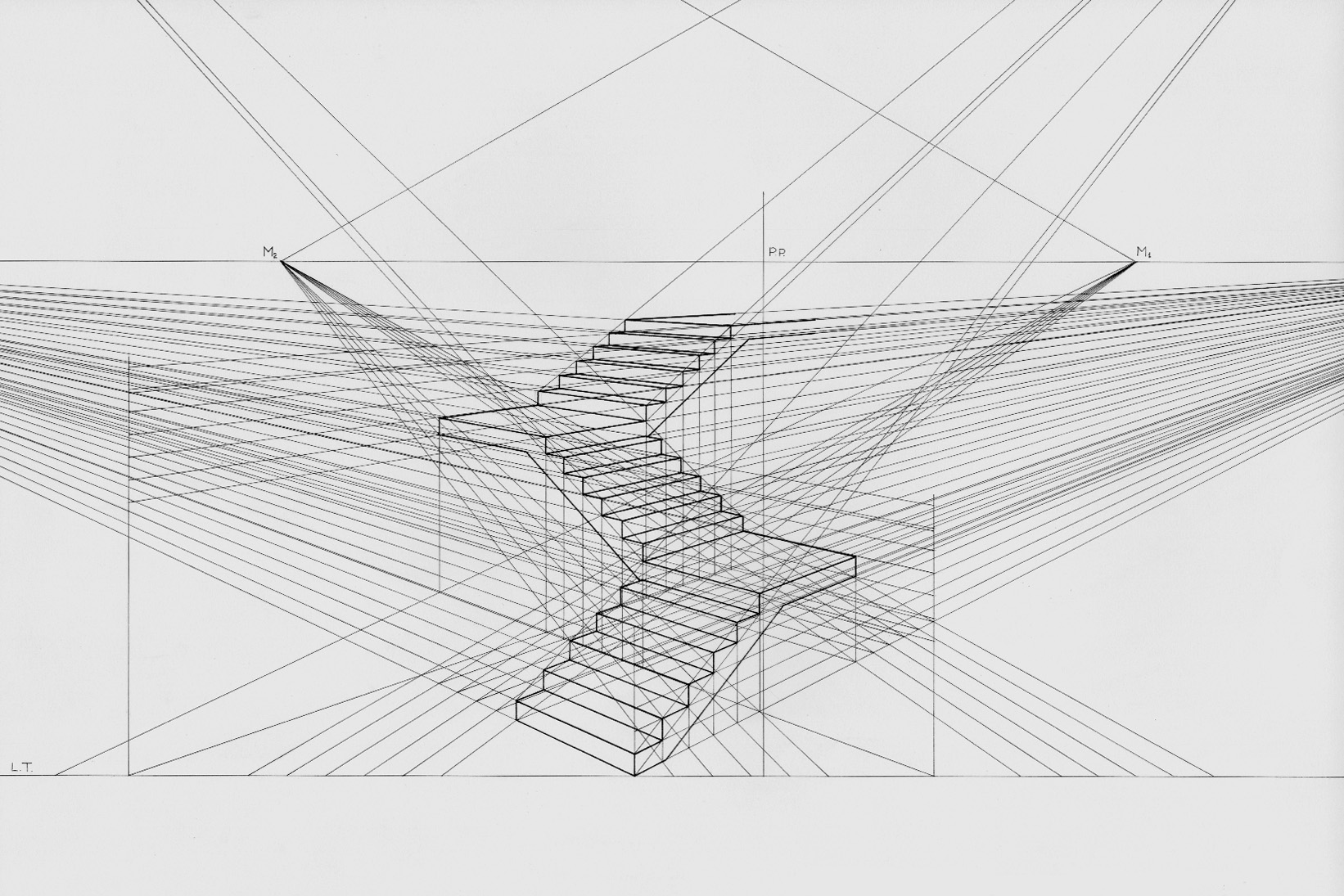
Vanishing Point
A vanishing point is a point on the image plane of a perspective drawing where the two-dimensional perspective projections of mutually parallel lines in three-dimensional space appear to converge. When the set of parallel lines is perpendicular to a picture plane, the construction is known as one-point perspective, and their vanishing point corresponds to the oculus, or "eye point", from which the image should be viewed for correct perspective geometry.Kirsti Andersen (2007) ''Geometry of an Art'', p. xxx, Springer, Traditional linear drawings use objects with one to three sets of parallels, defining one to three vanishing points. Italian humanist polymath and architect Leon Battista Alberti first introduced the concept in his treatise on perspective in art, '' De pictura'', written in 1435. Vector notation The vanishing point may also be referred to as the "direction point", as lines having the same directional vector, say ''D'', will have the same vanishing point. Mathem ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Perspective (graphical)
Linear or point-projection perspective (from la, perspicere 'to see through') is one of two types of 3D projection, graphical projection perspective in the graphic arts; the other is parallel projection. Linear perspective is an approximate representation, generally on a flat surface, of an image as it is seen by the eye. Perspective drawing is useful for representing a three-dimensional scene in a two-dimensional medium, like paper. The most characteristic features of linear perspective are that objects appear smaller as their distance from the observer increases, and that they are subject to ''foreshortening'', meaning that an object's dimensions along the line of sight appear shorter than its dimensions across the line of sight. All objects will recede to points in the distance, usually along the horizon line, but also above and below the horizon line depending on the view used. Italian Renaissance painters and architects including Masaccio, Paolo Uccello, Piero della Fran ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sturm–Liouville Theory
In mathematics and its applications, classical Sturm–Liouville theory is the theory of ''real'' second-order ''linear'' ordinary differential equations of the form: for given coefficient functions , , and , an unknown function ''y = y''(''x'') of the free variable , and an unknown constant λ. All homogeneous (i.e. with the right-hand side equal to zero) second-order linear ordinary differential equations can be reduced to this form. In addition, the solution is typically required to satisfy some boundary conditions at extreme values of ''x''. Each such equation () together with its boundary conditions constitutes a Sturm–Liouville problem. In the simplest case where all coefficients are continuous on the finite closed interval and has continuous derivative, a function ''y = y''(''x'') is called a ''solution'' if it is continuously differentiable and satisfies the equation () at every x\in (a,b). In the case of more general , , , the solutions must be understood in a weak ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Schrödinger Equation
The Schrödinger equation is a linear partial differential equation that governs the wave function of a quantum-mechanical system. It is a key result in quantum mechanics, and its discovery was a significant landmark in the development of the subject. The equation is named after Erwin Schrödinger, who postulated the equation in 1925, and published it in 1926, forming the basis for the work that resulted in his Nobel Prize in Physics in 1933. Conceptually, the Schrödinger equation is the quantum counterpart of Newton's second law in classical mechanics. Given a set of known initial conditions, Newton's second law makes a mathematical prediction as to what path a given physical system will take over time. The Schrödinger equation gives the evolution over time of a wave function, the quantum-mechanical characterization of an isolated physical system. The equation can be derived from the fact that the time-evolution operator must be unitary, and must therefore be generated by t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dirac Notation
Distributed Research using Advanced Computing (DiRAC) is an integrated supercomputing facility used for research in particle physics, astronomy and cosmology in the United Kingdom. DiRAC makes use of multi-core processors and provides a variety of computer architectures for use by the research community. DiRAC and DiRAC II Initially DiRAC was funded with an investment of £12 million from the Government of the United Kingdom's Large Facilities Capital Fund combined with funds from the Science and Technology Facilities Council (STFC) and a consortium of universities in the UK. In 2012, the DiRAC facility was upgraded with a further £15 million of UK government capital to create DiRAC II which has five installations: # University of Cambridge HPC Service with 10000 cores and 1 Petabyte clustered file system # Cambridge Cosmos shared memory Service with 1856 cores, 14 Terabytes of globally shared memory with Intel Xeon Phi coprocessors # University of Leicester IT Services with ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |




.png)