|
Kodaira Embedding Theorem
In mathematics, the Kodaira embedding theorem characterises non-singular projective varieties, over the complex numbers, amongst compact Kähler manifolds. In effect it says precisely which complex manifolds are defined by homogeneous polynomials. Kunihiko Kodaira's result is that for a compact Kähler manifold ''M'', with a Hodge metric, meaning that the cohomology class in degree 2 defined by the Kähler form ω is an ''integral'' cohomology class, there is a complex-analytic embedding of ''M'' into complex projective space of some high enough dimension ''N''. The fact that ''M'' embeds as an algebraic variety follows from its compactness by Chow's theorem. A Kähler manifold with a Hodge metric is occasionally called a Hodge manifold (named after W. V. D. Hodge), so Kodaira's results states that Hodge manifolds are projective. The converse that projective manifolds are Hodge manifolds is more elementary and was already known. Kodaira also proved (Kodaira 1963), by recou ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics with the major subdisciplines of number theory, algebra, geometry, and analysis, respectively. There is no general consensus among mathematicians about a common definition for their academic discipline. Most mathematical activity involves the discovery of properties of abstract objects and the use of pure reason to prove them. These objects consist of either abstractions from nature orin modern mathematicsentities that are stipulated to have certain properties, called axioms. A ''proof'' consists of a succession of applications of deductive rules to already established results. These results include previously proved theorems, axioms, andin case of abstraction from naturesome basic properties that are considered true starting points of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Algebraic Geometry And Analytic Geometry
In mathematics, algebraic geometry and analytic geometry are two closely related subjects. While algebraic geometry studies algebraic varieties, analytic geometry deals with complex manifolds and the more general analytic spaces defined locally by the vanishing of analytic functions of several complex variables. The deep relation between these subjects has numerous applications in which algebraic techniques are applied to analytic spaces and analytic techniques to algebraic varieties. Main statement Let ''X'' be a projective complex algebraic variety. Because ''X'' is a complex variety, its set of complex points ''X''(C) can be given the structure of a compact complex analytic space. This analytic space is denoted ''X''an. Similarly, if \mathcal is a sheaf on ''X'', then there is a corresponding sheaf \mathcal^\text on ''X''an. This association of an analytic object to an algebraic one is a functor. The prototypical theorem relating ''X'' and ''X''an says that for any t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Simon Donaldson
Sir Simon Kirwan Donaldson (born 20 August 1957) is an English mathematician known for his work on the topology of smooth (differentiable) four-dimensional manifolds, Donaldson–Thomas theory, and his contributions to Kähler geometry. He is currently a permanent member of the Simons Center for Geometry and Physics at Stony Brook University in New York, and a Professor in Pure Mathematics at Imperial College London. Biography Donaldson's father was an electrical engineer in the physiology department at the University of Cambridge, and his mother earned a science degree there. Donaldson gained a BA degree in mathematics from Pembroke College, Cambridge, in 1979, and in 1980 began postgraduate work at Worcester College, Oxford, at first under Nigel Hitchin and later under Michael Atiyah's supervision. Still a postgraduate student, Donaldson proved in 1982 a result that would establish his fame. He published the result in a paper "Self-dual connections and the topology of sm ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Annals Of Mathematics
The ''Annals of Mathematics'' is a mathematical journal published every two months by Princeton University and the Institute for Advanced Study. History The journal was established as ''The Analyst'' in 1874 and with Joel E. Hendricks as the founding editor-in-chief. It was "intended to afford a medium for the presentation and analysis of any and all questions of interest or importance in pure and applied Mathematics, embracing especially all new and interesting discoveries in theoretical and practical astronomy, mechanical philosophy, and engineering". It was published in Des Moines, Iowa, and was the earliest American mathematics journal to be published continuously for more than a year or two. This incarnation of the journal ceased publication after its tenth year, in 1883, giving as an explanation Hendricks' declining health, but Hendricks made arrangements to have it taken over by new management, and it was continued from March 1884 as the ''Annals of Mathematics''. The n ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Springer-Verlag
Springer Science+Business Media, commonly known as Springer, is a German multinational publishing company of books, e-books and peer-reviewed journals in science, humanities, technical and medical (STM) publishing. Originally founded in 1842 in Berlin, it expanded internationally in the 1960s, and through mergers in the 1990s and a sale to venture capitalists it fused with Wolters Kluwer and eventually became part of Springer Nature in 2015. Springer has major offices in Berlin, Heidelberg, Dordrecht, and New York City. History Julius Springer founded Springer-Verlag in Berlin in 1842 and his son Ferdinand Springer grew it from a small firm of 4 employees into Germany's then second largest academic publisher with 65 staff in 1872.Chronology ". Springer Science+Business Media. In 1964, Springer expanded its business internationally, o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Algebraic Geometry (book)
''Algebraic Geometry'' is an algebraic geometry textbook written by Robin Hartshorne and published by Springer-Verlag in 1977.MathSciNet lists more than 2500 citations of this book. Importance It was the first extended treatment of scheme theory written as a text intended to be accessible to graduate students. Contents The first chapter, titled "Varieties", deals with the classical algebraic geometry of varieties over algebraically closed fields. This chapter uses many classical results in commutative algebra, including Hilbert's Nullstellensatz In mathematics, Hilbert's Nullstellensatz (German for "theorem of zeros," or more literally, "zero-locus-theorem") is a theorem that establishes a fundamental relationship between geometry and algebra. This relationship is the basis of algebraic ..., with the books by Atiyah–Macdonald, Matsumura, and Zariski–Samuel as usual references. The second and the third chapters, "Schemes" and "Cohomology", form the technical heart of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Moishezon Manifold
In mathematics, a Moishezon manifold is a compact complex manifold such that the field of meromorphic functions on each component has transcendence degree equal the complex dimension of the component: :\dim_\mathbfM=a(M)=\operatorname_\mathbf\mathbf(M). Complex algebraic varieties have this property, but the converse is not true: Hironaka's example gives a smooth 3-dimensional Moishezon manifold that is not an algebraic variety or scheme. showed that a Moishezon manifold is a projective algebraic variety if and only if it admits a Kähler metric. showed that any Moishezon manifold carries an algebraic space In mathematics, algebraic spaces form a generalization of the schemes of algebraic geometry, introduced by Michael Artin for use in deformation theory. Intuitively, schemes are given by gluing together affine schemes using the Zariski topology, w ... structure; more precisely, the category of Moishezon spaces (similar to Moishezon manifolds, but are allowed to have singul ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hodge Structure
In mathematics, a Hodge structure, named after W. V. D. Hodge, is an algebraic structure at the level of linear algebra, similar to the one that Hodge theory gives to the cohomology groups of a smooth and compact Kähler manifold. Hodge structures have been generalized for all complex varieties (even if they are singular and non-complete) in the form of mixed Hodge structures, defined by Pierre Deligne (1970). A variation of Hodge structure is a family of Hodge structures parameterized by a manifold, first studied by Phillip Griffiths (1968). All these concepts were further generalized to mixed Hodge modules over complex varieties by Morihiko Saito (1989). Hodge structures Definition of Hodge structures A pure Hodge structure of integer weight ''n'' consists of an abelian group H_ and a decomposition of its complexification ''H'' into a direct sum of complex subspaces H^, where p+q=n, with the property that the complex conjugate of H^ is H^: :H := H_\otimes_ \Complex = \bigop ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fujita Conjecture
In mathematics, Fujita's conjecture is a problem in the theories of algebraic geometry and complex manifolds, unsolved . It is named after Takao Fujita, who formulated it in 1985. Statement In complex geometry, the conjecture states that for a positive holomorphic line bundle ''L'' on a compact complex manifold ''M'', the line bundle ''K''''M'' ⊗ ''L''⊗''m'' (where ''K''''M'' is a canonical line bundle of ''M'') is * spanned by sections when ''m'' ≥ ''n'' + 1 ; * very ample when ''m'' ≥ ''n'' + 2, where ''n'' is the complex dimension of ''M''. Note that for large ''m'' the line bundle ''K''''M'' ⊗ ''L''⊗''m'' is very ample by the standard Serre's vanishing theorem (and its complex analytic variant). Fujita conjecture provides an explicit bound on ''m'', which is optimal for projective spaces. Known cases For surfaces the Fujita conjecture follows from Reider's theorem In algebraic geometry, Reider's theorem gives conditions for a line bundle on a projective surface ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Positive Form
In complex geometry, the term ''positive form'' refers to several classes of real differential forms of Hodge type ''(p, p)''. (1,1)-forms Real (''p'',''p'')-forms on a complex manifold ''M'' are forms which are of type (''p'',''p'') and real, that is, lie in the intersection \Lambda^(M)\cap \Lambda^(M,). A real (1,1)-form \omega is called semi-positive (sometimes just ''positive''), respectively, positive (or ''positive definite'') if any of the following equivalent conditions holds: #-\omega is the imaginary part of a positive semidefinite (respectively, positive definite) Hermitian form. #For some basis dz_1, ... dz_n in the space \Lambda^M of (1,0)-forms, \sqrt\omega can be written diagonally, as \sqrt\omega = \sum_i \alpha_i dz_i\wedge d\bar z_i, with \alpha_i real and non-negative (respectively, positive). #For any (1,0)-tangent vector v\in T^M, -\sqrt\omega(v, \bar v) \geq 0 (respectively, >0). #For any real tangent vector v\in TM, \omega(v, I(v)) \geq 0 (respectively, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
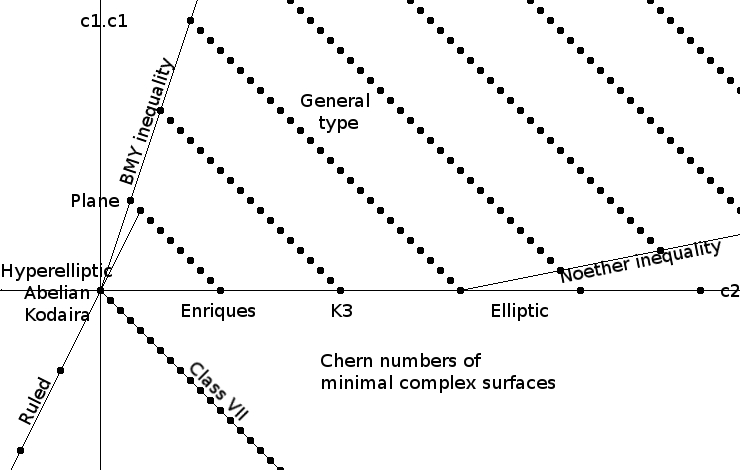
Enriques–Kodaira Classification
In mathematics, the Enriques–Kodaira classification is a classification of compact complex surfaces into ten classes. For each of these classes, the surfaces in the class can be parametrized by a moduli space. For most of the classes the moduli spaces are well understood, but for the class of surfaces of general type the moduli spaces seem too complicated to describe explicitly, though some components are known. Max Noether began the systematic study of algebraic surfaces, and Guido Castelnuovo proved important parts of the classification. described the classification of complex projective surfaces. later extended the classification to include non-algebraic compact surfaces. The analogous classification of surfaces in positive characteristic was begun by and completed by ; it is similar to the characteristic 0 projective case, except that one also gets singular and supersingular Enriques surfaces in characteristic 2, and quasi-hyperelliptic surfaces in characteristics 2 and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Algebraic Variety
Algebraic varieties are the central objects of study in algebraic geometry, a sub-field of mathematics. Classically, an algebraic variety is defined as the set of solutions of a system of polynomial equations over the real or complex numbers. Modern definitions generalize this concept in several different ways, while attempting to preserve the geometric intuition behind the original definition. Conventions regarding the definition of an algebraic variety differ slightly. For example, some definitions require an algebraic variety to be irreducible, which means that it is not the union of two smaller sets that are closed in the Zariski topology. Under this definition, non-irreducible algebraic varieties are called algebraic sets. Other conventions do not require irreducibility. The fundamental theorem of algebra establishes a link between algebra and geometry by showing that a monic polynomial (an algebraic object) in one variable with complex number coefficients is determined ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
