|
Joseph Louis François Bertrand
Joseph Louis François Bertrand (; 11 March 1822 – 5 April 1900) was a French mathematician who worked in the fields of number theory, differential geometry, probability theory, economics and thermodynamics. Biography Joseph Bertrand was the son of physician Alexandre Jacques François Bertrand and the brother of archaeologist Alexandre Bertrand. His father died when Joseph was only nine years old, but that did not stand in his way of learning and understanding algebraic and elementary geometric concepts, and he also could speak Latin fluently, all when he was of the same age of nine. At eleven years old he attended the course of the École Polytechnique as an auditor (open courses). From age eleven to seventeen, he obtained two bachelor's degrees, a license and a PhD with a thesis on the mathematical theory of electricity and is admitted first to the 1839 entrance examination of the École Polytechnique. Bertrand was a professor at the École Polytechnique and Collège de Fra ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Paris
Paris () is the capital and most populous city of France, with an estimated population of 2,165,423 residents in 2019 in an area of more than 105 km² (41 sq mi), making it the 30th most densely populated city in the world in 2020. Since the 17th century, Paris has been one of the world's major centres of finance, diplomacy, commerce, fashion, gastronomy, and science. For its leading role in the arts and sciences, as well as its very early system of street lighting, in the 19th century it became known as "the City of Light". Like London, prior to the Second World War, it was also sometimes called the capital of the world. The City of Paris is the centre of the Île-de-France region, or Paris Region, with an estimated population of 12,262,544 in 2019, or about 19% of the population of France, making the region France's primate city. The Paris Region had a GDP of €739 billion ($743 billion) in 2019, which is the highest in Europe. According to the Economis ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Game Theory
Game theory is the study of mathematical models of strategic interactions among rational agents. Myerson, Roger B. (1991). ''Game Theory: Analysis of Conflict,'' Harvard University Press, p.&nbs1 Chapter-preview links, ppvii–xi It has applications in all fields of social science, as well as in logic, systems science and computer science. Originally, it addressed two-person zero-sum games, in which each participant's gains or losses are exactly balanced by those of other participants. In the 21st century, game theory applies to a wide range of behavioral relations; it is now an umbrella term for the science of logical decision making in humans, animals, as well as computers. Modern game theory began with the idea of mixed-strategy equilibria in two-person zero-sum game and its proof by John von Neumann. Von Neumann's original proof used the Brouwer fixed-point theorem on continuous mappings into compact convex sets, which became a standard method in game theory and m ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Least Squares
The method of least squares is a standard approach in regression analysis to approximate the solution of overdetermined systems (sets of equations in which there are more equations than unknowns) by minimizing the sum of the squares of the residuals (a residual being the difference between an observed value and the fitted value provided by a model) made in the results of each individual equation. The most important application is in data fitting. When the problem has substantial uncertainties in the independent variable (the ''x'' variable), then simple regression and least-squares methods have problems; in such cases, the methodology required for fitting errors-in-variables models may be considered instead of that for least squares. Least squares problems fall into two categories: linear or ordinary least squares and nonlinear least squares, depending on whether or not the residuals are linear in all unknowns. The linear least-squares problem occurs in statistical regr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Royal Swedish Academy Of Sciences
The Royal Swedish Academy of Sciences ( sv, Kungliga Vetenskapsakademien) is one of the royal academies of Sweden. Founded on 2 June 1739, it is an independent, non-governmental scientific organization that takes special responsibility for promoting natural sciences and mathematics and strengthening their influence in society, whilst endeavouring to promote the exchange of ideas between various disciplines. The goals of the academy are: * to be a forum where researchers meet across subject boundaries, * to offer a unique environment for research, * to provide support to younger researchers, * to reward outstanding research efforts, * to communicate internationally among scientists, * to advance the case for science within society and to influence research policy priorities * to stimulate interest in mathematics and science in school, and * to disseminate and popularize scientific information in various forms. Every year, the academy awards the Nobel Prizes in physics and chem ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Reversible Process (thermodynamics)
In thermodynamics, a reversible process is a process, involving a system and its surroundings, whose direction can be reversed by infinitesimal changes in some properties of the surroundings, such as pressure or temperature. Throughout an entire reversible process, the system is in thermodynamic equilibrium, both physical and chemical, and ''nearly'' in pressure and temperature equilibrium with its surroundings. This prevents unbalanced forces and acceleration of moving system boundaries, which in turn avoids friction and other dissipation. To maintain equilibrium, reversible processes are extremely slow ( ''quasistatic''). The process must occur slowly enough that after some small change in a thermodynamic parameter, the physical processes in the system have enough time for the other parameters to self-adjust to match the new, changed parameter value. For example, if a container of water has sat in a room long enough to match the steady temperature of the surrounding air, fo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
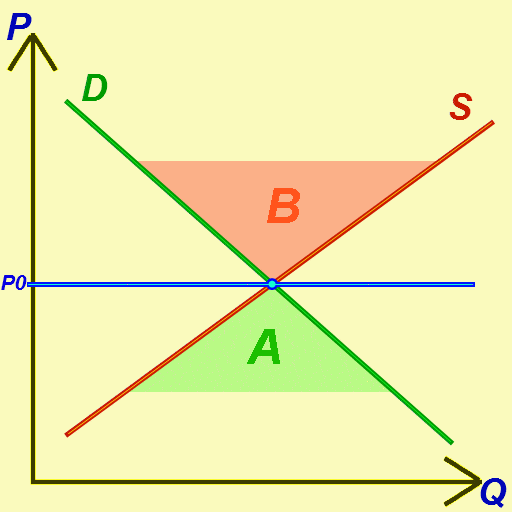
Economic Equilibrium
In economics, economic equilibrium is a situation in which economic forces such as supply and demand are balanced and in the absence of external influences the ( equilibrium) values of economic variables will not change. For example, in the standard text perfect competition, equilibrium occurs at the point at which quantity demanded and quantity supplied are equal. Market equilibrium in this case is a condition where a market price is established through competition such that the amount of goods or services sought by buyers is equal to the amount of goods or services produced by sellers. This price is often called the competitive price or market clearing price and will tend not to change unless demand or supply changes, and quantity is called the "competitive quantity" or market clearing quantity. But the concept of ''equilibrium'' in economics also applies to imperfectly competitive markets, where it takes the form of a Nash equilibrium. Understanding economic equilib ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bertrand Competition
Bertrand competition is a model of competition used in economics, named after Joseph Louis François Bertrand (1822–1900). It describes interactions among firms (sellers) that set prices and their customers (buyers) that choose quantities at the prices set. The model was formulated in 1883 by Bertrand in a review of Antoine Augustin Cournot's book ''Recherches sur les Principes Mathématiques de la Théorie des Richesses'' (1838) in which Cournot had put forward the Cournot model. Cournot's model argued that each firm should maximise its profit by selecting a quantity level and then adjusting price level to sell that quantity. The outcome of the model equilibrium involved firms pricing above marginal cost; hence, the competitive price. In his review, Bertrand argued that each firm should instead maximise its profits by selecting a price level that undercuts its competitors' prices, when their prices exceed marginal cost. The model was not formalized by Bertrand; however, the ide ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Antoine Augustin Cournot
Antoine Augustin Cournot (; 28 August 180131 March 1877) was a French philosopher and mathematician who also contributed to the development of economics. Biography Antoine Augustin Cournot was born at Gray, Haute-Saône. In 1821 he entered one of the most prestigious Grandes Écoles, the École Normale Supérieure, and, according to Sandmo: in 1823 he took a license degree in mathematics at Sorbonne University. He then became the private secretary of a field marshal who required assistance in writing his memoirs. This position left Cournot with considerable time for his own pursuits. In the course of his ten years in the field marshal's employment he took two doctoral degrees, one in mechanics and one in astronomy. In addition, he published a number of articles and even acquired a degree in law. Subsequently, Cournot held positions as professor of mathematics, chief examiner for undergraduate students, and rector of Dijon Academy. By the time Cournot died in 1877, he was ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cournot Competition
Cournot competition is an economic model used to describe an industry structure in which companies compete on the amount of output they will produce, which they decide on independently of each other and at the same time. It is named after Antoine Augustin Cournot (1801–1877) who was inspired by observing competition in a spring water duopoly. It has the following features: * There is more than one firm and all firms produce a homogeneous product, i.e., there is no product differentiation; * Firms do not cooperate, i.e., there is no collusion; * Firms have market power, i.e., each firm's output decision affects the good's price; * The number of firms is fixed; * Firms compete in quantities rather than prices; and * The firms are economically rational and act strategically, usually seeking to maximize profit given their competitors' decisions. An essential assumption of this model is the "not conjecture" that each firm aims to maximize profits, based on the expectation that its own ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Oligopoly
An oligopoly (from Greek ὀλίγος, ''oligos'' "few" and πωλεῖν, ''polein'' "to sell") is a market structure in which a market or industry is dominated by a small number of large sellers or producers. Oligopolies often result from the desire to maximize profits, which can lead to collusion between companies. This reduces competition, increases prices for consumers, and lowers wages for employees. Many industries have been cited as oligopolistic, including civil aviation, electricity providers, the telecommunications sector, Rail freight markets, food processing, funeral services, sugar refining, beer making, pulp and paper making, and automobile manufacturing. Most countries have laws outlawing anti-competitive behavior. EU competition law prohibits anti-competitive practices such as price-fixing and manipulating market supply and trade among competitors. In the US, the United States Department of Justice Antitrust Division and the Federal Trade Commiss ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Method Of Least Squares
The method of least squares is a standard approach in regression analysis to approximate the solution of overdetermined systems (sets of equations in which there are more equations than unknowns) by minimizing the sum of the squares of the residuals (a residual being the difference between an observed value and the fitted value provided by a model) made in the results of each individual equation. The most important application is in data fitting. When the problem has substantial uncertainties in the independent variable (the ''x'' variable), then simple regression and least-squares methods have problems; in such cases, the methodology required for fitting errors-in-variables models may be considered instead of that for least squares. Least squares problems fall into two categories: linear or ordinary least squares and nonlinear least squares, depending on whether or not the residuals are linear in all unknowns. The linear least-squares problem occurs in statistical regressio ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Theory Of Errors
In statistics, propagation of uncertainty (or propagation of error) is the effect of variables' uncertainties (or errors, more specifically random errors) on the uncertainty of a function based on them. When the variables are the values of experimental measurements they have uncertainties due to measurement limitations (e.g., instrument precision) which propagate due to the combination of variables in the function. The uncertainty ''u'' can be expressed in a number of ways. It may be defined by the absolute error . Uncertainties can also be defined by the relative error , which is usually written as a percentage. Most commonly, the uncertainty on a quantity is quantified in terms of the standard deviation, , which is the positive square root of the variance. The value of a quantity and its error are then expressed as an interval . If the statistical probability distribution of the variable is known or can be assumed, it is possible to derive confidence limits to describe th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |