|
Intercept Method
In astronomical navigation, the intercept method, also known as Marcq St. Hilaire method, is a method of calculating an observer's position on earth (geopositioning). It was originally called the ''azimuth intercept'' method because the process involves drawing a line which intercepts the azimuth line. This name was shortened to ''intercept'' method and the ''intercept distance'' was shortened to 'intercept'. The method yields a line of position (LOP) on which the observer is situated. The intersection of two or more such lines will define the observer's position, called a "fix". Sights may be taken at short intervals, usually during hours of twilight, or they may be taken at an interval of an hour or more (as in observing the Sun during the day). In either case, the lines of position, if taken at different times, must be advanced or retired to correct for the movement of the ship during the interval between observations. If observations are taken at short intervals, a few minutes ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Astronomical Navigation
Celestial navigation, also known as astronavigation, is the practice of position fixing using stars and other celestial bodies that enables a navigator to accurately determine their actual current physical position in space (or on the surface of the Earth) without having to rely solely on estimated positional calculations, commonly known as "dead reckoning", made in the absence of satellite navigation or other similar modern electronic or digital positioning means. Celestial navigation uses "sights", or timed angular measurements, taken typically between a celestial body (e.g. the Sun, the Moon, a planet, or a star) and the visible horizon. Celestial navigation can also take advantage of measurements between celestial bodies without reference to the Earth horizon, such as when the Moon and other selected bodies are used in the practice called "lunars" or lunar distance method, used for determining precise time when time is unknown. Celestial navigation by taking sights of the Su ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Haversine
The versine or versed sine is a trigonometric function found in some of the earliest (Sanskrit ''Aryabhatia'', Section I) s. The versine of an angle is 1 minus its cosine. There are several related functions, most notably the coversine and haversine. The latter, half a versine, is of particular importance in the of navigation. Overview The versine[...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Haversine Formula
The haversine formula determines the great-circle distance between two points on a sphere given their longitudes and latitudes. Important in navigation, it is a special case of a more general formula in spherical trigonometry, the law of haversines, that relates the sides and angles of spherical triangles. The first table of haversines in English was published by James Andrew in 1805, but Florian Cajori credits an earlier use by José de Mendoza y Ríos in 1801. (NB. ISBN and link for reprint of second edition by Cosimo, Inc., New York, 2013.) The term ''haversine'' was coined in 1835 by James Inman. (Fourth edition) These names follow from the fact that they are customarily written in terms of the haversine function, given by . The formulas could equally be written in terms of any multiple of the haversine, such as the older versine function (twice the haversine). Prior to the advent of computers, the elimination of division and multiplication by factors of two proved convenient ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Longitude
Longitude (, ) is a geographic coordinate that specifies the east–west position of a point on the surface of the Earth, or another celestial body. It is an angular measurement, usually expressed in degrees and denoted by the Greek letter lambda (λ). Meridians are semicircular lines running from pole to pole that connect points with the same longitude. The prime meridian defines 0° longitude; by convention the International Reference Meridian for the Earth passes near the Royal Observatory in Greenwich, England on the island of Great Britain. Positive longitudes are east of the prime meridian, and negative ones are west. Because of the Earth's rotation, there is a close connection between longitude and time measurement. Scientifically precise local time varies with longitude: a difference of 15° longitude corresponds to a one-hour difference in local time, due to the differing position in relation to the Sun. Comparing local time to an absolute measure of time allows ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Latitude
In geography, latitude is a coordinate that specifies the north– south position of a point on the surface of the Earth or another celestial body. Latitude is given as an angle that ranges from –90° at the south pole to 90° at the north pole, with 0° at the Equator. Lines of constant latitude, or ''parallels'', run east–west as circles parallel to the equator. Latitude and ''longitude'' are used together as a coordinate pair to specify a location on the surface of the Earth. On its own, the term "latitude" normally refers to the ''geodetic latitude'' as defined below. Briefly, the geodetic latitude of a point is the angle formed between the vector perpendicular (or ''normal'') to the ellipsoidal surface from the point, and the plane of the equator. Background Two levels of abstraction are employed in the definitions of latitude and longitude. In the first step the physical surface is modeled by the geoid, a surface which approximates the mean sea level over the ocean ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Navigation
Navigation is a field of study that focuses on the process of monitoring and controlling the movement of a craft or vehicle from one place to another.Bowditch, 2003:799. The field of navigation includes four general categories: land navigation, marine navigation, aeronautic navigation, and space navigation. It is also the term of art used for the specialized knowledge used by navigators to perform navigation tasks. All navigational techniques involve locating the navigator's position compared to known locations or patterns. Navigation, in a broader sense, can refer to any skill or study that involves the determination of position and direction. In this sense, navigation includes orienteering and pedestrian navigation. History In the European medieval period, navigation was considered part of the set of '' seven mechanical arts'', none of which were used for long voyages across open ocean. Polynesian navigation is probably the earliest form of open-ocean navigation; it was ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Intersection (land Navigation)
Position resection and intersection are methods for determining an unknown geographic position ( position finding) by measuring angles with respect to known positions. In ''resection'', the one point with unknown coordinates is occupied and sightings are taken to the known points; in ''intersection'', the two points with known coordinates are occupied and sightings are taken to the unknown point. Measurements can be made with a compass and topographic map (or nautical chart), theodolite or with a total station using known points of a geodetic network or landmarks of a map. Resection versus intersection Resection and its related method, ''intersection'', are used in surveying as well as in general land navigation (including inshore marine navigation using shore-based landmarks). Both methods involve taking azimuths or bearings to two or more objects, then drawing ''lines of position'' along those recorded bearings or azimuths. When intersecting, lines of position are used to fix th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Intersection (air Navigation)
In aviation, an intersection is a virtual navigational fix that helps aircraft maintain their flight plan. It is usually defined as the intersection (in the geometrical sense) of two VOR radials. They are usually identified as major airway intersections where aircraft, operating under instrument flight rules, often change direction of flight while en route. According to the Federal Aviation Regulations, some intersections are designated as mandatory reporting points for pilots who are not in radar contact with air traffic control. Intersections also play an important role in departure and approach procedures. All intersections have an alphabetical or alphanumeric designation. Near major airports, the intersection designation code typically consists of three letters followed by the runway number. Most other intersection designations consist of five-letter combinations that are either pronounceable or chosen for their mnemonic value, since either air traffic control or the fligh ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
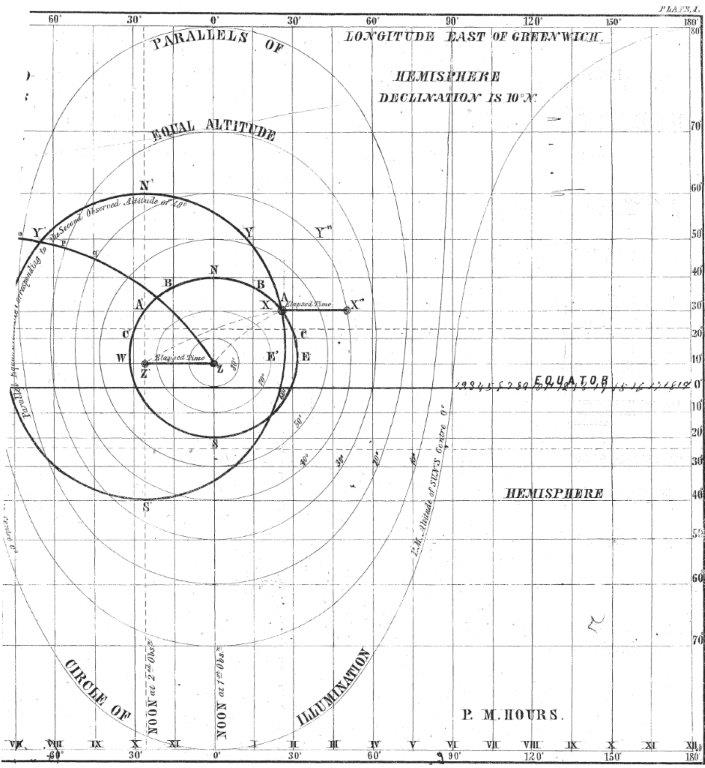
Sight Reduction
In astronavigation, sight reduction is the process of deriving from a sight, (in celestial navigation usually obtained using a sextant), the information needed for establishing a line of position, generally by intercept method. Sight is defined as the observation of the altitude, and sometimes also the azimuth, of a celestial body for a line of position; or the data obtained by such observation. The mathematical basis of sight reduction is the circle of equal altitude. The calculation can be done by computer, or by hand via tabular methods and longhand methods. Algorithm Given: * Lat, the latitude (North - positive, South - negative), Lon the longitude (East - positive, West - negative), both approximate (assumed); * Dec, the declination of the body observed; * GHA, the Greenwich hour angle of the body observed; * LHA = GHA + Lon, the local hour angle of the body observed. First calculate the altitude of the celestial body Hc using the equation of circle of equal altitude: \s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Circle Of Equal Altitude
The circle of equal altitude, also called circle of position (CoP), is defined as the locus of points on Earth on which an observer sees a celestial object such as the sun or a star, at a given time, with the same observed altitude. It was discovered by the American sea-captain Thomas Hubbard Sumner in 1837, published in 1843 and is the basis of an important method in celestial navigation Discovery Sumner discovered the line on a voyage from South Carolina to Greenock in Scotland in 1837. On December 17, as he was nearing the coast of Wales, he was uncertain of his position after several days of cloudy weather and no sights. A momentary opening in the clouds allowed him to determine the altidude of the sun. This, together with the chronometer time and the latitude enabled him to calculate the longitude. But he was not confident of his latitude, which depended on dead reckoning (DR). So he calculated longitude using his DR value and two more values of latitude 10' and 20' to the no ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Celestial Navigation
Celestial navigation, also known as astronavigation, is the practice of position fixing using stars and other celestial bodies that enables a navigator to accurately determine their actual current physical position in space (or on the surface of the Earth) without having to rely solely on estimated positional calculations, commonly known as "dead reckoning", made in the absence of satellite navigation or other similar modern electronic or digital positioning means. Celestial navigation uses "sights", or timed angular measurements, taken typically between a celestial body (e.g. the Sun, the Moon, a planet, or a star) and the visible horizon. Celestial navigation can also take advantage of measurements between celestial bodies without reference to the Earth horizon, such as when the Moon and other selected bodies are used in the practice called "lunars" or lunar distance method, used for determining precise time when time is unknown. Celestial navigation by taking sights of the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Horizon
The horizon is the apparent line that separates the surface of a celestial body from its sky when viewed from the perspective of an observer on or near the surface of the relevant body. This line divides all viewing directions based on whether it intersects the relevant body's surface or not. The ''true horizon'' is a theoretical line, which can only be observed to any degree of accuracy when it lies along a relatively smooth surface such as that of Earth's oceans. At many locations, this line is obscured by terrain, and on Earth it can also be obscured by life forms such as trees and/or human constructs such as buildings. The resulting intersection of such obstructions with the sky is called the ''visible horizon''. On Earth, when looking at a sea from a shore, the part of the sea closest to the horizon is called the offing. Pronounced, "Hor-I-zon". The true horizon surrounds the observer and it is typically assumed to be a circle, drawn on the surface of a perfectly spherica ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
