|
Identity (music)
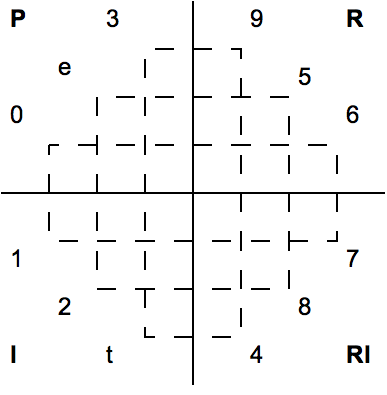
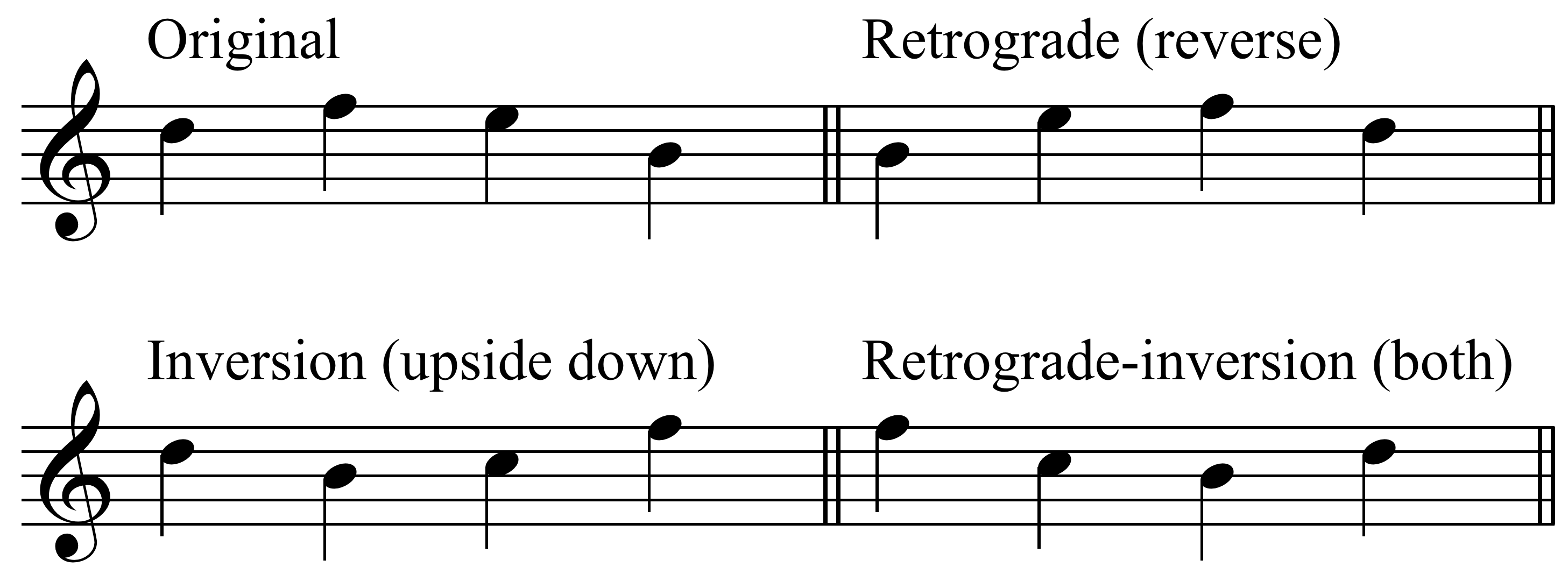
In post-tonal music theory, identity is similar to identity in universal algebra. An identity function is a permutation or transformation which transforms a pitch or pitch class set into itself. Generally this requires symmetry. For instance, inverting an augmented triad or C4 interval cycle, 048, produces itself. Performing a retrograde operation upon the tone row 01210 produces 01210. Doubling the length of a rhythm while doubling the tempo produces a rhythm of the same durations as the original. In addition to being a property of a specific set, identity is, by extension, the "family" of sets or set forms which satisfy a possible identity. These families are defined by symmetry, which means that an object is invariant to any of various transformations; including reflection and rotation. George Perle provides the following example:Perle, George (1995). ''The Right Notes: Twenty-Three Selected Essays by George Perle on Twentieth-Century Music'', p.237-238. . :"C-E, D-F, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Augmented Chord In The Chromatic Circle
Augment or augmentation may refer to: Language *Augment (Indo-European), a syllable added to the beginning of the word in certain Indo-European languages *Augment (Bantu languages), a morpheme that is prefixed to the noun class prefix of nouns in certain Bantu languages *Augment, a name sometimes given to the verbal ''ō-'' prefix in Nahuatl grammar Technology * Augmentation (obstetrics), the process by which the first and/or second stages of an already established labour is accelerated or potentiated by deliberate and artificial means *Augmentation (pharmacology), the combination of two or more drugs to achieve better treatment results *Augmented reality, a live view of a physical, real-world environment whose elements are ''augmented'' by computer-generated sensory input *Augmented cognition, a research field that aims at creating revolutionary human-computer interactions * Augment (Tymshare), a hypertext system derived from Douglas Engelbart's oN-Line System, renamed "Augment ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Interval Cycle
In music, an interval cycle is a collection of pitch classes created from a sequence of the same interval class.Whittall, Arnold. 2008. ''The Cambridge Introduction to Serialism'', p. 273-74. New York: Cambridge University Press. (pbk). In other words, a collection of pitches by starting with a certain note and going up by a certain interval until the original note is reached (e.g. starting from C, going up by 3 semitones repeatedly until eventually C is again reached - the cycle is the collection of all the notes met on the way). In other words, interval cycles "unfold a single recurrent interval in a series that closes with a return to the initial pitch class". See: wikt:cycle. Interval cycles are notated by George Perle using the letter "C" (for ''cycle''), with an interval class integer to distinguish the interval. Thus the diminished seventh chord would be C3 and the augmented triad would be C4. A superscript may be added to distinguish between transpositions, using ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Derived Row
In music using the twelve-tone technique, derivation is the construction of a row through segments. A derived row is a tone row whose entirety of twelve tones is constructed from a segment or portion of the whole, the generator. Anton Webern often used derived rows in his pieces. A partition is a segment created from a set through partitioning. Derivation Rows may be derived from a sub- set of any number of pitch classes that is a divisor of 12, the most common being the first three pitches or a trichord. This segment may then undergo transposition, inversion, retrograde, or any combination to produce the other parts of the row (in this case, the other three segments). One of the side effects of derived rows is invariance. For example, since a segment may be equivalent to the generating segment inverted and transposed, say, 6 semitones, when the entire row is inverted and transposed six semitones the generating segment will now consist of the pitch classes of the derived segment ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Point Reflection
In geometry, a point reflection (point inversion, central inversion, or inversion through a point) is a type of isometry of Euclidean space. An object that is invariant under a point reflection is said to possess point symmetry; if it is invariant under point reflection through its center, it is said to possess central symmetry or to be centrally symmetric. Point reflection can be classified as an affine transformation. Namely, it is an isometric involutive affine transformation, which has exactly one fixed point, which is the point of inversion. It is equivalent to a homothetic transformation with scale factor equal to −1. The point of inversion is also called homothetic center. Terminology The term ''reflection'' is loose, and considered by some an abuse of language, with ''inversion'' preferred; however, ''point reflection'' is widely used. Such maps are involutions, meaning that they have order 2 – they are their own inverse: applying them twice yields the identi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Klumpenhouwer Network
A Klumpenhouwer Network, named after its inventor, Canadian music theorist and former doctoral student of David Lewin's at Harvard, Henry Klumpenhouwer, is "any network that uses T and/or I operations ( transposition or inversion) to interpret interrelations among pcs" (pitch class sets).Lewin, David (1990). "Klumpenhouwer Networks and Some Isographies That Involve Them", p. 84, ''Music Theory Spectrum'', vol. 12, no. 1 (Spring), pp. 83–120. According to George Perle, "a Klumpenhouwer network is a chord analyzed in terms of its dyadic sums and differences," and "this kind of analysis of triadic combinations was implicit in," his "concept of the cyclic set from the beginning", Perle, George (1993). "Letter from George Perle", ''Music Theory Spectrum'', vol. 15, no. 2 (Autumn), pp. 300–303. cyclic sets being those " sets whose alternate elements unfold complementary cycles of a single interval." "Klumpenhouwer's idea, both simple and profound in its implications, is to ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rotational Symmetry
Rotational symmetry, also known as radial symmetry in geometry, is the property a shape has when it looks the same after some rotation by a partial turn. An object's degree of rotational symmetry is the number of distinct orientations in which it looks exactly the same for each rotation. Certain geometric objects are partially symmetrical when rotated at certain angles such as squares rotated 90°, however the only geometric objects that are fully rotationally symmetric at any angle are spheres, circles and other spheroids. Formal treatment Formally the rotational symmetry is symmetry with respect to some or all rotations in ''m''-dimensional Euclidean space. Rotations are direct isometries, i.e., isometries preserving orientation. Therefore, a symmetry group of rotational symmetry is a subgroup of ''E''+(''m'') (see Euclidean group). Symmetry with respect to all rotations about all points implies translational symmetry with respect to all translations, so space is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Reflection Symmetry
In mathematics, reflection symmetry, line symmetry, mirror symmetry, or mirror-image symmetry is symmetry with respect to a reflection. That is, a figure which does not change upon undergoing a reflection has reflectional symmetry. In 2D there is a line/axis of symmetry, in 3D a plane of symmetry. An object or figure which is indistinguishable from its transformed image is called mirror symmetric. In conclusion, a line of symmetry splits the shape in half and those halves should be identical. Symmetric function In formal terms, a mathematical object is symmetric with respect to a given operation such as reflection, rotation or translation, if, when applied to the object, this operation preserves some property of the object. The set of operations that preserve a given property of the object form a group. Two objects are symmetric to each other with respect to a given group of operations if one is obtained from the other by some of the operations (and vice versa). The ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Interval (music)
In music theory, an interval is a difference in pitch between two sounds. An interval may be described as horizontal, linear, or melodic if it refers to successively sounding tones, such as two adjacent pitches in a melody, and vertical or harmonic if it pertains to simultaneously sounding tones, such as in a chord. In Western music, intervals are most commonly differences between notes of a diatonic scale. Intervals between successive notes of a scale are also known as scale steps. The smallest of these intervals is a semitone. Intervals smaller than a semitone are called microtones. They can be formed using the notes of various kinds of non-diatonic scales. Some of the very smallest ones are called commas, and describe small discrepancies, observed in some tuning systems, between enharmonically equivalent notes such as C and D. Intervals can be arbitrarily small, and even imperceptible to the human ear. In physical terms, an interval is the ratio between two sonic fr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
George Perle
George Perle (6 May 1915 – 23 January 2009) was an American composer and music theorist. As a composer, his music was largely atonal, using methods similar to the twelve-tone technique of the Second Viennese School. This serialist style, and atonality in general, was the subject of much of his theoretical writings. His 1962 book, ''Serial Composition and Atonality: An Introduction to the Music of Schoenberg, Berg, and Webern'' remains a standard text for 20th-century classical music theory. Among Perle's awards was the 1986 Pulitzer Prize for Music for his Wind Quintet No. 4. Life and career Perle was born in Bayonne, New Jersey. He graduated from DePaul University, where he studied with Wesley LaViolette and received private lessons from Ernst Krenek. Later, he served as a technician fifth grade in the United States Army during World War II. He earned his doctorate at New York University in 1956. Perle composed with a technique of his own devising called "twelve-t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Set (music)
A set (pitch set, pitch-class set, set class, set form, set genus, pitch collection) in music theory, as in mathematics and general parlance, is a collection of objects. In musical contexts the term is traditionally applied most often to collections of pitches or pitch-classes, but theorists have extended its use to other types of musical entities, so that one may speak of sets of durations or timbres, for example.Wittlich, Gary (1975). "Sets and Ordering Procedures in Twentieth-Century Music", ''Aspects of Twentieth-Century Music'', p.475. Wittlich, Gary (ed.). Englewood Cliffs, New Jersey: Prentice-Hall. . A set by itself does not necessarily possess any additional structure, such as an ordering or permutation. Nevertheless, it is often musically important to consider sets that are equipped with an order relation (called ''segments''); in such contexts, bare sets are often referred to as "unordered", for the sake of emphasis. Two-element sets are called dyads, three- ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Augmented Triad
Augment or augmentation may refer to: Language *Augment (Indo-European), a syllable added to the beginning of the word in certain Indo-European languages * Augment (Bantu languages), a morpheme that is prefixed to the noun class prefix of nouns in certain Bantu languages *Augment, a name sometimes given to the verbal ''ō-'' prefix in Nahuatl grammar Technology * Augmentation (obstetrics), the process by which the first and/or second stages of an already established labour is accelerated or potentiated by deliberate and artificial means *Augmentation (pharmacology), the combination of two or more drugs to achieve better treatment results *Augmented reality, a live view of a physical, real-world environment whose elements are ''augmented'' by computer-generated sensory input * Augmented cognition, a research field that aims at creating revolutionary human-computer interactions * Augment (Tymshare), a hypertext system derived from Douglas Engelbart's oN-Line System, renamed "Augme ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Post-tonal Music Theory
Post-tonal music theory is the set of theories put forward to describe music written outside of, or 'after', the tonal system of the common practice period. It revolves around the idea of 'emancipating dissonance', that is, freeing the structure of music from the familiar harmonic patterns that are derived from natural overtones. As music becomes more complex, dissonance becomes indistinguishable from consonance. Overview In the latter part of the 19th century, composers began to move away from the tonal system. This is typified in Richard Wagner's music, especially ''Tristan und Isolde'' (the Tristan chord, for example). Arnold Schoenberg and his pupil Anton Webern proposed a theory on the emancipation of the dissonance to help analyse the general trend and, in particular, their own atonal music. Composers such as Charles Ives, Dane Rudhyar, and even Duke Ellington and Lou Harrison, connected the emancipation of the dissonance with the emancipation of society and humanity. The ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |