|
Geometric Exercises In Paper Folding
''Geometric Exercises in Paper Folding'' is a book on the mathematics of paper folding. It was written by Indian mathematician T. Sundara Row, first published in India in 1893, and later republished in many other editions. Its topics include paper constructions for regular polygons, symmetry, and algebraic curves. According to historian of mathematics Michael Friedman, it became "one of the main engines of the popularization of folding as a mathematical activity". Publication history ''Geometric Exercises in Paper Folding'' was first published by Addison & Co. in Madras in 1893. The book became known in Europe through a remark of Felix Klein in his book ''Vorträge über ausgewählte Fragen der Elementargeometrie'' (1895) and its translation ''Famous Problems Of Elementary Geometry'' (1897). Based on the success of ''Geometric Exercises in Paper Folding'' in Germany, the Open Court Press of Chicago published it in the US, with updates by Wooster Woodruff Beman and David Eugene Smi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematics Of Paper Folding
The discipline of origami or paper folding has received a considerable amount of mathematical study. Fields of interest include a given paper model's flat-foldability (whether the model can be flattened without damaging it), and the use of paper folds to solve up-to cubic mathematical equations. Computational origami is a recent branch of computer science that is concerned with studying algorithms that solve paper-folding problems. The field of computational origami has also grown significantly since its inception in the 1990s with Robert Lang's TreeMaker algorithm to assist in the precise folding of bases. Computational origami results either address origami design or origami foldability."Lecture: Recent Results in Computational Origami". ''Origami USA: We are the American national society devoted to origami, the art of paperfolding''. Retrieved 2022-05-08. In origami design problems, the goal is to design an object that can be folded out of paper given a specific target configura ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Collineation
In projective geometry, a collineation is a one-to-one and onto map (a bijection) from one projective space to another, or from a projective space to itself, such that the images of collinear points are themselves collinear. A collineation is thus an ''isomorphism'' between projective spaces, or an automorphism from a projective space to itself. Some authors restrict the definition of collineation to the case where it is an automorphism. The set of all collineations of a space to itself form a group, called the collineation group. Definition Simply, a collineation is a one-to-one map from one projective space to another, or from a projective space to itself, such that the images of collinear points are themselves collinear. One may formalize this using various ways of presenting a projective space. Also, the case of the projective line is special, and hence generally treated differently. Linear algebra For a projective space defined in terms of linear algebra (as the projectiviza ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Squared Triangular Number
In number theory, the sum of the first cubes is the square of the th triangular number. That is, :1^3+2^3+3^3+\cdots+n^3 = \left(1+2+3+\cdots+n\right)^2. The same equation may be written more compactly using the mathematical notation for summation: :\sum_^n k^3 = \bigg(\sum_^n k\bigg)^2. This identity is sometimes called Nicomachus's theorem, after Nicomachus of Gerasa (c. 60 – c. 120 CE). History Nicomachus, at the end of Chapter 20 of his ''Introduction to Arithmetic'', pointed out that if one writes a list of the odd numbers, the first is the cube of 1, the sum of the next two is the cube of 2, the sum of the next three is the cube of 3, and so on. He does not go further than this, but from this it follows that the sum of the first cubes equals the sum of the first n(n+1)/2 odd numbers, that is, the odd numbers from 1 to n(n+1)-1. The average of these numbers is obviously n(n+1)/2, and there are n(n+1)/2 of them, so their sum is \bigl(n(n+1)/2\bigr)^2. Many early mathem ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Gnomon (figure)
In geometry, a gnomon is a plane figure formed by removing a similar parallelogram from a corner of a larger parallelogram; or, more generally, a figure that, added to a given figure, makes a larger figure of the same shape. Building figurate numbers Figurate numbers were a concern of Pythagorean mathematics, and Pythagoras is credited with the notion that these numbers are generated from a ''gnomon'' or basic unit. The gnomon is the piece which needs to be added to a figurate number to transform it to the next bigger one. For example, the gnomon of the square number is the odd number, of the general form 2''n'' + 1, ''n'' = 1, 2, 3, ... . The square of size 8 composed of gnomons looks like this: ~~~~~~~~\begin 8&8&8&8&8&8&8&8\\ 8&7&7&7&7&7&7&7\\ 8&7&6&6&6&6&6&6\\ 8&7&6&5&5&5&5&5\\ 8&7&6&5&4&4&4&4\\ 8&7&6&5&4&3&3&3\\ 8&7&6&5&4&3&2&2\\ 8&7&6&5&4&3&2&1 \end To transform from the ''n-square'' (the square of size ''n'') to the (''n'' + 1)-square, one adjoins 2''n'' + 1 elements: one ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cassini Oval
In geometry, a Cassini oval is a quartic plane curve defined as the locus of points in the plane such that the product of the distances to two fixed points ( foci) is constant. This may be contrasted with an ellipse, for which the ''sum'' of the distances is constant, rather than the product. Cassini ovals are the special case of polynomial lemniscates when the polynomial used has degree 2. Cassini ovals are named after the astronomer Giovanni Domenico Cassini who studied them in the late 17th century. Cassini believed that the Sun traveled around the Earth on one of these ovals, with the Earth at one focus of the oval. Other names include Cassinian ovals, Cassinian curves and ovals of Cassini. Formal definition A Cassini oval is a set of points, such that for any point P of the set, the ''product'' of the distances , PP_1, ,\, , PP_2, to two fixed points P_1, P_2 is a constant, usually written as b^2 where b > 0: :\\ . As with an ellipse, the fixed points P_1,P_2 are ca ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cissoid Of Diocles
In geometry, the cissoid of Diocles (; named for Diocles) is a cubic plane curve notable for the property that it can be used to construct two mean proportionals to a given ratio. In particular, it can be used to double a cube. It can be defined as the cissoid of a circle and a line tangent to it with respect to the point on the circle opposite to the point of tangency. In fact, the curve family of cissoids is named for this example and some authors refer to it simply as ''the'' cissoid. It has a single cusp at the pole, and is symmetric about the diameter of the circle which is the line of tangency of the cusp. The line is an asymptote. It is a member of the conchoid of de Sluze family of curves and in form it resembles a tractrix. Construction and equations Let the radius of be . By translation and rotation, we may take to be the origin and the center of the circle to be (''a'', 0), so is . Then the polar equations of and are: :\begin & r=2a\sec\theta \\ & r=2a\ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Witch Of Agnesi
In mathematics, the witch of Agnesi () is a cubic plane curve defined from two diametrically opposite points of a circle. It gets its name from Italian mathematician Maria Gaetana Agnesi, and from a mistranslation of an Italian word for a sailing sheet. Before Agnesi, the same curve was studied by Fermat, Grandi, and Newton. The graph of the derivative of the arctangent function forms an example of the witch of Agnesi. As the probability density function of the Cauchy distribution, the witch of Agnesi has applications in probability theory. It also gives rise to Runge's phenomenon in the approximation of functions by polynomials, has been used to approximate the energy distribution of spectral lines, and models the shape of hills. The witch is tangent to its defining circle at one of the two defining points, and asymptotic to the tangent line to the circle at the other point. It has a unique vertex (a point of extreme curvature) at the point of tangency with its defining ci ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Semicubical Parabola
In mathematics, a cuspidal cubic or semicubical parabola is an algebraic plane curve that has an implicit equation of the form : y^2 - a^2 x^3 = 0 (with ) in some Cartesian coordinate system. Solving for leads to the ''explicit form'' : y = \pm a x^, which imply that every real point satisfies . The exponent explains the term ''semicubical parabola''. (A parabola can be described by the equation .) Solving the implicit equation for yields a second ''explicit form'' :x = \left(\frac\right)^. The parametric equation : \quad x = t^2, \quad y = a t^3 can also be deduced from the implicit equation by putting t = \frac. . The semicubical parabolas have a cuspidal singularity; hence the name of ''cuspidal cubic''. The arc length of the curve was calculated by the English mathematician William Neile and published in 1657 (see section History). Properties of semicubical parabolas Similarity Any semicubical parabola (t^2,at^3) is similar to the ''semicubical unit parabola'' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Conchoid (mathematics)
In geometry, a conchoid is a curve derived from a fixed point , another curve, and a length . It was invented by the ancient Greek mathematician Nicomedes. Description For every line through that intersects the given curve at the two points on the line which are from are on the conchoid. The conchoid is, therefore, the cissoid of the given curve and a circle of radius and center . They are called ''conchoids'' because the shape of their outer branches resembles conch shells. The simplest expression uses polar coordinates with at the origin. If :r=\alpha(\theta) expresses the given curve, then :r=\alpha(\theta)\pm d expresses the conchoid. If the curve is a line, then the conchoid is the ''conchoid of Nicomedes''. For instance, if the curve is the line , then the line's polar form is and therefore the conchoid can be expressed parametrically as :x=a \pm d \cos \theta,\, y=a \tan \theta \pm d \sin \theta. A limaçon is a conchoid with a circle as the given curve. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
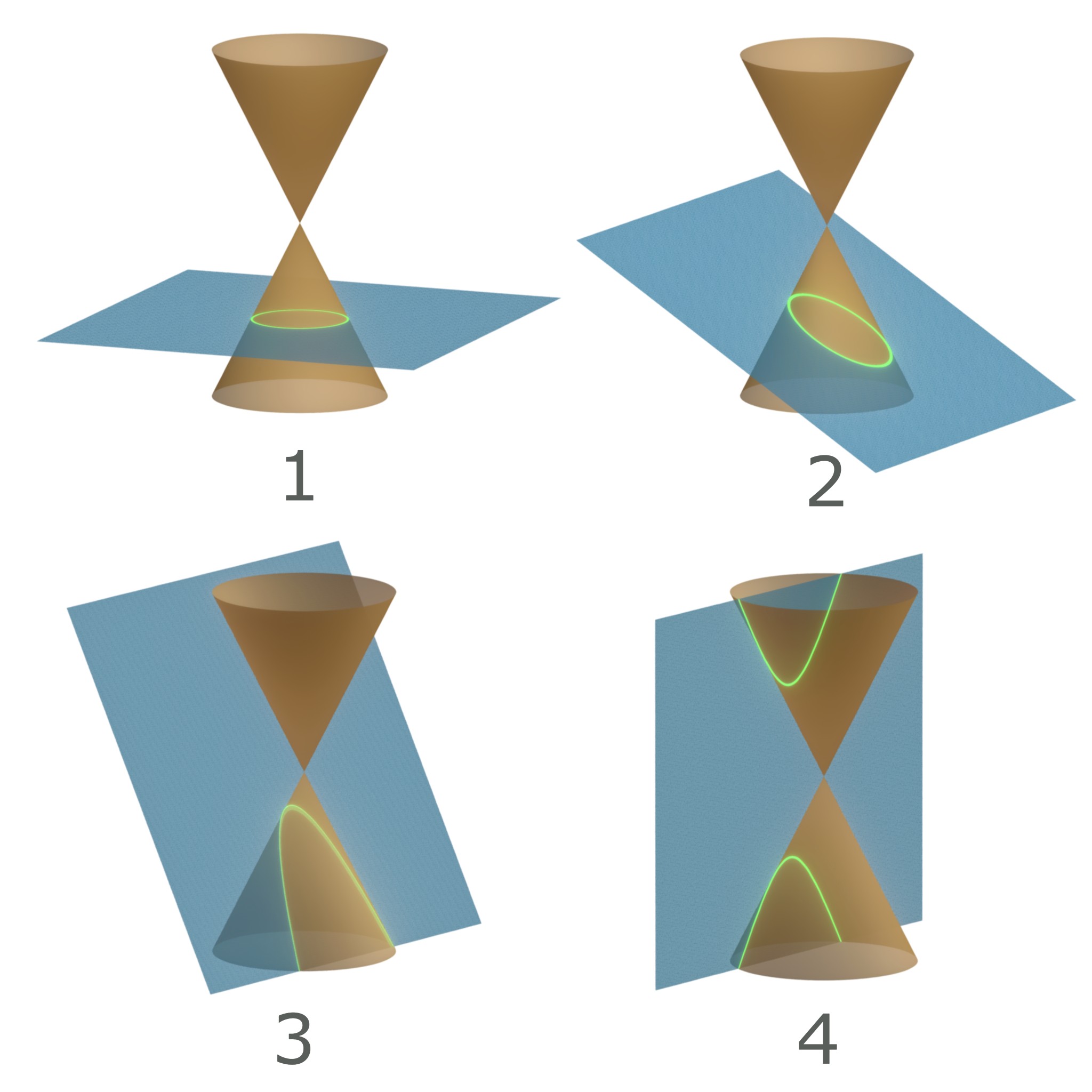
Conic Section
In mathematics, a conic section, quadratic curve or conic is a curve obtained as the intersection of the surface of a cone with a plane. The three types of conic section are the hyperbola, the parabola, and the ellipse; the circle is a special case of the ellipse, though historically it was sometimes called a fourth type. The ancient Greek mathematicians studied conic sections, culminating around 200 BC with Apollonius of Perga's systematic work on their properties. The conic sections in the Euclidean plane have various distinguishing properties, many of which can be used as alternative definitions. One such property defines a non-circular conic to be the set of those points whose distances to some particular point, called a ''focus'', and some particular line, called a ''directrix'', are in a fixed ratio, called the ''eccentricity''. The type of conic is determined by the value of the eccentricity. In analytic geometry, a conic may be defined as a plane algebraic curv ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Poncelet's Closure Theorem
In geometry, Poncelet's closure theorem, also known as Poncelet's porism, states that whenever a polygon is inscribed in one conic section and circumscribes another one, the polygon must be part of an infinite family of polygons that are all inscribed in and circumscribe the same two conics. It is named after French engineer and mathematician Jean-Victor Poncelet, who wrote about it in 1822; however, the triangular case was discovered significantly earlier, in 1746 by William Chapple. Poncelet's porism can be proved by an argument using an elliptic curve, whose points represent a combination of a line tangent to one conic and a crossing point of that line with the other conic. Statement Let ''C'' and ''D'' be two plane conics. If it is possible to find, for a given ''n'' > 2, one ''n''-sided polygon that is simultaneously inscribed in ''C'' (meaning that all of its vertices lie on ''C'') and circumscribed around ''D'' (meaning that all of its edges are tangent to ' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pascal's Theorem
In projective geometry, Pascal's theorem (also known as the ''hexagrammum mysticum theorem'') states that if six arbitrary points are chosen on a conic (which may be an ellipse, parabola or hyperbola in an appropriate affine plane) and joined by line segments in any order to form a hexagon, then the three pairs of opposite sides of the hexagon (extended if necessary) meet at three points which lie on a straight line, called the Pascal line of the hexagon. It is named after Blaise Pascal. The theorem is also valid in the Euclidean plane, but the statement needs to be adjusted to deal with the special cases when opposite sides are parallel. This theorem is a generalization of Pappus's (hexagon) theorem, which is the special case of a degenerate conic of two lines with three points on each line. Euclidean variants The most natural setting for Pascal's theorem is in a projective plane since any two lines meet and no exceptions need to be made for parallel lines. However, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |