|
Equivalence Class (music)
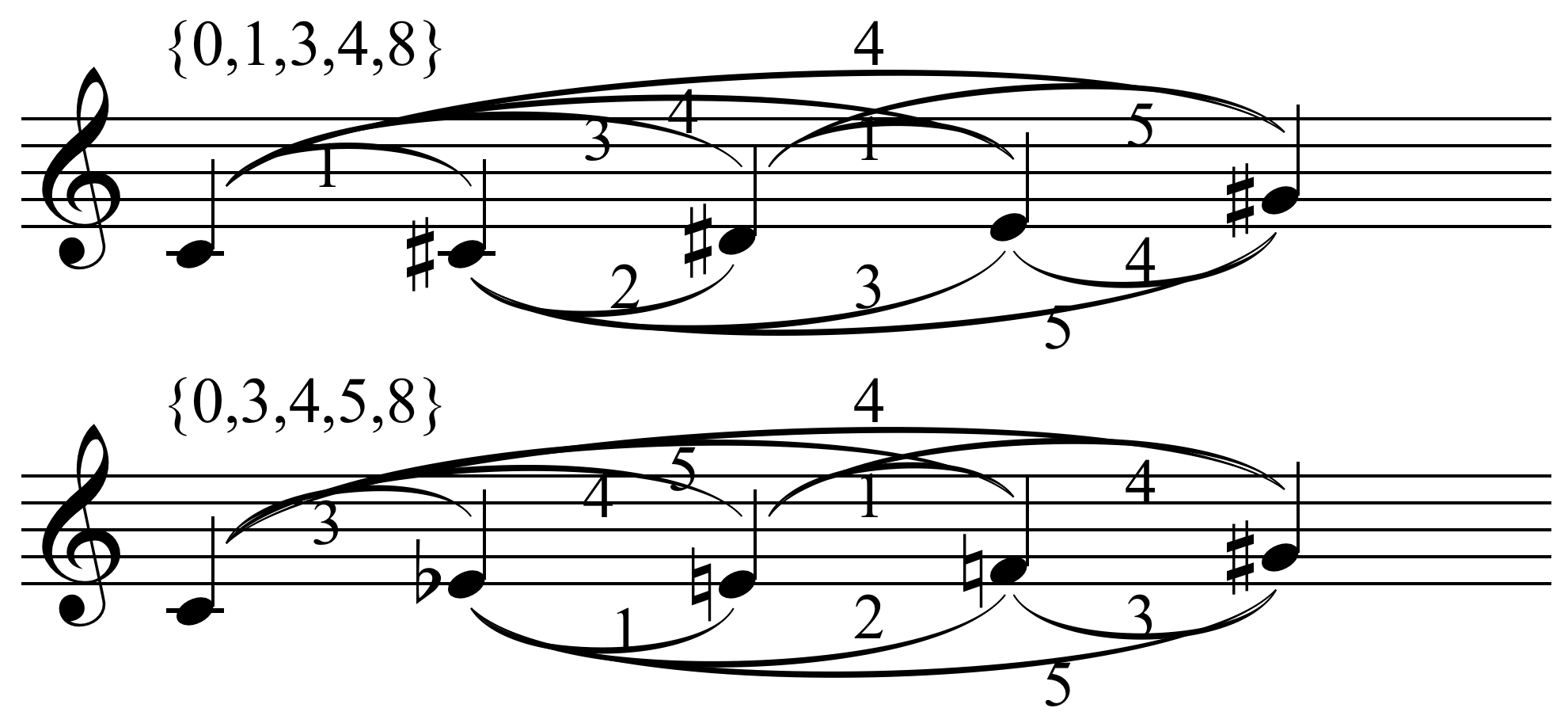
In music theory, equivalence class is an Equality (mathematics), equality (Equals sign, =) or equivalence relation, equivalence between properties of set (music), sets (unordered) or twelve-tone rows (ordered sets). A relation rather than an operation, it may be contrasted with derivation (music), derivation.Schuijer (2008). ''Analyzing Atonal Music: Pitch-Class Set Theory and Its Contexts'', p.85. . "It is not surprising that music theorists have different concepts of equivalence [from each other]..." "Indeed, an informal notion of equivalence has always been part of music theory and analysis. Pitch class set theory, however, has adhered to formal definitions of equivalence." Traditionally, octave equivalency is assumed, while inversion (music), inversional, permutation (music), permutational, and Transposition (music)#Transpositional equivalency, transpositional equivalency may or may not be considered (sequence (music), sequences and modulation (music), modulations are techniqu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Equivalence Class
In mathematics, when the elements of some set S have a notion of equivalence (formalized as an equivalence relation), then one may naturally split the set S into equivalence classes. These equivalence classes are constructed so that elements a and b belong to the same equivalence class if, and only if, they are equivalent. Formally, given a set S and an equivalence relation \sim on S, the of an element a in S is denoted /math> or, equivalently, to emphasize its equivalence relation \sim, and is defined as the set of all elements in S with which a is \sim-related. The definition of equivalence relations implies that the equivalence classes form a partition of S, meaning, that every element of the set belongs to exactly one equivalence class. The set of the equivalence classes is sometimes called the quotient set or the quotient space of S by \sim, and is denoted by S /. When the set S has some structure (such as a group operation or a topology) and the equivalence re ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Transposition (music)
In music, transposition refers to the process or operation of moving a collection of notes ( pitches or pitch classes) up or down in pitch by a constant interval. For example, a music transposer might transpose an entire piece of music into another key. Similarly, one might transpose a tone row or an unordered collection of pitches such as a chord so that it begins on another pitch. The transposition of a set ''A'' by ''n'' semitones is designated by ''T''''n''(''A''), representing the addition ( mod 12) of an integer ''n'' to each of the pitch class integers of the set ''A''. Thus the set (''A'') consisting of 0–1–2 transposed by 5 semitones is 5–6–7 (''T''5(''A'')) since , , and . Scalar transpositions In scalar transposition, every pitch in a collection is shifted up or down a fixed number of scale steps within some scale. The pitches remain in the same scale before and after the shift. This term covers both chromatic and diatonic transpositions as follo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Set Theory (music)
Musical set theory provides concepts for categorizing musical objects and describing their relationships. Howard Hanson first elaborated many of the concepts for analyzing tonal music. Other theorists, such as Allen Forte, further developed the theory for analyzing atonal music, drawing on the twelve-tone theory of Milton Babbitt. The concepts of musical set theory are very general and can be applied to tonal and atonal styles in any equal temperament tuning system, and to some extent more generally than that. One branch of musical set theory deals with collections ( sets and permutations) of pitches and pitch classes (pitch-class set theory), which may be ordered or unordered, and can be related by musical operations such as transposition, melodic inversion, and complementation. Some theorists apply the methods of musical set theory to the analysis of rhythm as well. Comparison with mathematical set theory Although musical set theory is often thought to involve the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Invariance (music)
The twelve-tone technique—also known as dodecaphony, twelve-tone serialism, and (in British usage) twelve-note composition—is a method of musical composition. The technique is a means of ensuring that all 12 notes of the chromatic scale are sounded equally often in a piece of music while preventing the emphasis of any one notePerle 1977, 2. through the use of tone rows, orderings of the 12 pitch classes. All 12 notes are thus given more or less equal importance, and the music avoids being in a key. The technique was first devised by Austrian composer Josef Matthias Hauer, who published his "law of the twelve tones" in 1919. In 1923, Arnold Schoenberg (1874–1951) developed his own, better-known version of 12-tone technique, which became associated with the "Second Viennese School" composers, who were the primary users of the technique in the first decades of its existence. Over time, the technique increased greatly in popularity and eventually became widely influential ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Identity (music)
In post-tonal music theory, identity is similar to identity in universal algebra. An identity function is a permutation or transformation which transforms a pitch or pitch class set into itself. Generally this requires symmetry. For instance, inverting an augmented triad or C4 interval cycle, 048, produces itself. Performing a retrograde operation upon the tone row 01210 produces 01210. Doubling the length of a rhythm while doubling the tempo produces a rhythm of the same durations as the original. In addition to being a property of a specific set, identity is, by extension, the "family" of sets or set forms which satisfy a possible identity. These families are defined by symmetry, which means that an object is invariant to any of various transformations; including reflection and rotation. George Perle George Perle (6 May 1915 – 23 January 2009) was an American composer and music theory, music theorist. As a composer, his music was largely atonality, atonal, us ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Enharmonic
In music, two written notes have enharmonic equivalence if they produce the same pitch but are notated differently. Similarly, written intervals, chords, or key signatures are considered enharmonic if they represent identical pitches that are notated differently. The term derives from Latin , in turn from Late Latin , from Ancient Greek (), from ('in') and ('harmony'). Definition The predominant tuning system in Western music is twelve-tone equal temperament (12 ), where each octave is divided into twelve equivalent half steps or semitones. The notes F and G are a whole step apart, so the note one semitone above F (F) and the note one semitone below G (G) indicate the same pitch. These written notes are ''enharmonic'', or ''enharmonically equivalent''. The choice of notation for a pitch can depend on its role in harmony; this notation keeps modern music compatible with earlier tuning systems, such as meantone temperaments. The choice can also depend on the note's re ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Identity (music)
In post-tonal music theory, identity is similar to identity in universal algebra. An identity function is a permutation or transformation which transforms a pitch or pitch class set into itself. Generally this requires symmetry. For instance, inverting an augmented triad or C4 interval cycle, 048, produces itself. Performing a retrograde operation upon the tone row 01210 produces 01210. Doubling the length of a rhythm while doubling the tempo produces a rhythm of the same durations as the original. In addition to being a property of a specific set, identity is, by extension, the "family" of sets or set forms which satisfy a possible identity. These families are defined by symmetry, which means that an object is invariant to any of various transformations; including reflection and rotation. George Perle George Perle (6 May 1915 – 23 January 2009) was an American composer and music theory, music theorist. As a composer, his music was largely atonality, atonal, us ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematical Set Theory
Set theory is the branch of mathematical logic that studies sets, which can be informally described as collections of objects. Although objects of any kind can be collected into a set, set theory – as a branch of mathematics – is mostly concerned with those that are relevant to mathematics as a whole. The modern study of set theory was initiated by the German mathematicians Richard Dedekind and Georg Cantor in the 1870s. In particular, Georg Cantor is commonly considered the founder of set theory. The non-formalized systems investigated during this early stage go under the name of ''naive set theory''. After the discovery of paradoxes within naive set theory (such as Russell's paradox, Cantor's paradox and the Burali-Forti paradox), various axiomatic systems were proposed in the early twentieth century, of which Zermelo–Fraenkel set theory (with or without the axiom of choice) is still the best-known and most studied. Set theory is commonly employed as a foundationa ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Milton Babbitt
Milton Byron Babbitt (May 10, 1916 – January 29, 2011) was an American composer, music theorist, mathematician, and teacher. He was a Pulitzer Prize and MacArthur Fellowship recipient, recognized for his serial and electronic music. Biography Babbitt was born in Philadelphia to Albert E. Babbitt and Sarah Potamkin, who were Jewish. He was raised in Jackson, Mississippi, and began studying the violin when he was four but soon switched to clarinet and saxophone. Early in his life he was attracted to jazz and theater music, and "played in every pit-orchestra that came to town". Babbitt was making his own arrangements of popular songs by age 7, "wrote a lot of pop tunes for school productions", and won a local songwriting contest when he was 13. A Jackson newspaper called Babbitt a "whiz kid" and noted "that he had perfect pitch and could add up his family's grocery bills in his head. In his teens he became a great fan of jazz cornet player Bix Beiderbecke". Babbitt's father was ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Common Practice Period
In Western classical music, the common practice period (CPP) was the period of about 250 years during which the tonal system was regarded as the only basis for composition. It began when composers' use of the tonal system had clearly superseded earlier systems, and ended when some composers began using significantly modified versions of the tonal system, and began developing other systems as well. Most features of common practice (the accepted concepts of composition during this time) persisted from the mid-Baroque period through the Classical and Romantic periods, roughly from 1650 to 1900. There was much stylistic evolution during these centuries, with patterns and conventions flourishing and then declining, such as the sonata form. The most prominent unifying feature throughout the period is a harmonic language to which music theorists can today apply Roman numeral chord analysis; however, the "common" in common practice does not directly refer to any type of harmony, rat ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Modulation (music)
In music, modulation is the change from one tonality ( tonic, or tonal center) to another. This may or may not be accompanied by a change in key signature (a key change). Modulations articulate or create the structure or form of many pieces, as well as add interest. Treatment of a chord as the tonic for less than a phrase is considered tonicization. Requirements *Harmonic: quasi- tonic, modulating dominant, pivot chordForte (1979), p. 267. * Melodic: recognizable segment of the scale of the quasi-tonic or strategically placed leading-tone *Metric and rhythmic: quasi-tonic and modulating dominant on metrically accented beats, prominent pivot chord The quasi-tonic is the tonic of the new key established by the modulation. The modulating dominant is the dominant of the quasi-tonic. The pivot chord is a predominant to the modulating dominant and a chord common to both the keys of the tonic and the quasi-tonic. For example, in a modulation to the dominant, ii/V–V/V–V co ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sequence (music)
In music, a sequence is the restatement (music), restatement of a motif (music), motif or longer melody, melodic (or harmony (music), harmonic) passage at a higher or lower pitch (music), pitch in the same voice.Benward and Saker (2003). ''Music: In Theory and Practice, Vol. I'', p.111-12. Seventh Edition. . It is one of the most common and simple methods of elaborating a melody in eighteenth and nineteenth century classical music (Classical period (music), Classical period and Romantic music). Characteristics of sequences: *Two segments, usually no more than three or four *Usually in only one direction: continually higher or lower *Segments continue by same interval distance It is possible for melody or harmony to form a sequence without the other participating. There are many types of sequences, each with a unique pattern. Listed below are some examples. Melodic sequences In a melody, a real sequence is a sequence where the subsequent segments are exact transposition (music) ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
