|
Eden’s Conjecture
In the mathematics of dynamical systems, Eden's conjecture states that the supremum of the local Lyapunov dimensions on the global attractor is achieved on a stationary point or an unstable periodic orbit embedded into the attractor. The validity of the conjecture was proved for a number of well-known systems having global attractor (e.g. for the global attractors in the Lorenz system, complex Ginzburg–Landau equation). It is named after Alp Eden, who proposed it in 1987. Kuznetsov–Eden's conjecture For local attractors, a ''conjecture on the Lyapunov dimension of self-excited attractor'', refined by N. Kuznetsov, is stated that for a typical system, the Lyapunov dimension of a self-excited attractor does not exceed the Lyapunov dimension of one of the unstable equilibria, the unstable manifold of which intersects with the basin of attraction and visualizes the attractor. The conjecture is valid, e.g., for the classical self-excited Lorenz attractor; for the self-e ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dynamical Systems
In mathematics, a dynamical system is a system in which a function describes the time dependence of a point in an ambient space. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water in a pipe, the random motion of particles in the air, and the number of fish each springtime in a lake. The most general definition unifies several concepts in mathematics such as ordinary differential equations and ergodic theory by allowing different choices of the space and how time is measured. Time can be measured by integers, by real or complex numbers or can be a more general algebraic object, losing the memory of its physical origin, and the space may be a manifold or simply a set, without the need of a smooth space-time structure defined on it. At any given time, a dynamical system has a state representing a point in an appropriate state space. This state is often given by a tuple of real numbers or by a vector in a geometrical manif ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
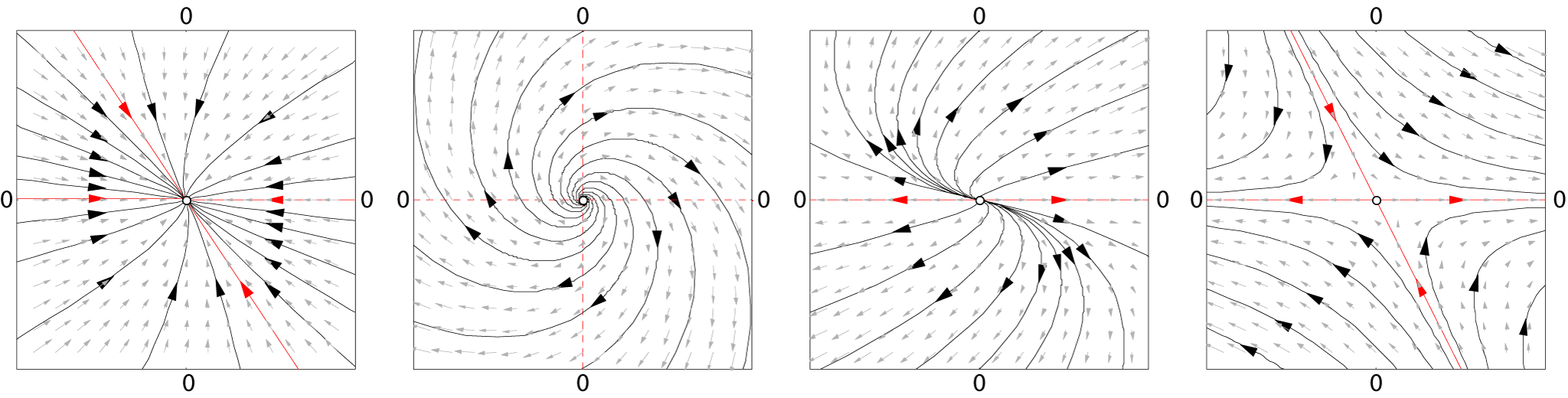
Lyapunov Dimension
In the mathematics of dynamical systems, the concept of Lyapunov dimension was suggested by Kaplan and Yorke for estimating the Hausdorff dimension of attractors. Further the concept has been developed and rigorously justified in a number of papers, and nowadays various different approaches to the definition of Lyapunov dimension are used. Remark that the attractors with noninteger Hausdorff dimension are called strange attractors. Since the direct numerical computation of the Hausdorff dimension of attractors is often a problem of high numerical complexity, estimations via the Lyapunov dimension became widely spread. The Lyapunov dimension was named after the Russian mathematician Aleksandr Lyapunov because of the close connection with the Lyapunov exponents. Definitions Consider a dynamical system \big(\_, (U\subseteq \mathbb^n, \, \cdot\, )\big) , where \varphi^t is the shift operator along the solutions: \varphi^t(u_0) = u(t,u_0), of ODE \dot = f(), t \leq 0, or differen ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Attractor
In the mathematical field of dynamical systems, an attractor is a set of states toward which a system tends to evolve, for a wide variety of starting conditions of the system. System values that get close enough to the attractor values remain close even if slightly disturbed. In finite-dimensional systems, the evolving variable may be represented algebraically as an ''n''-dimensional vector. The attractor is a region in ''n''-dimensional space. In physical systems, the ''n'' dimensions may be, for example, two or three positional coordinates for each of one or more physical entities; in economic systems, they may be separate variables such as the inflation rate and the unemployment rate. If the evolving variable is two- or three-dimensional, the attractor of the dynamic process can be represented geometrically in two or three dimensions, (as for example in the three-dimensional case depicted to the right). An attractor can be a point, a finite set of points, a curve, a manif ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lorenz System
The Lorenz system is a system of ordinary differential equations first studied by mathematician and meteorologist Edward Lorenz. It is notable for having chaotic solutions for certain parameter values and initial conditions. In particular, the Lorenz attractor is a set of chaotic solutions of the Lorenz system. In popular media the " butterfly effect" stems from the real-world implications of the Lorenz attractor, namely that in a chaotic physical system, in the absence of perfect knowledge of the initial conditions (even the minuscule disturbance of the air due to a butterfly flapping its wings), our ability to predict its future course will always fail. This underscores that physical systems can be completely deterministic and yet still be inherently unpredictable. The shape of the Lorenz attractor itself, when plotted in phase space, may also be seen to resemble a butterfly. Overview In 1963, Edward Lorenz, with the help of Ellen Fetter who was responsible for the numerical s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Alp Eden
Osman Alp Eden (born 1958) is a Turkish mathematician, scientist and professor of mathematics. He is a retired member of the Boğaziçi University Mathematics Department in İstanbul, Turkey. Education Alp Eden was born in İstanbul in 1958. He finished the high school Robert College of Istanbul in 1976. He graduated from Boğaziçi University Civil Engineering and Mathematics departments in 1981. He received his PhD in Mathematics under the supervision of Ciprian Ilie Foiaș at the Indiana University Bloomington in the United States in 1989. Academic career He worked in Arizona State University between 1989-1992. Then he became a full time member of the Department of Mathematics, at Boğaziçi University, Istanbul. In years he served as the chair of the department and the vice-dean of the Faculty of Arts and Sciences. He retired in 2015 and moved to Izmir. He is one of the founders of the Masters program in Financial Engineering at Boğaziçi University. Between 2006-2016 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hidden Attractor
In the bifurcation theory, a bounded oscillation that is born without loss of stability of stationary set is called a hidden oscillation. In nonlinear control theory, the birth of a hidden oscillation in a time-invariant control system with bounded states means crossing a boundary, in the domain of the parameters, where local stability of the stationary states implies global stability (see, e.g. Kalman's conjecture). If a hidden oscillation (or a set of such hidden oscillations filling a compact subset of the phase space of the dynamical system) attracts all nearby oscillations, then it is called a hidden attractor. For a dynamical system with a unique equilibrium point that is globally attractive, the birth of a hidden attractor corresponds to a qualitative change in behaviour from monostability to bi-stability. In the general case, a dynamical system may turn out to be multistable and have coexisting local attractors in the phase space. While trivial attractors, i.e. stable equ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hénon Map
The Hénon map, sometimes called Hénon–Pomeau attractor/map, is a discrete-time dynamical system. It is one of the most studied examples of dynamical systems that exhibit chaotic behavior. The Hénon map takes a point (''xn'', ''yn'') in the plane and maps it to a new point :\beginx_ = 1 - a x_n^2 + y_n\\y_ = b x_n.\end The map depends on two parameters, ''a'' and ''b'', which for the classical Hénon map have values of ''a'' = 1.4 and ''b'' = 0.3. For the classical values the Hénon map is chaotic. For other values of ''a'' and ''b'' the map may be chaotic, intermittent, or converge to a periodic orbit. An overview of the type of behavior of the map at different parameter values may be obtained from its orbit diagram. The map was introduced by Michel Hénon as a simplified model of the Poincaré section of the Lorenz model. For the classical map, an initial point of the plane will either approach a set of points known as the Hénon strange ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hidden Attractor
In the bifurcation theory, a bounded oscillation that is born without loss of stability of stationary set is called a hidden oscillation. In nonlinear control theory, the birth of a hidden oscillation in a time-invariant control system with bounded states means crossing a boundary, in the domain of the parameters, where local stability of the stationary states implies global stability (see, e.g. Kalman's conjecture). If a hidden oscillation (or a set of such hidden oscillations filling a compact subset of the phase space of the dynamical system) attracts all nearby oscillations, then it is called a hidden attractor. For a dynamical system with a unique equilibrium point that is globally attractive, the birth of a hidden attractor corresponds to a qualitative change in behaviour from monostability to bi-stability. In the general case, a dynamical system may turn out to be multistable and have coexisting local attractors in the phase space. While trivial attractors, i.e. stable equ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dynamical Systems
In mathematics, a dynamical system is a system in which a function describes the time dependence of a point in an ambient space. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water in a pipe, the random motion of particles in the air, and the number of fish each springtime in a lake. The most general definition unifies several concepts in mathematics such as ordinary differential equations and ergodic theory by allowing different choices of the space and how time is measured. Time can be measured by integers, by real or complex numbers or can be a more general algebraic object, losing the memory of its physical origin, and the space may be a manifold or simply a set, without the need of a smooth space-time structure defined on it. At any given time, a dynamical system has a state representing a point in an appropriate state space. This state is often given by a tuple of real numbers or by a vector in a geometrical manif ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Chaos Theory
Chaos theory is an interdisciplinary area of scientific study and branch of mathematics focused on underlying patterns and deterministic laws of dynamical systems that are highly sensitive to initial conditions, and were once thought to have completely random states of disorder and irregularities. Chaos theory states that within the apparent randomness of chaotic complex systems, there are underlying patterns, interconnection, constant feedback loops, repetition, self-similarity, fractals, and self-organization. The butterfly effect, an underlying principle of chaos, describes how a small change in one state of a deterministic nonlinear system can result in large differences in a later state (meaning that there is sensitive dependence on initial conditions). A metaphor for this behavior is that a butterfly flapping its wings in Brazil can cause a tornado in Texas. Small differences in initial conditions, such as those due to errors in measurements or due to rounding errors i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |