|
Descriptive Geometry
Descriptive geometry is the branch of geometry which allows the representation of three-dimensional objects in two dimensions by using a specific set of procedures. The resulting techniques are important for engineering, architecture, design and in art. The theoretical basis for descriptive geometry is provided by planar geometric projections. The earliest known publication on the technique was "Underweysung der Messung mit dem Zirckel und Richtscheyt", published in Linien, Nuremberg: 1525, by Albrecht Dürer. Italian architect Guarino Guarini was also a pioneer of projective and descriptive geometry, as is clear from his ''Placita Philosophica'' (1665), ''Euclides Adauctus'' (1671) and ''Architettura Civile'' (1686—not published until 1737), anticipating the work of Gaspard Monge (1746–1818), who is usually credited with the invention of descriptive geometry. Gaspard Monge is usually considered the "father of descriptive geometry" due to his developments in geometric pro ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Descriptive Geometry - Skew Lines Appearing Perpendicular
In the study of language, description or descriptive linguistics is the work of objectively analyzing and describing how language is actually used (or how it was used in the past) by a speech community. François & Ponsonnet (2013). All academic research in linguistics is descriptive; like all other scientific disciplines, it seeks to describe reality, without the bias of preconceived ideas about how it ought to be. Modern descriptive linguistics is based on a structural approach to language, as exemplified in the work of Leonard Bloomfield and others. This type of linguistics utilizes different methods in order to describe a language such as basic data collection, and different types of elicitation methods. Descriptive versus prescriptive linguistics Linguistic description is often contrasted with linguistic prescription, — entry for "Descriptivism and prescriptivism" quotation: "Contrasting terms in linguistics." (p.286) which is found especially in education and in publi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
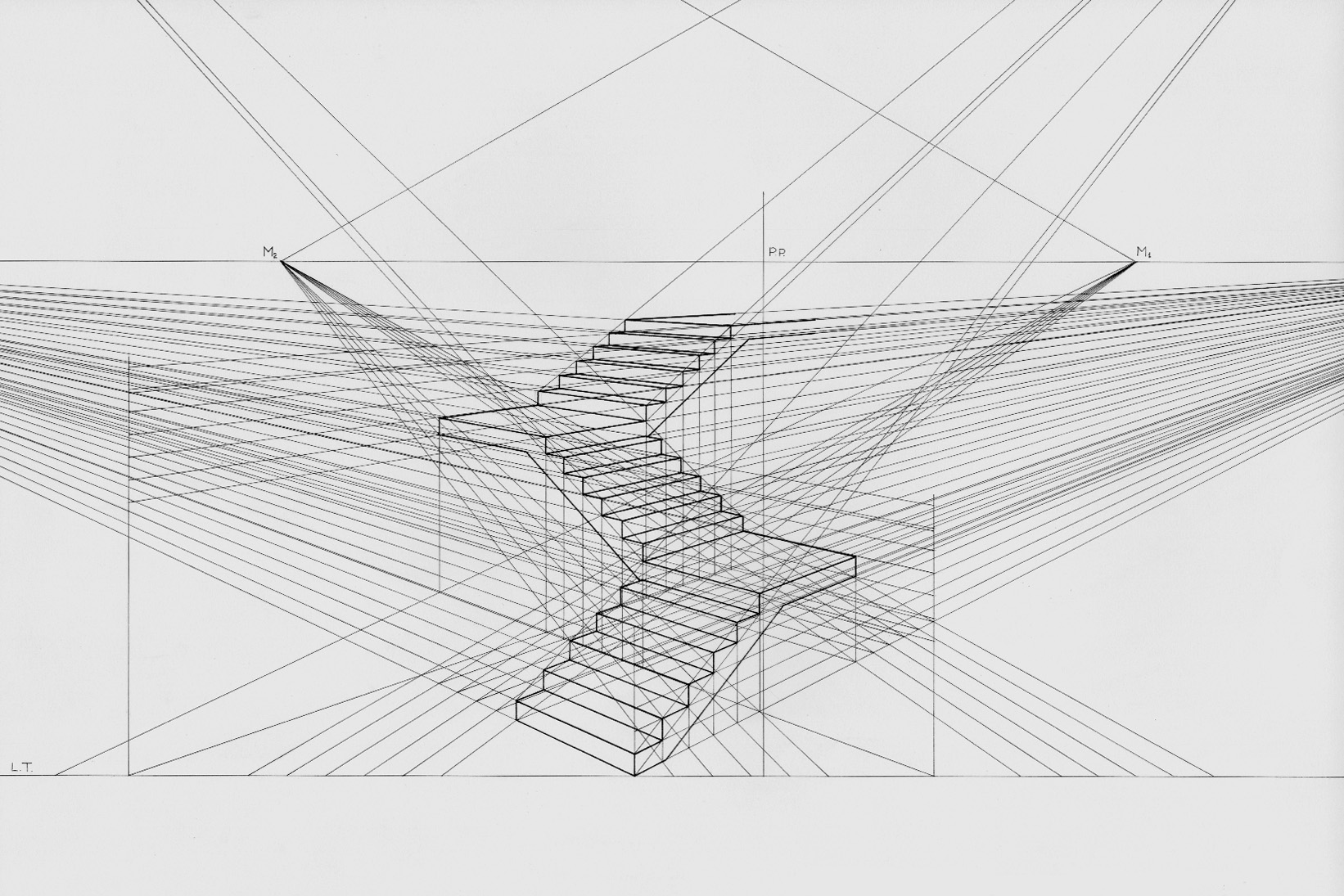
Perspective (graphical)
Linear or point-projection perspective (from la, perspicere 'to see through') is one of two types of 3D projection, graphical projection perspective in the graphic arts; the other is parallel projection. Linear perspective is an approximate representation, generally on a flat surface, of an image as it is seen by the eye. Perspective drawing is useful for representing a three-dimensional scene in a two-dimensional medium, like paper. The most characteristic features of linear perspective are that objects appear smaller as their distance from the observer increases, and that they are subject to ''foreshortening'', meaning that an object's dimensions along the line of sight appear shorter than its dimensions across the line of sight. All objects will recede to points in the distance, usually along the horizon line, but also above and below the horizon line depending on the view used. Italian Renaissance painters and architects including Masaccio, Paolo Uccello, Piero della Fran ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Perspective Projection
Linear or point-projection perspective (from la, perspicere 'to see through') is one of two types of graphical projection perspective in the graphic arts; the other is parallel projection. Linear perspective is an approximate representation, generally on a flat surface, of an image as it is seen by the eye. Perspective drawing is useful for representing a three-dimensional scene in a two-dimensional medium, like paper. The most characteristic features of linear perspective are that objects appear smaller as their distance from the observer increases, and that they are subject to ''foreshortening'', meaning that an object's dimensions along the line of sight appear shorter than its dimensions across the line of sight. All objects will recede to points in the distance, usually along the horizon line, but also above and below the horizon line depending on the view used. Italian Renaissance painters and architects including Masaccio, Paolo Uccello, Piero della Francesca and Luca ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Oblique Projection
Oblique projection is a simple type of technical drawing of graphical projection used for producing two-dimensional (2D) images of three-dimensional (3D) objects. The objects are not in perspective (graphical), perspective and so do not correspond to any view of an object that can be obtained in practice, but the technique yields somewhat convincing and useful. Oblique projection is commonly used in technical drawing. The cavalier projection was used by French military artists in the 18th century to depict fortifications. Oblique projection was used almost universally by Chinese artists from the 1st or 2nd centuries to the 18th century, especially to depict rectilinear objects such as houses. Various graphical projection techniques can be used in computer graphics, including in Computer Aided Design (CAD), computer games, computer generated animations, and special effects used in movies. Overview Oblique projection is a type of parallel projection: * it projects an ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Orthogonal Projection
In linear algebra and functional analysis, a projection is a linear transformation P from a vector space to itself (an endomorphism) such that P\circ P=P. That is, whenever P is applied twice to any vector, it gives the same result as if it were applied once (i.e. P is idempotent). It leaves its image unchanged. This definition of "projection" formalizes and generalizes the idea of graphical projection. One can also consider the effect of a projection on a geometrical object by examining the effect of the projection on points in the object. Definitions A projection on a vector space V is a linear operator P : V \to V such that P^2 = P. When V has an inner product and is complete (i.e. when V is a Hilbert space) the concept of orthogonality can be used. A projection P on a Hilbert space V is called an orthogonal projection if it satisfies \langle P \mathbf x, \mathbf y \rangle = \langle \mathbf x, P \mathbf y \rangle for all \mathbf x, \mathbf y \in V. A projection on a Hilber ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Trimetric Projection
Axonometric projection is a type of orthographic projection used for creating a pictorial drawing of an object, where the object is rotated around one or more of its axes to reveal multiple sides.Gary R. Bertoline et al. (2002) ''Technical Graphics Communication''. McGraw–Hill Professional, 2002. , p. 330. Overview "Axonometry" means "to measure along the axes". In German literature, axonometry is based on Pohlke's theorem, such that the scope of axonometric projection could encompass ''every'' type of parallel projection, including not only orthographic projection (and multiview projection), but also oblique projection. However, outside of German literature, the term "axonometric" is sometimes used only to distinguish between orthographic views where the principal axes of an object are ''not'' orthogonal to the projection plane, and orthographic views in which the principal axes of the object ''are'' orthogonal to the projection plane. (In multiview projection these woul ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dimetric Projection
Axonometric projection is a type of orthographic projection used for creating a pictorial drawing of an object, where the object is rotated around one or more of its axes to reveal multiple sides.Gary R. Bertoline et al. (2002) ''Technical Graphics Communication''. McGraw–Hill Professional, 2002. , p. 330. Overview "Axonometry" means "to measure along the axes". In German literature, axonometry is based on Pohlke's theorem, such that the scope of axonometric projection could encompass ''every'' type of parallel projection, including not only orthographic projection (and multiview projection), but also oblique projection. However, outside of German literature, the term "axonometric" is sometimes used only to distinguish between orthographic views where the principal axes of an object are ''not'' orthogonal to the projection plane, and orthographic views in which the principal axes of the object ''are'' orthogonal to the projection plane. (In multiview projection these woul ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Isometric Projection
Isometric projection is a method for visually representing three-dimensional objects in two dimensions in technical and engineering drawings. It is an axonometric projection in which the three coordinate axes appear equally foreshortened and the angle between any two of them is 120 degrees. Overview The term "isometric" comes from the Greek for "equal measure", reflecting that the scale along each axis of the projection is the same (unlike some other forms of graphical projection). An isometric view of an object can be obtained by choosing the viewing direction such that the angles between the projections of the ''x'', ''y'', and ''z'' axes are all the same, or 120°. For example, with a cube, this is done by first looking straight towards one face. Next, the cube is rotated ±45° about the vertical axis, followed by a rotation of approximately 35.264° (precisely arcsin or arctan , which is related to the Magic angle) about the horizontal axis. Note that with the cub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Axonometric Projection
Axonometric projection is a type of orthographic projection used for creating a pictorial drawing of an object, where the object is rotated around one or more of its axes to reveal multiple sides.Gary R. Bertoline et al. (2002) ''Technical Graphics Communication''. McGraw–Hill Professional, 2002. , p. 330. Overview "Axonometry" means "to measure along the axes". In German literature, axonometry is based on Pohlke's theorem, such that the scope of axonometric projection could encompass ''every'' type of parallel projection, including not only orthographic projection (and multiview projection), but also oblique projection. However, outside of German literature, the term "axonometric" is sometimes used only to distinguish between orthographic views where the principal axes of an object are ''not'' orthogonal to the projection plane, and orthographic views in which the principal axes of the object ''are'' orthogonal to the projection plane. (In multiview projection these woul ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Orthographic Projection
Orthographic projection (also orthogonal projection and analemma) is a means of representing Three-dimensional space, three-dimensional objects in Two-dimensional space, two dimensions. Orthographic projection is a form of parallel projection in which all the projection lines are orthogonal to the projection plane, resulting in every plane of the scene appearing in affine transformation on the viewing surface. The obverse of an orthographic projection is an oblique projection, which is a parallel projection in which the projection lines are ''not'' orthogonal to the projection plane. The term ''orthographic'' sometimes means a technique in multiview projection in which principal axes or the planes of the subject are also parallel with the projection plane to create the ''primary views''. If the principal planes or axes of an object in an orthographic projection are ''not'' parallel with the projection plane, the depiction is called ''axonometric'' or an ''auxiliary views''. (''A ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Graphical Projection
A 3D projection (or graphical projection) is a design technique used to display a three-dimensional (3D) object on a two-dimensional (2D) surface. These projections rely on visual perspective and aspect analysis to project a complex object for viewing capability on a simpler plane. 3D projections use the primary qualities of an object's basic shape to create a map of points, that are then connected to one another to create a visual element. The result is a graphic that contains conceptual properties to interpret that the figure or image as not actually flat (2D), but rather, as a solid object (3D) being viewed on a 2D display. 3D objects are largely displayed on two-dimensional mediums (i.e. paper and computer monitors). As such, graphical projections are a commonly used design element; notably, in engineering drawing, drafting, and computer graphics. Projections can be calculated through employment of mathematical analysis and formulae, or by using various geometric and op ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |