|
Dynkin Index
In mathematics, the Dynkin index I() of a finite-dimensional highest-weight representation of a compact simple Lie algebra \mathfrak g with highest weight \lambda is defined by \text_= 2I(\lambda) \text_, where V_0 is the 'defining representation', which is most often taken to be the fundamental representation if the Lie algebra under consideration is a matrix Lie algebra. The notation \text_V is the trace form on the representation \rho: \mathfrak \rightarrow \text(V). By Schur's lemma, since the trace forms are all invariant forms, they are related by constants, so the index is well-defined. Since the trace forms are bilinear forms, we can take traces to obtain :I(\lambda)=\frac(\lambda, \lambda +2\rho) where the Weyl vector :\rho=\frac\sum_ \alpha is equal to half of the sum of all the positive roots of \mathfrak g. The expression (\lambda, \lambda +2\rho) is the value of the quadratic Casimir in the representation V_\lambda. The index I(\lambda) is always a positiv ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics with the major subdisciplines of number theory, algebra, geometry, and analysis, respectively. There is no general consensus among mathematicians about a common definition for their academic discipline. Most mathematical activity involves the discovery of properties of abstract objects and the use of pure reason to prove them. These objects consist of either abstractions from nature orin modern mathematicsentities that are stipulated to have certain properties, called axioms. A ''proof'' consists of a succession of applications of deductive rules to already established results. These results include previously proved theorems, axioms, andin case of abstraction from naturesome basic properties that are considered true starting points of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Highest-weight Representation
In the mathematical field of representation theory, a weight of an algebra ''A'' over a field F is an algebra homomorphism from ''A'' to F, or equivalently, a one-dimensional representation of ''A'' over F. It is the algebra analogue of a multiplicative character of a group. The importance of the concept, however, stems from its application to representations of Lie algebras and hence also to representations of algebraic and Lie groups. In this context, a weight of a representation is a generalization of the notion of an eigenvalue, and the corresponding eigenspace is called a weight space. Motivation and general concept Given a set ''S'' of n\times n matrices over the same field, each of which is diagonalizable, and any two of which commute, it is always possible to simultaneously diagonalize all of the elements of ''S''.In fact, given a set of commuting matrices over an algebraically closed field, they are simultaneously triangularizable, without needing to assume that they are ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Compact (topology)
In mathematics, specifically general topology, compactness is a property that seeks to generalize the notion of a closed and bounded subset of Euclidean space by making precise the idea of a space having no "punctures" or "missing endpoints", i.e. that the space not exclude any ''limiting values'' of points. For example, the open interval (0,1) would not be compact because it excludes the limiting values of 0 and 1, whereas the closed interval ,1would be compact. Similarly, the space of rational numbers \mathbb is not compact, because it has infinitely many "punctures" corresponding to the irrational numbers, and the space of real numbers \mathbb is not compact either, because it excludes the two limiting values +\infty and -\infty. However, the ''extended'' real number line ''would'' be compact, since it contains both infinities. There are many ways to make this heuristic notion precise. These ways usually agree in a metric space, but may not be equivalent in other topologic ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lie Algebra
In mathematics, a Lie algebra (pronounced ) is a vector space \mathfrak g together with an Binary operation, operation called the Lie bracket, an Alternating multilinear map, alternating bilinear map \mathfrak g \times \mathfrak g \rightarrow \mathfrak g, that satisfies the Jacobi identity. The Lie bracket of two vectors x and y is denoted [x,y]. The vector space \mathfrak g together with this operation is a non-associative algebra, meaning that the Lie bracket is not necessarily associative property, associative. Lie algebras are closely related to Lie groups, which are group (mathematics), groups that are also smooth manifolds: any Lie group gives rise to a Lie algebra, which is its tangent space at the identity. Conversely, to any finite-dimensional Lie algebra over real or complex numbers, there is a corresponding connected space, connected Lie group unique up to finite coverings (Lie's third theorem). This Lie group–Lie algebra correspondence, correspondence allows one ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lie Algebra
In mathematics, a Lie algebra (pronounced ) is a vector space \mathfrak g together with an Binary operation, operation called the Lie bracket, an Alternating multilinear map, alternating bilinear map \mathfrak g \times \mathfrak g \rightarrow \mathfrak g, that satisfies the Jacobi identity. The Lie bracket of two vectors x and y is denoted [x,y]. The vector space \mathfrak g together with this operation is a non-associative algebra, meaning that the Lie bracket is not necessarily associative property, associative. Lie algebras are closely related to Lie groups, which are group (mathematics), groups that are also smooth manifolds: any Lie group gives rise to a Lie algebra, which is its tangent space at the identity. Conversely, to any finite-dimensional Lie algebra over real or complex numbers, there is a corresponding connected space, connected Lie group unique up to finite coverings (Lie's third theorem). This Lie group–Lie algebra correspondence, correspondence allows one ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fundamental Representation
In representation theory of Lie groups and Lie algebras, a fundamental representation is an irreducible finite-dimensional representation of a semisimple Lie group or Lie algebra whose highest weight is a fundamental weight. For example, the defining module of a classical Lie group is a fundamental representation. Any finite-dimensional irreducible representation of a semisimple Lie group or Lie algebra can be constructed from the fundamental representations by a procedure due to Élie Cartan. Thus in a certain sense, the fundamental representations are the elementary building blocks for arbitrary finite-dimensional representations. Examples * In the case of the general linear group, all fundamental representations are exterior products of the defining module. * In the case of the special unitary group SU(''n''), the ''n'' − 1 fundamental representations are the wedge products \operatorname^k\ ^n consisting of the alternating tensors, for ''k'' = 1,&nbs ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Matrix (mathematics)
In mathematics, a matrix (plural matrices) is a rectangular array or table of numbers, symbols, or expressions, arranged in rows and columns, which is used to represent a mathematical object or a property of such an object. For example, \begin1 & 9 & -13 \\20 & 5 & -6 \end is a matrix with two rows and three columns. This is often referred to as a "two by three matrix", a "-matrix", or a matrix of dimension . Without further specifications, matrices represent linear maps, and allow explicit computations in linear algebra. Therefore, the study of matrices is a large part of linear algebra, and most properties and operations of abstract linear algebra can be expressed in terms of matrices. For example, matrix multiplication represents composition of linear maps. Not all matrices are related to linear algebra. This is, in particular, the case in graph theory, of incidence matrices, and adjacency matrices. ''This article focuses on matrices related to linear algebra, and, unle ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Killing Form
In mathematics, the Killing form, named after Wilhelm Killing, is a symmetric bilinear form that plays a basic role in the theories of Lie groups and Lie algebras. Cartan's criteria (criterion of solvability and criterion of semisimplicity) show that Killing form has a close relationship to the semisimplicity of the Lie algebras. History and name The Killing form was essentially introduced into Lie algebra theory by in his thesis. In a historical survey of Lie theory, has described how the term ''"Killing form"'' first occurred in 1951 during one of his own reports for the Séminaire Bourbaki; it arose as a misnomer, since the form had previously been used by Lie theorists, without a name attached. Some other authors now employ the term ''" Cartan-Killing form"''. At the end of the 19th century, Killing had noted that the coefficients of the characteristic equation of a regular semisimple element of a Lie algebra are invariant under the adjoint group, from which it follows tha ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Schur's Lemma
In mathematics, Schur's lemma is an elementary but extremely useful statement in representation theory of groups and algebras. In the group case it says that if ''M'' and ''N'' are two finite-dimensional irreducible representations of a group ''G'' and ''φ'' is a linear map from ''M'' to ''N'' that commutes with the action of the group, then either ''φ'' is invertible, or ''φ'' = 0. An important special case occurs when ''M'' = ''N'', i.e. ''φ'' is a self-map; in particular, any element of the center of a group must act as a scalar operator (a scalar multiple of the identity) on ''M''. The lemma is named after Issai Schur who used it to prove the Schur orthogonality relations and develop the basics of the representation theory of finite groups. Schur's lemma admits generalisations to Lie groups and Lie algebras, the most common of which are due to Jacques Dixmier and Daniel Quillen. Representation theory of groups Representation theory is the study of homomorphi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bilinear Form
In mathematics, a bilinear form is a bilinear map on a vector space (the elements of which are called '' vectors'') over a field ''K'' (the elements of which are called ''scalars''). In other words, a bilinear form is a function that is linear in each argument separately: * and * and The dot product on \R^n is an example of a bilinear form. The definition of a bilinear form can be extended to include modules over a ring, with linear maps replaced by module homomorphisms. When is the field of complex numbers , one is often more interested in sesquilinear forms, which are similar to bilinear forms but are conjugate linear in one argument. Coordinate representation Let be an -dimensional vector space with basis . The matrix ''A'', defined by is called the ''matrix of the bilinear form'' on the basis . If the matrix represents a vector with respect to this basis, and analogously, represents another vector , then: B(\mathbf, \mathbf) = \mathbf^\textsf A\mathbf = \ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Weyl Vector
In mathematics, the Weyl character formula in representation theory describes the characters of irreducible representations of compact Lie groups in terms of their highest weights. It was proved by . There is a closely related formula for the character of an irreducible representation of a semisimple Lie algebra. In Weyl's approach to the representation theory of connected compact Lie groups, the proof of the character formula is a key step in proving that every dominant integral element actually arises as the highest weight of some irreducible representation. Important consequences of the character formula are the Weyl dimension formula and the Kostant multiplicity formula. By definition, the character \chi of a representation \pi of ''G'' is the trace of \pi(g), as a function of a group element g\in G. The irreducible representations in this case are all finite-dimensional (this is part of the Peter–Weyl theorem); so the notion of trace is the usual one from linear algebra. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
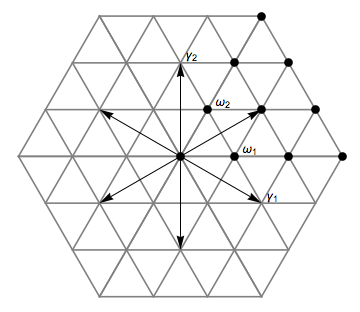
Positive Root
In mathematics, a root system is a configuration of vector space, vectors in a Euclidean space satisfying certain geometrical properties. The concept is fundamental in the theory of Lie groups and Lie algebras, especially the classification and representation theory of semisimple Lie algebras. Since Lie groups (and some analogues such as algebraic groups) and Lie algebras have become important in many parts of mathematics during the twentieth century, the apparently special nature of root systems belies the number of areas in which they are applied. Further, the classification scheme for root systems, by Dynkin diagrams, occurs in parts of mathematics with no overt connection to Lie theory (such as singularity theory). Finally, root systems are important for their own sake, as in spectral graph theory. Definitions and examples As a first example, consider the six vectors in 2-dimensional Euclidean space, R2, as shown in the image at the right; call them roots. These vectors Li ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |