|
Dynamic Discrete Choice
Dynamic discrete choice (DDC) models, also known as discrete choice models of dynamic programming, model an agent's choices over discrete options that have future implications. Rather than assuming observed choices are the result of static utility maximization, observed choices in DDC models are assumed to result from an agent's maximization of the present value of utility, generalizing the utility theory upon which discrete choice models are based. The goal of DDC methods is to estimate the structural parameters of the agent's decision process. Once these parameters are known, the researcher can then use the estimates to simulate how the agent would behave in a counterfactual state of the world. (For example, how a prospective college student's enrollment decision would change in response to a tuition increase.) Mathematical representation Agent n's maximization problem can be written mathematically as follows: : V\left(x_\right)=\max_ \mathbb \left(\sum_^T \sum_^J \beta^ \le ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dynamic Programming
Dynamic programming is both a mathematical optimization method and a computer programming method. The method was developed by Richard Bellman in the 1950s and has found applications in numerous fields, from aerospace engineering to economics. In both contexts it refers to simplifying a complicated problem by breaking it down into simpler sub-problems in a recursive manner. While some decision problems cannot be taken apart this way, decisions that span several points in time do often break apart recursively. Likewise, in computer science, if a problem can be solved optimally by breaking it into sub-problems and then recursively finding the optimal solutions to the sub-problems, then it is said to have ''optimal substructure''. If sub-problems can be nested recursively inside larger problems, so that dynamic programming methods are applicable, then there is a relation between the value of the larger problem and the values of the sub-problems.Cormen, T. H.; Leiserson, C. E.; Rives ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mixed Logit , a person who is of multiple races
*
*
{{disambiguation ...
Mixed is the past tense of ''mix''. Mixed may refer to: * Mixed (United Kingdom ethnicity category), an ethnicity category that has been used by the United Kingdom's Office for National Statistics since the 1991 Census * ''Mixed'' (album), a compilation album of two avant-garde jazz sessions featuring performances by the Cecil Taylor Unit and the Roswell Rudd Sextet See also * Mix (other) * Mixed breed, an animal whose parents are from different breeds or species * Mixed ethnicity Mixed race people are people of more than one race or ethnicity. A variety of terms have been used both historically and presently for mixed race people in a variety of contexts, including ''multiethnic'', ''polyethnic'', occasionally ''bi-eth ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
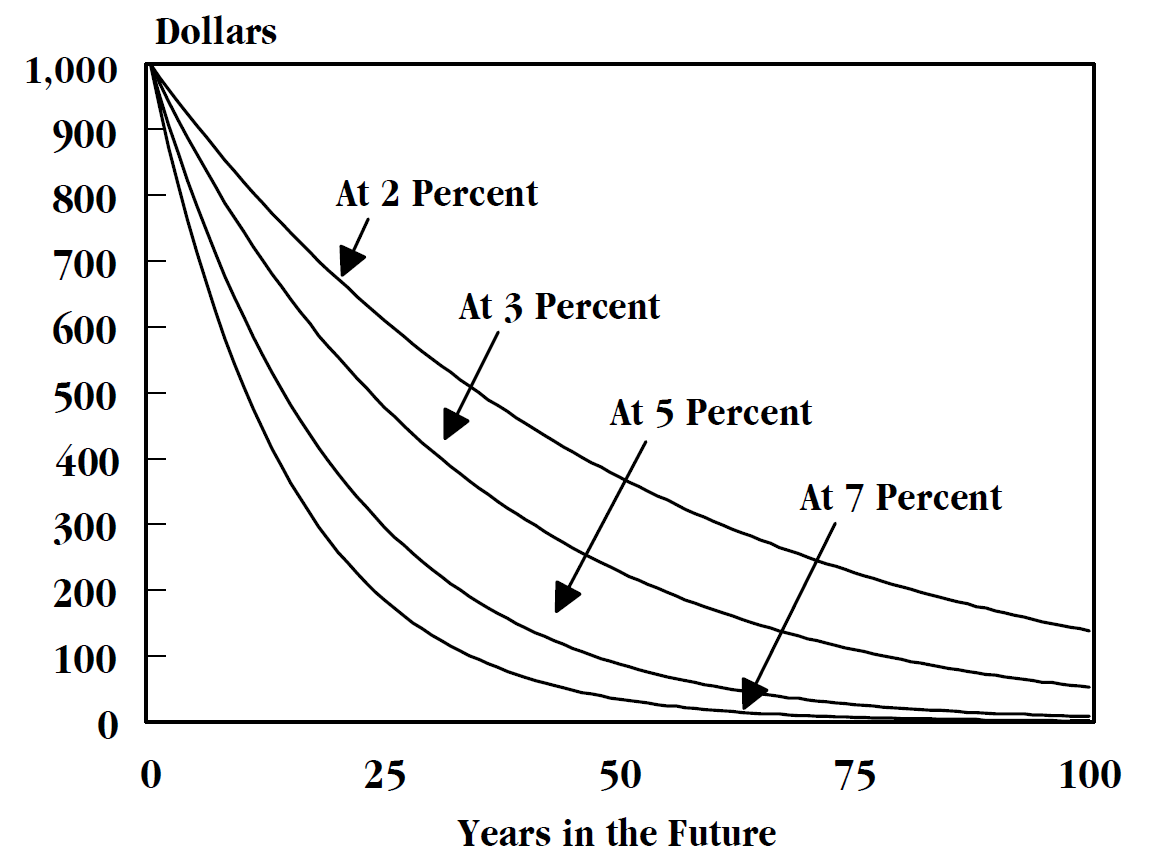
Discount Factor
Discounting is a financial mechanism in which a debtor obtains the right to delay payments to a creditor, for a defined period of time, in exchange for a charge or fee.See "Time Value", "Discount", "Discount Yield", "Compound Interest", "Efficient Market", "Market Value" and "Opportunity Cost" in Downes, J. and Goodman, J. E. ''Dictionary of Finance and Investment Terms'', Baron's Financial Guides, 2003. Essentially, the party that owes money in the present purchases the right to delay the payment until some future date.See "Discount", "Compound Interest", "Efficient Markets Hypothesis", "Efficient Resource Allocation", "Pareto-Optimality", "Price", "Price Mechanism" and "Efficient Market" in Black, John, ''Oxford Dictionary of Economics'', Oxford University Press, 2002. This transaction is based on the fact that most people prefer current interest to delayed interest because of mortality effects, impatience effects, and salience effects. The discount, or charge, is the difference ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Odometer
An odometer or odograph is an instrument used for measuring the distance traveled by a vehicle, such as a bicycle or car. The device may be electronic, mechanical, or a combination of the two (electromechanical). The noun derives from ancient Greek , ''hodómetron'', from , ''hodós'' ("path" or "gateway") and , ''métron'' ("measure"). Early forms of the odometer existed in the ancient Greco-Roman world as well as in ancient China. In countries using Imperial units or US customary units it is sometimes called a mileometer or milometer, the former name especially being prevalent in the United Kingdom and among members of the Commonwealth. History Classical Era Possibly the first evidence for the use of an odometer can be found in the works of the ancient Roman Pliny (NH 6. 61-62) and the ancient Greek Strabo (11.8.9). Both authors list the distances of routes traveled by Alexander the Great (r. 336-323 BC) as by his bematists Diognetus and Baeton. However, the high ac ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Engine
An engine or motor is a machine designed to convert one or more forms of energy into mechanical energy. Available energy sources include potential energy (e.g. energy of the Earth's gravitational field as exploited in hydroelectric power generation), heat energy (e.g. geothermal), chemical energy, electric potential and nuclear energy (from nuclear fission or nuclear fusion). Many of these processes generate heat as an intermediate energy form, so heat engines have special importance. Some natural processes, such as atmospheric convection cells convert environmental heat into motion (e.g. in the form of rising air currents). Mechanical energy is of particular importance in transportation, but also plays a role in many industrial processes such as cutting, grinding, crushing, and mixing. Mechanical heat engines convert heat into work via various thermodynamic processes. The internal combustion engine is perhaps the most common example of a mechanical heat engine, in which he ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Madison, Wisconsin
Madison is the county seat of Dane County and the capital city of the U.S. state of Wisconsin. As of the 2020 census the population was 269,840, making it the second-largest city in Wisconsin by population, after Milwaukee, and the 80th-largest in the U.S. The city forms the core of the Madison Metropolitan Area which includes Dane County and neighboring Iowa, Green, and Columbia counties for a population of 680,796. Madison is named for American Founding Father and President James Madison. The city is located on the traditional land of the Ho-Chunk, and the Madison area is known as ''Dejope'', meaning "four lakes", or ''Taychopera'', meaning "land of the four lakes", in the Ho-Chunk language. Located on an isthmus and lands surrounding four lakes—Lake Mendota, Lake Monona, Lake Kegonsa and Lake Waubesa—the city is home to the University of Wisconsin–Madison, the Wisconsin State Capitol, the Overture Center for the Arts, and the Henry Vilas Zoo. Madison is ho ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Madison Metro
Madison Metro Transit operates a bus service throughout the City of Madison, Wisconsin, United States and most of its immediate suburbs, including the City of Middleton, Fitchburg, Maple Bluff, Shorewood Hills, Sun Prairie, Verona, and a small portion of McFarland. System-wide, fixed route ridership was 13,385,628 in 2018. Metro Transit also provides supplemental transit services to some Madison middle and high schools. Several schools have switched to yellow buses in 2019. These routes are open to the general public, but have been designed to provide additional services during peak school times. Metro also serves the University of Wisconsin–Madison campus, Eagle Heights University apartments, and some off-campus residential areas, via routes 80, 81, 82, and 84. The "80 routes" are free of charge. Metro also connects with suburban mass transit services, such as the Monona Lift/Monona Express and Sun Prairie Shared Taxi Shuttle. Route network The route network focuses on h ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Optimal Stopping
In mathematics, the theory of optimal stopping or early stopping : (For French translation, secover storyin the July issue of ''Pour la Science'' (2009).) is concerned with the problem of choosing a time to take a particular action, in order to maximise an expected reward or minimise an expected cost. Optimal stopping problems can be found in areas of statistics, economics, and mathematical finance (related to the pricing of American options). A key example of an optimal stopping problem is the secretary problem. Optimal stopping problems can often be written in the form of a Bellman equation, and are therefore often solved using dynamic programming. Definition Discrete time case Stopping rule problems are associated with two objects: # A sequence of random variables X_1, X_2, \ldots, whose joint distribution is something assumed to be known # A sequence of 'reward' functions (y_i)_ which depend on the observed values of the random variables in 1: #: y_i=y_i (x_1, \ldots ,x ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Artelys Knitro
Artelys Knitro is a commercial software package for solving large scale nonlinear mathematical optimization problems. KNITRO – (the original solver name) short for "Nonlinear Interior point Trust Region Optimization" (the "K" is silent) – was co-created by Richard Waltz, Jorge Nocedal, Todd Plantenga and Richard Byrd. It was first introduced in 2001, as a derivative of academic research at Northwestern University (through Ziena Optimization LLC), and has since been continually improved by developers at Artelys. Optimization problems must be presented to Knitro in mathematical form, and should provide a way of computing function derivatives using sparse matrices (Knitro can compute derivatives approximation but in most cases providing the exact derivatives is beneficial). An often easier approach is to develop the optimization problem in an algebraic modeling language. The modeling environment computes function derivatives, and Knitro is called as a "solver" from within the env ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematical Programming With Equilibrium Constraints
Mathematical programming with equilibrium constraints (MPEC) is the study of constrained optimization problems where the constraints include variational inequalities or complementarities. MPEC is related to the Stackelberg game. MPEC is used in the study of engineering design, economic equilibrium, and multilevel games. MPEC is difficult to deal with because its feasible region is not necessarily convex or even connected Connected may refer to: Film and television * ''Connected'' (2008 film), a Hong Kong remake of the American movie ''Cellular'' * '' Connected: An Autoblogography About Love, Death & Technology'', a 2011 documentary film * ''Connected'' (2015 TV .... References * Z.-Q. Luo, J.-S. Pang and D. Ralph: ''Mathematical Programs with Equilibrium Constraints''. Cambridge University Press, 1996, . * B. Baumrucker, J. Renfro, L. T. Biegler, MPEC problem formulations and solution strategies with chemical engineering applications, Computers & Chemical Engineering, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Constrained Optimization
In mathematical optimization, constrained optimization (in some contexts called constraint optimization) is the process of optimizing an objective function with respect to some variables in the presence of constraints on those variables. The objective function is either a cost function or energy function, which is to be minimized, or a reward function or utility function, which is to be maximized. Constraints can be either hard constraints, which set conditions for the variables that are required to be satisfied, or soft constraints, which have some variable values that are penalized in the objective function if, and based on the extent that, the conditions on the variables are not satisfied. Relation to constraint-satisfaction problems The constrained-optimization problem (COP) is a significant generalization of the classic constraint-satisfaction problem (CSP) model. COP is a CSP that includes an ''objective function'' to be optimized. Many algorithms are used to handle ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kenneth Judd
Kenneth Lewis Judd (born March 24, 1953) is a computational economist at Stanford University, where he is the Paul H. Bauer Senior Fellow at the Hoover Institution. He received his PhD in economics from the University of Wisconsin in 1980. He is perhaps best known as the author of ''Numerical Methods in Economics'', and he is also among the editors of the ''Handbook of Computational Economics'' and of the ''Journal of Economic Dynamics and Control The ''Journal of Economic Dynamics and Control ''(JEDC) is a peer-reviewed scholarly journal devoted to computational economics, dynamic economic models, and macroeconomics. It is edited at the University of Amsterdam and published by Elsevier ...''. He is one of two authors behind the Chamley–Judd result that the optimal tax rate on capital income is zero. References External links Kenneth Judd's homepage* * Kenneth L. Judd (1980''Four Essays in Economic Theory'' Ph.D. dissertation, University of Wisconsin-Madison. * Je ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
