|
Category Of Medial Magmas
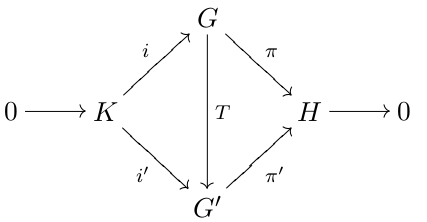
In mathematics, the category of medial magmas, also known as the medial category, and denoted Med, is the category whose objects are medial magmas (that is, sets with a medial binary operation), and whose morphisms are magma homomorphisms (which are equivalent to homomorphisms in the sense of universal algebra). The category Med has direct products, so the concept of a medial magma object (internal binary operation) makes sense. As a result, Med has all its objects as ''medial objects'', and this characterizes it. There is an inclusion functor from Set to Med as trivial magmas, with operations being the ''right'' projections : (''x'', ''y'') → ''y''. An injective endomorphism can be extended to an automorphism of a magma extension—the colimit of the constant sequence of the endomorphism. See also * Eckmann–Hilton argument In mathematics, the Eckmann–Hilton argument (or Eckmann–Hilton principle or Eckmann–Hilton theorem) is an argument about two unital magm ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics with the major subdisciplines of number theory, algebra, geometry, and analysis, respectively. There is no general consensus among mathematicians about a common definition for their academic discipline. Most mathematical activity involves the discovery of properties of abstract objects and the use of pure reason to prove them. These objects consist of either abstractions from nature orin modern mathematicsentities that are stipulated to have certain properties, called axioms. A ''proof'' consists of a succession of applications of deductive rules to already established results. These results include previously proved theorems, axioms, andin case of abstraction from naturesome basic properties that are considered true starting points of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Inclusion Functor
In mathematics, specifically category theory, a subcategory of a category ''C'' is a category ''S'' whose objects are objects in ''C'' and whose morphisms are morphisms in ''C'' with the same identities and composition of morphisms. Intuitively, a subcategory of ''C'' is a category obtained from ''C'' by "removing" some of its objects and arrows. Formal definition Let ''C'' be a category. A subcategory ''S'' of ''C'' is given by *a subcollection of objects of ''C'', denoted ob(''S''), *a subcollection of morphisms of ''C'', denoted hom(''S''). such that *for every ''X'' in ob(''S''), the identity morphism id''X'' is in hom(''S''), *for every morphism ''f'' : ''X'' → ''Y'' in hom(''S''), both the source ''X'' and the target ''Y'' are in ob(''S''), *for every pair of morphisms ''f'' and ''g'' in hom(''S'') the composite ''f'' o ''g'' is in hom(''S'') whenever it is defined. These conditions ensure that ''S'' is a category in its own right: its collection of objects is ob(''S ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sequence
In mathematics, a sequence is an enumerated collection of objects in which repetitions are allowed and order matters. Like a set, it contains members (also called ''elements'', or ''terms''). The number of elements (possibly infinite) is called the ''length'' of the sequence. Unlike a set, the same elements can appear multiple times at different positions in a sequence, and unlike a set, the order does matter. Formally, a sequence can be defined as a function from natural numbers (the positions of elements in the sequence) to the elements at each position. The notion of a sequence can be generalized to an indexed family, defined as a function from an ''arbitrary'' index set. For example, (M, A, R, Y) is a sequence of letters with the letter 'M' first and 'Y' last. This sequence differs from (A, R, M, Y). Also, the sequence (1, 1, 2, 3, 5, 8), which contains the number 1 at two different positions, is a valid sequence. Sequences can be ''finite'', as in these examples, or ''infi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Constant (mathematics)
In mathematics, the word constant conveys multiple meanings. As an adjective, it refers to non-variance (i.e. unchanging with respect to some other value); as a noun, it has two different meanings: * A fixed and well-defined number or other non-changing mathematical object. The terms '' mathematical constant'' or '' physical constant'' are sometimes used to distinguish this meaning. * A function whose value remains unchanged (i.e., a constant function). Such a constant is commonly represented by a variable which does not depend on the main variable(s) in question. For example, a general quadratic function is commonly written as: :a x^2 + b x + c\, , where , and are constants (or parameters), and a variable—a placeholder for the argument of the function being studied. A more explicit way to denote this function is :x\mapsto a x^2 + b x + c \, , which makes the function-argument status of (and by extension the constancy of , and ) clear. In this example , and are co ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Colimit
In category theory, a branch of mathematics, the abstract notion of a limit captures the essential properties of universal constructions such as products, pullbacks and inverse limits. The dual notion of a colimit generalizes constructions such as disjoint unions, direct sums, coproducts, pushouts and direct limits. Limits and colimits, like the strongly related notions of universal properties and adjoint functors, exist at a high level of abstraction. In order to understand them, it is helpful to first study the specific examples these concepts are meant to generalize. Definition Limits and colimits in a category C are defined by means of diagrams in C. Formally, a diagram of shape J in C is a functor from J to C: :F:J\to C. The category J is thought of as an index category, and the diagram F is thought of as indexing a collection of objects and morphisms in C patterned on J. One is most often interested in the case where the category J is a small or even finite category. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Extension (algebra)
In mathematics, a group extension is a general means of describing a group (mathematics), group in terms of a particular normal subgroup and quotient group. If Q and N are two groups, then G is an extension of Q by N if there is a short exact sequence :1\to N\;\overset\;G\;\overset\;Q \to 1. If G is an extension of Q by N, then G is a group, \iota(N) is a normal subgroup of G and the quotient group G/\iota(N) is isomorphic to the group Q. Group extensions arise in the context of the extension problem, where the groups Q and N are known and the properties of G are to be determined. Note that the phrasing "G is an extension of N by Q" is also used by some. Since any finite group G possesses a maximal normal subgroup N with simple group, simple factor group G/N, all finite groups may be constructed as a series of extensions with finite simple groups. This fact was a motivation for completing the classification of finite simple groups. An extension is called a central extension if ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Automorphism
In mathematics, an automorphism is an isomorphism from a mathematical object to itself. It is, in some sense, a symmetry of the object, and a way of mapping the object to itself while preserving all of its structure. The set of all automorphisms of an object forms a group, called the automorphism group. It is, loosely speaking, the symmetry group of the object. Definition In the context of abstract algebra, a mathematical object is an algebraic structure such as a group, ring, or vector space. An automorphism is simply a bijective homomorphism of an object with itself. (The definition of a homomorphism depends on the type of algebraic structure; see, for example, group homomorphism, ring homomorphism, and linear operator.) The identity morphism (identity mapping) is called the trivial automorphism in some contexts. Respectively, other (non-identity) automorphisms are called nontrivial automorphisms. The exact definition of an automorphism depends on the type of "mathematical ob ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Endomorphism
In mathematics, an endomorphism is a morphism from a mathematical object to itself. An endomorphism that is also an isomorphism is an automorphism. For example, an endomorphism of a vector space is a linear map , and an endomorphism of a group is a group homomorphism . In general, we can talk about endomorphisms in any category. In the category of sets, endomorphisms are functions from a set ''S'' to itself. In any category, the composition of any two endomorphisms of is again an endomorphism of . It follows that the set of all endomorphisms of forms a monoid, the full transformation monoid, and denoted (or to emphasize the category ). Automorphisms An invertible endomorphism of is called an automorphism. The set of all automorphisms is a subset of with a group structure, called the automorphism group of and denoted . In the following diagram, the arrows denote implication: Endomorphism rings Any two endomorphisms of an abelian group, , can be added toge ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Injective
In mathematics, an injective function (also known as injection, or one-to-one function) is a function that maps distinct elements of its domain to distinct elements; that is, implies . (Equivalently, implies in the equivalent contrapositive statement.) In other words, every element of the function's codomain is the image of one element of its domain. The term must not be confused with that refers to bijective functions, which are functions such that each element in the codomain is an image of exactly one element in the domain. A homomorphism between algebraic structures is a function that is compatible with the operations of the structures. For all common algebraic structures, and, in particular for vector spaces, an is also called a . However, in the more general context of category theory, the definition of a monomorphism differs from that of an injective homomorphism. This is thus a theorem that they are equivalent for algebraic structures; see for more details. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Projection (mathematics)
In mathematics, a projection is a mapping of a set (or other mathematical structure) into a subset (or sub-structure), which is equal to its square for mapping composition, i.e., which is idempotent. The restriction to a subspace of a projection is also called a ''projection'', even if the idempotence property is lost. An everyday example of a projection is the casting of shadows onto a plane (sheet of paper): the projection of a point is its shadow on the sheet of paper, and the projection (shadow) of a point on the sheet of paper is that point itself (idempotency). The shadow of a three-dimensional sphere is a closed disk. Originally, the notion of projection was introduced in Euclidean geometry to denote the projection of the three-dimensional Euclidean space onto a plane in it, like the shadow example. The two main projections of this kind are: * The projection from a point onto a plane or central projection: If ''C'' is a point, called the center of projection, then the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Magma Object
Magma () is the molten or semi-molten natural material from which all igneous rocks are formed. Magma is found beneath the surface of the Earth, and evidence of magmatism has also been discovered on other terrestrial planets and some natural satellites. Besides molten rock, magma may also contain suspended crystals and volcanic gas, gas bubbles. Magma is produced by melting of the mantle (geology), mantle or the Crust (geology), crust in various tectonics, tectonic settings, which on Earth include subduction zones, continental rift (geology), rift zones, mid-ocean ridges and Hotspot (geology), hotspots. Mantle and crustal melts migrate upwards through the crust where they are thought to be stored in magma chambers or trans-crustal crystal mush, crystal-rich mush zones. During magma's storage in the crust, its composition may be modified by Fractional crystallization (geology), fractional crystallization, contamination with crustal melts, magma mixing, and degassing. Following it ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Category (mathematics)
In mathematics, a category (sometimes called an abstract category to distinguish it from a concrete category) is a collection of "objects" that are linked by "arrows". A category has two basic properties: the ability to compose the arrows associatively and the existence of an identity arrow for each object. A simple example is the category of sets, whose objects are sets and whose arrows are functions. '' Category theory'' is a branch of mathematics that seeks to generalize all of mathematics in terms of categories, independent of what their objects and arrows represent. Virtually every branch of modern mathematics can be described in terms of categories, and doing so often reveals deep insights and similarities between seemingly different areas of mathematics. As such, category theory provides an alternative foundation for mathematics to set theory and other proposed axiomatic foundations. In general, the objects and arrows may be abstract entities of any kind, and the n ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |