|
Continuous Spectrum
In the physical sciences, the term ''spectrum'' was introduced first into optics by Isaac Newton in the 17th century, referring to the range of colors observed when white light was dispersion (optics), dispersed through a prism (optics), prism. Soon the term referred to a plot of light intensity (physics), intensity or power (physics), power as a function of frequency or wavelength, also known as a ''spectral density plot''. Later it expanded to apply to other waves, such as sound waves and sea waves that could also be measured as a function of frequency (e.g., noise spectrum, sea wave spectrum). It has also been expanded to more abstract "signals", whose power spectrum can be spectrum analyzer, analyzed and signal processing, processed. The term now applies to any signal that can be measured or decomposed along a continuous variable, such as energy in electron spectroscopy or mass-to-charge ratio in mass spectrometry. Spectrum is also used to refer to a graphical representation ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
EM Spectrum Properties Edit
EM, Em or em may refer to: Arts and entertainment Music * Em, the E minor musical scale * Em, the E minor chord * Electronic music, music that employs electronic musical instruments and electronic music technology in its production * Encyclopedia Metallum, an online metal music database * Eminem, American rapper Other uses in arts and entertainment * Em (comic strip), ''Em'' (comic strip), a comic strip by Maria Smedstad Companies and organizations * Em (restaurant), a restaurant in Mexico City * Aero Benin (IATA code), a defunct airline * Empire Airlines (IATA code), a charter and cargo airline based in Idaho, US * Erasmus Mundus, an international student-exchange program * ''Estado de Minas'', a Brazilian newspaper * European Movement, an international lobbying association * ExxonMobil, a large oil company formed from the merger of Exxon and Mobil in 1999 * La République En Marche! (sometimes shortened to "En Marche!"), a major French political party Economics * Emerging mar ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sea Wave Spectrum
In fluid dynamics, a wind wave, or wind-generated water wave, is a surface wave that occurs on the free surface of bodies of water as a result of the wind blowing over the water's surface. The contact distance in the direction of the wind is known as the '' fetch''. Waves in the oceans can travel thousands of kilometers before reaching land. Wind waves on Earth range in size from small ripples to waves over high, being limited by wind speed, duration, fetch, and water depth. When directly generated and affected by local wind, a wind wave system is called a wind sea. Wind waves will travel in a great circle route after being generated – curving slightly left in the southern hemisphere and slightly right in the northern hemisphere. After moving out of the area of fetch and no longer being affected by the local wind, wind waves are called '' swells'' and can travel thousands of kilometers. A noteworthy example of this is waves generated south of Tasmania during heavy win ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
1 E-7 M
The following are examples of orders of magnitude for different lengths. Overview Detailed list To help compare different orders of magnitude, the following list describes various lengths between 1.6 \times 10^ metres and 10^metres. Subatomic scale Atomic to cellular scale Cellular to human scale Human to astronomical scale Astronomical scale 1 quectometre and less The ' ( SI symbol: ') is a unit of length in the metric system equal to . To help compare different orders of magnitude, this section lists lengths shorter than 10−30 m (1 qm). *1.6 × 10−5 quectometres (1.6 × 10−35 metres) – the Planck length (Measures of distance shorter than this do not make physical sense, according to current theories of physics.) *1 qm – 1 quectometre, the smallest named subdivision of the metre in the SI base unit of length, one nonillionth of a metre. 1 rontometre The ' ( SI symbol: ') is a unit of length in the metric system equal to . *1 rm � ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Human Eye
The human eye is a sensory organ in the visual system that reacts to light, visible light allowing eyesight. Other functions include maintaining the circadian rhythm, and Balance (ability), keeping balance. The eye can be considered as a living optics, optical device. It is approximately spherical in shape, with its outer layers, such as the outermost, white part of the eye (the sclera) and one of its inner layers (the pigmented choroid) keeping the eye essentially stray light, light tight except on the eye's optic axis. In order, along the optic axis, the optical components consist of a first lens (the cornea, cornea—the clear part of the eye) that accounts for most of the optical power of the eye and accomplishes most of the Focus (optics), focusing of light from the outside world; then an aperture (the pupil) in a Diaphragm (optics), diaphragm (the Iris (anatomy), iris—the coloured part of the eye) that controls the amount of light entering the interior of the eye; then an ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Visible Spectrum
The visible spectrum is the spectral band, band of the electromagnetic spectrum that is visual perception, visible to the human eye. Electromagnetic radiation in this range of wavelengths is called ''visible light'' (or simply light). The optical spectrum is sometimes considered to be the same as the visible spectrum, but some authors define the term more broadly, to include the ultraviolet and infrared parts of the electromagnetic spectrum as well, known collectively as ''optical radiation''. A typical human eye will respond to wavelengths from about 380 to about 750 nanometers. In terms of frequency, this corresponds to a band in the vicinity of 400–790 Terahertz (unit), terahertz. These boundaries are not sharply defined and may vary per individual. Under optimal conditions, these limits of human perception can extend to 310 nm (ultraviolet) and 1100 nm (near infrared). The spectrum does not contain all the colors that the human visual system can distinguish. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Spectrometer
A spectrometer () is a scientific instrument used to separate and measure Spectrum, spectral components of a physical phenomenon. Spectrometer is a broad term often used to describe instruments that measure a continuous variable of a phenomenon where the spectral components are somehow mixed. In visible light a spectrometer can separate white light and measure individual narrow bands of color, called a spectrum. A mass spectrometer measures the spectrum of the masses of the atoms or molecules present in a gas. The first spectrometers were used to split light into an array of separate colors. Spectrometers were History_of_spectroscopy, developed in early studies of physics, astronomy, and chemistry. The capability of spectroscopy to determine Analytical_chemistry#Spectroscopy, chemical composition drove its advancement and continues to be one of its primary uses. Spectrometers are used in Astronomical spectroscopy, astronomy to analyze the chemical composition of Astronomical_spe ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Spectrograph
An optical spectrometer (spectrophotometer, spectrograph or spectroscope) is an instrument used to measure properties of light over a specific portion of the electromagnetic spectrum, typically used in spectroscopic analysis to identify materials. The variable measured is most often the irradiance of the light but could also, for instance, be the polarization state. The independent variable is usually the wavelength of the light or a closely derived physical quantity, such as the corresponding wavenumber or the photon energy, in units of measurement such as centimeters, reciprocal centimeters, or electron volts, respectively. A spectrometer is used in spectroscopy for producing spectral lines and measuring their wavelengths and intensities. Spectrometers may operate over a wide range of non-optical wavelengths, from gamma rays and X-rays into the far infrared. If the instrument is designed to measure the spectrum on an absolute scale rather than a relative one, then it ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Electromagnetic Radiation
In physics, electromagnetic radiation (EMR) is a self-propagating wave of the electromagnetic field that carries momentum and radiant energy through space. It encompasses a broad spectrum, classified by frequency or its inverse, wavelength, ranging from radio waves, microwaves, infrared, visible light, ultraviolet, X-rays, and gamma rays. All forms of EMR travel at the speed of light in a vacuum and exhibit wave–particle duality, behaving both as waves and as discrete particles called photons. Electromagnetic radiation is produced by accelerating charged particles such as from the Sun and other celestial bodies or artificially generated for various applications. Its interaction with matter depends on wavelength, influencing its uses in communication, medicine, industry, and scientific research. Radio waves enable broadcasting and wireless communication, infrared is used in thermal imaging, visible light is essential for vision, and higher-energy radiation, such ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fluorescent Lighting Spectrum Peaks Labelled
Fluorescence is one of two kinds of photoluminescence, the emission of light by a substance that has absorbed light or other electromagnetic radiation. When exposed to ultraviolet radiation, many substances will glow (fluoresce) with colored visible light. The color of the light emitted depends on the chemical composition of the substance. Fluorescent materials generally cease to glow nearly immediately when the radiation source stops. This distinguishes them from the other type of light emission, phosphorescence. Phosphorescent materials continue to emit light for some time after the radiation stops. This difference in duration is a result of quantum spin effects. Fluorescence occurs when a photon from incoming radiation is absorbed by a molecule, exciting it to a higher energy level, followed by the emission of light as the molecule returns to a lower energy state. The emitted light may have a longer wavelength and, therefore, a lower photon energy than the absorbed rad ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mass Spectrometry
Mass spectrometry (MS) is an analytical technique that is used to measure the mass-to-charge ratio of ions. The results are presented as a ''mass spectrum'', a plot of intensity as a function of the mass-to-charge ratio. Mass spectrometry is used in many different fields and is applied to pure samples as well as complex mixtures. A mass spectrum is a type of plot of the ion signal as a function of the mass-to-charge ratio. These spectra are used to determine the elemental or isotopic signature of a sample, the masses of particles and of molecules, and to elucidate the chemical identity or structure of molecules and other chemical compounds. In a typical MS procedure, a sample, which may be solid, liquid, or gaseous, is ionization, ionized, for example by bombarding it with a Electron ionization, beam of electrons. This may cause some of the sample's molecules to break up into positively charged fragments or simply become positively charged without fragmenting. These ions (fragmen ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
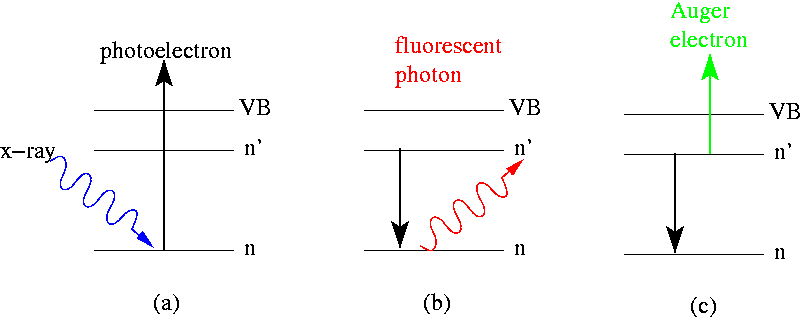
Electron Spectroscopy
Electron spectroscopy refers to a group formed by techniques based on the analysis of the energies of emitted electrons such as Photoelectric effect, photoelectrons and Auger electrons. This group includes X-ray photoelectron spectroscopy (XPS), which also known as Electron Spectroscopy for Chemical Analysis (ESCA), Electron energy loss spectroscopy (EELS), Ultraviolet photoelectron spectroscopy (UPS), and Auger electron spectroscopy (AES). These analytical techniques are used to identify and determine the elements and their electronic structures from the surface of a test sample. Samples can be solids, gases or liquids.Yang Leng; ''Materials Characterization: Introduction to Microscopic and Spectroscopic Methods (Second Edition)''; Publisher John Wiley & Sons, Incorporated 2013; p: 191-192, 221-224.Daintith, J.; ''Dictionary of Chemistry (6th Edition)''; Oxford University Press, 2008; p: 191, 416, 541 Chemical information is obtained only from the uppermost atomic layers of the sam ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
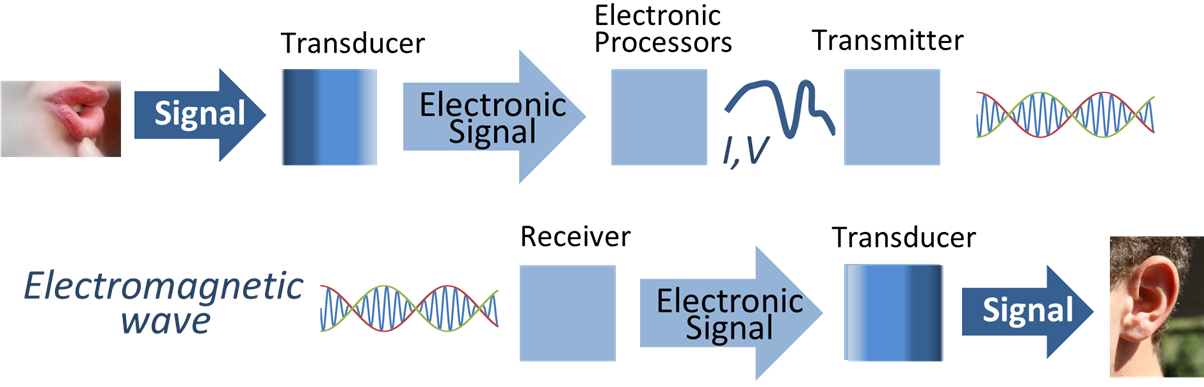
Signal Processing
Signal processing is an electrical engineering subfield that focuses on analyzing, modifying and synthesizing ''signals'', such as audio signal processing, sound, image processing, images, Scalar potential, potential fields, Seismic tomography, seismic signals, Altimeter, altimetry processing, and scientific measurements. Signal processing techniques are used to optimize transmissions, Data storage, digital storage efficiency, correcting distorted signals, improve subjective video quality, and to detect or pinpoint components of interest in a measured signal. History According to Alan V. Oppenheim and Ronald W. Schafer, the principles of signal processing can be found in the classical numerical analysis techniques of the 17th century. They further state that the digital refinement of these techniques can be found in the digital control systems of the 1940s and 1950s. In 1948, Claude Shannon wrote the influential paper "A Mathematical Theory of Communication" which was publis ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |