|
Compound Of Five Octahedra
The polyhedral compound, compound of five octahedra is one of the five regular polyhedron compounds. This polyhedron can be seen as either a polyhedral stellation or a Polyhedron compound, compound. This compound was first described by Edmund Hess in 1876. It is unique among the regular compounds for not having a regular convex hull. As a stellation It is the second stellation of the icosahedron, and given as List of Wenninger polyhedron models#Stellations of icosahedron, Wenninger model index 23. It can be constructed by a rhombic triacontahedron with rhombic-based Pyramid (geometry), pyramids added to all the faces, as shown by the five colored model image. (This construction does not generate the ''regular'' compound of five octahedra, but shares the same topology and can be smoothly deformed into the regular compound.) It has a density of greater than 1. As a compound It can also be seen as a polyhedral compound of five Octahedron, octahedra arranged in icosahedral s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Compound Of Five Octahedra
The compound of five octahedra is one of the five regular polyhedron compounds. This polyhedron can be seen as either a polyhedral stellation or a compound. This compound was first described by Edmund Hess in 1876. It is unique among the regular compounds for not having a regular convex hull. As a stellation It is the second stellation of the icosahedron, and given as Wenninger model index 23. It can be constructed by a rhombic triacontahedron with rhombic-based pyramids added to all the faces, as shown by the five colored model image. (This construction does not generate the ''regular'' compound of five octahedra, but shares the same topology and can be smoothly deformed into the regular compound.) It has a density of greater than 1. As a compound It can also be seen as a polyhedral compound of five octahedra arranged in icosahedral symmetry (Ih). The spherical and stereographic projections of this compound look the same as those of the disdyakis triacontahedron. But ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
List Of Wenninger Polyhedron Models
This is an indexed list of the uniform and stellated polyhedra from the book ''Polyhedron Models'', by Magnus Wenninger. The book was written as a guide book to building polyhedra as physical models. It includes templates of face elements for construction and helpful hints in building, and also brief descriptions on the theory behind these shapes. It contains the 75 nonprismatic Uniform polyhedron, uniform polyhedra, as well as 44 Stellation, stellated forms of the convex regular and quasiregular polyhedra. Models listed here can be cited as "Wenninger Model Number ''N''", or ''W''''N'' for brevity. The polyhedra are grouped in 5 tables: Regular (1–5), Semiregular (6–18), regular star polyhedra (20–22,41), Stellations and compounds (19–66), and uniform star polyhedra (67–119). ''The four regular star polyhedra are listed twice because they belong to both the uniform polyhedra and stellation groupings.'' Platonic solids (regular convex polyhedra) W1 to W5 Archimedean ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
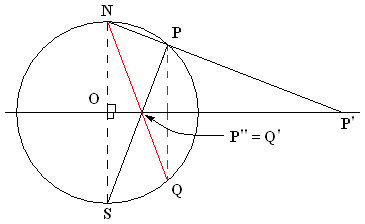
Stereographic Projection
In mathematics, a stereographic projection is a perspective projection of the sphere, through a specific point on the sphere (the ''pole'' or ''center of projection''), onto a plane (geometry), plane (the ''projection plane'') perpendicular to the diameter through the point. It is a smooth function, smooth, bijection, bijective function (mathematics), function from the entire sphere except the center of projection to the entire plane. It maps circle of a sphere, circles on the sphere to generalised circle, circles or lines on the plane, and is conformal map, conformal, meaning that it preserves angles at which curves meet and thus Local property, locally approximately preserves similarity (geometry), shapes. It is neither isometry, isometric (distance preserving) nor Equiareal map, equiareal (area preserving). The stereographic projection gives a way to representation (mathematics), represent a sphere by a plane. The metric tensor, metric induced metric, induced by the inverse s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Spherical Polyhedron
In geometry, a spherical polyhedron or spherical tiling is a tessellation, tiling of the sphere in which the surface is divided or partitioned by great arcs into bounded regions called spherical polygons. Much of the theory of symmetrical polyhedron, polyhedra is most conveniently derived in this way. The most familiar spherical polyhedron is the Ball (association football), soccer ball, thought of as a spherical truncated icosahedron. The next most popular spherical polyhedron is the beach ball, thought of as a hosohedron. Some #Improper_cases, "improper" polyhedra, such as hosohedron, hosohedra and their dual polyhedron, duals, dihedron, dihedra, exist as spherical polyhedra, but their flat-faced analogs are Degeneracy (mathematics), degenerate. The example hexagonal beach ball, is a hosohedron, and is its dual dihedron. History The first known man-made polyhedra are spherical polyhedra stone carving, carved in stone. Many have been found in Scotland, and appear to date fr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Icosahedral Symmetry
In mathematics, and especially in geometry, an object has icosahedral symmetry if it has the same symmetries as a regular icosahedron. Examples of other polyhedra with icosahedral symmetry include the regular dodecahedron (the dual of the icosahedron) and the rhombic triacontahedron. Every polyhedron with icosahedral symmetry has 60 rotational (or orientation-preserving) symmetries and 60 orientation-reversing symmetries (that combine a rotation and a reflection), for a total symmetry order of 120. The full symmetry group is the Coxeter group of type . It may be represented by Coxeter notation and Coxeter diagram . The set of rotational symmetries forms a subgroup that is isomorphic to the alternating group on 5 letters. Description Icosahedral symmetry is a mathematical property of objects indicating that an object has the same symmetries as a regular icosahedron. As point group Apart from the two infinite series of prismatic and antiprismatic symmetry, rotati ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Icosidodecahedron
In geometry, an icosidodecahedron is a polyhedron with twenty (''icosi'') triangular faces and twelve (''dodeca'') pentagonal faces. An icosidodecahedron has 30 identical vertices, with two triangles and two pentagons meeting at each, and 60 identical edges, each separating a triangle from a pentagon. As such it is one of the Archimedean solids and more particularly, a quasiregular polyhedron. Geometry An icosidodecahedron has icosahedral symmetry, and its first stellation is the compound of a dodecahedron and its dual icosahedron, with the vertices of the icosidodecahedron located at the midpoints of the edges of either. Its dual polyhedron is the rhombic triacontahedron. An icosidodecahedron can be split along any of six planes to form a pair of pentagonal rotundae, which belong among the Johnson solids. The icosidodecahedron can be considered a ''pentagonal gyrobirotunda'', as a combination of two rotundae (compare pentagonal orthobirotunda, one of the Johnson solids) ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stellations Of Icosahedron
''The Fifty-Nine Icosahedra'' is a book written and illustrated by H. S. M. Coxeter, P. Du Val, H. T. Flather and J. F. Petrie. It enumerates certain stellations of the regular convex or Platonic icosahedron, according to a set of rules put forward by J. C. P. Miller. First published by the University of Toronto in 1938, a Second Edition reprint by Springer-Verlag followed in 1982. Tarquin's 1999 Third Edition included new reference material and photographs by K. and D. Crennell. Authors' contributions Miller's rules Although Miller did not contribute to the book directly, he was a close colleague of Coxeter and Petrie. His contribution is immortalised in his set of rules for defining which stellation forms should be considered "properly significant and distinct": :''(i) The faces must lie in twenty planes, viz., the bounding planes of the regular icosahedron.'' :''(ii) All parts composing the faces must be the same in each plane, although they may be quite disconnected.'' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Icosahedron
In geometry, an icosahedron ( or ) is a polyhedron with 20 faces. The name comes and . The plural can be either "icosahedra" () or "icosahedrons". There are infinitely many non- similar shapes of icosahedra, some of them being more symmetrical than others. The best known is the (convex, non- stellated) regular icosahedron—one of the Platonic solids—whose faces are 20 equilateral triangles. Regular icosahedra There are two objects, one convex and one nonconvex, that can both be called regular icosahedra. Each has 30 edges and 20 equilateral triangle faces with five meeting at each of its twelve vertices. Both have icosahedral symmetry. The term "regular icosahedron" generally refers to the convex variety, while the nonconvex form is called a ''great icosahedron''. Convex regular icosahedron The convex regular icosahedron is usually referred to simply as the ''regular icosahedron'', one of the five regular Platonic solids, and is represented by its Schläfli symbol , con ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Compound Of Five Octahedra Stellation Facets
Compound may refer to: Architecture and built environments * Compound (enclosure), a cluster of buildings having a shared purpose, usually inside a fence or wall ** Compound (fortification), a version of the above fortified with defensive structures * Compound (migrant labour), a hostel for migrant workers such as those historically connected with mines in South Africa * The Compound, an area of Palm Bay, Florida, US * Komboni or compound, a type of slum in Zambia Government and law * Composition (fine), a legal procedure in use after the English Civil War ** Committee for Compounding with Delinquents, an English Civil War institution that allowed Parliament to compound the estates of Royalists * Compounding treason, an offence under the common law of England * Compounding a felony, a previous offense under the common law of England Linguistics * Compound (linguistics), a word that consists of more than one radical element * Compound sentence (linguistics), a type of sentence ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Convex Hull
In geometry, the convex hull or convex envelope or convex closure of a shape is the smallest convex set that contains it. The convex hull may be defined either as the intersection of all convex sets containing a given subset of a Euclidean space, or equivalently as the set of all convex combinations of points in the subset. For a bounded subset of the plane, the convex hull may be visualized as the shape enclosed by a rubber band stretched around the subset. Convex hulls of open sets are open, and convex hulls of compact sets are compact. Every compact convex set is the convex hull of its extreme points. The convex hull operator is an example of a closure operator, and every antimatroid can be represented by applying this closure operator to finite sets of points. The algorithmic problems of finding the convex hull of a finite set of points in the plane or other low-dimensional Euclidean spaces, and its dual problem of intersecting half-spaces, are fundamental problems of com ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stellation
In geometry, stellation is the process of extending a polygon in two dimensions, polyhedron in three dimensions, or, in general, a polytope in ''n'' dimensions to form a new figure. Starting with an original figure, the process extends specific elements such as its edges or face planes, usually in a symmetrical way, until they meet each other again to form the closed boundary of a new figure. The new figure is a stellation of the original. The word ''stellation'' comes from the Latin ''stellātus'', "starred", which in turn comes from Latin ''stella'', "star". Stellation is the reciprocal or dual process to ''faceting''. Kepler's definition In 1619 Kepler defined stellation for polygons and polyhedra as the process of extending edges or faces until they meet to form a new polygon or polyhedron. He stellated the regular dodecahedron to obtain two regular star polyhedra, the small stellated dodecahedron and great stellated dodecahedron. He also stellated the regular octahedron to o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |