|
Alexander–Briggs Notation
In the mathematical field of topology, knot theory is the study of mathematical knots. While inspired by knots which appear in daily life, such as those in shoelaces and rope, a mathematical knot differs in that the ends are joined so it cannot be undone, the simplest knot being a ring (or "unknot"). In mathematical language, a knot is an embedding of a circle in 3-dimensional Euclidean space, \mathbb^3 (in topology, a circle is not bound to the classical geometric concept, but to all of its homeomorphisms). Two mathematical knots are equivalent if one can be transformed into the other via a deformation of \mathbb^3 upon itself (known as an ambient isotopy); these transformations correspond to manipulations of a knotted string that do not involve cutting it or passing through itself. Knots can be described in various ways. Using different description methods, there may be more than one description of the same knot. For example, a common method of describing a knot is a planar di ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tabela De Nós Matemáticos 01, Crop
''Durio graveolens,'' sometimes called the red-fleshed durian, orange-fleshed durian, or yellow durian, is a species of tree in the family Malvaceae. It is one of six species of durian named by Italian naturalist Odoardo Beccari. The specific epithet ('strong smelling' or 'rank') is due to the odor. Although most species of ''Durio'' (most notably ''Durio dulcis'') have a strong scent, the red-fleshed type of ''D. graveolens'' has a mild scent. It is native to Southeast Asia. ''D. graveolens'' is an edible durian, perhaps the most popular 'wild' species of durian, and it is sold commercially regionally. However, its Congener (biology), congener ''Durio zibethinus'' is the typical species eaten and dominates sales worldwide. Names This species should not be confused with the popular durian clones from Malaysia known as 'Red Flesh' (D164) and 'Red Prawn' (D175), as both of those belong to ''D. zibethinus''. However, ''D. graveolens'' does have one registered variety, 'DQ2 nye ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
3-manifold
In mathematics, a 3-manifold is a space that locally looks like Euclidean 3-dimensional space. A 3-manifold can be thought of as a possible shape of the universe. Just as a sphere looks like a plane to a small enough observer, all 3-manifolds look like our universe does to a small enough observer. This is made more precise in the definition below. Introduction Definition A topological space ''X'' is a 3-manifold if it is a second-countable Hausdorff space and if every point in ''X'' has a neighbourhood that is homeomorphic to Euclidean 3-space. Mathematical theory of 3-manifolds The topological, piecewise-linear, and smooth categories are all equivalent in three dimensions, so little distinction is made in whether we are dealing with say, topological 3-manifolds, or smooth 3-manifolds. Phenomena in three dimensions can be strikingly different from phenomena in other dimensions, and so there is a prevalence of very specialized techniques that do not generalize to dimensions g ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Alexandre-Théophile Vandermonde
Alexandre-Théophile Vandermonde (28 February 1735 – 1 January 1796) was a French mathematician, musician and chemist who worked with Bézout and Lavoisier; his name is now principally associated with determinant theory in mathematics. He was born in Paris, and died there. Biography Vandermonde was a violinist, and became engaged with mathematics only around 1770. In ''Mémoire sur la résolution des équations'' (1771) he reported on symmetric functions and solution of cyclotomic polynomials; this paper anticipated later Galois theory (see also abstract algebra for the role of Vandermonde in the genesis of group theory). In ''Remarques sur des problèmes de situation'' (1771) he studied knight's tours, and presaged the development of knot theory by explicitly noting the importance of topological features when discussing the properties of knots: ''"Whatever the twists and turns of a system of threads in space, one can always obtain an expression for the calculation of its dimen ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Peter Tait
{{hndis, Tait, Peter ...
Peter Tait may refer to: * Peter Tait (physicist) (1831–1901), Scottish mathematical physicist * Peter Tait (footballer) (1936–1990), English professional footballer * Peter Tait (mayor) (1915–1996), New Zealand politician * Peter Tait (radio presenter) (1950–2002), English radio presenter * Peter Tait (sport shooter) (born 1949), Australian Paralympian * Peter Tait (rugby union) (1906–1980), Scottish rugby union player See also *Tait (surname) Tait is a Scottish surname which means "pleasure" or "delight." The origins of the name can be traced back as far as 1100. Notable people with the surname include: * Alan Tait (born 1964), Scottish rugby player and coach * Alasdair Tait, Britis ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Celtic Knot
Celtic knots ( ga, snaidhm Cheilteach, cy, cwlwm Celtaidd, kw, kolm Keltek, gd, snaidhm Ceilteach) are a variety of knots and stylized graphical representations of knots used for decoration, used extensively in the Celtic style of Insular art. These knots are most known for their adaptation for use in the ornamentation of Christian monuments and manuscripts, such as the 8th-century St. Teilo Gospels, the Book of Kells and the Lindisfarne Gospels. Most are endless knots, and many are varieties of basket weave knots. History The use of interlace patterns had its origins in the late Roman Empire. Knot patterns first appeared in the third and fourth centuries AD and can be seen in Roman floor mosaics of that time. Interesting developments in the artistic use of interlaced knot patterns are found in Byzantine architecture and book illumination, Coptic art, Celtic art, Islamic art, Kievan Rus'ian book illumination, Ethiopian art, and European architecture and book illum ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Book Of Kells
The Book of Kells ( la, Codex Cenannensis; ga, Leabhar Cheanannais; Dublin, Trinity College Library, MS A. I. 8 sometimes known as the Book of Columba) is an illuminated manuscript Gospel book in Latin, containing the four Gospels of the New Testament together with various prefatory texts and tables. It was created in a Columban monastery in either Ireland, Scotland or England, and may have had contributions from various Columban institutions from each of these areas. It is believed to have been created 800 AD. The text of the Gospels is largely drawn from the Vulgate, although it also includes several passages drawn from the earlier versions of the Bible known as the Vetus Latina. It is regarded as a masterwork of Western calligraphy and the pinnacle of Insular illumination. The manuscript takes its name from the Abbey of Kells, County Meath, which was its home for centuries. The illustrations and ornamentation of the Book of Kells surpass those of other Insular Gospel ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Celtic Christianity
Celtic Christianity ( kw, Kristoneth; cy, Cristnogaeth; gd, Crìosdaidheachd; gv, Credjue Creestee/Creestiaght; ga, Críostaíocht/Críostúlacht; br, Kristeniezh; gl, Cristianismo celta) is a form of Christianity that was common, or held to be common, across the Celtic-speaking world during the Early Middle Ages. Some writers have described a distinct Celtic Church uniting the Celtic peoples and distinguishing them from adherents of the Roman Church, while others classify Celtic Christianity as a set of distinctive practices occurring in those areas. Varying scholars reject the former notion, but note that there were certain traditions and practices present in both the Irish and British churches that were not seen in the wider Christian world. Such practices include: a distinctive system for determining the dating of Easter, a style of monastic tonsure, a unique system of penance, and the popularity of going into "exile for Christ". Additionally, there were other pract ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Borromean Rings
In mathematics, the Borromean rings are three simple closed curves in three-dimensional space that are topologically linked and cannot be separated from each other, but that break apart into two unknotted and unlinked loops when any one of the three is cut or removed. Most commonly, these rings are drawn as three circles in the plane, in the pattern of a Venn diagram, alternatingly crossing over and under each other at the points where they cross. Other triples of curves are said to form the Borromean rings as long as they are topologically equivalent to the curves depicted in this drawing. The Borromean rings are named after the Italian House of Borromeo, who used the circular form of these rings as a coat of arms, but designs based on the Borromean rings have been used in many cultures, including by the Norsemen and in Japan. They have been used in Christian symbolism as a sign of the Trinity, and in modern commerce as the logo of Ballantine beer, giving them the alternative ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tibetan Buddhism
Tibetan Buddhism (also referred to as Indo-Tibetan Buddhism, Lamaism, Lamaistic Buddhism, Himalayan Buddhism, and Northern Buddhism) is the form of Buddhism practiced in Tibet and Bhutan, where it is the dominant religion. It is also in majority regions surrounding the Himalayan areas of India (such as Ladakh, Sikkim, Arunachal Pradesh, and a minority in Himachal Pradesh and Uttarakhand), in much of Central Asia, in the southern Siberian regions such as Tuva, and in Mongolia. Tibetan Buddhism evolved as a form of Mahāyāna Buddhism stemming from the latest stages of Indian Buddhism (which also included many Vajrayāna elements). It thus preserves many Indian Buddhist tantric practices of the post-Gupta early medieval period (500 to 1200 CE), along with numerous native Tibetan developments. In the pre-modern era, Tibetan Buddhism spread outside of Tibet primarily due to the influence of the Mongol Yuan dynasty (1271–1368), founded by Kublai Khan, which had ruled China, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Endless Knot
Endless knot in a Burmese Pali manuscript The endless knot or eternal knot is a symbolic knot and one of the Eight Auspicious Symbols. It is an important symbol in Hinduism, Jainism and Buddhism. It is an important cultural marker in places significantly influenced by Tibetan Buddhism such as Tibet, Mongolia, Tuva, Kalmykia, and Buryatia. It is also found in Celtic and Chinese symbolism. History The endless knot appears on clay tablets from the Indus Valley civilization (2500 BC) and on a historic era inscription. Interpretations Buddhism Various Buddhist interpretations of the symbol are: * The endless knot iconography symbolised Samsara ''i.e.'', the endless cycle of suffering of birth, death and rebirth within Tibetan Buddhism. * The inter-twining of wisdom and compassion. * Interplay and interaction of the opposing forces in the dualistic world of manifestation, leading to their union, and ultimately to harmony in the universe. * The mutual dependence of relig ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Chinese Knotting
Chinese knotting, also known as () and decorative knots in non-Chinese cultures, is a decorative handcraft art that began as a form of Chinese folk art in the Tang dynasty, Tang and Song dynasty (960–1279 CE) in China. This form of craft originated and was derived from the () culture which already existed in China since the ancient times. As a form of art, it is also called Chinese traditional decorative knots. Chinese knotting was later popularized in the Ming dynasty, Ming and spread to Japan and Korea. There are many different shapes of Chinese knots, the most common being Butterfly, butterflies, Flower, flowers, Bird, birds, Chinese dragon, dragons, fish, and even shoes. Culturally they were expected to ward off evil spirits similar to bagua mirrors or act as good-luck charms for Chinese marriages. Around the times of the Chinese New Year, Chinese new year festival, Chinese knot decorations can be seen hanging on walls, doors of homes and as shop decorations to add some ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
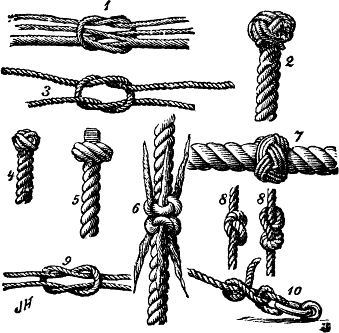
Knot Tying
A knot is an intentional complication in cordage which may be practical or decorative, or both. Practical knots are classified by function, including hitches, bends, loop knots, and splices: a ''hitch'' fastens a rope to another object; a ''bend'' fastens two ends of a rope to each another; a ''loop knot'' is any knot creating a loop; and ''splice'' denotes any multi-strand knot, including bends and loops. A knot may also refer, in the strictest sense, to a stopper or knob at the end of a rope to keep that end from slipping through a grommet or eye. Knots have excited interest since ancient times for their practical uses, as well as their topological intricacy, studied in the area of mathematics known as knot theory. History Knots and knotting have been used and studied throughout history. For example, Chinese knotting is a decorative handicraft art that began as a form of Chinese folk art in the Tang and Song Dynasty (960–1279 AD) in China, later popularized in t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
.jpeg/1200px-Tibetan_Buddhism_(214837929).jpeg "Click for more on -> Tibetan Buddhism")
