|
11-cell
In mathematics, the 11-cell (or hendecachoron) is a self-dual abstract regular 4-polytope ( four-dimensional polytope). Its 11 cells are hemi-icosahedral. It has 11 vertices, 55 edges and 55 faces. It has Schläfli symbol , with 3 hemi-icosahedra (Schläfli type ) around each edge. It has symmetry order 660, computed as the product of the number of cells (11) and the symmetry of each cell (60). The symmetry structure is the abstract group projective special linear group L2(11). It was discovered in 1977 by Branko Grünbaum, who constructed it by pasting hemi-icosahedra together, three at each edge, until the shape closed up. It was independently discovered by H. S. M. Coxeter in 1984, who studied its structure and symmetry in greater depth. Related polytopes Orthographic projection of 10-simplex with 11 vertices, 55 edges. The abstract ''11-cell'' contains the same number of vertices and edges as the 10-dimensional 10-simplex, and contains 1/3 of its 165 faces. Thus it ca ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Abstract Polytope
In mathematics, an abstract polytope is an algebraic partially ordered set which captures the dyadic property of a traditional polytope without specifying purely geometric properties such as points and lines. A geometric polytope is said to be a ''realization'' of an abstract polytope in some real N-dimensional space, typically Euclidean. This abstract definition allows more general combinatorial structures than traditional definitions of a polytope, thus allowing new objects that have no counterpart in traditional theory. Introductory concepts Traditional versus abstract polytopes In Euclidean geometry, two shapes that are not similar can nonetheless share a common structure. For example a square and a trapezoid both comprise an alternating chain of four vertices and four sides, which makes them quadrilaterals. They are said to be isomorphic or “structure preserving”. This common structure may be represented in an underlying abstract polytope, a purely algebra ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Abstract Polytope
In mathematics, an abstract polytope is an algebraic partially ordered set which captures the dyadic property of a traditional polytope without specifying purely geometric properties such as points and lines. A geometric polytope is said to be a ''realization'' of an abstract polytope in some real N-dimensional space, typically Euclidean. This abstract definition allows more general combinatorial structures than traditional definitions of a polytope, thus allowing new objects that have no counterpart in traditional theory. Introductory concepts Traditional versus abstract polytopes In Euclidean geometry, two shapes that are not similar can nonetheless share a common structure. For example a square and a trapezoid both comprise an alternating chain of four vertices and four sides, which makes them quadrilaterals. They are said to be isomorphic or “structure preserving”. This common structure may be represented in an underlying abstract polytope, a purely algebra ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
57-cell
In mathematics, the 57-cell (pentacontakaiheptachoron) is a self-dual abstract regular 4-polytope ( four-dimensional polytope). Its 57 cells are hemi-dodecahedra. It also has 57 vertices, 171 edges and 171 two-dimensional faces. The symmetry order is 3420, from the product of the number of cells (57) and the symmetry of each cell (60). The symmetry abstract structure is the projective special linear group, L2(19). It has Schläfli symbol with 5 hemi-dodecahedral cells around each edge. It was discovered by . Perkel graph The vertices and edges form the Perkel graph, the unique distance-regular graph with intersection array , discovered by . See also * 11-cell – abstract regular polytope with hemi-icosahedral cells. * 120-cell – regular 4-polytope with dodecahedral cells * Order-5 dodecahedral honeycomb - regular hyperbolic honeycomb with same Schläfli symbol In geometry, the Schläfli symbol is a notation of the form \ that defines regular polytopes and tessell ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
4-polytope
In geometry, a 4-polytope (sometimes also called a polychoron, polycell, or polyhedroid) is a four-dimensional polytope. It is a connected and closed figure, composed of lower-dimensional polytopal elements: vertices, edges, faces (polygons), and cells (polyhedra). Each face is shared by exactly two cells. The 4-polytopes were discovered by the Swiss mathematician Ludwig Schläfli before 1853. The two-dimensional analogue of a 4-polytope is a polygon, and the three-dimensional analogue is a polyhedron. Topologically 4-polytopes are closely related to the uniform honeycombs, such as the cubic honeycomb, which tessellate 3-space; similarly the 3D cube is related to the infinite 2D square tiling. Convex 4-polytopes can be ''cut and unfolded'' as nets in 3-space. Definition A 4-polytope is a closed four-dimensional figure. It comprises vertices (corner points), edges, faces and cells. A cell is the three-dimensional analogue of a face, and is therefore a polyhedron. Each fa ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Icosahedral Honeycomb
In geometry, the icosahedral honeycomb is one of four compact, regular, space-filling tessellations (or honeycombs) in hyperbolic 3-space. With Schläfli symbol there are three icosahedra around each edge, and 12 icosahedra around each vertex, in a regular dodecahedral vertex figure. Description The dihedral angle of a regular icosahedron is around 138.2°, so it is impossible to fit three icosahedra around an edge in Euclidean 3-space. However, in hyperbolic space, properly scaled icosahedra can have dihedral angles of exactly 120 degrees, so three of those can fit around an edge. Related regular honeycombs There are four regular compact honeycombs in 3D hyperbolic space: Related regular polytopes and honeycombs It is a member of a sequence of regular polychora and honeycombs with deltrahedral cells: It is also a member of a sequence of regular polychora and honeycombs , with vertex figures composed of pentagons: Uniform honeycombs There are nine uniform h ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
5-simplex
In five-dimensional geometry, a 5-simplex is a self-dual regular 5-polytope. It has six vertices, 15 edges, 20 triangle faces, 15 tetrahedral cells, and 6 5-cell facets. It has a dihedral angle of cos−1(), or approximately 78.46°. The 5-simplex is a solution to the problem: ''Make 20 equilateral triangles using 15 matchsticks, where each side of every triangle is exactly one matchstick.'' Alternate names It can also be called a hexateron, or hexa-5-tope, as a 6- facetted polytope in 5-dimensions. The name ''hexateron'' is derived from ''hexa-'' for having six facets and '' teron'' (with ''ter-'' being a corruption of ''tetra-'') for having four-dimensional facets. By Jonathan Bowers, a hexateron is given the acronym hix. As a configuration This configuration matrix represents the 5-simplex. The rows and columns correspond to vertices, edges, faces, cells and 4-faces. The diagonal numbers say how many of each element occur in the whole 5-simplex. The nondiagonal numb ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
10-simplex
In geometry, a 10-simplex is a self-dual regular 10-polytope. It has 11 vertices, 55 edges, 165 triangle faces, 330 tetrahedral cells, 462 5-cell 4-faces, 462 5-simplex 5-faces, 330 6-simplex 6-faces, 165 7-simplex 7-faces, 55 8-simplex 8-faces, and 11 9-simplex 9-faces. Its dihedral angle is cos−1(1/10), or approximately 84.26°. It can also be called a hendecaronnon, or hendeca-10-tope, as an 11- facetted polytope in 10-dimensions. The name ''hendecaronnon'' is derived from ''hendeca'' for 11 facets in Greek and -ronn (variation of ennea for nine), having 9-dimensional facets, and ''-on''. Coordinates The Cartesian coordinates of the vertices of an origin-centered regular 10-simplex having edge length 2 are: :\left(\sqrt,\ \sqrt,\ 1/6,\ \sqrt,\ \sqrt,\ \sqrt,\ \sqrt,\ \sqrt,\ \sqrt,\ \pm1\right) :\left(\sqrt,\ \sqrt,\ 1/6,\ \sqrt,\ \sqrt,\ \sqrt,\ \sqrt,\ \sqrt,\ -2\sqrt,\ 0\right) :\left(\sqrt,\ \sqrt,\ 1/6,\ \sqrt,\ \sqrt,\ \sqrt,\ \sqrt,\ -\sqrt,\ 0,\ 0\right) :\ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
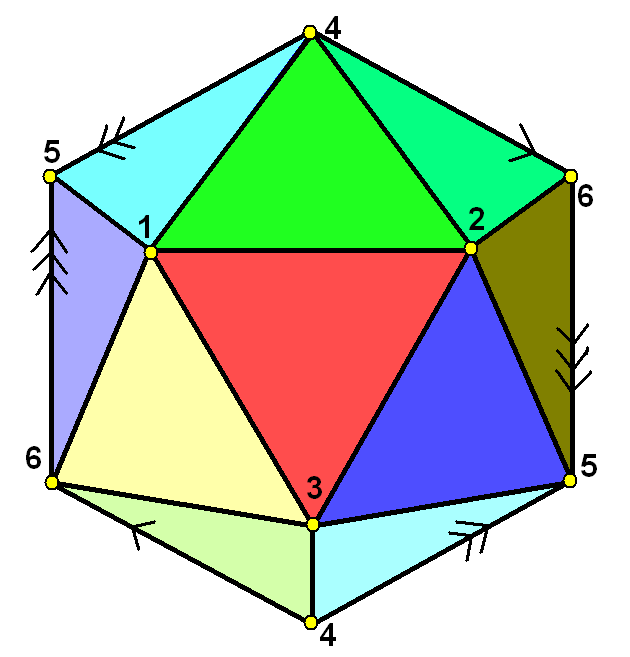
Hemi-icosahedron Coloured
A hemi-icosahedron is an abstract regular polyhedron, containing half the faces of a regular icosahedron. It can be realized as a projective polyhedron (a tessellation of the real projective plane by 10 triangles), which can be visualized by constructing the projective plane as a hemisphere where opposite points along the boundary are connected and dividing the hemisphere into three equal parts. Geometry It has 10 triangular faces, 15 edges, and 6 vertices. It is also related to the nonconvex uniform polyhedron, the tetrahemihexahedron, which could be topologically identical to the hemi-icosahedron if each of the 3 square faces were divided into two triangles. Graphs It can be represented symmetrically on faces, and vertices as Schlegel diagrams: The complete graph K6 It has the same vertices and edges as the 5-dimensional 5-simplex which has a complete graph of edges, but only contains half of the (20) faces. From the point of view of graph theory this is an embedding of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hemi-icosahedron
A hemi-icosahedron is an abstract regular polyhedron, containing half the faces of a regular icosahedron. It can be realized as a projective polyhedron (a tessellation of the real projective plane by 10 triangles), which can be visualized by constructing the projective plane as a hemisphere where opposite points along the boundary are connected and dividing the hemisphere into three equal parts. Geometry It has 10 triangular faces, 15 edges, and 6 vertices. It is also related to the nonconvex uniform polyhedron, the tetrahemihexahedron, which could be topologically identical to the hemi-icosahedron if each of the 3 square faces were divided into two triangles. Graphs It can be represented symmetrically on faces, and vertices as Schlegel diagrams: The complete graph K6 It has the same vertices and edges as the 5-dimensional 5-simplex which has a complete graph of edges, but only contains half of the (20) faces. From the point of view of graph theory this is an embedding o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hemi-icosahedron
A hemi-icosahedron is an abstract regular polyhedron, containing half the faces of a regular icosahedron. It can be realized as a projective polyhedron (a tessellation of the real projective plane by 10 triangles), which can be visualized by constructing the projective plane as a hemisphere where opposite points along the boundary are connected and dividing the hemisphere into three equal parts. Geometry It has 10 triangular faces, 15 edges, and 6 vertices. It is also related to the nonconvex uniform polyhedron, the tetrahemihexahedron, which could be topologically identical to the hemi-icosahedron if each of the 3 square faces were divided into two triangles. Graphs It can be represented symmetrically on faces, and vertices as Schlegel diagrams: The complete graph K6 It has the same vertices and edges as the 5-dimensional 5-simplex which has a complete graph of edges, but only contains half of the (20) faces. From the point of view of graph theory this is an embedding o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Projective Special Linear Group
In mathematics, especially in the group theoretic area of algebra, the projective linear group (also known as the projective general linear group or PGL) is the induced action of the general linear group of a vector space ''V'' on the associated projective space P(''V''). Explicitly, the projective linear group is the quotient group :PGL(''V'') = GL(''V'')/Z(''V'') where GL(''V'') is the general linear group of ''V'' and Z(''V'') is the subgroup of all nonzero scalar transformations of ''V''; these are quotiented out because they act trivially on the projective space and they form the kernel of the action, and the notation "Z" reflects that the scalar transformations form the center of the general linear group. The projective special linear group, PSL, is defined analogously, as the induced action of the special linear group on the associated projective space. Explicitly: :PSL(''V'') = SL(''V'')/SZ(''V'') where SL(''V'') is the special linear group over ''V'' and SZ(''V'') is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Self-dual Polytope
In geometry, every polyhedron is associated with a second dual structure, where the Vertex (geometry), vertices of one correspond to the Face (geometry), faces of the other, and the edges between pairs of vertices of one correspond to the edges between pairs of faces of the other. Such dual figures remain combinatorial or Abstract polytope, abstract polyhedra, but not all can also be constructed as geometric polyhedra. Starting with any given polyhedron, the dual of its dual is the original polyhedron. Duality preserves the Symmetry, symmetries of a polyhedron. Therefore, for many classes of polyhedra defined by their symmetries, the duals belong to a corresponding symmetry class. For example, the regular polyhedrathe (convex) Platonic solids and (star) Kepler–Poinsot polyhedraform dual pairs, where the regular tetrahedron is #Self-dual polyhedra, self-dual. The dual of an Isogonal figure, isogonal polyhedron (one in which any two vertices are equivalent under symmetries of the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |