Icosahedral honeycomb on:
[Wikipedia]
[Google]
[Amazon]
In

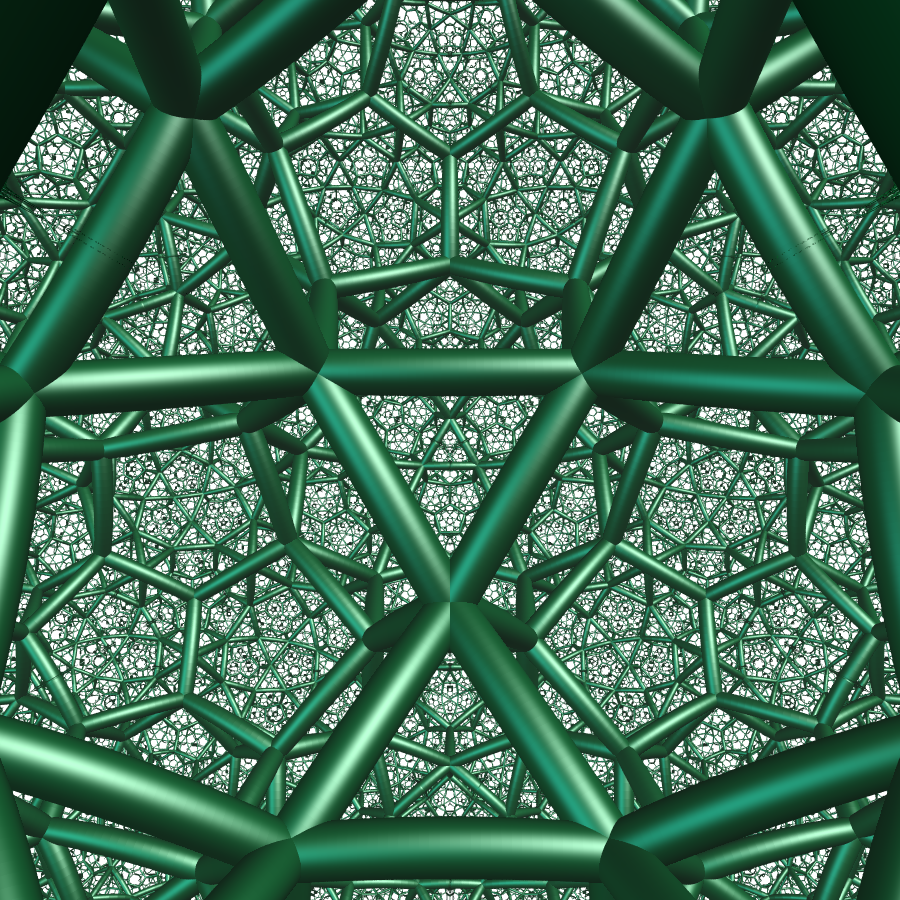
Perspective projections from center of Poincaré disk model




 : Viewed from center of triangular prism
: Viewed from center of triangular prism
 : Viewed from center of triangular prism
: Viewed from center of triangular prism
 : Centered on hexagonal prism
: Centered on hexagonal prism
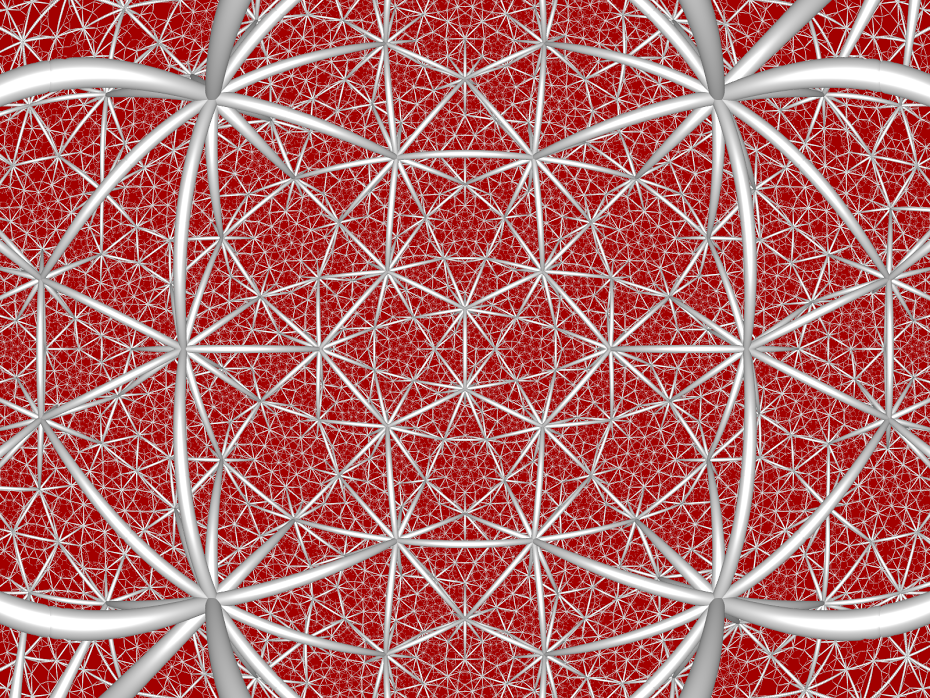

geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is c ...
, the icosahedral honeycomb is one of four compact, regular, space-filling tessellation
A tessellation or tiling is the covering of a surface, often a plane (mathematics), plane, using one or more geometric shapes, called ''tiles'', with no overlaps and no gaps. In mathematics, tessellation can be generalized to high-dimensional ...
s (or honeycombs) in hyperbolic 3-space
In mathematics, hyperbolic space of dimension n is the unique simply connected, n-dimensional Riemannian manifold of constant sectional curvature equal to -1. It is homogeneous, and satisfies the stronger property of being a symmetric space. ...
. With Schläfli symbol there are three icosahedra
In geometry, an icosahedron ( or ) is a polyhedron with 20 faces. The name comes and . The plural can be either "icosahedra" () or "icosahedrons".
There are infinitely many non- similar shapes of icosahedra, some of them being more symmetrica ...
around each edge
Edge or EDGE may refer to:
Technology Computing
* Edge computing, a network load-balancing system
* Edge device, an entry point to a computer network
* Adobe Edge, a graphical development application
* Microsoft Edge, a web browser developed ...
, and 12 icosahedra around each vertex
Vertex, vertices or vertexes may refer to:
Science and technology Mathematics and computer science
*Vertex (geometry), a point where two or more curves, lines, or edges meet
* Vertex (computer graphics), a data structure that describes the positio ...
, in a regular dodecahedral
In geometry, a dodecahedron (Greek , from ''dōdeka'' "twelve" + ''hédra'' "base", "seat" or "face") or duodecahedron is any polyhedron with twelve flat faces. The most familiar dodecahedron is the regular dodecahedron with regular pentagon ...
vertex figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off.
Definitions
Take some corner or vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw line ...
.
Description
Thedihedral angle
A dihedral angle is the angle between two intersecting planes or half-planes. In chemistry, it is the clockwise angle between half-planes through two sets of three atoms, having two atoms in common. In solid geometry, it is defined as the un ...
of a regular icosahedron
In geometry, a regular icosahedron ( or ) is a convex polyhedron with 20 faces, 30 edges and 12 vertices. It is one of the five Platonic solids, and the one with the most faces.
It has five equilateral triangular faces meeting at each vertex. It ...
is around 138.2°, so it is impossible to fit three icosahedra around an edge in Euclidean 3-space. However, in hyperbolic space, properly scaled icosahedra can have dihedral angles of exactly 120 degrees, so three of those can fit around an edge.
Related regular honeycombs
There are four regular compact honeycombs in 3D hyperbolic space:Related regular polytopes and honeycombs
It is a member of a sequence ofregular polychora
In mathematics, a regular 4-polytope is a regular four-dimensional polytope. They are the four-dimensional analogues of the regular polyhedra in three dimensions and the regular polygons in two dimensions.
There are six convex and ten star regu ...
and honeycombs with deltrahedral cells:
It is also a member of a sequence of regular polychora
In mathematics, a regular 4-polytope is a regular four-dimensional polytope. They are the four-dimensional analogues of the regular polyhedra in three dimensions and the regular polygons in two dimensions.
There are six convex and ten star regu ...
and honeycombs , with vertex figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off.
Definitions
Take some corner or vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw line ...
s composed of pentagons:
Uniform honeycombs
There are nine uniform honeycombs in the ,5,3Coxeter group
In mathematics, a Coxeter group, named after H. S. M. Coxeter, is an abstract group that admits a formal description in terms of reflections (or kaleidoscopic mirrors). Indeed, the finite Coxeter groups are precisely the finite Euclidean refl ...
family, including this regular form as well as the bitruncated form, t1,2, , also called ''truncated dodecahedral honeycomb'', each of whose cells are truncated dodecahedra.
Rectified icosahedral honeycomb
The rectified icosahedral honeycomb, t1, , has alternatingdodecahedron
In geometry, a dodecahedron (Greek , from ''dōdeka'' "twelve" + ''hédra'' "base", "seat" or "face") or duodecahedron is any polyhedron with twelve flat faces. The most familiar dodecahedron is the regular dodecahedron with regular pentagon ...
and icosidodecahedron
In geometry, an icosidodecahedron is a polyhedron with twenty (''icosi'') triangular faces and twelve (''dodeca'') pentagonal faces. An icosidodecahedron has 30 identical vertices, with two triangles and two pentagons meeting at each, and 60 i ...
cells, with a triangular prism
In geometry, a triangular prism is a three-sided prism; it is a polyhedron made of a triangular base, a translated copy, and 3 faces joining corresponding sides. A right triangular prism has rectangular sides, otherwise it is ''oblique''. A ...
vertex figure:
: 

Perspective projections from center of Poincaré disk model
Related honeycomb
There are four rectified compact regular honeycombs:Truncated icosahedral honeycomb
The truncated icosahedral honeycomb, t0,1, , has alternatingdodecahedron
In geometry, a dodecahedron (Greek , from ''dōdeka'' "twelve" + ''hédra'' "base", "seat" or "face") or duodecahedron is any polyhedron with twelve flat faces. The most familiar dodecahedron is the regular dodecahedron with regular pentagon ...
and truncated icosahedron
In geometry, the truncated icosahedron is an Archimedean solid, one of 13 convex isogonal nonprismatic solids whose 32 faces are two or more types of regular polygons. It is the only one of these shapes that does not contain triangles or squares. ...
cells, with a triangular pyramid
In geometry, a tetrahedron (plural: tetrahedra or tetrahedrons), also known as a triangular pyramid, is a polyhedron composed of four triangular faces, six straight edges, and four vertex corners. The tetrahedron is the simplest of all the o ...
vertex figure.

Related honeycombs
Bitruncated icosahedral honeycomb
The bitruncated icosahedral honeycomb, t1,2, , hastruncated dodecahedron
In geometry, the truncated dodecahedron is an Archimedean solid. It has 12 regular decagonal faces, 20 regular triangular faces, 60 vertices and 90 edges.
Geometric relations
This polyhedron can be formed from a regular dodecahedron by tr ...
cells with a tetragonal disphenoid
In geometry, a disphenoid () is a tetrahedron whose four faces are congruent acute-angled triangles. It can also be described as a tetrahedron in which every two edges that are opposite each other have equal lengths. Other names for the same sh ...
vertex figure.

Related honeycombs
Cantellated icosahedral honeycomb
The cantellated icosahedral honeycomb, t0,2, , hasrhombicosidodecahedron
In geometry, the rhombicosidodecahedron is an Archimedean solid, one of thirteen convex isogonal nonprismatic solids constructed of two or more types of regular polygon faces.
It has 20 regular triangular faces, 30 square (geometry), square face ...
, icosidodecahedron
In geometry, an icosidodecahedron is a polyhedron with twenty (''icosi'') triangular faces and twelve (''dodeca'') pentagonal faces. An icosidodecahedron has 30 identical vertices, with two triangles and two pentagons meeting at each, and 60 i ...
, and triangular prism
In geometry, a triangular prism is a three-sided prism; it is a polyhedron made of a triangular base, a translated copy, and 3 faces joining corresponding sides. A right triangular prism has rectangular sides, otherwise it is ''oblique''. A ...
cells, with a wedge
A wedge is a triangular shaped tool, and is a portable inclined plane, and one of the six simple machines. It can be used to separate two objects or portions of an object, lift up an object, or hold an object in place. It functions by converti ...
vertex figure.

Related honeycombs
Cantitruncated icosahedral honeycomb
The cantitruncated icosahedral honeycomb, t0,1,2, , hastruncated icosidodecahedron
In geometry, a truncated icosidodecahedron, rhombitruncated icosidodecahedron,Wenninger Model Number 16 great rhombicosidodecahedron,Williams (Section 3-9, p. 94)Cromwell (p. 82) omnitruncated dodecahedron or omnitruncated icosahedronNorman Wooda ...
, truncated dodecahedron
In geometry, the truncated dodecahedron is an Archimedean solid. It has 12 regular decagonal faces, 20 regular triangular faces, 60 vertices and 90 edges.
Geometric relations
This polyhedron can be formed from a regular dodecahedron by tr ...
, and triangular prism
In geometry, a triangular prism is a three-sided prism; it is a polyhedron made of a triangular base, a translated copy, and 3 faces joining corresponding sides. A right triangular prism has rectangular sides, otherwise it is ''oblique''. A ...
cells, with a mirrored sphenoid
In geometry, a tetrahedron (plural: tetrahedra or tetrahedrons), also known as a triangular pyramid, is a polyhedron composed of four triangular faces, six straight edges, and four vertex corners. The tetrahedron is the simplest of all the o ...
vertex figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off.
Definitions
Take some corner or vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw line ...
.

Related honeycombs
Runcinated icosahedral honeycomb
The runcinated icosahedral honeycomb, t0,3, , hasicosahedron
In geometry, an icosahedron ( or ) is a polyhedron with 20 faces. The name comes and . The plural can be either "icosahedra" () or "icosahedrons".
There are infinitely many non- similar shapes of icosahedra, some of them being more symmetrica ...
and triangular prism
In geometry, a triangular prism is a three-sided prism; it is a polyhedron made of a triangular base, a translated copy, and 3 faces joining corresponding sides. A right triangular prism has rectangular sides, otherwise it is ''oblique''. A ...
cells, with a pentagonal antiprism
In geometry, the pentagonal antiprism is the third in an infinite set of antiprisms formed by an even-numbered sequence of triangle sides closed by two polygon caps. It consists of two pentagons joined to each other by a ring of 10 triangles for ...
vertex figure.
 : Viewed from center of triangular prism
: Viewed from center of triangular prism
Related honeycombs
Runcitruncated icosahedral honeycomb
The runcitruncated icosahedral honeycomb, t0,1,3, , hastruncated icosahedron
In geometry, the truncated icosahedron is an Archimedean solid, one of 13 convex isogonal nonprismatic solids whose 32 faces are two or more types of regular polygons. It is the only one of these shapes that does not contain triangles or squares. ...
, rhombicosidodecahedron
In geometry, the rhombicosidodecahedron is an Archimedean solid, one of thirteen convex isogonal nonprismatic solids constructed of two or more types of regular polygon faces.
It has 20 regular triangular faces, 30 square (geometry), square face ...
, hexagonal prism
In geometry, the hexagonal prism is a prism with hexagonal base. Prisms are polyhedrons; this polyhedron has 8 faces, 18 edges, and 12 vertices..
Since it has 8 faces, it is an octahedron. However, the term ''octahedron'' is primarily used ...
, and triangular prism
In geometry, a triangular prism is a three-sided prism; it is a polyhedron made of a triangular base, a translated copy, and 3 faces joining corresponding sides. A right triangular prism has rectangular sides, otherwise it is ''oblique''. A ...
cells, with an isosceles-trapezoidal pyramid
A pyramid (from el, πυραμίς ') is a structure whose outer surfaces are triangular and converge to a single step at the top, making the shape roughly a pyramid in the geometric sense. The base of a pyramid can be trilateral, quadrilat ...
vertex figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off.
Definitions
Take some corner or vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw line ...
.
The runcicantellated icosahedral honeycomb is equivalent to the runcitruncated icosahedral honeycomb.
 : Viewed from center of triangular prism
: Viewed from center of triangular prism
Related honeycombs
Omnitruncated icosahedral honeycomb
The omnitruncated icosahedral honeycomb, t0,1,2,3, , hastruncated icosidodecahedron
In geometry, a truncated icosidodecahedron, rhombitruncated icosidodecahedron,Wenninger Model Number 16 great rhombicosidodecahedron,Williams (Section 3-9, p. 94)Cromwell (p. 82) omnitruncated dodecahedron or omnitruncated icosahedronNorman Wooda ...
and hexagonal prism
In geometry, the hexagonal prism is a prism with hexagonal base. Prisms are polyhedrons; this polyhedron has 8 faces, 18 edges, and 12 vertices..
Since it has 8 faces, it is an octahedron. However, the term ''octahedron'' is primarily used ...
cells, with a phyllic disphenoid vertex figure.
 : Centered on hexagonal prism
: Centered on hexagonal prism
Related honeycombs
Omnisnub icosahedral honeycomb
The omnisnub icosahedral honeycomb, h(t0,1,2,3), , hassnub dodecahedron
In geometry, the snub dodecahedron, or snub icosidodecahedron, is an Archimedean solid, one of thirteen convex isogonal nonprismatic solids constructed by two or more types of regular polygon faces.
The snub dodecahedron has 92 faces (the most ...
, octahedron
In geometry, an octahedron (plural: octahedra, octahedrons) is a polyhedron with eight faces. The term is most commonly used to refer to the regular octahedron, a Platonic solid composed of eight equilateral triangles, four of which meet at ea ...
, and tetrahedron
In geometry, a tetrahedron (plural: tetrahedra or tetrahedrons), also known as a triangular pyramid, is a polyhedron composed of four triangular faces, six straight edges, and four vertex corners. The tetrahedron is the simplest of all th ...
cells, with an irregular vertex figure. It is vertex-transitive
In geometry, a polytope (e.g. a polygon or polyhedron) or a tiling is isogonal or vertex-transitive if all its vertices are equivalent under the symmetries of the figure. This implies that each vertex is surrounded by the same kinds of face in ...
, but cannot be made with uniform cells.
Partially diminished icosahedral honeycomb
The partially diminished icosahedral honeycomb or parabidiminished icosahedral honeycomb, pd, is a non-Wythoffian uniform honeycomb withdodecahedron
In geometry, a dodecahedron (Greek , from ''dōdeka'' "twelve" + ''hédra'' "base", "seat" or "face") or duodecahedron is any polyhedron with twelve flat faces. The most familiar dodecahedron is the regular dodecahedron with regular pentagon ...
and pentagonal antiprism
In geometry, the pentagonal antiprism is the third in an infinite set of antiprisms formed by an even-numbered sequence of triangle sides closed by two polygon caps. It consists of two pentagons joined to each other by a ring of 10 triangles for ...
cells, with a tetrahedrally diminished dodecahedron
In geometry, a tetrahedrally diminished dodecahedron (also tetrahedrally stellated icosahedron or propello tetrahedron) is a topologically self-dual polyhedron made of 16 vertices, 30 edges, and 16 faces (4 equilateral triangles and 12 identical ...
vertex figure. The icosahedral cells of the are diminished at opposite vertices (parabidiminished), leaving a pentagonal antiprism
In geometry, the pentagonal antiprism is the third in an infinite set of antiprisms formed by an even-numbered sequence of triangle sides closed by two polygon caps. It consists of two pentagons joined to each other by a ring of 10 triangles for ...
( parabidiminished icosahedron) core, and creating new dodecahedron cells above and below.


See also
*Convex uniform honeycombs in hyperbolic space
In hyperbolic geometry, a uniform honeycomb in hyperbolic space is a uniform tessellation of uniform polyhedral cells. In 3-dimensional hyperbolic space there are nine Coxeter group families of compact convex uniform honeycombs, generated as Wyt ...
* Regular tessellations of hyperbolic 3-space
* Seifert–Weber space In mathematics, Seifert–Weber space (introduced by Herbert Seifert and Constantin Weber) is a closed hyperbolic 3-manifold. It is also known as Seifert–Weber dodecahedral space and hyperbolic dodecahedral space. It is one of the first discover ...
* 11-cell
In mathematics, the 11-cell (or hendecachoron) is a self-dual abstract regular 4-polytope ( four-dimensional polytope). Its 11 cells are hemi-icosahedral. It has 11 vertices, 55 edges and 55 faces. It has Schläfli symbol , with 3 hemi-icosahedr ...
- An abstract regular polychoron which shares the Schläfli symbol.
References
*Coxeter
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
, ''Regular Polytopes
In mathematics, a regular polytope is a polytope whose symmetry group acts transitively on its flags, thus giving it the highest degree of symmetry. All its elements or -faces (for all , where is the dimension of the polytope) — cells, ...
'', 3rd. ed., Dover Publications, 1973. . (Tables I and II: Regular polytopes and honeycombs, pp. 294–296)
*Coxeter
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
, ''The Beauty of Geometry: Twelve Essays'', Dover Publications, 1999 (Chapter 10: Regular honeycombs in hyperbolic space, Summary tables II,III,IV,V, p212-213)
* Norman Johnson ''Uniform Polytopes'', Manuscript
** N.W. Johnson: ''The Theory of Uniform Polytopes and Honeycombs'', Ph.D. Dissertation, University of Toronto, 1966
** N.W. Johnson: ''Geometries and Transformations'', (2018) Chapter 13: Hyperbolic Coxeter groups
* {{KlitzingPolytopes, ../incmats/x3o5o3o.htm, Hyperbolic H3 honeycombs, hyperbolic order 3 icosahedral tesselation
Honeycombs (geometry)
Self-dual tilings