subwavelength grating on:
[Wikipedia]
[Google]
[Amazon]
 In
In

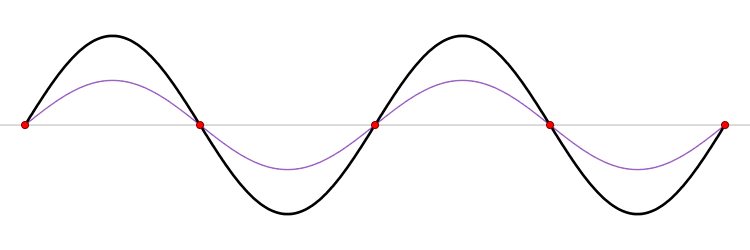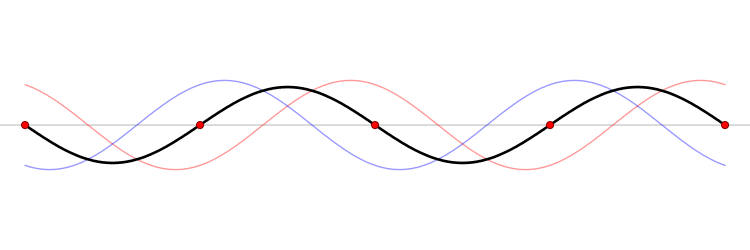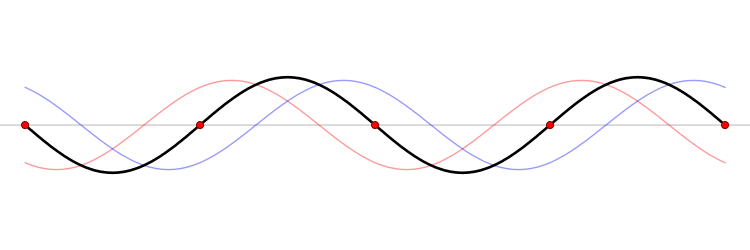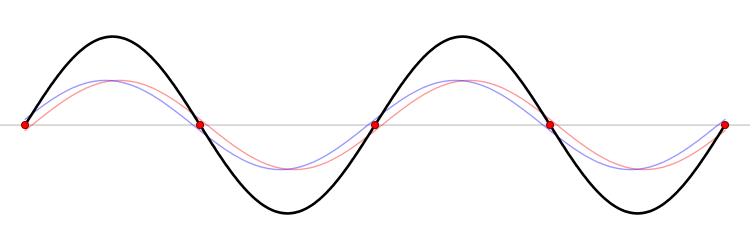


 The speed of a wave depends upon the medium in which it propagates. In particular, the speed of light in a medium is less than in
The speed of a wave depends upon the medium in which it propagates. In particular, the speed of light in a medium is less than in
 Wavelength can be a useful concept even if the wave is not periodic in space. For example, in an ocean wave approaching shore, shown in the figure, the incoming wave undulates with a varying ''local'' wavelength that depends in part on the depth of the sea floor compared to the wave height. The analysis of the wave can be based upon comparison of the local wavelength with the local water depth.
Wavelength can be a useful concept even if the wave is not periodic in space. For example, in an ocean wave approaching shore, shown in the figure, the incoming wave undulates with a varying ''local'' wavelength that depends in part on the depth of the sea floor compared to the wave height. The analysis of the wave can be based upon comparison of the local wavelength with the local water depth.
 Waves that are sinusoidal in time but propagate through a medium whose properties vary with position (an ''inhomogeneous'' medium) may propagate at a velocity that varies with position, and as a result may not be sinusoidal in space. The figure at right shows an example. As the wave slows down, the wavelength gets shorter and the amplitude increases; after a place of maximum response, the short wavelength is associated with a high loss and the wave dies out.
The analysis of
Waves that are sinusoidal in time but propagate through a medium whose properties vary with position (an ''inhomogeneous'' medium) may propagate at a velocity that varies with position, and as a result may not be sinusoidal in space. The figure at right shows an example. As the wave slows down, the wavelength gets shorter and the amplitude increases; after a place of maximum response, the short wavelength is associated with a high loss and the wave dies out.
The analysis of
 Waves in crystalline solids are not continuous, because they are composed of vibrations of discrete particles arranged in a regular lattice. This produces
Waves in crystalline solids are not continuous, because they are composed of vibrations of discrete particles arranged in a regular lattice. This produces
 The concept of wavelength is most often applied to sinusoidal, or nearly sinusoidal, waves, because in a linear system the sinusoid is the unique shape that propagates with no shape change – just a phase change and potentially an amplitude change.
See
The wavelength (or alternatively
The concept of wavelength is most often applied to sinusoidal, or nearly sinusoidal, waves, because in a linear system the sinusoid is the unique shape that propagates with no shape change – just a phase change and potentially an amplitude change.
See
The wavelength (or alternatively  If a traveling wave has a fixed shape that repeats in space or in time, it is a ''periodic wave''.
Such waves are sometimes regarded as having a wavelength even though they are not sinusoidal.
As shown in the figure, wavelength is measured between consecutive corresponding points on the waveform.
If a traveling wave has a fixed shape that repeats in space or in time, it is a ''periodic wave''.
Such waves are sometimes regarded as having a wavelength even though they are not sinusoidal.
As shown in the figure, wavelength is measured between consecutive corresponding points on the waveform.
 Localized
Localized
 When sinusoidal waveforms add, they may reinforce each other (constructive interference) or cancel each other (destructive interference) depending upon their relative phase. This phenomenon is used in the
When sinusoidal waveforms add, they may reinforce each other (constructive interference) or cancel each other (destructive interference) depending upon their relative phase. This phenomenon is used in the
 The notion of path difference and constructive or destructive interference used above for the double-slit experiment applies as well to the display of a single slit of light intercepted on a screen. The main result of this interference is to spread out the light from the narrow slit into a broader image on the screen. This distribution of wave energy is called
The notion of path difference and constructive or destructive interference used above for the double-slit experiment applies as well to the display of a single slit of light intercepted on a screen. The main result of this interference is to spread out the light from the narrow slit into a broader image on the screen. This distribution of wave energy is called
 A quantity related to the wavelength is the angular wavelength (also known as reduced wavelength), usually symbolized by ''ƛ'' (lambda-bar). It is equal to the "regular" wavelength "reduced" by a factor of 2π (''ƛ'' = ''λ''/2π). It is usually encountered in quantum mechanics, where it is used in combination with the
A quantity related to the wavelength is the angular wavelength (also known as reduced wavelength), usually symbolized by ''ƛ'' (lambda-bar). It is equal to the "regular" wavelength "reduced" by a factor of 2π (''ƛ'' = ''λ''/2π). It is usually encountered in quantum mechanics, where it is used in combination with the
Conversion: Wavelength to Frequency and vice versa – Sound waves and radio waves
* ttp://www.magnetkern.de/spektrum.html The visible electromagnetic spectrum displayed in web colors with according wavelengths {{Authority control Waves Length
physics
Physics is the natural science that studies matter, its fundamental constituents, its motion and behavior through space and time, and the related entities of energy and force. "Physical science is that department of knowledge which r ...
, the wavelength is the spatial period of a periodic wave—the distance over which the wave's shape repeats.
It is the distance between consecutive corresponding points of the same phase
Phase or phases may refer to:
Science
*State of matter, or phase, one of the distinct forms in which matter can exist
*Phase (matter), a region of space throughout which all physical properties are essentially uniform
* Phase space, a mathematic ...
on the wave, such as two adjacent crests, troughs, or zero crossing
A zero-crossing is a point where the sign of a mathematical function changes (e.g. from positive to negative), represented by an intercept of the axis (zero value) in the graph of the function. It is a commonly used term in electronics, mathemat ...
s, and is a characteristic of both traveling waves and standing wave
In physics, a standing wave, also known as a stationary wave, is a wave that oscillates in time but whose peak amplitude profile does not move in space. The peak amplitude of the wave oscillations at any point in space is constant with respect ...
s, as well as other spatial wave patterns.
The inverse of the wavelength is called the spatial frequency
In mathematics, physics, and engineering, spatial frequency is a characteristic of any structure that is periodic across position in space. The spatial frequency is a measure of how often sinusoidal components (as determined by the Fourier tra ...
. Wavelength is commonly designated by the Greek letter
The Greek alphabet has been used to write the Greek language since the late 9th or early 8th century BCE. It is derived from the earlier Phoenician alphabet, and was the earliest known alphabetic script to have distinct letters for vowels as w ...
''lambda
Lambda (}, ''lám(b)da'') is the 11th letter of the Greek alphabet, representing the voiced alveolar lateral approximant . In the system of Greek numerals, lambda has a value of 30. Lambda is derived from the Phoenician Lamed . Lambda gave rise ...
'' (λ).
The term ''wavelength'' is also sometimes applied to modulated
In electronics and telecommunications, modulation is the process of varying one or more properties of a periodic waveform, called the ''carrier signal'', with a separate signal called the ''modulation signal'' that typically contains informatio ...
waves, and to the sinusoidal envelopes
An envelope is a common packaging item, usually made of thin, flat material. It is designed to contain a flat object, such as a letter or card.
Traditional envelopes are made from sheets of paper cut to one of three shapes: a rhombus, a sh ...
of modulated waves or waves formed by interference
Interference is the act of interfering, invading, or poaching. Interference may also refer to:
Communications
* Interference (communication), anything which alters, modifies, or disrupts a message
* Adjacent-channel interference, caused by extr ...
of several sinusoids.
Assuming a sinusoidal wave moving at a fixed wave speed, wavelength is inversely proportional to frequency
Frequency is the number of occurrences of a repeating event per unit of time. It is also occasionally referred to as ''temporal frequency'' for clarity, and is distinct from ''angular frequency''. Frequency is measured in hertz (Hz) which is eq ...
of the wave: waves with higher frequencies have shorter wavelengths, and lower frequencies have longer wavelengths.
Wavelength depends on the medium (for example, vacuum, air, or water) that a wave travels through. Examples of waves are sound wave
In physics, sound is a vibration that propagates as an acoustic wave, through a transmission medium such as a gas, liquid or solid.
In human physiology and psychology, sound is the ''reception'' of such waves and their ''perception'' by the ...
s, light
Light or visible light is electromagnetic radiation that can be perceived by the human eye. Visible light is usually defined as having wavelengths in the range of 400–700 nanometres (nm), corresponding to frequencies of 750–420 tera ...
, water wave
In fluid dynamics, a wind wave, water wave, or wind-generated water wave, is a surface wave that occurs on the free surface of bodies of water as a result from the wind blowing over the water surface. The contact distance in the direction of t ...
s and periodic electrical signals in a conductor. A sound
In physics, sound is a vibration that propagates as an acoustic wave, through a transmission medium such as a gas, liquid or solid.
In human physiology and psychology, sound is the ''reception'' of such waves and their ''perception'' by the ...
wave is a variation in air pressure
Pressure (symbol: ''p'' or ''P'') is the force applied perpendicular to the surface of an object per unit area over which that force is distributed. Gauge pressure (also spelled ''gage'' pressure)The preferred spelling varies by country and e ...
, while in light
Light or visible light is electromagnetic radiation that can be perceived by the human eye. Visible light is usually defined as having wavelengths in the range of 400–700 nanometres (nm), corresponding to frequencies of 750–420 tera ...
and other electromagnetic radiation
In physics, electromagnetic radiation (EMR) consists of waves of the electromagnetic field, electromagnetic (EM) field, which propagate through space and carry momentum and electromagnetic radiant energy. It includes radio waves, microwaves, inf ...
the strength of the electric
Electricity is the set of physical phenomena associated with the presence and motion of matter that has a property of electric charge. Electricity is related to magnetism, both being part of the phenomenon of electromagnetism, as described by ...
and the magnetic field
A magnetic field is a vector field that describes the magnetic influence on moving electric charges, electric currents, and magnetic materials. A moving charge in a magnetic field experiences a force perpendicular to its own velocity and to ...
vary. Water waves are variations in the height of a body of water. In a crystal lattice vibration
In physics, a phonon is a collective excitation in a periodic, elastic arrangement of atoms or molecules in condensed matter, specifically in solids and some liquids. A type of quasiparticle, a phonon is an excited state in the quantum mechanical ...
, atomic positions vary.
The range of wavelengths or frequencies for wave phenomena is called a spectrum
A spectrum (plural ''spectra'' or ''spectrums'') is a condition that is not limited to a specific set of values but can vary, without gaps, across a continuum. The word was first used scientifically in optics to describe the rainbow of colors i ...
. The name originated with the visible light spectrum
The visible spectrum is the portion of the electromagnetic spectrum that is visible to the human eye. Electromagnetic radiation in this range of wavelengths is called ''visible light'' or simply light. A typical human eye will respond to wav ...
but now can be applied to the entire electromagnetic spectrum
The electromagnetic spectrum is the range of frequencies (the spectrum) of electromagnetic radiation and their respective wavelengths and photon energies.
The electromagnetic spectrum covers electromagnetic waves with frequencies ranging from ...
as well as to a sound spectrum
In the physical sciences, the term ''spectrum'' was introduced first into optics by Isaac Newton in the 17th century, referring to the range of colors observed when white light was dispersed through a prism.
Soon the term referred to a plot o ...
or vibration spectrum
A molecular vibration is a periodic motion of the atoms of a molecule relative to each other, such that the center of mass of the molecule remains unchanged. The typical vibrational frequencies range from less than 1013 Hz to approximately 1014 Hz ...
.
Sinusoidal waves
Inlinear
Linearity is the property of a mathematical relationship (''function'') that can be graphically represented as a straight line. Linearity is closely related to '' proportionality''. Examples in physics include rectilinear motion, the linear r ...
media, any wave pattern can be described in terms of the independent propagation of sinusoidal components. The wavelength ''λ'' of a sinusoidal waveform traveling at constant speed '''' is given by
:
where '''' is called the phase speed (magnitude of the phase velocity
The phase velocity of a wave is the rate at which the wave propagates in any medium. This is the velocity at which the phase of any one frequency component of the wave travels. For such a component, any given phase of the wave (for example, ...
) of the wave and '''' is the wave's frequency
Frequency is the number of occurrences of a repeating event per unit of time. It is also occasionally referred to as ''temporal frequency'' for clarity, and is distinct from ''angular frequency''. Frequency is measured in hertz (Hz) which is eq ...
. In a dispersive medium
In optics, and by analogy other branches of physics dealing with wave propagation, dispersion is the phenomenon in which the phase velocity of a wave depends on its frequency; sometimes the term chromatic dispersion is used for specificity to ...
, the phase speed itself depends upon the frequency of the wave, making the relationship between wavelength and frequency nonlinear.
In the case of electromagnetic radiation
In physics, electromagnetic radiation (EMR) consists of waves of the electromagnetic field, electromagnetic (EM) field, which propagate through space and carry momentum and electromagnetic radiant energy. It includes radio waves, microwaves, inf ...
—such as light—in free space
A vacuum is a space devoid of matter. The word is derived from the Latin adjective ''vacuus'' for "vacant" or "void". An approximation to such vacuum is a region with a gaseous pressure much less than atmospheric pressure. Physicists often dis ...
, the phase speed is the speed of light
The speed of light in vacuum, commonly denoted , is a universal physical constant that is important in many areas of physics. The speed of light is exactly equal to ). According to the special theory of relativity, is the upper limit ...
, about 3×108 m/s. Thus the wavelength of a 100 MHz electromagnetic (radio) wave is about: 3×108 m/s divided by 108 Hz = 3 metres. The wavelength of visible light ranges from deep red
Red is the color at the long wavelength end of the visible spectrum of light, next to orange and opposite violet. It has a dominant wavelength of approximately 625–740 nanometres. It is a primary color in the RGB color model and a secondar ...
, roughly 700 nm, to violet
Violet may refer to:
Common meanings
* Violet (color), a spectral color with wavelengths shorter than blue
* One of a list of plants known as violet, particularly:
** ''Viola'' (plant), a genus of flowering plants
Places United States
* Viol ...
, roughly 400 nm (for other examples, see electromagnetic spectrum
The electromagnetic spectrum is the range of frequencies (the spectrum) of electromagnetic radiation and their respective wavelengths and photon energies.
The electromagnetic spectrum covers electromagnetic waves with frequencies ranging from ...
).
For sound wave
In physics, sound is a vibration that propagates as an acoustic wave, through a transmission medium such as a gas, liquid or solid.
In human physiology and psychology, sound is the ''reception'' of such waves and their ''perception'' by the ...
s in air, the speed of sound
The speed of sound is the distance travelled per unit of time by a sound wave as it propagates through an elastic medium. At , the speed of sound in air is about , or one kilometre in or one mile in . It depends strongly on temperature as w ...
is 343 m/s (at room temperature and atmospheric pressure). The wavelengths of sound frequencies audible to the human ear (20 Hz–20 kHz) are thus between approximately 17 m and 17 mm, respectively. Somewhat higher frequencies are used by bat
Bats are mammals of the order Chiroptera.''cheir'', "hand" and πτερόν''pteron'', "wing". With their forelimbs adapted as wings, they are the only mammals capable of true and sustained flight. Bats are more agile in flight than most ...
s so they can resolve targets smaller than 17 mm. Wavelengths in audible sound are much longer than those in visible light.

Standing waves
Astanding wave
In physics, a standing wave, also known as a stationary wave, is a wave that oscillates in time but whose peak amplitude profile does not move in space. The peak amplitude of the wave oscillations at any point in space is constant with respect ...
is an undulatory motion that stays in one place. A sinusoidal standing wave includes stationary points of no motion, called nodes
In general, a node is a localized swelling (a "knot") or a point of intersection (a Vertex (graph theory), vertex).
Node may refer to:
In mathematics
*Vertex (graph theory), a vertex in a mathematical graph
*Vertex (geometry), a point where two ...
, and the wavelength is twice the distance between nodes.
The upper figure shows three standing waves in a box. The walls of the box are considered to require the wave to have nodes at the walls of the box (an example of boundary conditions
In mathematics, in the field of differential equations, a boundary value problem is a differential equation together with a set of additional constraints, called the boundary conditions. A solution to a boundary value problem is a solution to th ...
) determining which wavelengths are allowed. For example, for an electromagnetic wave, if the box has ideal metal walls, the condition for nodes at the walls results because the metal walls cannot support a tangential electric field, forcing the wave to have zero amplitude at the wall.
The stationary wave can be viewed as the sum of two traveling sinusoidal waves of oppositely directed velocities. Consequently, wavelength, period, and wave velocity are related just as for a traveling wave. For example, the speed of light
The speed of light in vacuum, commonly denoted , is a universal physical constant that is important in many areas of physics. The speed of light is exactly equal to ). According to the special theory of relativity, is the upper limit ...
can be determined from observation of standing waves in a metal box containing an ideal vacuum.
Mathematical representation
Traveling sinusoidal waves are often represented mathematically in terms of their velocity ''v'' (in the x direction), frequency ''f'' and wavelength ''λ'' as: : where ''y'' is the value of the wave at any position ''x'' and time ''t'', and ''A'' is theamplitude
The amplitude of a periodic variable is a measure of its change in a single period (such as time or spatial period). The amplitude of a non-periodic signal is its magnitude compared with a reference value. There are various definitions of amplit ...
of the wave. They are also commonly expressed in terms of wavenumber
In the physical sciences, the wavenumber (also wave number or repetency) is the ''spatial frequency'' of a wave, measured in cycles per unit distance (ordinary wavenumber) or radians per unit distance (angular wavenumber). It is analogous to temp ...
''k'' (2π times the reciprocal of wavelength) and angular frequency
In physics, angular frequency "''ω''" (also referred to by the terms angular speed, circular frequency, orbital frequency, radian frequency, and pulsatance) is a scalar measure of rotation rate. It refers to the angular displacement per unit tim ...
''ω'' (2π times the frequency) as:
:
in which wavelength and wavenumber are related to velocity and frequency as:
:
or
:
In the second form given above, the phase is often generalized to , by replacing the wavenumber ''k'' with a wave vector
In physics, a wave vector (or wavevector) is a vector used in describing a wave, with a typical unit being cycle per metre. It has a magnitude and direction. Its magnitude is the wavenumber of the wave (inversely proportional to the wavelength), ...
that specifies the direction and wavenumber of a plane wave
In physics, a plane wave is a special case of wave or field: a physical quantity whose value, at any moment, is constant through any plane that is perpendicular to a fixed direction in space.
For any position \vec x in space and any time t, th ...
in 3-space
Three-dimensional space (also: 3D space, 3-space or, rarely, tri-dimensional space) is a geometric setting in which three values (called ''parameters'') are required to determine the position of an element (i.e., point). This is the informa ...
, parameterized by position vector r. In that case, the wavenumber ''k'', the magnitude of k, is still in the same relationship with wavelength as shown above, with ''v'' being interpreted as scalar speed in the direction of the wave vector. The first form, using reciprocal wavelength in the phase, does not generalize as easily to a wave in an arbitrary direction.
Generalizations to sinusoids of other phases, and to complex exponentials, are also common; see plane wave
In physics, a plane wave is a special case of wave or field: a physical quantity whose value, at any moment, is constant through any plane that is perpendicular to a fixed direction in space.
For any position \vec x in space and any time t, th ...
. The typical convention of using the cosine phase instead of the sine
In mathematics, sine and cosine are trigonometric functions of an angle. The sine and cosine of an acute angle are defined in the context of a right triangle: for the specified angle, its sine is the ratio of the length of the side that is oppo ...
phase when describing a wave is based on the fact that the cosine is the real part of the complex exponential in the wave
:
General media
 The speed of a wave depends upon the medium in which it propagates. In particular, the speed of light in a medium is less than in
The speed of a wave depends upon the medium in which it propagates. In particular, the speed of light in a medium is less than in vacuum
A vacuum is a space devoid of matter. The word is derived from the Latin adjective ''vacuus'' for "vacant" or "void". An approximation to such vacuum is a region with a gaseous pressure much less than atmospheric pressure. Physicists often dis ...
, which means that the same frequency will correspond to a shorter wavelength in the medium than in vacuum, as shown in the figure at right.
This change in speed upon entering a medium causes refraction
In physics, refraction is the redirection of a wave as it passes from one medium to another. The redirection can be caused by the wave's change in speed or by a change in the medium. Refraction of light is the most commonly observed phenomeno ...
, or a change in direction of waves that encounter the interface between media at an angle.
To aid imagination, this bending of the wave often is compared to the analogy of a column of marching soldiers crossing from solid ground into mud. See, for example,
For electromagnetic waves
In physics, electromagnetic radiation (EMR) consists of waves of the electromagnetic (EM) field, which propagate through space and carry momentum and electromagnetic radiant energy. It includes radio waves, microwaves, infrared, (visible) lig ...
, this change in the angle of propagation is governed by Snell's law
Snell's law (also known as Snell–Descartes law and ibn-Sahl law and the law of refraction) is a formula used to describe the relationship between the angles of incidence and refraction, when referring to light or other waves passing through ...
.
The wave velocity in one medium not only may differ from that in another, but the velocity typically varies with wavelength. As a result, the change in direction upon entering a different medium changes with the wavelength of the wave.
For electromagnetic waves the speed in a medium is governed by its ''refractive index
In optics, the refractive index (or refraction index) of an optical medium is a dimensionless number that gives the indication of the light bending ability of that medium.
The refractive index determines how much the path of light is bent, or ...
'' according to
:
where ''c'' is the speed of light
The speed of light in vacuum, commonly denoted , is a universal physical constant that is important in many areas of physics. The speed of light is exactly equal to ). According to the special theory of relativity, is the upper limit ...
in vacuum and ''n''(λ0) is the refractive index of the medium at wavelength λ0, where the latter is measured in vacuum rather than in the medium. The corresponding wavelength in the medium is
:
When wavelengths of electromagnetic radiation are quoted, the wavelength in vacuum usually is intended unless the wavelength is specifically identified as the wavelength in some other medium. In acoustics, where a medium is essential for the waves to exist, the wavelength value is given for a specified medium.
The variation in speed of light with wavelength is known as dispersion
Dispersion may refer to:
Economics and finance
*Dispersion (finance), a measure for the statistical distribution of portfolio returns
*Price dispersion, a variation in prices across sellers of the same item
*Wage dispersion, the amount of variatio ...
, and is also responsible for the familiar phenomenon in which light is separated into component colors by a prism
Prism usually refers to:
* Prism (optics), a transparent optical component with flat surfaces that refract light
* Prism (geometry), a kind of polyhedron
Prism may also refer to:
Science and mathematics
* Prism (geology), a type of sedimentary ...
. Separation occurs when the refractive index inside the prism varies with wavelength, so different wavelengths propagate at different speeds inside the prism, causing them to refract
In physics, refraction is the redirection of a wave as it passes from one medium to another. The redirection can be caused by the wave's change in speed or by a change in the medium. Refraction of light is the most commonly observed phenomeno ...
at different angles. The mathematical relationship that describes how the speed of light within a medium varies with wavelength is known as a dispersion relation
In the physical sciences and electrical engineering, dispersion relations describe the effect of dispersion on the properties of waves in a medium. A dispersion relation relates the wavelength or wavenumber of a wave to its frequency. Given the d ...
.
Nonuniform media
 Waves that are sinusoidal in time but propagate through a medium whose properties vary with position (an ''inhomogeneous'' medium) may propagate at a velocity that varies with position, and as a result may not be sinusoidal in space. The figure at right shows an example. As the wave slows down, the wavelength gets shorter and the amplitude increases; after a place of maximum response, the short wavelength is associated with a high loss and the wave dies out.
The analysis of
Waves that are sinusoidal in time but propagate through a medium whose properties vary with position (an ''inhomogeneous'' medium) may propagate at a velocity that varies with position, and as a result may not be sinusoidal in space. The figure at right shows an example. As the wave slows down, the wavelength gets shorter and the amplitude increases; after a place of maximum response, the short wavelength is associated with a high loss and the wave dies out.
The analysis of differential equation
In mathematics, a differential equation is an equation that relates one or more unknown functions and their derivatives. In applications, the functions generally represent physical quantities, the derivatives represent their rates of change, an ...
s of such systems is often done approximately, using the ''WKB method
In mathematical physics, the WKB approximation or WKB method is a method for finding approximate solutions to linear differential equations with spatially varying coefficients. It is typically used for a semiclassical calculation in quantum mecha ...
'' (also known as the ''Liouville–Green method''). The method integrates phase through space using a local wavenumber
In the physical sciences, the wavenumber (also wave number or repetency) is the ''spatial frequency'' of a wave, measured in cycles per unit distance (ordinary wavenumber) or radians per unit distance (angular wavenumber). It is analogous to temp ...
, which can be interpreted as indicating a "local wavelength" of the solution as a function of time and space.
This method treats the system locally as if it were uniform with the local properties; in particular, the local wave velocity associated with a frequency is the only thing needed to estimate the corresponding local wavenumber or wavelength. In addition, the method computes a slowly changing amplitude to satisfy other constraints of the equations or of the physical system, such as for conservation of energy
In physics and chemistry, the law of conservation of energy states that the total energy of an isolated system remains constant; it is said to be ''conserved'' over time. This law, first proposed and tested by Émilie du Châtelet, means th ...
in the wave.
Crystals
aliasing
In signal processing and related disciplines, aliasing is an effect that causes different signals to become indistinguishable (or ''aliases'' of one another) when sampled. It also often refers to the distortion or artifact that results when a ...
because the same vibration can be considered to have a variety of different wavelengths, as shown in the figure.See Figure 4.20 in and Figure 2.3 in Descriptions using more than one of these wavelengths are redundant; it is conventional to choose the longest wavelength that fits the phenomenon. The range of wavelengths sufficient to provide a description of all possible waves in a crystalline medium corresponds to the wave vectors confined to the Brillouin zone
In mathematics and solid state physics, the first Brillouin zone is a uniquely defined primitive cell in reciprocal space. In the same way the Bravais lattice is divided up into Wigner–Seitz cells in the real lattice, the reciprocal lattice i ...
.
This indeterminacy in wavelength in solids is important in the analysis of wave phenomena such as energy bands and lattice vibrations. It is mathematically equivalent to the aliasing
In signal processing and related disciplines, aliasing is an effect that causes different signals to become indistinguishable (or ''aliases'' of one another) when sampled. It also often refers to the distortion or artifact that results when a ...
of a signal that is sampled
Sample or samples may refer to:
Base meaning
* Sample (statistics), a subset of a population – complete data set
* Sample (signal), a digital discrete sample of a continuous analog signal
* Sample (material), a specimen or small quantity of so ...
at discrete intervals.
More general waveforms
 The concept of wavelength is most often applied to sinusoidal, or nearly sinusoidal, waves, because in a linear system the sinusoid is the unique shape that propagates with no shape change – just a phase change and potentially an amplitude change.
See
The wavelength (or alternatively
The concept of wavelength is most often applied to sinusoidal, or nearly sinusoidal, waves, because in a linear system the sinusoid is the unique shape that propagates with no shape change – just a phase change and potentially an amplitude change.
See
The wavelength (or alternatively wavenumber
In the physical sciences, the wavenumber (also wave number or repetency) is the ''spatial frequency'' of a wave, measured in cycles per unit distance (ordinary wavenumber) or radians per unit distance (angular wavenumber). It is analogous to temp ...
or wave vector
In physics, a wave vector (or wavevector) is a vector used in describing a wave, with a typical unit being cycle per metre. It has a magnitude and direction. Its magnitude is the wavenumber of the wave (inversely proportional to the wavelength), ...
) is a characterization of the wave in space, that is functionally related to its frequency, as constrained by the physics of the system. Sinusoids are the simplest traveling wave
In physics, mathematics, and related fields, a wave is a propagating dynamic disturbance (change from equilibrium) of one or more quantities. Waves can be periodic, in which case those quantities oscillate repeatedly about an equilibrium (re ...
solutions, and more complex solutions can be built up by superposition.
In the special case of dispersion-free and uniform media, waves other than sinusoids propagate with unchanging shape and constant velocity. In certain circumstances, waves of unchanging shape also can occur in nonlinear media; for example, the figure shows ocean waves in shallow water that have sharper crests and flatter troughs than those of a sinusoid, typical of a cnoidal wave
In fluid dynamics, a cnoidal wave is a nonlinear and exact periodic wave solution of the Korteweg–de Vries equation. These solutions are in terms of the Jacobi elliptic function ''cn'', which is why they are coined ''cn''oidal waves. They are ...
,
a traveling wave so named because it is described by the Jacobi elliptic function In mathematics, the Jacobi elliptic functions are a set of basic elliptic functions. They are found in the description of the motion of a pendulum (see also pendulum (mathematics)), as well as in the design of electronic elliptic filters. While tri ...
of ''m''-th order, usually denoted as .
Large-amplitude ocean wave
In fluid dynamics, a wind wave, water wave, or wind-generated water wave, is a surface wave that occurs on the free surface of bodies of water as a result from the wind blowing over the water surface. The contact distance in the direction o ...
s with certain shapes can propagate unchanged, because of properties of the nonlinear surface-wave medium.
Wave packets
 Localized
Localized wave packet
In physics, a wave packet (or wave train) is a short "burst" or "envelope" of localized wave action that travels as a unit. A wave packet can be analyzed into, or can be synthesized from, an infinite set of component sinusoidal waves of diffe ...
s, "bursts" of wave action where each wave packet travels as a unit, find application in many fields of physics. A wave packet has an ''envelope'' that describes the overall amplitude of the wave; within the envelope, the distance between adjacent peaks or troughs is sometimes called a ''local wavelength''.
An example is shown in the figure. In general, the ''envelope'' of the wave packet moves at a speed different from the constituent waves.
Using Fourier analysis
In mathematics, Fourier analysis () is the study of the way general functions may be represented or approximated by sums of simpler trigonometric functions. Fourier analysis grew from the study of Fourier series, and is named after Josep ...
, wave packets can be analyzed into infinite sums (or integrals) of sinusoidal waves of different wavenumber
In the physical sciences, the wavenumber (also wave number or repetency) is the ''spatial frequency'' of a wave, measured in cycles per unit distance (ordinary wavenumber) or radians per unit distance (angular wavenumber). It is analogous to temp ...
s or wavelengths.See, for example, Figs. 2.8–2.10 in
Louis de Broglie
Louis Victor Pierre Raymond, 7th Duc de Broglie (, also , or ; 15 August 1892 – 19 March 1987) was a French physicist and aristocrat who made groundbreaking contributions to quantum theory. In his 1924 PhD thesis, he postulated the wave na ...
postulated that all particles with a specific value of momentum
In Newtonian mechanics, momentum (more specifically linear momentum or translational momentum) is the product of the mass and velocity of an object. It is a vector quantity, possessing a magnitude and a direction. If is an object's mass an ...
''p'' have a wavelength ''λ = h/p'', where ''h'' is Planck's constant. This hypothesis was at the basis of quantum mechanics
Quantum mechanics is a fundamental theory in physics that provides a description of the physical properties of nature at the scale of atoms and subatomic particles. It is the foundation of all quantum physics including quantum chemistry, ...
. Nowadays, this wavelength is called the de Broglie wavelength
Matter waves are a central part of the theory of quantum mechanics, being an example of wave–particle duality. All matter exhibits wave-like behavior. For example, a beam of electrons can be diffracted just like a beam of light or a water wave ...
. For example, the electron
The electron ( or ) is a subatomic particle with a negative one elementary electric charge. Electrons belong to the first generation of the lepton particle family,
and are generally thought to be elementary particles because they have no kn ...
s in a CRT display have a De Broglie wavelength of about 10−13 m. To prevent the wave function
A wave function in quantum physics is a mathematical description of the quantum state of an isolated quantum system. The wave function is a complex-valued probability amplitude, and the probabilities for the possible results of measurements mad ...
for such a particle being spread over all space, de Broglie proposed using wave packets to represent particles that are localized in space.
The spatial spread of the wave packet, and the spread of the wavenumber
In the physical sciences, the wavenumber (also wave number or repetency) is the ''spatial frequency'' of a wave, measured in cycles per unit distance (ordinary wavenumber) or radians per unit distance (angular wavenumber). It is analogous to temp ...
s of sinusoids that make up the packet, correspond to the uncertainties in the particle's position and momentum, the product of which is bounded by Heisenberg uncertainty principle
In quantum mechanics, the uncertainty principle (also known as Heisenberg's uncertainty principle) is any of a variety of mathematical inequalities asserting a fundamental limit to the accuracy with which the values for certain pairs of physic ...
.
Interference and diffraction
Double-slit interference
interferometer
Interferometry is a technique which uses the ''interference'' of superimposed waves to extract information. Interferometry typically uses electromagnetic waves and is an important investigative technique in the fields of astronomy, fiber op ...
. A simple example is an experiment due to Young
Young may refer to:
* Offspring, the product of reproduction of a new organism produced by one or more parents
* Youth, the time of life when one is young, often meaning the time between childhood and adulthood
Music
* The Young, an American roc ...
where light is passed through two slits.
As shown in the figure, light is passed through two slits and shines on a screen. The path of the light to a position on the screen is different for the two slits, and depends upon the angle θ the path makes with the screen. If we suppose the screen is far enough from the slits (that is, ''s'' is large compared to the slit separation ''d'') then the paths are nearly parallel, and the path difference is simply ''d'' sin θ. Accordingly, the condition for constructive interference is:
:
where ''m'' is an integer, and for destructive interference is:
:
Thus, if the wavelength of the light is known, the slit separation can be determined from the interference pattern or ''fringes'', and ''vice versa''.
For multiple slits, the pattern is
:
where ''q'' is the number of slits, and ''g'' is the grating constant. The first factor, ''I''1, is the single-slit result, which modulates the more rapidly varying second factor that depends upon the number of slits and their spacing. In the figure ''I''1 has been set to unity, a very rough approximation.
The effect of interference is to ''redistribute'' the light, so the energy contained in the light is not altered, just where it shows up.
Single-slit diffraction
 The notion of path difference and constructive or destructive interference used above for the double-slit experiment applies as well to the display of a single slit of light intercepted on a screen. The main result of this interference is to spread out the light from the narrow slit into a broader image on the screen. This distribution of wave energy is called
The notion of path difference and constructive or destructive interference used above for the double-slit experiment applies as well to the display of a single slit of light intercepted on a screen. The main result of this interference is to spread out the light from the narrow slit into a broader image on the screen. This distribution of wave energy is called diffraction
Diffraction is defined as the interference or bending of waves around the corners of an obstacle or through an aperture into the region of geometrical shadow of the obstacle/aperture. The diffracting object or aperture effectively becomes a s ...
.
Two types of diffraction are distinguished, depending upon the separation between the source and the screen: Fraunhofer diffraction
In optics, the Fraunhofer diffraction equation is used to model the diffraction of waves when plane waves are incident on a diffracting object, and the diffraction pattern is viewed at a sufficiently long distance (a distance satisfying Fraunhofer ...
or far-field diffraction at large separations and Fresnel diffraction
In optics, the Fresnel diffraction equation for near-field diffraction is an approximation of the Kirchhoff–Fresnel diffraction that can be applied to the propagation of waves in the near field. It is used to calculate the diffraction pattern ...
or near-field diffraction at close separations.
In the analysis of the single slit, the non-zero width of the slit is taken into account, and each point in the aperture is taken as the source of one contribution to the beam of light (''Huygens' wavelets''). On the screen, the light arriving from each position within the slit has a different path length, albeit possibly a very small difference. Consequently, interference occurs.
In the Fraunhofer diffraction pattern sufficiently far from a single slit, within a small-angle approximation
The small-angle approximations can be used to approximate the values of the main trigonometric functions, provided that the angle in question is small and is measured in radians:
:
\begin
\sin \theta &\approx \theta \\
\cos \theta &\approx 1 - \ ...
, the intensity spread ''S'' is related to position ''x'' via a squared sinc function
In mathematics, physics and engineering, the sinc function, denoted by , has two forms, normalized and unnormalized..
In mathematics, the historical unnormalized sinc function is defined for by
\operatornamex = \frac.
Alternatively, the u ...
:
: with
where ''L'' is the slit width, ''R'' is the distance of the pattern (on the screen) from the slit, and λ is the wavelength of light used. The function ''S'' has zeros where ''u'' is a non-zero integer, where are at ''x'' values at a separation proportion to wavelength.
Diffraction-limited resolution
Diffraction is the fundamental limitation on the resolving power of optical instruments, such astelescope
A telescope is a device used to observe distant objects by their emission, absorption, or reflection of electromagnetic radiation. Originally meaning only an optical instrument using lenses, curved mirrors, or a combination of both to observe ...
s (including radiotelescope
A radio telescope is a specialized antenna and radio receiver used to detect radio waves from astronomical radio sources in the sky. Radio telescopes are the main observing instrument used in radio astronomy, which studies the radio frequency po ...
s) and microscopes
A microscope () is a laboratory instrument used to examine objects that are too small to be seen by the naked eye. Microscopy is the science of investigating small objects and structures using a microscope. Microscopic means being invisibl ...
.
For a circular aperture, the diffraction-limited image spot is known as an Airy disk
In optics, the Airy disk (or Airy disc) and Airy pattern are descriptions of the best- focused spot of light that a perfect lens with a circular aperture can make, limited by the diffraction of light. The Airy disk is of importance in physics, ...
; the distance ''x'' in the single-slit diffraction formula is replaced by radial distance ''r'' and the sine is replaced by 2''J''1, where ''J''1 is a first order Bessel function
Bessel functions, first defined by the mathematician Daniel Bernoulli and then generalized by Friedrich Bessel, are canonical solutions of Bessel's differential equation
x^2 \frac + x \frac + \left(x^2 - \alpha^2 \right)y = 0
for an arbitrary ...
.
The resolvable ''spatial'' size of objects viewed through a microscope is limited according to the Rayleigh criterion
Angular resolution describes the ability of any image-forming device such as an optical or radio telescope, a microscope, a camera, or an eye, to distinguish small details of an object, thereby making it a major determinant of image resolution. ...
, the radius to the first null of the Airy disk, to a size proportional to the wavelength of the light used, and depending on the numerical aperture
In optics, the numerical aperture (NA) of an optical system is a dimensionless number that characterizes the range of angles over which the system can accept or emit light. By incorporating index of refraction in its definition, NA has the proper ...
:
:
where the numerical aperture is defined as for θ being the half-angle of the cone of rays accepted by the microscope objective
In optical engineering, the objective is the optical element that gathers light from the object being observed and focuses the light rays to produce a real image. Objectives can be a single lens or mirror, or combinations of several optical elem ...
.
The ''angular'' size of the central bright portion (radius to first null of the Airy disk
In optics, the Airy disk (or Airy disc) and Airy pattern are descriptions of the best- focused spot of light that a perfect lens with a circular aperture can make, limited by the diffraction of light. The Airy disk is of importance in physics, ...
) of the image diffracted by a circular aperture, a measure most commonly used for telescopes and cameras, is:
:
where λ is the wavelength of the waves that are focused for imaging, ''D'' the entrance pupil
In an optical system, the entrance pupil is the optical image of the physical aperture stop, as 'seen' through the front (the object side) of the lens system. The corresponding image of the aperture as seen through the back of the lens system is ...
diameter of the imaging system, in the same units, and the angular resolution δ is in radians.
As with other diffraction patterns, the pattern scales in proportion to wavelength, so shorter wavelengths can lead to higher resolution.
Subwavelength
The term ''subwavelength'' is used to describe an object having one or more dimensions smaller than the length of the wave with which the object interacts. For example, the term ''subwavelength-diameter optical fibre
A subwavelength-diameter optical fibre (SDF or SDOF) is an optical fibre whose diameter is less than the wavelength of the light being propagated through it. An SDF usually consists of long thick parts (same as conventional optical fibres) at both ...
'' means an optical fibre
An optical fiber, or optical fibre in Commonwealth English, is a flexible, transparent fiber made by drawing glass (silica) or plastic to a diameter slightly thicker than that of a human hair. Optical fibers are used most often as a means to ...
whose diameter is less than the wavelength of light propagating through it.
A subwavelength particle is a particle smaller than the wavelength of light with which it interacts (see Rayleigh scattering
Rayleigh scattering ( ), named after the 19th-century British physicist Lord Rayleigh (John William Strutt), is the predominantly elastic scattering of light or other electromagnetic radiation by particles much smaller than the wavelength of the ...
). Subwavelength aperture
In optics, an aperture is a hole or an opening through which light travels. More specifically, the aperture and focal length of an optical system determine the cone angle of a bundle of rays that come to a focus in the image plane.
An opt ...
s are holes smaller than the wavelength of light propagating through them. Such structures have applications in extraordinary optical transmission, and zero-mode waveguide zero-mode waveguide is an optical waveguide that guides light energy into a volume that is small in all dimensions compared to the wavelength of the light.
Zero-mode waveguides have been developed for rapid parallel sensing of zeptolitre sample vo ...
s, among other areas of photonics
Photonics is a branch of optics that involves the application of generation, detection, and manipulation of light in form of photons through emission, transmission, modulation, signal processing, switching, amplification, and sensing. Though ...
.
''Subwavelength'' may also refer to a phenomenon involving subwavelength objects; for example, subwavelength imaging.
Angular wavelength
reduced Planck constant
The Planck constant, or Planck's constant, is a fundamental physical constant of foundational importance in quantum mechanics. The constant gives the relationship between the energy of a photon and its frequency, and by the mass-energy equivale ...
(symbol ''ħ'', h-bar) and the angular frequency
In physics, angular frequency "''ω''" (also referred to by the terms angular speed, circular frequency, orbital frequency, radian frequency, and pulsatance) is a scalar measure of rotation rate. It refers to the angular displacement per unit tim ...
(symbol ''ω'') or angular wavenumber
In the physical sciences, the wavenumber (also wave number or repetency) is the ''spatial frequency'' of a wave, measured in cycles per unit distance (ordinary wavenumber) or radians per unit distance (angular wavenumber). It is analogous to temp ...
(symbol ''k''). It is also used to measure the amplitude of mechanical waves, where the amplitude is equal to displacement divided by reduced wavelength.
See also
*Emission spectrum
The emission spectrum of a chemical element or chemical compound is the spectrum of frequencies of electromagnetic radiation emitted due to an electron making a atomic electron transition, transition from a high energy state to a lower energy st ...
* Envelope (waves)
In physics and engineering, the envelope of an oscillating signal is a smooth curve outlining its extremes. The envelope thus generalizes the concept of a constant amplitude into an instantaneous amplitude. The figure illustrates a modulated sine ...
* Fraunhofer lines
In physics and optics, the Fraunhofer lines are a set of spectral absorption lines named after the German physicist Joseph von Fraunhofer (1787–1826). The lines were originally observed as dark features (absorption lines) in the optical spectru ...
– dark lines in the solar spectrum, traditionally used as standard optical wavelength references
* Index of wave articles
This is a list of Wave topics.
0–9
*21 cm line
A
*Abbe prism
*Absorption spectroscopy
*Absorption spectrum
* Absorption wavemeter
*Acoustic wave
* Acoustic wave equation
*Acoustics
* Acousto-optic effect
*Acousto-optic modulator
*Acous ...
* Length measurement
Length measurement, distance measurement, or range measurement (ranging) refers to the many ways in which length, distance, or range can be measured. The most commonly used approaches are the rulers, followed by transit-time methods and the ...
* Spectral line
A spectral line is a dark or bright line in an otherwise uniform and continuous spectrum, resulting from emission or absorption of light in a narrow frequency range, compared with the nearby frequencies. Spectral lines are often used to iden ...
* Spectroscopy
Spectroscopy is the field of study that measures and interprets the electromagnetic spectra that result from the interaction between electromagnetic radiation and matter as a function of the wavelength or frequency of the radiation. Matter wa ...
* Spectrum
A spectrum (plural ''spectra'' or ''spectrums'') is a condition that is not limited to a specific set of values but can vary, without gaps, across a continuum. The word was first used scientifically in optics to describe the rainbow of colors i ...
References
External links
Conversion: Wavelength to Frequency and vice versa – Sound waves and radio waves
* ttp://www.magnetkern.de/spektrum.html The visible electromagnetic spectrum displayed in web colors with according wavelengths {{Authority control Waves Length