Snub Antiprism on:
[Wikipedia]
[Google]
[Amazon]
 In
In
The symmetry group contains inversion
The right -antiprisms have congruent regular -gon bases and congruent isosceles triangle side faces, thus have the same (dihedral) symmetry group as the uniform -antiprism, for .
 In
In geometry
Geometry (; ) is a branch of mathematics concerned with properties of space such as the distance, shape, size, and relative position of figures. Geometry is, along with arithmetic, one of the oldest branches of mathematics. A mathematician w ...
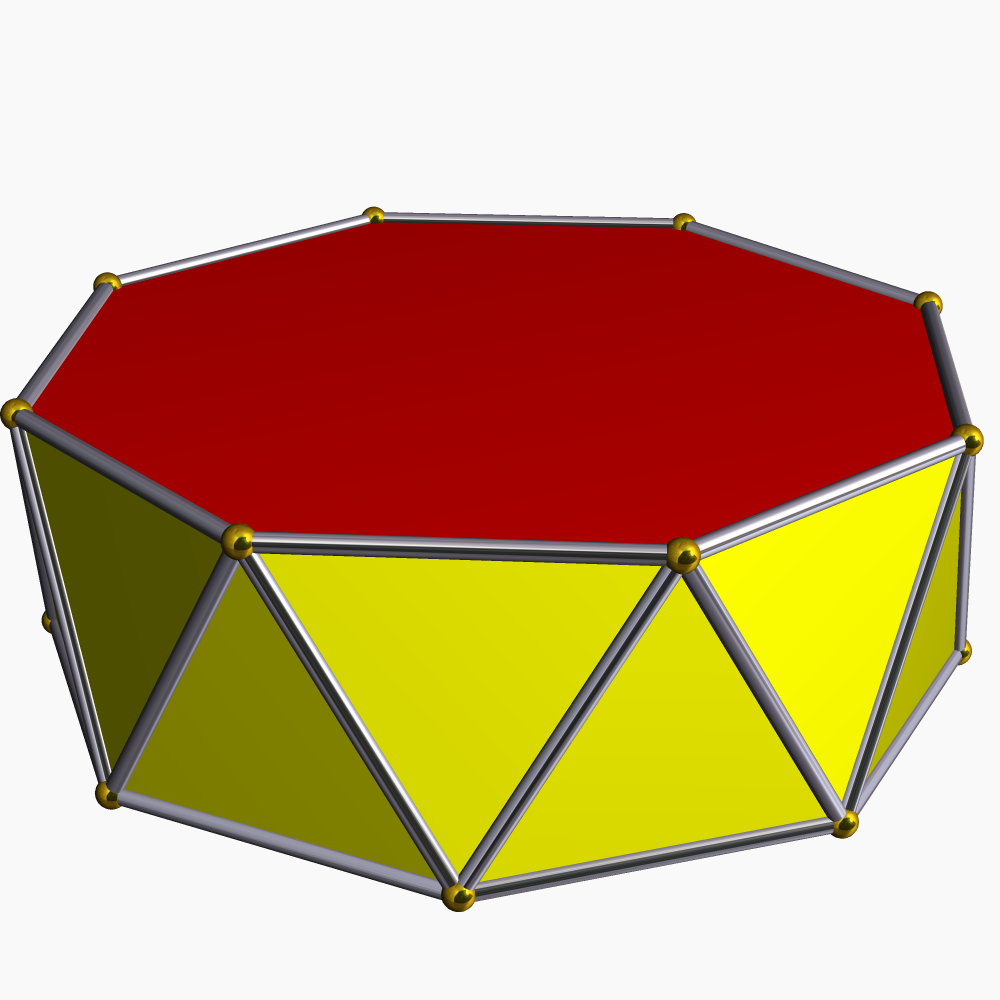
, an antiprism or is a polyhedron
In geometry, a polyhedron (: polyhedra or polyhedrons; ) is a three-dimensional figure with flat polygonal Face (geometry), faces, straight Edge (geometry), edges and sharp corners or Vertex (geometry), vertices. The term "polyhedron" may refer ...
composed of two parallel
Parallel may refer to:
Mathematics
* Parallel (geometry), two lines in the Euclidean plane which never intersect
* Parallel (operator), mathematical operation named after the composition of electrical resistance in parallel circuits
Science a ...
direct
Direct may refer to:
Mathematics
* Directed set, in order theory
* Direct limit of (pre), sheaves
* Direct sum of modules, a construction in abstract algebra which combines several vector spaces
Computing
* Direct access (disambiguation), ...
copies (not mirror images) of an polygon
In geometry, a polygon () is a plane figure made up of line segments connected to form a closed polygonal chain.
The segments of a closed polygonal chain are called its '' edges'' or ''sides''. The points where two edges meet are the polygon ...
, connected by an alternating band of triangle
A triangle is a polygon with three corners and three sides, one of the basic shapes in geometry. The corners, also called ''vertices'', are zero-dimensional points while the sides connecting them, also called ''edges'', are one-dimension ...
s. They are represented by the Conway notation .
Antiprisms are a subclass of prismatoid
In geometry, a prismatoid is a polyhedron whose vertex (geometry), vertices all lie in two parallel Plane (geometry), planes. Its lateral faces can be trapezoids or triangles. If both planes have the same number of vertices, and the lateral faces ...
s, and are a (degenerate) type of snub polyhedron
In geometry, a snub polyhedron is a polyhedron obtained by performing a Snub (geometry), snub operation: alternation (geometry), alternating a corresponding omnitruncation, omnitruncated or truncation (geometry), truncated polyhedron, depending ...
.
Antiprisms are similar to prisms, except that the bases are twisted relatively to each other, and that the side faces (connecting the bases) are triangles, rather than quadrilateral
In Euclidean geometry, geometry a quadrilateral is a four-sided polygon, having four Edge (geometry), edges (sides) and four Vertex (geometry), corners (vertices). The word is derived from the Latin words ''quadri'', a variant of four, and ''l ...
s.
The dual polyhedron
In geometry, every polyhedron is associated with a second dual structure, where the vertices of one correspond to the faces of the other, and the edges between pairs of vertices of one correspond to the edges between pairs of faces of the other ...
of an -gonal antiprism is an -gonal trapezohedron
In geometry, an trapezohedron, -trapezohedron, -antidipyramid, -antibipyramid, or -deltohedron Remarks: the faces of a deltohedron are deltoids; a (non-twisted) kite or deltoid can be Dissection (geometry), dissected into two isosceles triangle ...
.
History
In his 1619 book ''Harmonices Mundi
''Harmonice Mundi'' (Latin: ''The Harmony of the World'', 1619) is a book by Johannes Kepler. In the work, written entirely in Latin, Kepler discusses harmony and congruence in geometrical forms and physical phenomena. The final section of t ...
'', Johannes Kepler
Johannes Kepler (27 December 1571 – 15 November 1630) was a German astronomer, mathematician, astrologer, Natural philosophy, natural philosopher and writer on music. He is a key figure in the 17th-century Scientific Revolution, best know ...
observed the existence of the infinite family of antiprisms. This has conventionally been thought of as the first discovery of these shapes, but they may have been known earlier: an unsigned printing block for the net of a hexagonal antiprism
In geometry, the hexagonal antiprism is the 4th in an infinite set of antiprisms formed by an even-numbered sequence of triangle sides closed by two polygon caps.
Antiprisms are similar to prism (geometry), prisms except the bases are twisted re ...
has been attributed to Hieronymus Andreae
Hieronymus Andreae, or Andreä, or Hieronymus Formschneider, (died 7 May 1556) was a German woodblock cutter ("formschneider"), printer, publisher and typographer closely associated with Albrecht Dürer. Andreae's best known achievements inclu ...
, who died in 1556.
The German form of the word "antiprism" was used for these shapes in the 19th century; Karl Heinze credits its introduction to . Although the English "anti-prism" had been used earlier for an optical prism used to cancel the effects of a primary optical element, the first use of "antiprism" in English in its geometric sense appears to be in the early 20th century in the works of H. S. M. Coxeter.
Special cases
Right antiprism
For an antiprism with regular -gon bases, one usually considers the case where these two copies are twisted by an angle of degrees. The axis of a regular polygon is the lineperpendicular
In geometry, two geometric objects are perpendicular if they intersect at right angles, i.e. at an angle of 90 degrees or π/2 radians. The condition of perpendicularity may be represented graphically using the '' perpendicular symbol'', � ...
to the polygon plane and lying in the polygon centre.
For an antiprism with congruent
Congruence may refer to:
Mathematics
* Congruence (geometry), being the same size and shape
* Congruence or congruence relation, in abstract algebra, an equivalence relation on an algebraic structure that is compatible with the structure
* In modu ...
regular -gon bases, twisted by an angle of degrees, more regularity is obtained if the bases have the same axis: are ''coaxial
In geometry, coaxial means that several three-dimensional linear or planar forms share a common axis. The two-dimensional analog is ''concentric''.
Common examples:
A coaxial cable has a wire conductor in the centre (D), a circumferential ou ...
''; i.e. (for non-coplanar
In geometry, a set of points in space are coplanar if there exists a geometric plane that contains them all. For example, three points are always coplanar, and if the points are distinct and non-collinear, the plane they determine is unique. How ...
bases): if the line connecting the base centers is perpendicular to the base planes. Then the antiprism is called a right antiprism, and its side faces are isosceles triangle
In geometry, an isosceles triangle () is a triangle that has two Edge (geometry), sides of equal length and two angles of equal measure. Sometimes it is specified as having ''exactly'' two sides of equal length, and sometimes as having ''at le ...
s.
The symmetry group
In group theory, the symmetry group of a geometric object is the group of all transformations under which the object is invariant, endowed with the group operation of composition. Such a transformation is an invertible mapping of the amb ...
of a right -antiprism is of order known as an antiprismatic symmetry
In geometry, dihedral symmetry in three dimensions is one of three infinite sequences of point groups in three dimensions which have a symmetry group that as an abstract group is a dihedral group Dih''n'' (for ''n'' ≥ 2).
Types
Ther ...
, because it could be obtained by rotation of the bottom half of a prism by in relation to the top half. A concave polyhedron created in this way would have this symmetry group, hence prefix "anti" before "prismatic". There are two exceptions having groups different than :
*: the regular tetrahedron
In geometry, a tetrahedron (: tetrahedra or tetrahedrons), also known as a triangular pyramid, is a polyhedron composed of four triangular Face (geometry), faces, six straight Edge (geometry), edges, and four vertex (geometry), vertices. The tet ...
, which has the larger symmetry group of order , which has three versions of as subgroups;
*: the regular octahedron
In geometry, an octahedron (: octahedra or octahedrons) is any polyhedron with eight faces. One special case is the regular octahedron, a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex. Many types of i ...
, which has the larger symmetry group of order , which has four versions of as subgroups.
If a right 2- or 3-antiprism is not uniform, then its symmetry group is or as usual.The symmetry group contains inversion
if and only if
In logic and related fields such as mathematics and philosophy, "if and only if" (often shortened as "iff") is paraphrased by the biconditional, a logical connective between statements. The biconditional is true in two cases, where either bo ...
is odd.
The rotation group
In mathematics, the orthogonal group in dimension , denoted , is the group of distance-preserving transformations of a Euclidean space of dimension that preserve a fixed point, where the group operation is given by composing transformations. ...
is of order , except in the cases of:
*: the regular tetrahedron, which has the larger rotation group of order , which has only one subgroup ;
*: the regular octahedron, which has the larger rotation group of order , which has four versions of as subgroups.
If a right 2- or 3-antiprism is not uniform, then its rotation group is or as usual.The right -antiprisms have congruent regular -gon bases and congruent isosceles triangle side faces, thus have the same (dihedral) symmetry group as the uniform -antiprism, for .
Uniform antiprism
Auniform
A uniform is a variety of costume worn by members of an organization while usually participating in that organization's activity. Modern uniforms are most often worn by armed forces and paramilitary organizations such as police, emergency serv ...
-antiprism has two congruent
Congruence may refer to:
Mathematics
* Congruence (geometry), being the same size and shape
* Congruence or congruence relation, in abstract algebra, an equivalence relation on an algebraic structure that is compatible with the structure
* In modu ...
''regular'' -gons as base faces, and ''equilateral'' triangles as side faces. As do uniform prisms, the uniform antiprisms form an infinite class of vertex-transitive polyhedra. For , one has the digonal antiprism (degenerate antiprism), which is visually identical to the regular tetrahedron
In geometry, a tetrahedron (: tetrahedra or tetrahedrons), also known as a triangular pyramid, is a polyhedron composed of four triangular Face (geometry), faces, six straight Edge (geometry), edges, and four vertex (geometry), vertices. The tet ...
; for , the regular octahedron
In geometry, an octahedron (: octahedra or octahedrons) is any polyhedron with eight faces. One special case is the regular octahedron, a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex. Many types of i ...
is a ''triangular antiprism'' (non-degenerate antiprism).
The Schlegel diagram
In geometry, a Schlegel diagram is a projection of a polytope from \mathbb^d into \mathbb^ through a point just outside one of its facets. The resulting entity is a polytopal subdivision of the facet in \mathbb^ that, together with the ori ...
s of these semiregular antiprisms are as follows:
Cartesian coordinates
Cartesian coordinates
In geometry, a Cartesian coordinate system (, ) in a plane is a coordinate system that specifies each point uniquely by a pair of real numbers called ''coordinates'', which are the signed distances to the point from two fixed perpendicular o ...
for the vertices of a right
Rights are law, legal, social, or ethics, ethical principles of freedom or Entitlement (fair division), entitlement; that is, rights are the fundamental normative rules about what is allowed of people or owed to people according to some legal sy ...
-antiprism (i.e. with regular -gon bases and isosceles triangle side faces, circumradius of the bases equal to 1) are:
:
where ;
if the -antiprism is uniform (i.e. if the triangles are equilateral), then:
Volume and surface area
Let be the edge-length of auniform
A uniform is a variety of costume worn by members of an organization while usually participating in that organization's activity. Modern uniforms are most often worn by armed forces and paramilitary organizations such as police, emergency serv ...
-gonal antiprism; then the volume is:
and the surface area is:
Furthermore, the volume of a regular right -gonal antiprism with side length of its bases and height is given by:
Derivation
The circumradius of the horizontal circumcircle of the regular -gon at the base is : The vertices at the base are at : the vertices at the top are at : Via linear interpolation, points on the outer triangular edges of the antiprism that connect vertices at the bottom with vertices at the top are at : and at : By building the sums of the squares of the and coordinates in one of the previous two vectors, the squared circumradius of this section at altitude is : The horizontal section at altitude above the base is a -gon (truncated -gon) with sides of length alternating with sides of length . (These are derived from the length of the difference of the previous two vectors.) It can be dissected into isoceless triangles of edges and (semiperimeter ) plus isoceless triangles of edges and (semiperimeter ). According to Heron's formula the areas of these triangles are : and : The area of the section is