Quantum vibration on:
[Wikipedia]
[Google]
[Amazon]
 The quantum harmonic oscillator is the quantum-mechanical analog of the classical harmonic oscillator. Because an arbitrary smooth
The quantum harmonic oscillator is the quantum-mechanical analog of the classical harmonic oscillator. Because an arbitrary smooth
 The
The
The probability of each energy eigenstates can be calculated to find the energy distribution of the wavefunction: which corresponds to
 The Schrödinger equation for a particle in a spherically-symmetric three-dimensional harmonic oscillator can be solved explicitly by separation of variables. This procedure is analogous to the separation performed in the
The Schrödinger equation for a particle in a spherically-symmetric three-dimensional harmonic oscillator can be solved explicitly by separation of variables. This procedure is analogous to the separation performed in the
 We introduce, then, a set of "normal coordinates" , defined as the
We introduce, then, a set of "normal coordinates" , defined as the 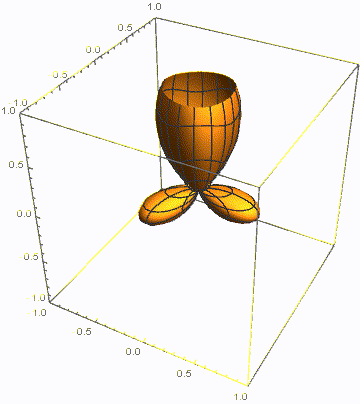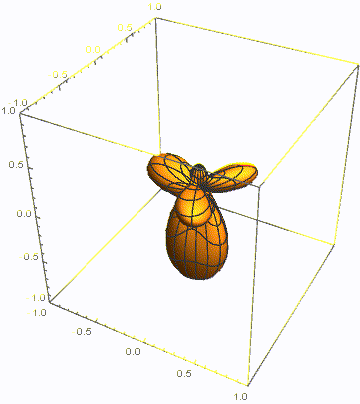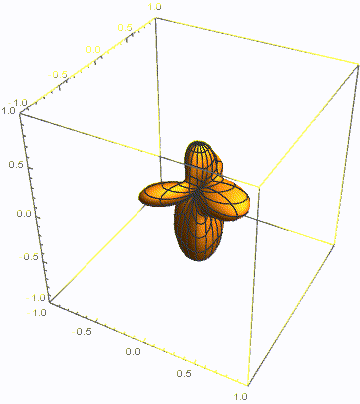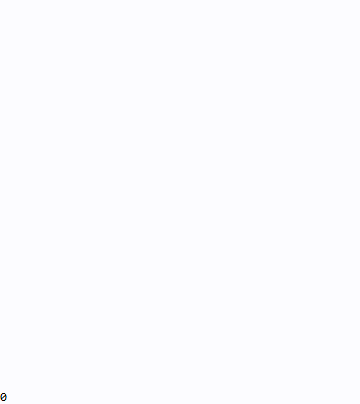 From the general result
it is easy to show, through elementary trigonometry, that the potential energy term is
where
The Hamiltonian may be written in wave vector space as
Note that the couplings between the position variables have been transformed away; if the s and s were
From the general result
it is easy to show, through elementary trigonometry, that the potential energy term is
where
The Hamiltonian may be written in wave vector space as
Note that the couplings between the position variables have been transformed away; if the s and s were
Quantum Harmonic Oscillator Live 3D intensity plots of quantum harmonic oscillator
Driven and damped quantum harmonic oscillator (lecture notes of course "quantum optics in electric circuits")
{{DEFAULTSORT:Quantum Harmonic Oscillator Quantum models Oscillators
 The quantum harmonic oscillator is the quantum-mechanical analog of the classical harmonic oscillator. Because an arbitrary smooth
The quantum harmonic oscillator is the quantum-mechanical analog of the classical harmonic oscillator. Because an arbitrary smooth potential
Potential generally refers to a currently unrealized ability. The term is used in a wide variety of fields, from physics to the social sciences to indicate things that are in a state where they are able to change in ways ranging from the simple r ...
can usually be approximated as a harmonic potential
The quantum harmonic oscillator is the quantum-mechanical analog of the classical harmonic oscillator. Because an arbitrary smooth potential can usually be approximated as a harmonic potential at the vicinity of a stable equilibrium point, ...
at the vicinity of a stable equilibrium point
In mathematics, specifically in differential equations, an equilibrium point is a constant solution to a differential equation.
Formal definition
The point \tilde\in \mathbb^n is an equilibrium point for the differential equation
:\frac = ...
, it is one of the most important model systems in quantum mechanics. Furthermore, it is one of the few quantum-mechanical systems for which an exact, analytical solution
In mathematics, an expression or equation is in closed form if it is formed with constants, variables, and a set of functions considered as ''basic'' and connected by arithmetic operations (, and integer powers) and function composition. C ...
is known.
One-dimensional harmonic oscillator
Hamiltonian and energy eigenstates
TheHamiltonian
Hamiltonian may refer to:
* Hamiltonian mechanics, a function that represents the total energy of a system
* Hamiltonian (quantum mechanics), an operator corresponding to the total energy of that system
** Dyall Hamiltonian, a modified Hamiltonian ...
of the particle is:
where is the particle's mass, is the force constant, is the angular frequency
In physics, angular frequency (symbol ''ω''), also called angular speed and angular rate, is a scalar measure of the angle rate (the angle per unit time) or the temporal rate of change of the phase argument of a sinusoidal waveform or sine ...
of the oscillator, is the position operator
In quantum mechanics, the position operator is the operator that corresponds to the position observable of a particle.
When the position operator is considered with a wide enough domain (e.g. the space of tempered distributions), its eigenvalues ...
(given by in the coordinate basis), and is the momentum operator
In quantum mechanics, the momentum operator is the operator associated with the linear momentum. The momentum operator is, in the position representation, an example of a differential operator. For the case of one particle in one spatial dimensio ...
(given by in the coordinate basis). The first term in the Hamiltonian represents the kinetic energy of the particle, and the second term represents its potential energy, as in Hooke's law
In physics, Hooke's law is an empirical law which states that the force () needed to extend or compress a spring by some distance () scales linearly with respect to that distance—that is, where is a constant factor characteristic of ...
.
The time-independent Schrödinger equation
The Schrödinger equation is a partial differential equation that governs the wave function of a non-relativistic quantum-mechanical system. Its discovery was a significant landmark in the development of quantum mechanics. It is named after E ...
(TISE) is,
where denotes a real number (which needs to be determined) that will specify a time-independent energy level
A quantum mechanics, quantum mechanical system or particle that is bound state, bound—that is, confined spatially—can only take on certain discrete values of energy, called energy levels. This contrasts with classical mechanics, classical pa ...
, or eigenvalue
In linear algebra, an eigenvector ( ) or characteristic vector is a vector that has its direction unchanged (or reversed) by a given linear transformation. More precisely, an eigenvector \mathbf v of a linear transformation T is scaled by a ...
, and the solution denotes that level's energy eigenstate
In quantum physics, a quantum state is a mathematical entity that embodies the knowledge of a quantum system. Quantum mechanics specifies the construction, evolution, and measurement of a quantum state. The result is a prediction for the system re ...
.
Then solve the differential equation representing this eigenvalue problem in the coordinate basis, for the wave function
In quantum physics, a wave function (or wavefunction) is a mathematical description of the quantum state of an isolated quantum system. The most common symbols for a wave function are the Greek letters and (lower-case and capital psi (letter) ...
, using a spectral method
Spectral methods are a class of techniques used in applied mathematics and scientific computing to numerically solve certain differential equations. The idea is to write the solution of the differential equation as a sum of certain " basis funct ...
. It turns out that there is a family of solutions. In this basis, they amount to Hermite functions,
The functions ''Hn'' are the physicists' Hermite polynomials
In mathematics, the Hermite polynomials are a classical orthogonal polynomial sequence.
The polynomials arise in:
* signal processing as Hermitian wavelets for wavelet transform analysis
* probability, such as the Edgeworth series, as well a ...
,
The corresponding energy levels are
The expectation values of position and momentum combined with variance of each variable can be derived from the wavefunction to understand the behavior of the energy eigenkets. They are shown to be and owing to the symmetry of the problem, whereas:
The variance in both position and momentum are observed to increase for higher energy levels. The lowest energy level has value of which is its minimum value due to uncertainty relation and also corresponds to a gaussian wavefunction.
This energy spectrum is noteworthy for three reasons. First, the energies are quantized, meaning that only discrete energy values (integer-plus-half multiples of ) are possible; this is a general feature of quantum-mechanical systems when a particle is confined. Second, these discrete energy levels are equally spaced, unlike in the Bohr model
In atomic physics, the Bohr model or Rutherford–Bohr model was a model of the atom that incorporated some early quantum concepts. Developed from 1911 to 1918 by Niels Bohr and building on Ernest Rutherford's nuclear Rutherford model, model, i ...
of the atom, or the particle in a box
In quantum mechanics, the particle in a box model (also known as the infinite potential well or the infinite square well) describes the movement of a free particle in a small space surrounded by impenetrable barriers. The model is mainly used a ...
. Third, the lowest achievable energy (the energy of the state, called the ground state
The ground state of a quantum-mechanical system is its stationary state of lowest energy; the energy of the ground state is known as the zero-point energy of the system. An excited state is any state with energy greater than the ground state ...
) is not equal to the minimum of the potential well, but above it; this is called zero-point energy
Zero-point energy (ZPE) is the lowest possible energy that a quantum mechanical system may have. Unlike in classical mechanics, quantum systems constantly Quantum fluctuation, fluctuate in their lowest energy state as described by the Heisen ...
. Because of the zero-point energy, the position and momentum of the oscillator in the ground state are not fixed (as they would be in a classical oscillator), but have a small range of variance, in accordance with the Heisenberg uncertainty principle
The uncertainty principle, also known as Heisenberg's indeterminacy principle, is a fundamental concept in quantum mechanics. It states that there is a limit to the precision with which certain pairs of physical properties, such as position a ...
.
The ground state probability density is concentrated at the origin, which means the particle spends most of its time at the bottom of the potential well, as one would expect for a state with little energy. As the energy increases, the probability density peaks at the classical "turning points", where the state's energy coincides with the potential energy. (See the discussion below of the highly excited states.) This is consistent with the classical harmonic oscillator, in which the particle spends more of its time (and is therefore more likely to be found) near the turning points, where it is moving the slowest. The correspondence principle
In physics, a correspondence principle is any one of several premises or assertions about the relationship between classical and quantum mechanics.
The physicist Niels Bohr coined the term in 1920 during the early development of quantum theory; ...
is thus satisfied. Moreover, special nondispersive wave packet
In physics, a wave packet (also known as a wave train or wave group) is a short burst of localized wave action that travels as a unit, outlined by an Envelope (waves), envelope. A wave packet can be analyzed into, or can be synthesized from, a ...
s, with minimum uncertainty, called coherent states
In physics, specifically in quantum mechanics, a coherent state is the specific quantum state of the quantum harmonic oscillator, often described as a state that has dynamics most closely resembling the oscillatory behavior of a classical harmo ...
oscillate very much like classical objects, as illustrated in the figure; they are ''not'' eigenstates of the Hamiltonian.
Ladder operator method
The "ladder operator
In linear algebra (and its application to quantum mechanics), a raising or lowering operator (collectively known as ladder operators) is an operator that increases or decreases the eigenvalue of another operator. In quantum mechanics, the raisin ...
" method, developed by Paul Dirac
Paul Adrien Maurice Dirac ( ; 8 August 1902 – 20 October 1984) was an English mathematician and Theoretical physics, theoretical physicist who is considered to be one of the founders of quantum mechanics. Dirac laid the foundations for bot ...
, allows extraction of the energy eigenvalues without directly solving the differential equation. It is generalizable to more complicated problems, notably in quantum field theory
In theoretical physics, quantum field theory (QFT) is a theoretical framework that combines Field theory (physics), field theory and the principle of relativity with ideas behind quantum mechanics. QFT is used in particle physics to construct phy ...
. Following this approach, we define the operators and its adjoint
In mathematics, the term ''adjoint'' applies in several situations. Several of these share a similar formalism: if ''A'' is adjoint to ''B'', then there is typically some formula of the type
:(''Ax'', ''y'') = (''x'', ''By'').
Specifically, adjoin ...
,
Note these operators classically are exactly the generators of normalized rotation in the phase space of and , ''i.e'' they describe the forwards and backwards evolution in time of a classical harmonic oscillator.
These operators lead to the following representation of and ,
The operator is not Hermitian {{Short description, none
Numerous things are named after the French mathematician Charles Hermite (1822–1901):
Hermite
* Cubic Hermite spline, a type of third-degree spline
* Gauss–Hermite quadrature, an extension of Gaussian quadrature me ...
, since itself and its adjoint are not equal. The energy eigenstates , when operated on by these ladder operators, give
From the relations above, we can also define a number operator , which has the following property:
The following commutator
In mathematics, the commutator gives an indication of the extent to which a certain binary operation fails to be commutative. There are different definitions used in group theory and ring theory.
Group theory
The commutator of two elements, ...
s can be easily obtained by substituting the canonical commutation relation
In quantum mechanics, the canonical commutation relation is the fundamental relation between canonical conjugate quantities (quantities which are related by definition such that one is the Fourier transform of another). For example,
hat x,\hat p ...
,
and the Hamilton operator can be expressed as
so the eigenstates of are also the eigenstates of energy.
To see that, we can apply to a number state :
Using the property of the number operator :
we get:
Thus, since solves the TISE for the Hamiltonian operator , is also one of its eigenstates with the corresponding eigenvalue:
QED.
The commutation property yields
and similarly,
This means that acts on to produce, up to a multiplicative constant, , and acts on to produce . For this reason, is called an annihilation operator ("lowering operator"), and a creation operator ("raising operator"). The two operators together are called ladder operator
In linear algebra (and its application to quantum mechanics), a raising or lowering operator (collectively known as ladder operators) is an operator that increases or decreases the eigenvalue of another operator. In quantum mechanics, the raisin ...
s.
Given any energy eigenstate, we can act on it with the lowering operator, , to produce another eigenstate with less energy. By repeated application of the lowering operator, it seems that we can produce energy eigenstates down to . However, since
the smallest eigenvalue of the number operator is 0, and
In this case, subsequent applications of the lowering operator will just produce zero, instead of additional energy eigenstates. Furthermore, we have shown above that
Finally, by acting on , 0⟩ with the raising operator and multiplying by suitable normalization factors, we can produce an infinite set of energy eigenstates
such that
which matches the energy spectrum given in the preceding section.
Arbitrary eigenstates can be expressed in terms of , 0⟩,
Analytical questions
The preceding analysis is algebraic, using only the commutation relations between the raising and lowering operators. Once the algebraic analysis is complete, one should turn to analytical questions. First, one should find the ground state, that is, the solution of the equation . In the position representation, this is the first-order differential equation whose solution is easily found to be theGaussian
Carl Friedrich Gauss (1777–1855) is the eponym of all of the topics listed below.
There are over 100 topics all named after this German mathematician and scientist, all in the fields of mathematics, physics, and astronomy. The English eponymo ...
The normalization constant is , and satisfies the normalization condition .
Conceptually, it is important that there is only one solution of this equation; if there were, say, two linearly independent ground states, we would get two independent chains of eigenvectors for the harmonic oscillator. Once the ground state is computed, one can show inductively that the excited states are Hermite polynomials times the Gaussian ground state, using the explicit form of the raising operator in the position representation. One can also prove that, as expected from the uniqueness of the ground state, the Hermite functions energy eigenstates constructed by the ladder method form a ''complete'' orthonormal set of functions.
Explicitly connecting with the previous section, the ground state , 0⟩ in the position representation is determined by ,
hence
so that , and so on.
Natural length and energy scales
The quantum harmonic oscillator possesses natural scales for length and energy, which can be used to simplify the problem. These can be found bynondimensionalization
Nondimensionalization is the partial or full removal of physical dimensions from an equation involving physical quantities by a suitable substitution of variables. This technique can simplify and parameterize problems where measured units are ...
.
The result is that, if ''energy'' is measured in units of and ''distance'' in units of , then the Hamiltonian simplifies to
while the energy eigenfunctions and eigenvalues simplify to Hermite functions and integers offset by a half,
where are the Hermite polynomials
In mathematics, the Hermite polynomials are a classical orthogonal polynomial sequence.
The polynomials arise in:
* signal processing as Hermitian wavelets for wavelet transform analysis
* probability, such as the Edgeworth series, as well a ...
.
To avoid confusion, these "natural units" will mostly not be adopted in this article. However, they frequently come in handy when performing calculations, by bypassing clutter.
For example, the fundamental solution
In mathematics, a fundamental solution for a linear partial differential operator is a formulation in the language of distribution theory of the older idea of a Green's function (although unlike Green's functions, fundamental solutions do not ...
(propagator
In quantum mechanics and quantum field theory, the propagator is a function that specifies the probability amplitude for a particle to travel from one place to another in a given period of time, or to travel with a certain energy and momentum. I ...
) of , the time-dependent Schrödinger operator for this oscillator, simply boils down to the Mehler kernel The Mehler kernel is a complex-valued function found to be the propagator of the quantum harmonic oscillator.
It was first discovered by Mehler in 1866, and since then, as Einar Hille remarked in 1932, "has been rediscovered by almost everybody ...
,
where . The most general solution for a given initial configuration then is simply
Coherent states
 The
The coherent states
In physics, specifically in quantum mechanics, a coherent state is the specific quantum state of the quantum harmonic oscillator, often described as a state that has dynamics most closely resembling the oscillatory behavior of a classical harmo ...
(also known as Glauber states) of the harmonic oscillator are special nondispersive wave packet
In physics, a wave packet (also known as a wave train or wave group) is a short burst of localized wave action that travels as a unit, outlined by an Envelope (waves), envelope. A wave packet can be analyzed into, or can be synthesized from, a ...
s, with minimum uncertainty , whose observable
In physics, an observable is a physical property or physical quantity that can be measured. In classical mechanics, an observable is a real-valued "function" on the set of all possible system states, e.g., position and momentum. In quantum ...
s' expectation values evolve like a classical system. They are eigenvectors of the annihilation operator, ''not'' the Hamiltonian, and form an overcomplete basis which consequentially lacks orthogonality.
The coherent states are indexed by and expressed in the basis as
Since coherent states are not energy eigenstates, their time evolution is not a simple shift in wavefunction phase. The time-evolved states are, however, also coherent states but with phase-shifting parameter instead: .
Because and via the Kermack-McCrae identity, the last form is equivalent to a unitary
Unitary may refer to:
Mathematics
* Unitary divisor
* Unitary element
* Unitary group
* Unitary matrix
* Unitary morphism
* Unitary operator
* Unitary transformation
* Unitary representation
* Unitarity (physics)
* ''E''-unitary inverse semigr ...
displacement operator acting on the ground state: . Calculating the expectation values:
where is the phase contributed by complex . These equations confirm the oscillating behavior of the particle.
The uncertainties calculated using the numeric method are:
which gives . Since the only wavefunction that can have lowest position-momentum uncertainty, , is a gaussian wavefunction, and since the coherent state wavefunction has minimum position-momentum uncertainty, we note that the general gaussian wavefunction in quantum mechanics has the form:Substituting the expectation values as a function of time, gives the required time varying wavefunction.The probability of each energy eigenstates can be calculated to find the energy distribution of the wavefunction: which corresponds to
Poisson distribution
In probability theory and statistics, the Poisson distribution () is a discrete probability distribution that expresses the probability of a given number of events occurring in a fixed interval of time if these events occur with a known const ...
.
Highly excited states
When is large, the eigenstates are localized into the classical allowed region, that is, the region in which a classical particle with energy can move. The eigenstates are peaked near the turning points: the points at the ends of the classically allowed region where the classical particle changes direction. This phenomenon can be verified through asymptotics of the Hermite polynomials, and also through theWKB approximation
In mathematical physics, the WKB approximation or WKB method is a technique for finding approximate solutions to Linear differential equation, linear differential equations with spatially varying coefficients. It is typically used for a Semiclass ...
.
The frequency of oscillation at is proportional to the momentum of a classical particle of energy and position . Furthermore, the square of the amplitude (determining the probability density) is ''inversely'' proportional to , reflecting the length of time the classical particle spends near . The system behavior in a small neighborhood of the turning point does not have a simple classical explanation, but can be modeled using an Airy function
In the physical sciences, the Airy function (or Airy function of the first kind) is a special function named after the British astronomer George Biddell Airy (1801–1892). The function Ai(''x'') and the related function Bi(''x''), are Linear in ...
. Using properties of the Airy function, one may estimate the probability of finding the particle outside the classically allowed region, to be approximately
This is also given, asymptotically, by the integral
Phase space solutions
In the phase space formulation of quantum mechanics, eigenstates of the quantum harmonic oscillator in several different representations of thequasiprobability distribution
A quasiprobability distribution is a mathematical object similar to a probability distribution but which relaxes some of Kolmogorov's axioms of probability theory. Quasiprobability distributions arise naturally in the study of quantum mechanics ...
can be written in closed form. The most widely used of these is for the Wigner quasiprobability distribution.
The Wigner quasiprobability distribution for the energy eigenstate is, in the natural units described above,
where ''Ln'' are the Laguerre polynomials
In mathematics, the Laguerre polynomials, named after Edmond Laguerre (1834–1886), are nontrivial solutions of Laguerre's differential equation:
xy'' + (1 - x)y' + ny = 0,\
y = y(x)
which is a second-order linear differential equation. Thi ...
. This example illustrates how the Hermite and Laguerre polynomials are linked through the Wigner map.
Meanwhile, the Husimi Q function of the harmonic oscillator eigenstates have an even simpler form. If we work in the natural units described above, we have
This claim can be verified using the Segal–Bargmann transform. Specifically, since the raising operator in the Segal–Bargmann representation is simply multiplication by and the ground state is the constant function 1, the normalized harmonic oscillator states in this representation are simply . At this point, we can appeal to the formula for the Husimi Q function in terms of the Segal–Bargmann transform.
''N''-dimensional isotropic harmonic oscillator
The one-dimensional harmonic oscillator is readily generalizable to dimensions, where . In one dimension, the position of the particle was specified by a singlecoordinate
In geometry, a coordinate system is a system that uses one or more numbers, or coordinates, to uniquely determine and standardize the position of the points or other geometric elements on a manifold such as Euclidean space. The coordinates are ...
, . In dimensions, this is replaced by position coordinates, which we label . Corresponding to each position coordinate is a momentum; we label these . The canonical commutation relations
In quantum mechanics, the canonical commutation relation is the fundamental relation between canonical conjugate quantities (quantities which are related by definition such that one is the Fourier transform of another). For example,
[\hat x,\hat p ...
between these operators are
The Hamiltonian for this system is
As the form of this Hamiltonian makes clear, the -dimensional harmonic oscillator is exactly analogous to independent one-dimensional harmonic oscillators with the same mass and spring constant. In this case, the quantities would refer to the positions of each of the particles. This is a convenient property of the potential, which allows the potential energy to be separated into terms depending on one coordinate each.
This observation makes the solution straightforward. For a particular set of quantum numbers the energy eigenfunctions for the -dimensional oscillator are expressed in terms of the 1-dimensional eigenfunctions as:
In the ladder operator method, we define sets of ladder operators,
By an analogous procedure to the one-dimensional case, we can then show that each of the and operators lower and raise the energy by respectively. The Hamiltonian is
This Hamiltonian is invariant under the dynamic symmetry group (the unitary group in dimensions), defined by
where is an element in the defining matrix representation of .
The energy levels of the system are
As in the one-dimensional case, the energy is quantized. The ground state energy is times the one-dimensional ground energy, as we would expect using the analogy to independent one-dimensional oscillators. There is one further difference: in the one-dimensional case, each energy level corresponds to a unique quantum state. In -dimensions, except for the ground state, the energy levels are ''degenerate'', meaning there are several states with the same energy.
The degeneracy can be calculated relatively easily. As an example, consider the 3-dimensional case: Define . All states with the same will have the same energy. For a given , we choose a particular . Then . There are possible pairs . can take on the values to , and for each the value of is fixed. The degree of degeneracy therefore is:
Formula for general and being the dimension of the symmetric irreducible -th power representation of the unitary group
The special case = 3, given above, follows directly from this general equation. This is however, only true for distinguishable particles, or one particle in dimensions (as dimensions are distinguishable). For the case of bosons in a one-dimension harmonic trap, the degeneracy scales as the number of ways to partition an integer using integers less than or equal to .
This arises due to the constraint of putting quanta into a state ket where and , which are the same constraints as in integer partition.
Example: 3D isotropic harmonic oscillator
 The Schrödinger equation for a particle in a spherically-symmetric three-dimensional harmonic oscillator can be solved explicitly by separation of variables. This procedure is analogous to the separation performed in the
The Schrödinger equation for a particle in a spherically-symmetric three-dimensional harmonic oscillator can be solved explicitly by separation of variables. This procedure is analogous to the separation performed in the hydrogen-like atom
A hydrogen-like atom (or hydrogenic atom) is any atom or ion with a single valence electron. These atoms are isoelectronic with hydrogen. Examples of hydrogen-like atoms include, but are not limited to, hydrogen itself, all alkali metals such as ...
problem, but with a different spherically symmetric potential
where is the mass of the particle. Because will be used below for the magnetic quantum number, mass is indicated by , instead of , as earlier in this article.
The solution to the equation is:
where
: is a normalization constant; ;
:
are generalized Laguerre polynomials; The order of the polynomial is a non-negative integer;
* is a spherical harmonic function;
* is the reduced Planck constant
The Planck constant, or Planck's constant, denoted by h, is a fundamental physical constant of foundational importance in quantum mechanics: a photon's energy is equal to its frequency multiplied by the Planck constant, and the wavelength of a ...
:
The energy eigenvalue is
The energy is usually described by the single quantum number
In quantum physics and chemistry, quantum numbers are quantities that characterize the possible states of the system.
To fully specify the state of the electron in a hydrogen atom, four quantum numbers are needed. The traditional set of quantu ...
Because is a non-negative integer, for every even we have and for every odd we have . The magnetic quantum number is an integer satisfying , so for every and ''ℓ'' there are 2''ℓ'' + 1 different quantum state
In quantum physics, a quantum state is a mathematical entity that embodies the knowledge of a quantum system. Quantum mechanics specifies the construction, evolution, and measurement of a quantum state. The result is a prediction for the system ...
s, labeled by . Thus, the degeneracy at level is
where the sum starts from 0 or 1, according to whether is even or odd.
This result is in accordance with the dimension formula above, and amounts to the dimensionality of a symmetric representation of , the relevant degeneracy group.
Applications
Harmonic oscillators lattice: phonons
The notation of a harmonic oscillator can be extended to a one-dimensional lattice of many particles. Consider a one-dimensional quantum mechanical ''harmonic chain'' of ''N'' identical atoms. This is the simplest quantum mechanical model of a lattice, and we will see howphonon
A phonon is a collective excitation in a periodic, elastic arrangement of atoms or molecules in condensed matter, specifically in solids and some liquids. In the context of optically trapped objects, the quantized vibration mode can be defined a ...
s arise from it. The formalism that we will develop for this model is readily generalizable to two and three dimensions.
As in the previous section, we denote the positions of the masses by , as measured from their equilibrium positions (i.e. if the particle is at its equilibrium position). In two or more dimensions, the are vector quantities. The Hamiltonian
Hamiltonian may refer to:
* Hamiltonian mechanics, a function that represents the total energy of a system
* Hamiltonian (quantum mechanics), an operator corresponding to the total energy of that system
** Dyall Hamiltonian, a modified Hamiltonian ...
for this system is
where is the (assumed uniform) mass of each atom, and and are the position and momentum
In Newtonian mechanics, momentum (: momenta or momentums; more specifically linear momentum or translational momentum) is the product of the mass and velocity of an object. It is a vector quantity, possessing a magnitude and a direction. ...
operators for the ''i'' th atom and the sum is made over the nearest neighbors (nn). However, it is customary to rewrite the Hamiltonian in terms of the normal modes
A normal mode of a dynamical system is a pattern of motion in which all parts of the system move sinusoidally with the same frequency and with a fixed phase relation. The free motion described by the normal modes takes place at fixed frequencies. ...
of the wavevector
In physics, a wave vector (or wavevector) is a vector used in describing a wave, with a typical unit being cycle per metre. It has a magnitude and direction. Its magnitude is the wavenumber of the wave (inversely proportional to the wavelength), ...
rather than in terms of the particle coordinates so that one can work in the more convenient Fourier space.
 We introduce, then, a set of "normal coordinates" , defined as the
We introduce, then, a set of "normal coordinates" , defined as the discrete Fourier transform
In mathematics, the discrete Fourier transform (DFT) converts a finite sequence of equally-spaced Sampling (signal processing), samples of a function (mathematics), function into a same-length sequence of equally-spaced samples of the discre ...
s of the s, and "conjugate momenta" defined as the Fourier transforms of the s,
The quantity will turn out to be the wave number
In the physical sciences, the wavenumber (or wave number), also known as repetency, is the spatial frequency of a wave. Ordinary wavenumber is defined as the number of wave cycles divided by length; it is a physical quantity with dimension of r ...
of the phonon, i.e. 2''π'' divided by the wavelength
In physics and mathematics, wavelength or spatial period of a wave or periodic function is the distance over which the wave's shape repeats.
In other words, it is the distance between consecutive corresponding points of the same ''phase (waves ...
. It takes on quantized values, because the number of atoms is finite.
This preserves the desired commutation relations in either real space or wave vector space
 From the general result
it is easy to show, through elementary trigonometry, that the potential energy term is
where
The Hamiltonian may be written in wave vector space as
Note that the couplings between the position variables have been transformed away; if the s and s were
From the general result
it is easy to show, through elementary trigonometry, that the potential energy term is
where
The Hamiltonian may be written in wave vector space as
Note that the couplings between the position variables have been transformed away; if the s and s were hermitian {{Short description, none
Numerous things are named after the French mathematician Charles Hermite (1822–1901):
Hermite
* Cubic Hermite spline, a type of third-degree spline
* Gauss–Hermite quadrature, an extension of Gaussian quadrature me ...
(which they are not), the transformed Hamiltonian would describe ''uncoupled'' harmonic oscillators.
The form of the quantization depends on the choice of boundary conditions; for simplicity, we impose ''periodic'' boundary conditions, defining the -th atom as equivalent to the first atom. Physically, this corresponds to joining the chain at its ends. The resulting quantization is
The upper bound to comes from the minimum wavelength, which is twice the lattice spacing , as discussed above.
The harmonic oscillator eigenvalues or energy levels for the mode are
If we ignore the zero-point energy
Zero-point energy (ZPE) is the lowest possible energy that a quantum mechanical system may have. Unlike in classical mechanics, quantum systems constantly Quantum fluctuation, fluctuate in their lowest energy state as described by the Heisen ...
then the levels are evenly spaced at
So an exact amount of energy
Energy () is the physical quantity, quantitative physical property, property that is transferred to a physical body, body or to a physical system, recognizable in the performance of Work (thermodynamics), work and in the form of heat and l ...
, must be supplied to the harmonic oscillator lattice to push it to the next energy level. In analogy to the photon
A photon () is an elementary particle that is a quantum of the electromagnetic field, including electromagnetic radiation such as light and radio waves, and the force carrier for the electromagnetic force. Photons are massless particles that can ...
case when the electromagnetic field
An electromagnetic field (also EM field) is a physical field, varying in space and time, that represents the electric and magnetic influences generated by and acting upon electric charges. The field at any point in space and time can be regarde ...
is quantised, the quantum of vibrational energy is called a phonon
A phonon is a collective excitation in a periodic, elastic arrangement of atoms or molecules in condensed matter, specifically in solids and some liquids. In the context of optically trapped objects, the quantized vibration mode can be defined a ...
.
All quantum systems show wave-like and particle-like properties. The particle-like properties of the phonon are best understood using the methods of second quantization
Second quantization, also referred to as occupation number representation, is a formalism used to describe and analyze quantum many-body systems. In quantum field theory, it is known as canonical quantization, in which the fields (typically as ...
and operator techniques described elsewhere.
In the continuum limit
In mathematical physics and mathematics, the continuum limit or scaling limit of a lattice model characterizes its behaviour in the limit as the lattice spacing goes to zero. It is often useful to use lattice models to approximate real-world pr ...
, , , while is held fixed. The canonical coordinates devolve to the decoupled momentum modes of a scalar field, , whilst the location index (''not the displacement dynamical variable'') becomes the parameter argument of the scalar field, .
Molecular vibrations
* The vibrations of adiatomic molecule
Diatomic molecules () are molecules composed of only two atoms, of the same or different chemical elements. If a diatomic molecule consists of two atoms of the same element, such as hydrogen () or oxygen (), then it is said to be homonuclear mol ...
are an example of a two-body version of the quantum harmonic oscillator. In this case, the angular frequency is given by where is the reduced mass
In physics, reduced mass is a measure of the effective inertial mass of a system with two or more particles when the particles are interacting with each other. Reduced mass allows the two-body problem to be solved as if it were a one-body probl ...
and and are the masses of the two atoms.
* The Hooke's atom is a simple model of the helium
Helium (from ) is a chemical element; it has chemical symbol, symbol He and atomic number 2. It is a colorless, odorless, non-toxic, inert gas, inert, monatomic gas and the first in the noble gas group in the periodic table. Its boiling point is ...
atom using the quantum harmonic oscillator.
* Modelling phonons, as discussed above.
* A charge with mass in a uniform magnetic field is an example of a one-dimensional quantum harmonic oscillator: Landau quantization
In quantum mechanics, the energies of Cyclotron motion#Cyclotron resonance, cyclotron orbits of charged particles in a uniform magnetic field are quantized to discrete values, thus known as Landau levels. These levels are Degenerate energy level, d ...
.
See also
* * * * * * * * *Notes
References
Bibliography
* * *External links
Quantum Harmonic Oscillator
Driven and damped quantum harmonic oscillator (lecture notes of course "quantum optics in electric circuits")
{{DEFAULTSORT:Quantum Harmonic Oscillator Quantum models Oscillators