Polynomials Calculating Sums Of Powers Of Arithmetic Progressions on:
[Wikipedia]
[Google]
[Amazon]
In
Correct version.
 In 1713,
In 1713,
 For ''p'' = 1, it is clear that
For ''p'' = 3, the result that
is known as
For ''p'' = 1, it is clear that
For ''p'' = 3, the result that
is known as
Lester R. Ford Award
* *{{Cite news , last=Orosi , first=Greg , year=2018 , title=A Simple Derivation Of Faulhaber's Formula , url=http://www.math.nthu.edu.tw/~amen/2018/AMEN-170803.pdf , periodical = Applied Mathematics E-Notes , volume =18 , pages =124–126
A visual proof for the sum of squares and cubes
Finite differences
mathematics
Mathematics is a field of study that discovers and organizes methods, Mathematical theory, theories and theorems that are developed and Mathematical proof, proved for the needs of empirical sciences and mathematics itself. There are many ar ...
, Faulhaber's formula, named after the early 17th century mathematician Johann Faulhaber
Johann Faulhaber (5 May 1580 – 10 September 1635) was a German mathematician, specifically, a calculator ('':de:Rechenmeister, Rechenmeister'').
Biography
Born in Ulm, Faulhaber was a trained weaver who later took the role of a surveyor of th ...
, expresses the sum of the ''p''-th powers of the first ''n'' positive integers
as a polynomial in ''n''. In modern notation, Faulhaber's formula is
Here, is the binomial coefficient
In mathematics, the binomial coefficients are the positive integers that occur as coefficients in the binomial theorem. Commonly, a binomial coefficient is indexed by a pair of integers and is written \tbinom. It is the coefficient of the t ...
"''p'' + 1 choose ''r''", and the ''Bj'' are the Bernoulli numbers
In mathematics, the Bernoulli numbers are a sequence of rational numbers which occur frequently in analysis. The Bernoulli numbers appear in (and can be defined by) the Taylor series expansions of the tangent and hyperbolic tangent functions, ...
with the convention that .
The result: Faulhaber's formula
Faulhaber's formula concerns expressing the sum of the ''p''-th powers of the first ''n'' positive integers as a (''p'' + 1)th-degreepolynomial
In mathematics, a polynomial is a Expression (mathematics), mathematical expression consisting of indeterminate (variable), indeterminates (also called variable (mathematics), variables) and coefficients, that involves only the operations of addit ...
function of ''n''.
The first few examples are well known. For ''p'' = 0, we have
For ''p'' = 1, we have the triangular number
A triangular number or triangle number counts objects arranged in an equilateral triangle. Triangular numbers are a type of figurate number, other examples being square numbers and cube numbers. The th triangular number is the number of dots in ...
s
For ''p'' = 2, we have the square pyramidal number
In mathematics, a pyramid number, or square pyramidal number, is a natural number that counts the stacked spheres in a pyramid (geometry), pyramid with a square base. The study of these numbers goes back to Archimedes and Fibonacci. They are part ...
s
The coefficients of Faulhaber's formula in its general form involve the Bernoulli number
In mathematics, the Bernoulli numbers are a sequence of rational numbers which occur frequently in analysis. The Bernoulli numbers appear in (and can be defined by) the Taylor series expansions of the tangent and hyperbolic tangent function ...
s ''Bj''. The Bernoulli numbers begin
where here we use the convention that . The Bernoulli numbers have various definitions (see Bernoulli number#Definitions), such as that they are the coefficients of the exponential generating function
Then Faulhaber's formula is that
Here, the ''Bj'' are the Bernoulli numbers
In mathematics, the Bernoulli numbers are a sequence of rational numbers which occur frequently in analysis. The Bernoulli numbers appear in (and can be defined by) the Taylor series expansions of the tangent and hyperbolic tangent functions, ...
as above, and
is the binomial coefficient
In mathematics, the binomial coefficients are the positive integers that occur as coefficients in the binomial theorem. Commonly, a binomial coefficient is indexed by a pair of integers and is written \tbinom. It is the coefficient of the t ...
"''p'' + 1 choose ''k''".
Examples
So, for example, one has for , The first seven examples of Faulhaber's formula areHistory
Ancient period
The history of the problem begins in antiquity, its special cases arising as solutions to related inquiries. The case coincides historically with the problem of calculating the sum of the first terms of anarithmetic progression
An arithmetic progression or arithmetic sequence is a sequence of numbers such that the difference from any succeeding term to its preceding term remains constant throughout the sequence. The constant difference is called common difference of that ...
. In chronological order, early discoveries include:
:, a formula known by the Pythagorean school
Pythagorean, meaning of or pertaining to the ancient Ionian mathematician, philosopher, and music theorist Pythagoras, may refer to:
Philosophy
* Pythagoreanism, the esoteric and metaphysical beliefs purported to have been held by Pythagoras
* N ...
for its connection with triangular numbers
A triangular number or triangle number counts objects arranged in an equilateral triangle. Triangular numbers are a type of figurate number, other examples being square numbers and cube numbers. The th triangular number is the number of dots in ...
.
: a result showing that the sum of the first positive odd numbers is a perfect square. This formula was likely also known to the Pythagoreans, who in constructing figurate numbers
The term figurate number is used by different writers for members of different sets of numbers, generalizing from triangular numbers to different shapes (polygonal numbers) and different dimensions (polyhedral numbers). The ancient Greek mathemat ...
realized that the gnomon
A gnomon (; ) is the part of a sundial that casts a shadow. The term is used for a variety of purposes in mathematics and other fields, typically to measure directions, position, or time.
History
A painted stick dating from 2300 BC that was ...
of the th perfect square is precisely the th odd number.
: a formula that calculates the sum of the squares of the first positive integers, as demonstrated in ''Spirals
In mathematics, a spiral is a curve which emanates from a point, moving further away as it revolves around the point. It is a subtype of whorled patterns, a broad group that also includes concentric objects.
Two-dimensional
A two-dimension ...
'', a work of Archimedes
Archimedes of Syracuse ( ; ) was an Ancient Greece, Ancient Greek Greek mathematics, mathematician, physicist, engineer, astronomer, and Invention, inventor from the ancient city of Syracuse, Sicily, Syracuse in History of Greek and Hellenis ...
.
: a formula that calculates the sum of the cubes of the first positive integers, discovered as a corollary of a theorem of Nicomachus of Gerasa
Nicomachus of Gerasa (; ) was an Ancient Greek Neopythagorean philosopher from Gerasa, in the Roman province of Syria (now Jerash, Jordan). Like many Pythagoreans, Nicomachus wrote about the mystical properties of numbers, best known for his ...
.
Middle period
Over time, many other mathematicians became interested in the problem and made various contributions to its solution. These includeAryabhata
Aryabhata ( ISO: ) or Aryabhata I (476–550 CE) was the first of the major mathematician-astronomers from the classical age of Indian mathematics and Indian astronomy. His works include the '' Āryabhaṭīya'' (which mentions that in 3600 ' ...
, Al-Karaji
(; c. 953 – c. 1029) was a 10th-century Persian mathematician and engineer who flourished at Baghdad. He was born in Karaj, a city near Tehran. His three principal surviving works are mathematical: ''Al-Badi' fi'l-hisab'' (''Wonderful on ...
, Ibn al-Haytham
Ḥasan Ibn al-Haytham (Latinization of names, Latinized as Alhazen; ; full name ; ) was a medieval Mathematics in medieval Islam, mathematician, Astronomy in the medieval Islamic world, astronomer, and Physics in the medieval Islamic world, p ...
, Thomas Harriot
Thomas Harriot (; – 2 July 1621), also spelled Harriott, Hariot or Heriot, was an English astronomer, mathematician, ethnographer and translator to whom the theory of refraction is attributed. Thomas Harriot was also recognized for his con ...
, Johann Faulhaber
Johann Faulhaber (5 May 1580 – 10 September 1635) was a German mathematician, specifically, a calculator ('':de:Rechenmeister, Rechenmeister'').
Biography
Born in Ulm, Faulhaber was a trained weaver who later took the role of a surveyor of th ...
, Pierre de Fermat
Pierre de Fermat (; ; 17 August 1601 – 12 January 1665) was a French mathematician who is given credit for early developments that led to infinitesimal calculus, including his technique of adequality. In particular, he is recognized for his d ...
and Blaise Pascal
Blaise Pascal (19June 162319August 1662) was a French mathematician, physicist, inventor, philosopher, and Catholic Church, Catholic writer.
Pascal was a child prodigy who was educated by his father, a tax collector in Rouen. His earliest ...
who recursively solved the problem of the sum of powers of successive integers by considering an identity that allowed to obtain a polynomial of degree already knowing the previous ones.
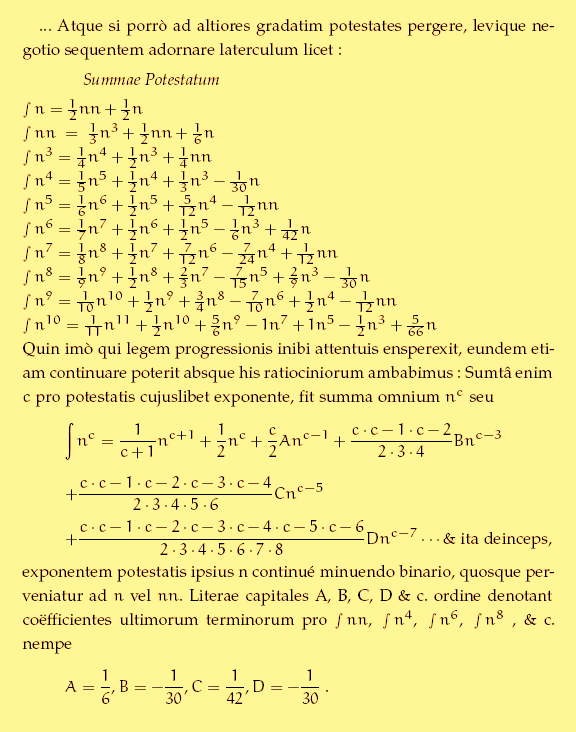
Faulhaber's formula is also called Bernoulli's formula. Faulhaber did not know the properties of the coefficients later discovered by Bernoulli. Rather, he knew at least the first 17 cases, as well as the existence of the Faulhaber polynomials for odd powers described below. The arxiv.org paper has a misprint in the formula for the sum of 11th powers, which was corrected in the printed versionCorrect version.
 In 1713,
In 1713, Jacob Bernoulli
Jacob Bernoulli (also known as James in English or Jacques in French; – 16 August 1705) was a Swiss mathematician. He sided with Gottfried Wilhelm Leibniz during the Leibniz–Newton calculus controversy and was an early proponent of Leibniz ...
published under the title ''Summae Potestatum'' an expression of the sum of the powers of the first integers as a ()th-degree polynomial function
In mathematics, a polynomial is a mathematical expression consisting of indeterminates (also called variables) and coefficients, that involves only the operations of addition, subtraction, multiplication and exponentiation to nonnegative int ...
of , with coefficients involving numbers , now called Bernoulli number
In mathematics, the Bernoulli numbers are a sequence of rational numbers which occur frequently in analysis. The Bernoulli numbers appear in (and can be defined by) the Taylor series expansions of the tangent and hyperbolic tangent function ...
s:
:
Introducing also the first two Bernoulli numbers (which Bernoulli did not), the previous formula becomes
using the Bernoulli number of the second kind for which , or
using the Bernoulli number of the first kind for which
A rigorous proof of these formulas and Faulhaber's assertion that such formulas would exist for all odd powers took until , two centuries later. Jacobi benefited from the progress of mathematical analysis using the development in infinite series of an exponential function generating Bernoulli numbers
In mathematics, the Bernoulli numbers are a sequence of rational numbers which occur frequently in analysis. The Bernoulli numbers appear in (and can be defined by) the Taylor series expansions of the tangent and hyperbolic tangent functions, ...
.
Modern period
In 1982 A.W.F. Edwards publishes an article in which he shows that Pascal's identity can be expressed by means of triangular matrices containing thePascal's triangle
In mathematics, Pascal's triangle is an infinite triangular array of the binomial coefficients which play a crucial role in probability theory, combinatorics, and algebra. In much of the Western world, it is named after the French mathematician Bla ...
deprived of 'last element of each line:
:
The example is limited by the choice of a fifth order matrix but is easily extendable to higher orders. The equation can be written as: and multiplying the two sides of the equation to the left by , inverse of the matrix A, we obtain which allows to arrive directly at the polynomial coefficients without directly using the Bernoulli numbers. Other authors after Edwards dealing with various aspects of the power sum problem take the matrix path and studying aspects of the problem in their articles useful tools such as the Vandermonde vector. Other researchers continue to explore through the traditional analytic route and generalize the problem of the sum of successive integers to any geometric progression
Proof with exponential generating function
Let denote the sum under consideration for integer Define the following exponentialgenerating function
In mathematics, a generating function is a representation of an infinite sequence of numbers as the coefficients of a formal power series. Generating functions are often expressed in closed form (rather than as a series), by some expression invo ...
with (initially) indeterminate
We find
This is an entire function in so that can be taken to be any complex number.
We next recall the exponential generating function for the Bernoulli polynomials
In mathematics, the Bernoulli polynomials, named after Jacob Bernoulli, combine the Bernoulli numbers and binomial coefficients. They are used for series expansion of functions, and with the Euler–MacLaurin formula.
These polynomials occur ...
where denotes the Bernoulli number with the convention . This may be converted to a generating function with the convention by the addition of to the coefficient of in each , see Bernoulli polynomials#Explicit formula for example. does not need to be changed.
so that
It follows that
for all .
Faulhaber polynomials
The term Faulhaber polynomials is used by some authors to refer to another polynomial sequence related to that given above. Write Faulhaber observed that if ''p'' is odd then is a polynomial function of ''a''.Nicomachus's theorem
In number theory, the sum of the first cubes is the square of the th triangular number. That is,
:1^3+2^3+3^3+\cdots+n^3 = \left(1+2+3+\cdots+n\right)^2.
The same equation may be written more compactly using the mathematical notation for summa ...
.
Further, we have
(see , , , , ).
More generally,
Some authors call the polynomials in ''a'' on the right-hand sides of these identities ''Faulhaber polynomials''. These polynomials are divisible by because the Bernoulli number
In mathematics, the Bernoulli numbers are a sequence of rational numbers which occur frequently in analysis. The Bernoulli numbers appear in (and can be defined by) the Taylor series expansions of the tangent and hyperbolic tangent function ...
is 0 for odd .
Inversely, writing for simplicity , we have
and generally
Faulhaber also knew that if a sum for an odd power is given by
then the sum for the even power just below is given by
Note that the polynomial in parentheses is the derivative of the polynomial above with respect to ''a''.
Since ''a'' = ''n''(''n'' + 1)/2, these formulae show that for an odd power (greater than 1), the sum is a polynomial in ''n'' having factors ''n''2 and (''n'' + 1)2, while for an even power the polynomial has factors ''n'', ''n'' + 1/2 and ''n'' + 1.
Expressing products of power sums as linear combinations of power sums
Products of two (and thus by iteration, several) power sums can be written as linear combinations of power sums with either all degrees even or all degrees odd, depending on the total degree of the product as a polynomial in , e.g. . Note that the sums of coefficients must be equal on both sides, as can be seen by putting , which makes all the equal to 1. Some general formulae include: Note that in the second formula, for even the term corresponding to is different from the other terms in the sum, while for odd , this additional term vanishes because of .Matrix form
Faulhaber's formula can also be written in a form usingmatrix multiplication
In mathematics, specifically in linear algebra, matrix multiplication is a binary operation that produces a matrix (mathematics), matrix from two matrices. For matrix multiplication, the number of columns in the first matrix must be equal to the n ...
.
Take the first seven examples
Writing these polynomials as a product between matrices gives
where
Surprisingly, inverting the matrix of polynomial coefficients yields something more familiar:
In the inverted matrix, Pascal's triangle
In mathematics, Pascal's triangle is an infinite triangular array of the binomial coefficients which play a crucial role in probability theory, combinatorics, and algebra. In much of the Western world, it is named after the French mathematician Bla ...
can be recognized, without the last element of each row, and with alternating signs.
Let be the matrix obtained from by changing the signs of the entries in odd diagonals, that is by replacing by , let be the matrix obtained from with a similar transformation, then
and
Also
This is because it is evident that
and that therefore polynomials of degree of the form subtracted the monomial difference they become .
This is true for every order, that is, for each positive integer , one has and
Thus, it is possible to obtain the coefficients of the polynomials of the sums of powers of successive integers without resorting to the numbers of Bernoulli but by inverting the matrix easily obtained from the triangle of Pascal.
Variations
* Replacing with , we find the alternative expression: * Subtracting from both sides of the original formula and incrementing by , we get : where can be interpreted as "negative" Bernoulli numbers with . * We may also expand in terms of the Bernoulli polynomials to find which implies Since whenever is odd, the factor may be removed when . * It can also be expressed in terms ofStirling numbers of the second kind
In mathematics, particularly in combinatorics, a Stirling number of the second kind (or Stirling partition number) is the number of ways to partition a set of ''n'' objects into ''k'' non-empty subsets and is denoted by S(n,k) or \textstyle \lef ...
and falling factorials as This is due to the definition of the Stirling numbers of the second kind as monomial
In mathematics, a monomial is, roughly speaking, a polynomial which has only one term. Two definitions of a monomial may be encountered:
# A monomial, also called a power product or primitive monomial, is a product of powers of variables with n ...
s in terms of falling factorials, and the behaviour of falling factorials under the indefinite sum In discrete calculus the indefinite sum operator (also known as the antidifference operator), denoted by \sum _x or \Delta^ , is the linear operator, inverse of the forward difference operator \Delta . It relates to the forward difference operato ...
.
Interpreting the Stirling numbers of the second kind, , as the number of set partitions of into parts, the identity has a direct combinatorial proof since both sides count the number of functions with maximal. The index of summation on the left hand side represents , while the index on the right hand side is represents the number of elements in the image of f.
*There is also a similar (but somehow simpler) expression: using the idea of telescoping and the binomial theorem
In elementary algebra, the binomial theorem (or binomial expansion) describes the algebraic expansion of powers of a binomial. According to the theorem, the power expands into a polynomial with terms of the form , where the exponents and a ...
, one gets '' Pascal's identity'':
:This in particular yields the examples below – e.g., take to get the first example. In a similar fashion we also find
* A generalized expression involving the Eulerian number
In combinatorics, the Eulerian number A(n,k) is the number of permutations of the numbers 1 to ''n'' in which exactly ''k'' elements are greater than the previous element (permutations with ''k'' "ascents").
Leonhard Euler investigated them and ...
s is
:.
* Faulhaber's formula was generalized by Guo and Zeng to a -analog.
Relationship to Riemann zeta function
Using , one can write If we consider the generating function in the large limit for , then we find Heuristically, this suggests that This result agrees with the value of theRiemann zeta function
The Riemann zeta function or Euler–Riemann zeta function, denoted by the Greek letter (zeta), is a mathematical function of a complex variable defined as \zeta(s) = \sum_^\infty \frac = \frac + \frac + \frac + \cdots for and its analytic c ...
for negative integers on appropriately analytically continuing .
Faulhaber's formula can be written in terms of the Hurwitz zeta function
In mathematics, the Hurwitz zeta function is one of the many zeta functions. It is formally defined for complex variables with and by
:\zeta(s,a) = \sum_^\infty \frac.
This series is absolutely convergent for the given values of and and c ...
:
Umbral form
In theumbral calculus
The term umbral calculus has two related but distinct meanings.
In mathematics, before the 1970s, umbral calculus referred to the surprising similarity between seemingly unrelated polynomial equations and certain shadowy techniques used to prove ...
, one treats the Bernoulli numbers , , , ... ''as if'' the index ''j'' in were actually an exponent, and so ''as if'' the Bernoulli numbers were powers of some object ''B''.
Using this notation, Faulhaber's formula can be written as
Here, the expression on the right must be understood by expanding out to get terms that can then be interpreted as the Bernoulli numbers. Specifically, using the binomial theorem
In elementary algebra, the binomial theorem (or binomial expansion) describes the algebraic expansion of powers of a binomial. According to the theorem, the power expands into a polynomial with terms of the form , where the exponents and a ...
, we get
A derivation of Faulhaber's formula using the umbral form is available in ''The Book of Numbers'' by John Horton Conway
John Horton Conway (26 December 1937 – 11 April 2020) was an English mathematician. He was active in the theory of finite groups, knot theory, number theory, combinatorial game theory and coding theory. He also made contributions to many b ...
and Richard K. Guy.
Classically, this umbral form was considered as a notational convenience. In the modern umbral calculus, on the other hand, this is given a formal mathematical underpinning. One considers the linear functional
In mathematics, a linear form (also known as a linear functional, a one-form, or a covector) is a linear mapIn some texts the roles are reversed and vectors are defined as linear maps from covectors to scalars from a vector space to its field of ...
''T'' on the vector space
In mathematics and physics, a vector space (also called a linear space) is a set (mathematics), set whose elements, often called vector (mathematics and physics), ''vectors'', can be added together and multiplied ("scaled") by numbers called sc ...
of polynomials in a variable ''b'' given by Then one can say
A general formula
The series as a function of is often abbreviated as . Beardon has published formulas for powers of , including a 1996 paper which demonstrated that integer powers of can be written as a linear sum of terms in the sequence : : The first few resulting identities are then : : :. Although other specific cases of – including and – are known, no general formula for for positive integers and has yet been reported. A 2019 paper by Derby proved that: :. This can be calculated in matrix form, as described above. The case replicates Beardon's formula for and confirms the above-stated results for and or . Results for higher powers include: : : :.Notes
External links
* * * A very rare book, but Knuth has placed a photocopy in the Stanford library, call number QA154.8 F3 1631a f MATH. () * (Winner ofLester R. Ford Award
* *{{Cite news , last=Orosi , first=Greg , year=2018 , title=A Simple Derivation Of Faulhaber's Formula , url=http://www.math.nthu.edu.tw/~amen/2018/AMEN-170803.pdf , periodical = Applied Mathematics E-Notes , volume =18 , pages =124–126
A visual proof for the sum of squares and cubes
Finite differences