Perimeter Dike Of C-34 Impoundment on:
[Wikipedia]
[Google]
[Amazon]
 A perimeter is a closed
A perimeter is a closed
 The perimeter is the distance around a shape. Perimeters for more general shapes can be calculated, as any path, with , where is the length of the path and is an infinitesimal line element. Both of these must be replaced by algebraic forms in order to be practically calculated. If the perimeter is given as a closed piecewise smooth plane curve with
:
then its length can be computed as follows:
:
A generalized notion of perimeter, which includes hypersurfaces bounding volumes in -
The perimeter is the distance around a shape. Perimeters for more general shapes can be calculated, as any path, with , where is the length of the path and is an infinitesimal line element. Both of these must be replaced by algebraic forms in order to be practically calculated. If the perimeter is given as a closed piecewise smooth plane curve with
:
then its length can be computed as follows:
:
A generalized notion of perimeter, which includes hypersurfaces bounding volumes in -

 The perimeter of a
The perimeter of a
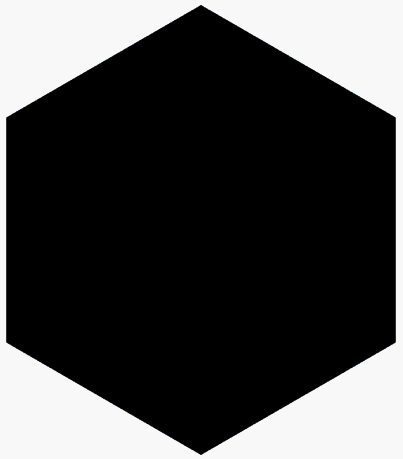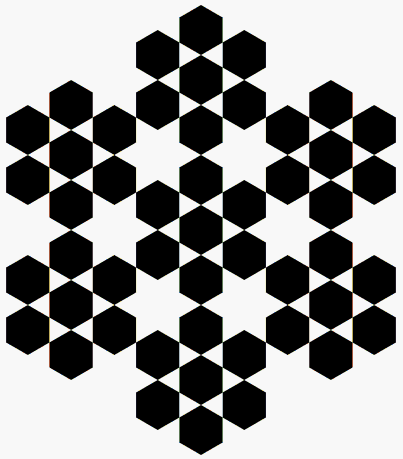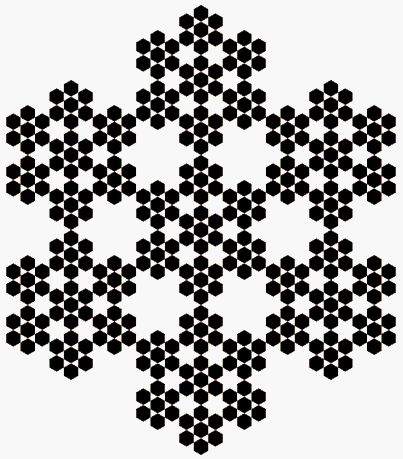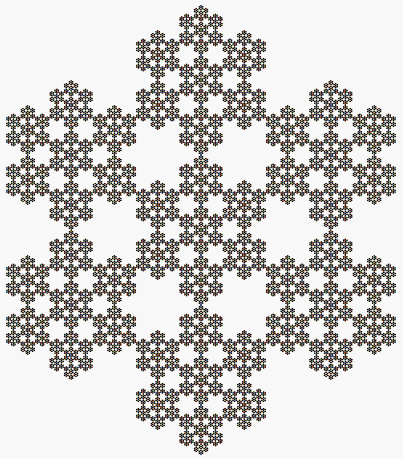
 The perimeter and the
The perimeter and the
path
A path is a route for physical travel – see Trail.
Path or PATH may also refer to:
Physical paths of different types
* Bicycle path
* Bridle path, used by people on horseback
* Course (navigation), the intended path of a vehicle
* Desire p ...
that encompasses, surrounds, or outlines either a two dimensional
In mathematics, a plane is a Euclidean (flat), two-dimensional surface that extends indefinitely. A plane is the two-dimensional analogue of a point (zero dimensions), a line (one dimension) and three-dimensional space. Planes can arise as s ...
shape
A shape or figure is a graphics, graphical representation of an object or its external boundary, outline, or external Surface (mathematics), surface, as opposed to other properties such as color, Surface texture, texture, or material type.
A pl ...
or a one-dimensional
In physics and mathematics, a sequence of ''n'' numbers can specify a location in ''n''-dimensional space. When , the set of all such locations is called a one-dimensional space. An example of a one-dimensional space is the number line, where the ...
length
Length is a measure of distance. In the International System of Quantities, length is a quantity with dimension distance. In most systems of measurement a base unit for length is chosen, from which all other units are derived. In the Interna ...
. The perimeter of a circle
A circle is a shape consisting of all points in a plane that are at a given distance from a given point, the centre. Equivalently, it is the curve traced out by a point that moves in a plane so that its distance from a given point is const ...
or an ellipse
In mathematics, an ellipse is a plane curve surrounding two focus (geometry), focal points, such that for all points on the curve, the sum of the two distances to the focal points is a constant. It generalizes a circle, which is the special ty ...
is called its circumference
In geometry, the circumference (from Latin ''circumferens'', meaning "carrying around") is the perimeter of a circle or ellipse. That is, the circumference would be the arc length of the circle, as if it were opened up and straightened out to ...
.
Calculating the perimeter has several practical applications. A calculated perimeter is the length of fence required to surround a yard or garden. The perimeter of a wheel/circle (its circumference) describes how far it will roll in one revolution
In political science, a revolution (Latin: ''revolutio'', "a turn around") is a fundamental and relatively sudden change in political power and political organization which occurs when the population revolts against the government, typically due ...
. Similarly, the amount of string wound around a spool is related to the spool's perimeter; if the length of the string was exact, it would equal the perimeter.
Formulas
dimensional
In physics and mathematics, the dimension of a mathematical space (or object) is informally defined as the minimum number of coordinates needed to specify any point within it. Thus, a line has a dimension of one (1D) because only one coordi ...
Euclidean space
Euclidean space is the fundamental space of geometry, intended to represent physical space. Originally, that is, in Euclid's Elements, Euclid's ''Elements'', it was the three-dimensional space of Euclidean geometry, but in modern mathematics ther ...
s, is described by the theory of Caccioppoli set
In mathematics, a Caccioppoli set is a set whose boundary is measurable and has (at least locally) a ''finite measure''. A synonym is set of (locally) finite perimeter. Basically, a set is a Caccioppoli set if its characteristic function is a func ...
s.
Polygons
Polygon
In geometry, a polygon () is a plane figure that is described by a finite number of straight line segments connected to form a closed ''polygonal chain'' (or ''polygonal circuit''). The bounded plane region, the bounding circuit, or the two toge ...
s are fundamental to determining perimeters, not only because they are the simplest shapes but also because the perimeters of many shapes are calculated by approximating them with sequences of polygons tending to these shapes. The first mathematician known to have used this kind of reasoning is Archimedes
Archimedes of Syracuse (;; ) was a Greek mathematician, physicist, engineer, astronomer, and inventor from the ancient city of Syracuse in Sicily. Although few details of his life are known, he is regarded as one of the leading scientists ...
, who approximated the perimeter of a circle by surrounding it with regular polygons.
The perimeter of a polygon equals the sum
Sum most commonly means the total of two or more numbers added together; see addition.
Sum can also refer to:
Mathematics
* Sum (category theory), the generic concept of summation in mathematics
* Sum, the result of summation, the additio ...
of the lengths of its sides (edges). In particular, the perimeter of a rectangle
In Euclidean plane geometry, a rectangle is a quadrilateral with four right angles. It can also be defined as: an equiangular quadrilateral, since equiangular means that all of its angles are equal (360°/4 = 90°); or a parallelogram containi ...
of width and length equals
An equilateral polygon is a polygon which has all sides of the same length (for example, a rhombus is a 4-sided equilateral polygon). To calculate the perimeter of an equilateral polygon, one must multiply the common length of the sides by the number of sides.
A regular polygon may be characterized by the number of its sides and by its circumradius
In geometry, the circumscribed circle or circumcircle of a polygon is a circle that passes through all the vertices of the polygon. The center of this circle is called the circumcenter and its radius is called the circumradius.
Not every polyg ...
, that is to say, the constant distance between its centre
Center or centre may refer to:
Mathematics
*Center (geometry), the middle of an object
* Center (algebra), used in various contexts
** Center (group theory)
** Center (ring theory)
* Graph center, the set of all vertices of minimum eccentricity ...
and each of its vertices. The length of its sides can be calculated using trigonometry
Trigonometry () is a branch of mathematics that studies relationships between side lengths and angles of triangles. The field emerged in the Hellenistic world during the 3rd century BC from applications of geometry to astronomical studies. T ...
. If is a regular polygon's radius and is the number of its sides, then its perimeter is
:
A splitter of a triangle
A triangle is a polygon with three Edge (geometry), edges and three Vertex (geometry), vertices. It is one of the basic shapes in geometry. A triangle with vertices ''A'', ''B'', and ''C'' is denoted \triangle ABC.
In Euclidean geometry, an ...
is a cevian (a segment from a vertex to the opposite side) that divides the perimeter into two equal lengths, this common length being called the semiperimeter
In geometry, the semiperimeter of a polygon is half its perimeter. Although it has such a simple derivation from the perimeter, the semiperimeter appears frequently enough in formulas for triangles and other figures that it is given a separate name ...
of the triangle. The three splitters of a triangle all intersect each other at the Nagel point of the triangle.
A cleaver of a triangle is a segment from the midpoint of a side of a triangle to the opposite side such that the perimeter is divided into two equal lengths. The three cleavers of a triangle all intersect each other at the triangle's Spieker center.
Circumference of a circle
 The perimeter of a
The perimeter of a circle
A circle is a shape consisting of all points in a plane that are at a given distance from a given point, the centre. Equivalently, it is the curve traced out by a point that moves in a plane so that its distance from a given point is const ...
, often called the circumference, is proportional to its diameter
In geometry, a diameter of a circle is any straight line segment that passes through the center of the circle and whose endpoints lie on the circle. It can also be defined as the longest chord of the circle. Both definitions are also valid for ...
and its radius
In classical geometry, a radius ( : radii) of a circle or sphere is any of the line segments from its center to its perimeter, and in more modern usage, it is also their length. The name comes from the latin ''radius'', meaning ray but also the ...
. That is to say, there exists a constant number pi, (the Greek ''p'' for perimeter), such that if is the circle's perimeter and its diameter then,
:
In terms of the radius of the circle, this formula becomes,
:
To calculate a circle's perimeter, knowledge of its radius or diameter and the number suffices. The problem is that is not rational (it cannot be expressed as the quotient of two integer
An integer is the number zero (), a positive natural number (, , , etc.) or a negative integer with a minus sign (−1, −2, −3, etc.). The negative numbers are the additive inverses of the corresponding positive numbers. In the language ...
s), nor is it algebraic (it is not a root of a polynomial equation with rational coefficients). So, obtaining an accurate approximation of is important in the calculation. The computation of the digits of is relevant to many fields, such as mathematical analysis
Analysis is the branch of mathematics dealing with continuous functions, limit (mathematics), limits, and related theories, such as Derivative, differentiation, Integral, integration, measure (mathematics), measure, infinite sequences, series (m ...
, algorithmics and computer science
Computer science is the study of computation, automation, and information. Computer science spans theoretical disciplines (such as algorithms, theory of computation, information theory, and automation) to Applied science, practical discipli ...
.
Perception of perimeter

 The perimeter and the
The perimeter and the area
Area is the quantity that expresses the extent of a region on the plane or on a curved surface. The area of a plane region or ''plane area'' refers to the area of a shape
A shape or figure is a graphics, graphical representation of an obje ...
are two main measures of geometric figures. Confusing them is a common error, as well as believing that the greater one of them is, the greater the other must be. Indeed, a commonplace observation is that an enlargement (or a reduction) of a shape make its area grow (or decrease) as well as its perimeter. For example, if a field is drawn on a 1/ scale map, the actual field perimeter can be calculated multiplying the drawing perimeter by . The real area is times the area of the shape on the map. Nevertheless, there is no relation between the area and the perimeter of an ordinary shape. For example, the perimeter of a rectangle of width 0.001 and length 1000 is slightly above 2000, while the perimeter of a rectangle of width 0.5 and length 2 is 5. Both areas are equal to 1.
Proclus
Proclus Lycius (; 8 February 412 – 17 April 485), called Proclus the Successor ( grc-gre, Πρόκλος ὁ Διάδοχος, ''Próklos ho Diádokhos''), was a Greek Neoplatonist philosopher, one of the last major classical philosophers ...
(5th century) reported that Greek peasants "fairly" parted fields relying on their perimeters. However, a field's production is proportional to its area, not to its perimeter, so many naive peasants may have gotten fields with long perimeters but small areas (thus, few crops).
If one removes a piece from a figure, its area decreases but its perimeter may not. In the case of very irregular shapes, confusion between the perimeter and the convex hull
In geometry, the convex hull or convex envelope or convex closure of a shape is the smallest convex set that contains it. The convex hull may be defined either as the intersection of all convex sets containing a given subset of a Euclidean space ...
may arise. The convex hull of a figure may be visualized as the shape formed by a rubber band stretched around it. In the animated picture on the left, all the figures have the same convex hull; the big, first hexagon
In geometry, a hexagon (from Ancient Greek, Greek , , meaning "six", and , , meaning "corner, angle") is a six-sided polygon. The total of the internal angles of any simple polygon, simple (non-self-intersecting) hexagon is 720°.
Regular hexa ...
.
Isoperimetry
The isoperimetric problem is to determine a figure with the largest area, amongst those having a given perimeter. The solution is intuitive; it is thecircle
A circle is a shape consisting of all points in a plane that are at a given distance from a given point, the centre. Equivalently, it is the curve traced out by a point that moves in a plane so that its distance from a given point is const ...
. In particular, this can be used to explain why drops of fat on a broth surface are circular.
This problem may seem simple, but its mathematical proof requires some sophisticated theorems. The isoperimetric problem is sometimes simplified by restricting the type of figures to be used. In particular, to find the quadrilateral, or the triangle, or another particular figure, with the largest area amongst those with the same shape having a given perimeter. The solution to the quadrilateral isoperimetric problem is the square, and the solution to the triangle problem is the equilateral triangle. In general, the polygon with sides having the largest area and a given perimeter is the regular polygon, which is closer to being a circle than is any irregular polygon with the same number of sides.
Etymology
The word comes from the Greek περίμετρος ''perimetros'', from περί ''peri'' "around" and μέτρον ''metron'' "measure".See also
* Arclength *Area
Area is the quantity that expresses the extent of a region on the plane or on a curved surface. The area of a plane region or ''plane area'' refers to the area of a shape
A shape or figure is a graphics, graphical representation of an obje ...
* Coastline paradox
* Girth (geometry)
In three-dimensional geometry, the girth of a geometric object, in a certain direction, is the perimeter of its parallel projection in that direction... For instance, the girth of a unit cube in a direction parallel to one of the three coordinate a ...
* Pythagorean theorem
In mathematics, the Pythagorean theorem or Pythagoras' theorem is a fundamental relation in Euclidean geometry between the three sides of a right triangle. It states that the area of the square whose side is the hypotenuse (the side opposite t ...
* Surface area
The surface area of a solid object is a measure of the total area that the surface of the object occupies. The mathematical definition of surface area in the presence of curved surfaces is considerably more involved than the definition of arc ...
* Volume
Volume is a measure of occupied three-dimensional space. It is often quantified numerically using SI derived units (such as the cubic metre and litre) or by various imperial or US customary units (such as the gallon, quart, cubic inch). The de ...
* Wetted perimeter
The wetted perimeter is the perimeter of the cross sectional area that is "wet". The length of line of the intersection of channel wetted surface with a cross sectional plane normal to the flow direction. The term wetted perimeter is common in ci ...
References
External links
* * {{Authority control Elementary geometry Length