Kepler's laws on:
[Wikipedia]
[Google]
[Amazon]
 In
In
p. 189.
From the bottom of p. 189: ''"Sed res est certissima exactissimaque quod ''proportio qua est inter binorum quorumcunque Planetarum tempora periodica, sit præcise sesquialtera proportionis'' mediarum distantiarum, ... "'' (But it is absolutely certain and exact that the ''proportion between the periodic times of any two planets is precisely the sesquialternate proportion'' .e., the ratio of 3:2of their mean distances, ... ")
An English translation of Kepler's ''Harmonices Mundi'' is available as: Johannes Kepler with E. J. Aiton, A. M. Duncan, and J. V. Field, trans., ''The Harmony of the World'' (Philadelphia, Pennsylvania: American Philosophical Society, 1997); see especiall
p. 411
Kepler had believed in the
pages 554–555
From pp. 554–555: ''" ... plane ut est cum sex planet circa Solem, ... prodit Marius in suo mundo Ioviali ista 3.5.8.13 (vel 14. Galilæo) ... Periodica vero tempora prodit idem Marius ... sunt maiora simplis, minora vero duplis."'' (... just as it is clearly
page 492 Scholia III.
In the margin beside the relevant paragraph is printed: ''Vendelini ingeniosa speculatio circa motus & intervalla satellitum Jovis''. (Wendelin's clever speculation about the movement and distances of Jupiter's satellites.) From p. 492: ''"III. Non minus Kepleriana ingeniosa est Vendelini ... & D. 7. 164/1000. pro penextimo, & D. 16. 756/1000. pro extimo."'' (No less clever

 Mathematically, an ellipse can be represented by the formula:
:
where is the
Mathematically, an ellipse can be represented by the formula:
:
where is the
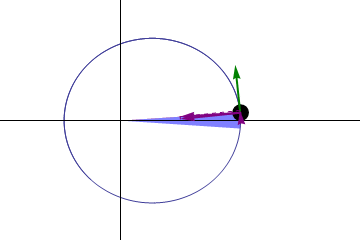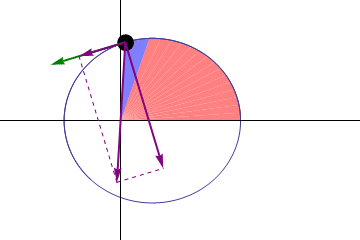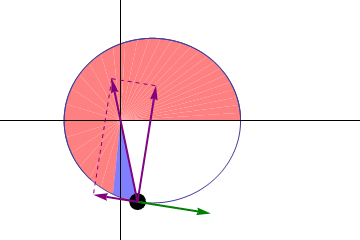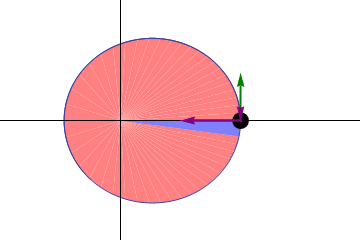 The orbital radius and angular velocity of the planet in the elliptical orbit will vary. This is shown in the animation: the planet travels faster when closer to the Sun, then slower when farther from the Sun. Kepler's second law states that the blue sector has constant area.
In a small time the planet sweeps out a small triangle having base line and height and area , so the constant
The orbital radius and angular velocity of the planet in the elliptical orbit will vary. This is shown in the animation: the planet travels faster when closer to the Sun, then slower when farther from the Sun. Kepler's second law states that the blue sector has constant area.
In a small time the planet sweeps out a small triangle having base line and height and area , so the constant
 The Keplerian problem assumes an
The Keplerian problem assumes an
applet
*
Derivation of Kepler's Laws
(from Newton's laws) at ''Physics Stack Exchange''. * Crowell, Benjamin
Light and Matter
an
https://web.archive.org/web/20060910225253/http://www.phy.syr.edu/courses/java/mc_html/kepler.html
an interactive Java applet that aids in the understanding of Kepler's Second Law. * Audio – Cain/Gay (2010
Astronomy Cast
Johannes Kepler and His Laws of Planetary Motion * University of Tennessee's Dept. Physics & Astronomy: Astronomy 161 page on Johannes Kepler: The Laws of Planetary Motion
* Equant compared to Kepler: interactive mode
* Kepler's Third Law:interactive mode
* Solar System Simulator
educational web pages by David P. Stern {{DEFAULTSORT:Kepler's Laws Of Planetary Motion 1609 in science 1619 in science Copernican Revolution Equations of astronomy Equations Johannes Kepler Orbits
astronomy
Astronomy () is a natural science that studies astronomical object, celestial objects and phenomena. It uses mathematics, physics, and chemistry in order to explain their origin and chronology of the Universe, evolution. Objects of interest ...
, Kepler's laws of planetary motion, published by Johannes Kepler
Johannes Kepler (; ; 27 December 1571 – 15 November 1630) was a German astronomer, mathematician, astrologer, natural philosopher and writer on music. He is a key figure in the 17th-century Scientific Revolution, best known for his laws ...
between 1609 and 1619, describe the orbits
In celestial mechanics, an orbit is the curved trajectory of an object such as the trajectory of a planet around a star, or of a natural satellite around a planet, or of an artificial satellite around an object or position in space such as a p ...
of planet
A planet is a large, rounded astronomical body that is neither a star nor its remnant. The best available theory of planet formation is the nebular hypothesis, which posits that an interstellar cloud collapses out of a nebula to create a you ...
s around the Sun
The Sun is the star at the center of the Solar System. It is a nearly perfect ball of hot plasma, heated to incandescence by nuclear fusion reactions in its core. The Sun radiates this energy mainly as light, ultraviolet, and infrared radi ...
. The laws
Law is a set of rules that are created and are law enforcement, enforceable by social or governmental institutions to regulate behavior,Robertson, ''Crimes against humanity'', 90. with its precise definition a matter of longstanding debate. ...
modified the heliocentric theory
Heliocentrism (also known as the Heliocentric model) is the astronomical model in which the Earth and planets revolve around the Sun at the center of the universe. Historically, heliocentrism was opposed to geocentrism, which placed the Earth at ...
of Nicolaus Copernicus
Nicolaus Copernicus (; pl, Mikołaj Kopernik; gml, Niklas Koppernigk, german: Nikolaus Kopernikus; 19 February 1473 – 24 May 1543) was a Renaissance polymath, active as a mathematician, astronomer, and Catholic Church, Catholic cano ...
, replacing its circular orbit
A circular orbit is an orbit with a fixed distance around the barycenter; that is, in the shape of a circle.
Listed below is a circular orbit in astrodynamics or celestial mechanics under standard assumptions. Here the centripetal force is ...
s and epicycles with elliptical
Elliptical may mean:
* having the shape of an ellipse, or more broadly, any oval shape
** in botany, having an elliptic leaf shape
** of aircraft wings, having an elliptical planform
* characterised by ellipsis (the omission of words), or by conc ...
trajectories, and explaining how planetary velocities
Velocity is the directional speed of an object in motion as an indication of its rate of change in position as observed from a particular frame of reference and as measured by a particular standard of time (e.g. northbound). Velocity is a ...
vary. The three laws state that:
# The orbit
In celestial mechanics, an orbit is the curved trajectory of an object such as the trajectory of a planet around a star, or of a natural satellite around a planet, or of an artificial satellite around an object or position in space such as a p ...
of a planet is an ellipse
In mathematics, an ellipse is a plane curve surrounding two focus (geometry), focal points, such that for all points on the curve, the sum of the two distances to the focal points is a constant. It generalizes a circle, which is the special ty ...
with the Sun at one of the two foci
Focus, or its plural form foci may refer to:
Arts
* Focus or Focus Festival, former name of the Adelaide Fringe arts festival in South Australia Film
*''Focus'', a 1962 TV film starring James Whitmore
* ''Focus'' (2001 film), a 2001 film based ...
.
# A line segment
In geometry, a line segment is a part of a straight line that is bounded by two distinct end points, and contains every point on the line that is between its endpoints. The length of a line segment is given by the Euclidean distance between ...
joining a planet and the Sun sweeps out equal areas during equal intervals of time.
# The square of a planet's orbital period
The orbital period (also revolution period) is the amount of time a given astronomical object takes to complete one orbit around another object. In astronomy, it usually applies to planets or asteroids orbiting the Sun, moons orbiting planets ...
is proportional to the cube of the length of the semi-major axis
In geometry, the major axis of an ellipse is its longest diameter: a line segment that runs through the center and both foci, with ends at the two most widely separated points of the perimeter. The semi-major axis (major semiaxis) is the long ...
of its orbit.
The elliptical orbits of planets were indicated by calculations of the orbit of Mars
Mars is the fourth planet from the Sun and the second-smallest planet in the Solar System, only being larger than Mercury (planet), Mercury. In the English language, Mars is named for the Mars (mythology), Roman god of war. Mars is a terr ...
. From this, Kepler inferred that other bodies in the Solar System
The Solar SystemCapitalization of the name varies. The International Astronomical Union, the authoritative body regarding astronomical nomenclature, specifies capitalizing the names of all individual astronomical objects but uses mixed "Solar S ...
, including those farther away from the Sun, also have elliptical orbits. The second law helps to establish that when a planet is closer to the Sun, it travels faster. The third law expresses that the farther a planet is from the Sun, the slower its orbital speed, and vice versa.
Isaac Newton
Sir Isaac Newton (25 December 1642 – 20 March 1726/27) was an English mathematician, physicist, astronomer, alchemist, theologian, and author (described in his time as a "natural philosopher"), widely recognised as one of the grea ...
showed in 1687 that relationships like Kepler's would apply in the Solar System as a consequence of his own laws of motion and law of universal gravitation.
Comparison to Copernicus
Johannes Kepler
Johannes Kepler (; ; 27 December 1571 – 15 November 1630) was a German astronomer, mathematician, astrologer, natural philosopher and writer on music. He is a key figure in the 17th-century Scientific Revolution, best known for his laws ...
's laws improved the model of Copernicus
Nicolaus Copernicus (; pl, Mikołaj Kopernik; gml, Niklas Koppernigk, german: Nikolaus Kopernikus; 19 February 1473 – 24 May 1543) was a Renaissance polymath, active as a mathematician, astronomer, and Catholic canon, who formulated ...
. According to Copernicus:
# The planetary orbit is a circle with epicycles.
# The Sun is approximately at the center of the orbit.
# The speed of the planet in the main orbit is constant.
Despite being correct in saying that the planets revolved around the Sun, Copernicus was incorrect in defining their orbits. It was Kepler who correctly defined the orbit of planets as followed:
# The planetary orbit is ''not'' a circle with epicycles, but an ''ellipse
In mathematics, an ellipse is a plane curve surrounding two focus (geometry), focal points, such that for all points on the curve, the sum of the two distances to the focal points is a constant. It generalizes a circle, which is the special ty ...
''.
# The Sun is ''not'' near the center but at a '' focal point'' of the elliptical orbit.
# Neither the linear speed nor the angular speed of the planet in the orbit is constant, but the '' area speed'' (closely linked historically with the concept of angular momentum
In physics, angular momentum (rarely, moment of momentum or rotational momentum) is the rotational analog of linear momentum. It is an important physical quantity because it is a conserved quantity—the total angular momentum of a closed syst ...
) is constant.
The eccentricity
Eccentricity or eccentric may refer to:
* Eccentricity (behavior), odd behavior on the part of a person, as opposed to being "normal"
Mathematics, science and technology Mathematics
* Off-Centre (geometry), center, in geometry
* Eccentricity (g ...
of the orbit of the Earth
Earth orbits the Sun at an average distance of 149.60 million km (92.96 million mi) in a counterclockwise direction as viewed from above the Northern Hemisphere. One complete orbit takes days (1 sidereal year), during which time Earth ...
makes the time from the March equinox
The March equinox or northward equinox is the equinox on the Earth when the subsolar point appears to leave the Southern Hemisphere and cross the celestial equator, heading northward as seen from Earth. The March equinox is known as the verna ...
to the September equinox
The September equinox (or southward equinox) is the moment when the Sun appears to cross the celestial equator, heading southward. Because of differences between the calendar year and the tropical year, the September equinox may occur anytime ...
, around 186 days, unequal to the time from the September equinox to the March equinox, around 179 days. A diameter would cut the orbit into equal parts, but the plane through the Sun parallel to the equator
The equator is a circle of latitude, about in circumference, that divides Earth into the Northern and Southern hemispheres. It is an imaginary line located at 0 degrees latitude, halfway between the North and South poles. The term can als ...
of the Earth cuts the orbit into two parts with areas in a 186 to 179 ratio, so the eccentricity of the orbit of the Earth is approximately
:
which is close to the correct value (0.016710218). The accuracy of this calculation requires that the two dates chosen be along the elliptical orbit's minor axis and that the midpoints of each half be along the major axis. As the two dates chosen here are equinoxes, this will be correct when perihelion
An apsis (; ) is the farthest or nearest point in the orbit of a planetary body about its primary body. For example, the apsides of the Earth are called the aphelion and perihelion.
General description
There are two apsides in any ellip ...
, the date the Earth is closest to the Sun, falls on a solstice
A solstice is an event that occurs when the Sun appears to reach its most northerly or southerly excursion relative to the celestial equator on the celestial sphere. Two solstices occur annually, around June 21 and December 21. In many countr ...
. The current perihelion, near January 4, is fairly close to the solstice of December 21 or 22.
Nomenclature
It took nearly two centuries for current formulation of Kepler's work to take on its settled form.Voltaire
François-Marie Arouet (; 21 November 169430 May 1778) was a French Age of Enlightenment, Enlightenment writer, historian, and philosopher. Known by his ''Pen name, nom de plume'' M. de Voltaire (; also ; ), he was famous for his wit, and his ...
's ''Eléments de la philosophie de Newton'' (''Elements of Newton's Philosophy'') of 1738 was the first publication to use the terminology of "laws". The '' Biographical Encyclopedia of Astronomers'' in its article on Kepler (p. 620) states that the terminology of scientific laws for these discoveries was current at least from the time of Joseph de Lalande
Joseph is a common male given name, derived from the Hebrew Yosef (יוֹסֵף). "Joseph" is used, along with "Josef", mostly in English, French and partially German languages. This spelling is also found as a variant in the languages of the mo ...
. It was the exposition of Robert Small, in ''An account of the astronomical discoveries of Kepler'' (1814) that made up the set of three laws, by adding in the third. Small also claimed, against the history, that these were empirical law
Scientific laws or laws of science are statements, based on reproducibility, repeated experiments or observations, that describe or prediction, predict a range of natural phenomena. The term ''law'' has diverse usage in many cases (approximate, a ...
s, based on inductive reasoning
Inductive reasoning is a method of reasoning in which a general principle is derived from a body of observations. It consists of making broad generalizations based on specific observations. Inductive reasoning is distinct from ''deductive'' re ...
.
Further, the current usage of "Kepler's Second Law" is something of a misnomer. Kepler had two versions, related in a qualitative sense: the "distance law" and the "area law". The "area law" is what became the Second Law in the set of three; but Kepler did himself not privilege it in that way.
History
Kepler published his first two laws about planetary motion in 1609, having found them by analyzing the astronomical observations ofTycho Brahe
Tycho Brahe ( ; born Tyge Ottesen Brahe; generally called Tycho (14 December 154624 October 1601) was a Danish astronomer, known for his comprehensive astronomical observations, generally considered to be the most accurate of his time. He was k ...
. Kepler's third law was published in 1619.Johannes Kepler, ''Harmonices Mundi'' he Harmony of the World(Linz, (Austria): Johann Planck, 1619), book 5, chapter 3p. 189.
From the bottom of p. 189: ''"Sed res est certissima exactissimaque quod ''proportio qua est inter binorum quorumcunque Planetarum tempora periodica, sit præcise sesquialtera proportionis'' mediarum distantiarum, ... "'' (But it is absolutely certain and exact that the ''proportion between the periodic times of any two planets is precisely the sesquialternate proportion'' .e., the ratio of 3:2of their mean distances, ... ")
An English translation of Kepler's ''Harmonices Mundi'' is available as: Johannes Kepler with E. J. Aiton, A. M. Duncan, and J. V. Field, trans., ''The Harmony of the World'' (Philadelphia, Pennsylvania: American Philosophical Society, 1997); see especiall
p. 411
Kepler had believed in the
Copernican model
Copernican heliocentrism is the astronomical model developed by Nicolaus Copernicus and published in 1543. This model positioned the Sun at the center of the Universe, motionless, with Earth and the other planets orbiting around it in circular pa ...
of the Solar System, which called for circular orbits, but he could not reconcile Brahe's highly precise observations with a circular fit to Mars' orbit – Mars coincidentally having the highest eccentricity
Eccentricity or eccentric may refer to:
* Eccentricity (behavior), odd behavior on the part of a person, as opposed to being "normal"
Mathematics, science and technology Mathematics
* Off-Centre (geometry), center, in geometry
* Eccentricity (g ...
of all planets except Mercury. His first law reflected this discovery.
In 1621, Kepler noted that his third law applies to the four brightest moons of Jupiter
Jupiter is the fifth planet from the Sun and the List of Solar System objects by size, largest in the Solar System. It is a gas giant with a mass more than two and a half times that of all the other planets in the Solar System combined, but ...
.In 1621, Johannes Kepler noted that Jupiter's moons obey (approximately) his third law in his ''Epitome Astronomiae Copernicanae
The ''Epitome Astronomiae Copernicanae'' was an astronomy book on the heliocentric system published by Johannes Kepler in the period 1618 to 1621. The first volume (books I–III) was printed in 1618, the second (book IV) in 1620, and the third ...
'' pitome of Copernican Astronomy(Linz ("Lentiis ad Danubium"), (Austria): Johann Planck, 1622), book 4, part 2pages 554–555
From pp. 554–555: ''" ... plane ut est cum sex planet circa Solem, ... prodit Marius in suo mundo Ioviali ista 3.5.8.13 (vel 14. Galilæo) ... Periodica vero tempora prodit idem Marius ... sunt maiora simplis, minora vero duplis."'' (... just as it is clearly
rue
''Ruta graveolens'', commonly known as rue, common rue or herb-of-grace, is a species of ''Ruta'' grown as an ornamental plant and herb. It is native to the Balkan Peninsula. It is grown throughout the world in gardens, especially for its bluis ...
among the six planets around the Sun, so also it is among the four oonsof Jupiter, because around the body of Jupiter any atellitethat can go farther from it, orbits slower, and even that rbit's periodis not in the same proportion, but greater han the distance from Jupiter
Han may refer to:
Ethnic groups
* Han Chinese, or Han People (): the name for the largest Chinese people, ethnic group in China, which also constitutes the world's largest ethnic group.
** Han Taiwanese (): the name for the ethnic group of ...
that is, 3/2 (''sescupla'') of the proportion of each of the distances from Jupiter, which is clearly the very roportionas is used for the six planets above. In his ook
Ook, OoK or OOK may refer to:
* Ook Chung (born 1963), Korean-Canadian writer from Quebec
* On-off keying, in radio technology
* Toksook Bay Airport (IATA code OOK), in Alaska
* Ook!, an esoteric programming language based on Brainfuck
* Ook, th ...
''The World of Jupiter'' 'Mundus Jovialis'', 1614 imon Mayr or"Marius" 573–1624presents these distances, from Jupiter, of the four oonsof Jupiter: 3, 5, 8, 13 (or 14 ccording toGalileo) ote: The distances of Jupiter's moons from Jupiter are expressed as multiples of Jupiter's diameter.... Mayr presents their time periods: 1 day 18 1/2 hours, 3 days 13 1/3 hours, 7 days 2 hours, 16 days 18 hours: for all f these datathe proportion is greater than double, thus greater than he proportion
He or HE may refer to:
Language
* He (pronoun), an English pronoun
* He (kana), the romanization of the Japanese kana へ
* He (letter), the fifth letter of many Semitic alphabets
* He (Cyrillic), a letter of the Cyrillic script called ''He'' in ...
of the distances 3, 5, 8, 13 or 14, although less than he proportion
He or HE may refer to:
Language
* He (pronoun), an English pronoun
* He (kana), the romanization of the Japanese kana へ
* He (letter), the fifth letter of many Semitic alphabets
* He (Cyrillic), a letter of the Cyrillic script called ''He'' in ...
of the squares, which double the proportions of the distances, namely 9, 25, 64, 169 or 196, just as power of3/2 is also greater than 1 but less than 2.) Godefroy Wendelin
Godfried Wendelen or Govaert Wendelen, Latinized Godefridus Wendelinus, or sometimes Vendelinus and in French-language sources referred to as Godefroy Wendelin (6 June 1580 – 24 October 1667) was an astronomer and Catholic priest from Lièg ...
also made this observation in 1643.Godefroy Wendelin wrote a letter to Giovanni Battista Riccioli about the relationship between the distances of the Jovian moons from Jupiter and the periods of their orbits, showing that the periods and distances conformed to Kepler's third law. See: Joanne Baptista Riccioli, ''Almagestum novum'' ... (Bologna (Bononia), (Italy): Victor Benati, 1651), volume 1page 492 Scholia III.
In the margin beside the relevant paragraph is printed: ''Vendelini ingeniosa speculatio circa motus & intervalla satellitum Jovis''. (Wendelin's clever speculation about the movement and distances of Jupiter's satellites.) From p. 492: ''"III. Non minus Kepleriana ingeniosa est Vendelini ... & D. 7. 164/1000. pro penextimo, & D. 16. 756/1000. pro extimo."'' (No less clever
han
Han may refer to:
Ethnic groups
* Han Chinese, or Han People (): the name for the largest ethnic group in China, which also constitutes the world's largest ethnic group.
** Han Taiwanese (): the name for the ethnic group of the Taiwanese p ...
Kepler's is the most keen astronomer Wendelin's investigation of the proportion of the periods and distances of Jupiter's satellites, which he had communicated to me with great generosity na very long and very learned letter. So, just as in he case ofthe larger planets, the planets' mean distances from the Sun are respectively in the 3/2 ratio of their periods ; so the distances of these minor planets of Jupiter from Jupiter (which are 3, 5, 8, and 14) are respectively in the 3/2 ratio of heir
Inheritance is the practice of receiving private property, titles, debts, entitlements, privileges, rights, and obligations upon the death of an individual. The rules of inheritance differ among societies and have changed over time. Officiall ...
periods (which are 1.769 days for the innermost o 3.554 days for the next to the innermost uropa 7.164 days for the next to the outermost anymede and 16.756 days for the outermost allisto.) The second law, in the "area law" form, was contested by Nicolaus Mercator
Nicholas (Nikolaus) Mercator (c. 1620, Holstein – 1687, Versailles), also known by his German name Kauffmann, was a 17th-century mathematician.
He was born in Eutin, Schleswig-Holstein, Germany and educated at Rostock and Leyden after which he ...
in a book from 1664, but by 1670 his ''Philosophical Transactions
''Philosophical Transactions of the Royal Society'' is a scientific journal published by the Royal Society. In its earliest days, it was a private venture of the Royal Society's secretary. It was established in 1665, making it the first journa ...
'' were in its favour. As the century proceeded it became more widely accepted. The reception in Germany changed noticeably between 1688, the year in which Newton's '' Principia'' was published and was taken to be basically Copernican, and 1690, by which time work of Gottfried Leibniz
Gottfried Wilhelm (von) Leibniz . ( – 14 November 1716) was a German polymath active as a mathematician, philosopher, scientist and diplomat. He is one of the most prominent figures in both the history of philosophy and the history of mathem ...
on Kepler had been published.
Newton was credited with understanding that the second law is not special to the inverse square law of gravitation, being a consequence just of the radial nature of that law, whereas the other laws do depend on the inverse square form of the attraction. Carl Runge
Carl David Tolmé Runge (; 30 August 1856 – 3 January 1927) was a German mathematician, physicist, and spectroscopist.
He was co-developer and co-eponym of the Runge–Kutta method (German pronunciation: ), in the field of what is today known a ...
and Wilhelm Lenz
Wilhelm Lenz (February 8, 1888 in Frankfurt am Main – April 30, 1957 in Hamburg) was a German physicist, most notable for his invention of the Ising model and for his application of the Laplace–Runge–Lenz vector to the old quantum mechanical ...
much later identified a symmetry principle in the phase space
In dynamical system theory, a phase space is a space in which all possible states of a system are represented, with each possible state corresponding to one unique point in the phase space. For mechanical systems, the phase space usually ...
of planetary motion (the orthogonal group
In mathematics, the orthogonal group in dimension , denoted , is the Group (mathematics), group of isometry, distance-preserving transformations of a Euclidean space of dimension that preserve a fixed point, where the group operation is given by ...
O(4) acting) which accounts for the first and third laws in the case of Newtonian gravitation, as conservation of angular momentum
In physics, angular momentum (rarely, moment of momentum or rotational momentum) is the rotational analog of linear momentum. It is an important physical quantity because it is a conserved quantity—the total angular momentum of a closed system ...
does via rotational symmetry for the second law.
Formulary
The mathematical model of the kinematics of a planet subject to the laws allows a large range of further calculations.First law
The orbit of every planet is an ellipse with the Sun at one of the twofoci Focus, or its plural form foci may refer to: Arts * Focus or Focus Festival, former name of the Adelaide Fringe arts festival in South Australia Film *''Focus'', a 1962 TV film starring James Whitmore * ''Focus'' (2001 film), a 2001 film based ....
semi-latus rectum
In mathematics, a conic section, quadratic curve or conic is a curve obtained as the intersection of the surface of a cone with a plane. The three types of conic section are the hyperbola, the parabola, and the ellipse; the circle is a speci ...
, ''ε'' is the eccentricity
Eccentricity or eccentric may refer to:
* Eccentricity (behavior), odd behavior on the part of a person, as opposed to being "normal"
Mathematics, science and technology Mathematics
* Off-Centre (geometry), center, in geometry
* Eccentricity (g ...
of the ellipse, ''r'' is the distance from the Sun to the planet, and ''θ'' is the angle to the planet's current position from its closest approach, as seen from the Sun. So (''r'', ''θ'') are polar coordinates
In mathematics, the polar coordinate system is a two-dimensional coordinate system in which each point on a plane is determined by a distance from a reference point and an angle from a reference direction. The reference point (analogous to the or ...
.
For an ellipse 0 < ''ε'' < 1 ; in the limiting case ''ε'' = 0, the orbit is a circle with the Sun at the centre (i.e. where there is zero eccentricity).
At ''θ'' = 0°, perihelion
An apsis (; ) is the farthest or nearest point in the orbit of a planetary body about its primary body. For example, the apsides of the Earth are called the aphelion and perihelion.
General description
There are two apsides in any ellip ...
, the distance is minimum
:
At ''θ'' = 90° and at ''θ'' = 270° the distance is equal to .
At ''θ'' = 180°, aphelion
An apsis (; ) is the farthest or nearest point in the orbit of a planetary body about its primary body. For example, the apsides of the Earth are called the aphelion and perihelion.
General description
There are two apsides in any ell ...
, the distance is maximum (by definition, aphelion is – invariably – perihelion plus 180°)
:
The semi-major axis
In geometry, the major axis of an ellipse is its longest diameter: a line segment that runs through the center and both foci, with ends at the two most widely separated points of the perimeter. The semi-major axis (major semiaxis) is the long ...
''a'' is the arithmetic mean
In mathematics and statistics, the arithmetic mean ( ) or arithmetic average, or just the ''mean'' or the ''average'' (when the context is clear), is the sum of a collection of numbers divided by the count of numbers in the collection. The colle ...
between ''r''min and ''r''max:
:
The semi-minor axis
In geometry, the major axis of an ellipse is its longest diameter: a line segment that runs through the center and both focus (geometry), foci, with ends at the two most widely separated points of the perimeter. The semi-major axis (major wikt: ...
''b'' is the geometric mean
In mathematics, the geometric mean is a mean or average which indicates a central tendency of a set of numbers by using the product of their values (as opposed to the arithmetic mean which uses their sum). The geometric mean is defined as the ...
between ''r''min and ''r''max:
:
The semi-latus rectum ''p'' is the harmonic mean
In mathematics, the harmonic mean is one of several kinds of average, and in particular, one of the Pythagorean means. It is sometimes appropriate for situations when the average rate is desired.
The harmonic mean can be expressed as the recipro ...
between ''r''min and ''r''max:
:
The eccentricity ''ε'' is the coefficient of variation
In probability theory and statistics, the coefficient of variation (CV), also known as relative standard deviation (RSD), is a standardized measure of dispersion of a probability distribution or frequency distribution. It is often expressed as ...
between ''r''min and ''r''max:
:
The area
Area is the quantity that expresses the extent of a region on the plane or on a curved surface. The area of a plane region or ''plane area'' refers to the area of a shape
A shape or figure is a graphics, graphical representation of an obje ...
of the ellipse is
:
The special case of a circle is ''ε'' = 0, resulting in ''r'' = ''p'' = ''r''min = ''r''max = ''a'' = ''b'' and ''A'' = ''πr''2.
Second law
A line joining a planet and the Sun sweeps out equal areas during equal intervals of time.Bryant, Jeff; Pavlyk, Oleksandr.
Kepler's Second Law
, ''Wolfram Demonstrations Project The Wolfram Demonstrations Project is an organized, open-source collection of small (or medium-size) interactive programs called Demonstrations, which are meant to visually and interactively represent ideas from a range of fields. It is hos ...''. Retrieved December 27, 2009.
 The orbital radius and angular velocity of the planet in the elliptical orbit will vary. This is shown in the animation: the planet travels faster when closer to the Sun, then slower when farther from the Sun. Kepler's second law states that the blue sector has constant area.
In a small time the planet sweeps out a small triangle having base line and height and area , so the constant
The orbital radius and angular velocity of the planet in the elliptical orbit will vary. This is shown in the animation: the planet travels faster when closer to the Sun, then slower when farther from the Sun. Kepler's second law states that the blue sector has constant area.
In a small time the planet sweeps out a small triangle having base line and height and area , so the constant areal velocity
In classical mechanics, areal velocity (also called sector velocity or sectorial velocity) is a pseudovector whose length equals the rate of change at which area is swept out by a particle as it moves along a curve. In the adjoining figure, supp ...
is
The area enclosed by the elliptical orbit is . So the period satisfies
:
and the mean motion
In orbital mechanics, mean motion (represented by ''n'') is the angular speed required for a body to complete one orbit, assuming constant speed in a circular orbit which completes in the same time as the variable speed, elliptical orbit of the ac ...
of the planet around the Sun
:
satisfies
:
And so,
Third law
The ratio of the square of an object'sThis captures the relationship between the distance of planets from the Sun, and their orbital periods. Kepler enunciated in 1619 this third law in a laborious attempt to determine what he viewed as the "orbital period The orbital period (also revolution period) is the amount of time a given astronomical object takes to complete one orbit around another object. In astronomy, it usually applies to planets or asteroids orbiting the Sun, moons orbiting planets ...with the cube of the semi-major axis of its orbit is the same for all objects orbiting the same primary.
music of the spheres
The ''musica universalis'' (literally universal music), also called music of the spheres or harmony of the spheres, is a philosophical concept that regards proportions in the movements of celestial bodies – the Sun, Moon, and planets – as a fo ...
" according to precise laws, and express it in terms of musical notation. It was therefore known as the ''harmonic law''.
Using Newton's law of gravitation (published 1687), this relation can be found in the case of a circular orbit by setting the centripetal force
A centripetal force (from Latin ''centrum'', "center" and ''petere'', "to seek") is a force that makes a body follow a curved path. Its direction is always orthogonal to the motion of the body and towards the fixed point of the instantaneous c ...
equal to the gravitational force:
:
Then, expressing the angular velocity ω in terms of the orbital period and then rearranging, results in Kepler's Third Law:
:
A more detailed derivation can be done with general elliptical orbits, instead of circles, as well as orbiting the center of mass, instead of just the large mass. This results in replacing a circular radius, , with the semi-major axis, , of the elliptical relative motion of one mass relative to the other, as well as replacing the large mass with . However, with planet masses being so much smaller than the Sun, this correction is often ignored. The full corresponding formula is:
:
where is the mass of the Sun
The solar mass () is a standard unit of mass in astronomy, equal to approximately . It is often used to indicate the masses of other stars, as well as stellar clusters, nebulae, galaxies and black holes. It is approximately equal to the mass ...
, is the mass of the planet, is the gravitational constant
The gravitational constant (also known as the universal gravitational constant, the Newtonian constant of gravitation, or the Cavendish gravitational constant), denoted by the capital letter , is an empirical physical constant involved in ...
, is the orbital period and is the elliptical semi-major axis, and is the astronomical unit
The astronomical unit (symbol: au, or or AU) is a unit of length, roughly the distance from Earth to the Sun and approximately equal to or 8.3 light-minutes. The actual distance from Earth to the Sun varies by about 3% as Earth orbits t ...
, the average distance from earth to the sun.
The following table shows the data used by Kepler to empirically derive his law:
Upon finding this pattern Kepler wrote:
For comparison, here are modern estimates:
Planetary acceleration
Isaac Newton
Sir Isaac Newton (25 December 1642 – 20 March 1726/27) was an English mathematician, physicist, astronomer, alchemist, theologian, and author (described in his time as a "natural philosopher"), widely recognised as one of the grea ...
computed in his ''Philosophiæ Naturalis Principia Mathematica
(English: ''Mathematical Principles of Natural Philosophy'') often referred to as simply the (), is a book by Isaac Newton that expounds Newton's laws of motion and his law of universal gravitation. The ''Principia'' is written in Latin and ...
'' the acceleration
In mechanics, acceleration is the rate of change of the velocity of an object with respect to time. Accelerations are vector quantities (in that they have magnitude and direction). The orientation of an object's acceleration is given by the ...
of a planet moving according to Kepler's first and second laws.
# The ''direction'' of the acceleration is towards the Sun.
# The ''magnitude'' of the acceleration is inversely proportional to the square of the planet's distance from the Sun (the ''inverse square law'').
This implies that the Sun may be the physical cause of the acceleration of planets. However, Newton states in his ''Principia'' that he considers forces from a mathematical point of view, not a physical, thereby taking an instrumentalist view. Moreover, he does not assign a cause to gravity.
Newton defined the force
In physics, a force is an influence that can change the motion of an object. A force can cause an object with mass to change its velocity (e.g. moving from a state of rest), i.e., to accelerate. Force can also be described intuitively as a p ...
acting on a planet to be the product of its mass
Mass is an intrinsic property of a body. It was traditionally believed to be related to the quantity of matter in a physical body, until the discovery of the atom and particle physics. It was found that different atoms and different elementar ...
and the acceleration (see Newton's laws of motion
Newton's laws of motion are three basic laws of classical mechanics that describe the relationship between the motion of an object and the forces acting on it. These laws can be paraphrased as follows:
# A body remains at rest, or in moti ...
). So:
# Every planet is attracted towards the Sun.
# The force acting on a planet is directly proportional to the mass of the planet and is inversely proportional to the square of its distance from the Sun.
The Sun plays an unsymmetrical part, which is unjustified. So he assumed, in Newton's law of universal gravitation
Newton's law of universal gravitation is usually stated as that every particle attracts every other particle in the universe with a force that is proportional to the product of their masses and inversely proportional to the square of the distanc ...
:
# All bodies in the Solar System attract one another.
# The force between two bodies is in direct proportion to the product of their masses and in inverse proportion to the square of the distance between them.
As the planets have small masses compared to that of the Sun, the orbits conform approximately to Kepler's laws. Newton's model improves upon Kepler's model, and fits actual observations more accurately. (See two-body problem
In classical mechanics, the two-body problem is to predict the motion of two massive objects which are abstractly viewed as point particles. The problem assumes that the two objects interact only with one another; the only force affecting each ...
.)
Below comes the detailed calculation of the acceleration of a planet moving according to Kepler's first and second laws.
Acceleration vector
From theheliocentric
Heliocentrism (also known as the Heliocentric model) is the astronomical model in which the Earth and planets revolve around the Sun at the center of the universe. Historically, heliocentrism was opposed to geocentrism, which placed the Earth at ...
point of view consider the vector to the planet where is the distance to the planet and is a unit vector
In mathematics, a unit vector in a normed vector space is a vector (often a spatial vector) of length 1. A unit vector is often denoted by a lowercase letter with a circumflex, or "hat", as in \hat (pronounced "v-hat").
The term ''direction vecto ...
pointing towards the planet.
where is the unit vector whose direction is 90 degrees counterclockwise of , and is the polar angle, and where a dot on top of the variable signifies differentiation with respect to time.
Differentiate the position vector twice to obtain the velocity vector and the acceleration vector:
So
where the radial acceleration is
and the transversal acceleration is
Inverse square law
Kepler's second law says that is constant. The transversal acceleration is zero: So the acceleration of a planet obeying Kepler's second law is directed towards the Sun. The radial acceleration is Kepler's first law states that the orbit is described by the equation: Differentiating with respect to time or Differentiating once more The radial acceleration satisfies Substituting the equation of the ellipse gives The relation gives the simple final result This means that the acceleration vector of any planet obeying Kepler's first and second law satisfies theinverse square law
In science, an inverse-square law is any scientific law stating that a specified physical quantity is inversely proportional to the square of the distance from the source of that physical quantity. The fundamental cause for this can be understoo ...
where
is a constant, and is the unit vector pointing from the Sun towards the planet, and is the distance between the planet and the Sun.
Since mean motion where is the period, according to Kepler's third law, has the same value for all the planets. So the inverse square law for planetary accelerations applies throughout the entire Solar System.
The inverse square law is a differential equation
In mathematics, a differential equation is an equation that relates one or more unknown functions and their derivatives. In applications, the functions generally represent physical quantities, the derivatives represent their rates of change, an ...
. The solutions to this differential equation include the Keplerian motions, as shown, but they also include motions where the orbit is a hyperbola
In mathematics, a hyperbola (; pl. hyperbolas or hyperbolae ; adj. hyperbolic ) is a type of smooth curve lying in a plane, defined by its geometric properties or by equations for which it is the solution set. A hyperbola has two pieces, cal ...
or parabola
In mathematics, a parabola is a plane curve which is mirror-symmetrical and is approximately U-shaped. It fits several superficially different mathematical descriptions, which can all be proved to define exactly the same curves.
One descript ...
or a straight line
In geometry, a line is an infinitely long object with no width, depth, or curvature. Thus, lines are One-dimensional space, one-dimensional objects, though they may exist in Two-dimensional Euclidean space, two, Three-dimensional space, three, ...
. (See Kepler orbit
Johannes Kepler (; ; 27 December 1571 – 15 November 1630) was a German astronomer, mathematician, astrologer, natural philosopher and writer on music. He is a key figure in the 17th-century Scientific Revolution, best known for his laws ...
.)
Newton's law of gravitation
ByNewton's second law
Newton's laws of motion are three basic laws of classical mechanics that describe the relationship between the motion of an object and the forces acting on it. These laws can be paraphrased as follows:
# A body remains at rest, or in motion ...
, the gravitational force that acts on the planet is:
where is the mass of the planet and has the same value for all planets in the Solar System. According to Newton's third law
Newton's laws of motion are three basic laws of classical mechanics that describe the relationship between the motion of an object and the forces acting on it. These laws can be paraphrased as follows:
# A body remains at rest, or in moti ...
, the Sun is attracted to the planet by a force of the same magnitude. Since the force is proportional to the mass of the planet, under the symmetric consideration, it should also be proportional to the mass of the Sun, . So
where is the gravitational constant
The gravitational constant (also known as the universal gravitational constant, the Newtonian constant of gravitation, or the Cavendish gravitational constant), denoted by the capital letter , is an empirical physical constant involved in ...
.
The acceleration of solar system body number ''i'' is, according to Newton's laws:
where is the mass of body ''j'', is the distance between body ''i'' and body ''j'', is the unit vector from body ''i'' towards body ''j'', and the vector summation is over all bodies in the Solar System, besides ''i'' itself.
In the special case where there are only two bodies in the Solar System, Earth and Sun, the acceleration becomes
which is the acceleration of the Kepler motion. So this Earth moves around the Sun according to Kepler's laws.
If the two bodies in the Solar System are Moon and Earth the acceleration of the Moon becomes
So in this approximation, the Moon moves around the Earth according to Kepler's laws.
In the three-body case the accelerations are
These accelerations are not those of Kepler orbits, and the three-body problem
In physics and classical mechanics, the three-body problem is the problem of taking the initial positions and velocities (or momenta) of three point masses and solving for their subsequent motion according to Newton's laws of motion and Newton's ...
is complicated. But Keplerian approximation is the basis for perturbation
Perturbation or perturb may refer to:
* Perturbation theory, mathematical methods that give approximate solutions to problems that cannot be solved exactly
* Perturbation (geology), changes in the nature of alluvial deposits over time
* Perturbat ...
calculations. (See Lunar theory Lunar theory attempts to account for the motions of the Moon. There are many small variations (or perturbations) in the Moon's motion, and many attempts have been made to account for them. After centuries of being problematic, lunar motion can now ...
.)
Position as a function of time
Kepler used his two first laws to compute the position of a planet as a function of time. His method involves the solution of atranscendental equation
In applied mathematics, a transcendental equation is an equation over the real number, real (or complex number, complex) numbers that is not algebraic equation, algebraic, that is, if at least one of its sides describes a transcendental function. ...
called Kepler's equation
In orbital mechanics, Kepler's equation relates various geometric properties of the orbit of a body subject to a central force.
It was first derived by Johannes Kepler in 1609 in Chapter 60 of his ''Astronomia nova'', and in book V of his '' Epi ...
.
The procedure for calculating the heliocentric polar coordinates (''r'',''θ'') of a planet as a function of the time ''t'' since perihelion
An apsis (; ) is the farthest or nearest point in the orbit of a planetary body about its primary body. For example, the apsides of the Earth are called the aphelion and perihelion.
General description
There are two apsides in any ellip ...
, is the following five steps:
# Compute the mean motion
In orbital mechanics, mean motion (represented by ''n'') is the angular speed required for a body to complete one orbit, assuming constant speed in a circular orbit which completes in the same time as the variable speed, elliptical orbit of the ac ...
, where ''P'' is the period.
# Compute the mean anomaly
In celestial mechanics, the mean anomaly is the fraction of an elliptical orbit's period that has elapsed since the orbiting body passed periapsis, expressed as an angle which can be used in calculating the position of that body in the classical ...
, where ''t'' is the time since perihelion.
# Compute the eccentric anomaly In orbital mechanics, the eccentric anomaly is an angular parameter that defines the position of a body that is moving along an elliptic Kepler orbit. The eccentric anomaly is one of three angular parameters ("anomalies") that define a position ...
''E'' by solving Kepler's equation: where is the eccentricity.
# Compute the true anomaly
In celestial mechanics, true anomaly is an angular parameter that defines the position of a body moving along a Keplerian orbit. It is the angle between the direction of periapsis and the current position of the body, as seen from the main focus ...
''θ'' by solving the equation:
# Compute the heliocentric distance ''r'': where is the semimajor axis.
The Cartesian velocity vector can then be calculated as , where is the standard gravitational parameter
In celestial mechanics, the standard gravitational parameter ''μ'' of a celestial body is the product of the gravitational constant ''G'' and the mass ''M'' of the bodies. For two bodies the parameter may be expressed as G(m1+m2), or as GM when ...
.
The important special case of circular orbit, ''ε'' = 0, gives . Because the uniform circular motion was considered to be ''normal'', a deviation from this motion was considered an anomaly.
The proof of this procedure is shown below.
Mean anomaly, ''M''
elliptical orbit
In astrodynamics or celestial mechanics, an elliptic orbit or elliptical orbit is a Kepler orbit with an eccentricity of less than 1; this includes the special case of a circular orbit, with eccentricity equal to 0. In a stricter sense, it ...
and the four points:
* ''s'' the Sun (at one focus of ellipse);
* ''z'' the perihelion
An apsis (; ) is the farthest or nearest point in the orbit of a planetary body about its primary body. For example, the apsides of the Earth are called the aphelion and perihelion.
General description
There are two apsides in any ellip ...
* ''c'' the center of the ellipse
* ''p'' the planet
and
* distance between center and perihelion, the semimajor axis,
* the eccentricity,
* the semiminor axis,
* the distance between Sun and planet.
* the direction to the planet as seen from the Sun, the true anomaly
In celestial mechanics, true anomaly is an angular parameter that defines the position of a body moving along a Keplerian orbit. It is the angle between the direction of periapsis and the current position of the body, as seen from the main focus ...
.
The problem is to compute the polar coordinates
In mathematics, the polar coordinate system is a two-dimensional coordinate system in which each point on a plane is determined by a distance from a reference point and an angle from a reference direction. The reference point (analogous to the or ...
(''r'',''θ'') of the planet from the time since perihelion, ''t''.
It is solved in steps. Kepler considered the circle with the major axis as a diameter, and
* the projection of the planet to the auxiliary circle
* the point on the circle such that the sector areas , ''zcy'', and , ''zsx'', are equal,
* the mean anomaly
In celestial mechanics, the mean anomaly is the fraction of an elliptical orbit's period that has elapsed since the orbiting body passed periapsis, expressed as an angle which can be used in calculating the position of that body in the classical ...
.
The sector areas are related by
The circular sector
A circular sector, also known as circle sector or disk sector (symbol: ⌔), is the portion of a disk (a closed region bounded by a circle) enclosed by two radii and an arc, where the smaller area is known as the ''minor sector'' and the large ...
area
The area swept since perihelion,
is by Kepler's second law proportional to time since perihelion. So the mean anomaly, ''M'', is proportional to time since perihelion, ''t''.
where ''n'' is the mean motion
In orbital mechanics, mean motion (represented by ''n'') is the angular speed required for a body to complete one orbit, assuming constant speed in a circular orbit which completes in the same time as the variable speed, elliptical orbit of the ac ...
.
Eccentric anomaly, ''E''
When the mean anomaly ''M'' is computed, the goal is to compute the true anomaly ''θ''. The function ''θ'' = ''f''(''M'') is, however, not elementary. Kepler's solution is to use ''x'' as seen from the centre, theeccentric anomaly In orbital mechanics, the eccentric anomaly is an angular parameter that defines the position of a body that is moving along an elliptic Kepler orbit. The eccentric anomaly is one of three angular parameters ("anomalies") that define a position ...
as an intermediate variable, and first compute ''E'' as a function of ''M'' by solving Kepler's equation below, and then compute the true anomaly ''θ'' from the eccentric anomaly ''E''. Here are the details.
Division by ''a''2/2 gives Kepler's equation
In orbital mechanics, Kepler's equation relates various geometric properties of the orbit of a body subject to a central force.
It was first derived by Johannes Kepler in 1609 in Chapter 60 of his ''Astronomia nova'', and in book V of his '' Epi ...
This equation gives ''M'' as a function of ''E''. Determining ''E'' for a given ''M'' is the inverse problem. Iterative numerical algorithms are commonly used.
Having computed the eccentric anomaly ''E'', the next step is to calculate the true anomaly ''θ''.
But note: Cartesian position coordinates with reference to the center of ellipse are (''a'' cos ''E'', ''b'' sin ''E'')
With reference to the Sun (with coordinates (''c'',0) = (''ae'',0) ), ''r'' = (''a'' cos ''E'' – ''ae'', ''b'' sin ''E'')
True anomaly would be arctan(''r''''y''/''r''''x''), magnitude of ''r'' would be .
True anomaly, ''θ''
Note from the figure that so that Dividing by and inserting from Kepler's first law to get The result is a usable relationship between the eccentric anomaly ''E'' and the true anomaly ''θ''. A computationally more convenient form follows by substituting into thetrigonometric identity
In trigonometry, trigonometric identities are Equality (mathematics), equalities that involve trigonometric functions and are true for every value of the occurring Variable (mathematics), variables for which both sides of the equality are defined. ...
:
Get
Multiplying by 1 + ''ε'' gives the result
This is the third step in the connection between time and position in the orbit.
Distance, ''r''
The fourth step is to compute the heliocentric distance ''r'' from the true anomaly ''θ'' by Kepler's first law: Using the relation above between ''θ'' and ''E'' the final equation for the distance ''r'' is:See also
*Circular motion
In physics, circular motion is a movement of an object along the circumference of a circle or rotation along a circular path. It can be uniform, with constant angular rate of rotation and constant speed, or non-uniform with a changing rate of ro ...
* Free-fall time The free-fall time is the characteristic time that would take a body to collapse under its own gravitational attraction, if no other forces existed to oppose the collapse. As such, it plays a fundamental role in setting the timescale for a wide vari ...
* Gravity
In physics, gravity () is a fundamental interaction which causes mutual attraction between all things with mass or energy. Gravity is, by far, the weakest of the four fundamental interactions, approximately 1038 times weaker than the stro ...
* Kepler orbit
Johannes Kepler (; ; 27 December 1571 – 15 November 1630) was a German astronomer, mathematician, astrologer, natural philosopher and writer on music. He is a key figure in the 17th-century Scientific Revolution, best known for his laws ...
* Kepler problem
In classical mechanics, the Kepler problem is a special case of the two-body problem, in which the two bodies interact by a central force ''F'' that varies in strength as the inverse square of the distance ''r'' between them. The force may be ei ...
* Kepler's equation
In orbital mechanics, Kepler's equation relates various geometric properties of the orbit of a body subject to a central force.
It was first derived by Johannes Kepler in 1609 in Chapter 60 of his ''Astronomia nova'', and in book V of his '' Epi ...
* Laplace–Runge–Lenz vector
In classical mechanics, the Laplace–Runge–Lenz (LRL) vector is a vector used chiefly to describe the shape and orientation of the orbit of one astronomical body around another, such as a binary star or a planet revolving around a star. For t ...
* Specific relative angular momentum
In celestial mechanics, the specific relative angular momentum (often denoted \vec or \mathbf) of a body is the angular momentum of that body divided by its mass. In the case of two orbiting bodies it is the vector product of their relative positi ...
, relatively easy derivation of Kepler's laws starting with conservation of angular momentum
Explanatory notes
Citations
General bibliography
* Kepler's life is summarized on pages 523–627 and Book Five of his ''magnum opus'', ''Harmonice Mundi
''Harmonice Mundi (Harmonices mundi libri V)''The full title is ''Ioannis Keppleri Harmonices mundi libri V'' (''The Five Books of Johannes Kepler's The Harmony of the World''). (Latin: ''The Harmony of the World'', 1619) is a book by Johannes ...
'' (''harmonies of the world''), is reprinted on pages 635–732 of ''On the Shoulders of Giants'': The Great Works of Physics and Astronomy (works by Copernicus, Kepler
Johannes Kepler (; ; 27 December 1571 – 15 November 1630) was a German astronomer, mathematician, astrologer, natural philosopher and writer on music. He is a key figure in the 17th-century Scientific Revolution, best known for his laws o ...
, Galileo
Galileo di Vincenzo Bonaiuti de' Galilei (15 February 1564 – 8 January 1642) was an Italian astronomer, physicist and engineer, sometimes described as a polymath. Commonly referred to as Galileo, his name was pronounced (, ). He was ...
, Newton
Newton most commonly refers to:
* Isaac Newton (1642–1726/1727), English scientist
* Newton (unit), SI unit of force named after Isaac Newton
Newton may also refer to:
Arts and entertainment
* ''Newton'' (film), a 2017 Indian film
* Newton ( ...
, and Einstein
Albert Einstein ( ; ; 14 March 1879 – 18 April 1955) was a German-born theoretical physicist, widely acknowledged to be one of the greatest and most influential physicists of all time. Einstein is best known for developing the theory ...
). Stephen Hawking, ed. 2002
* A derivation of Kepler's third law of planetary motion is a standard topic in engineering mechanics classes. See, for example, pages 161–164 of .
* Murray and Dermott, Solar System Dynamics, Cambridge University Press 1999,
* V. I. Arnold, ''Mathematical Methods of Classical Mechanics'', Chapter 2. Springer 1989,
External links
* B.Surendranath Reddy; animation of Kepler's laws:applet
*
Derivation of Kepler's Laws
(from Newton's laws) at ''Physics Stack Exchange''. * Crowell, Benjamin
Light and Matter
an
online book
An online book is a resource in book-like form that is only available to read on the Internet. It differs from the common idea of an e-book, which is usually available for users to download and read locally on a computer, smartphone or on an e-re ...
that gives a proof of the first law without the use of calculus (see section 15.7)
* David McNamara and Gianfranco Vidali, ''Kepler's Second Law – Java Interactive Tutorial''https://web.archive.org/web/20060910225253/http://www.phy.syr.edu/courses/java/mc_html/kepler.html
an interactive Java applet that aids in the understanding of Kepler's Second Law. * Audio – Cain/Gay (2010
Astronomy Cast
Johannes Kepler and His Laws of Planetary Motion * University of Tennessee's Dept. Physics & Astronomy: Astronomy 161 page on Johannes Kepler: The Laws of Planetary Motion
* Equant compared to Kepler: interactive mode
* Kepler's Third Law:interactive mode
* Solar System Simulator
educational web pages by David P. Stern {{DEFAULTSORT:Kepler's Laws Of Planetary Motion 1609 in science 1619 in science Copernican Revolution Equations of astronomy Equations Johannes Kepler Orbits