Huygens–Fresnel Principle on:
[Wikipedia]
[Google]
[Amazon]

 The Huygens–Fresnel principle (named after
The Huygens–Fresnel principle (named after
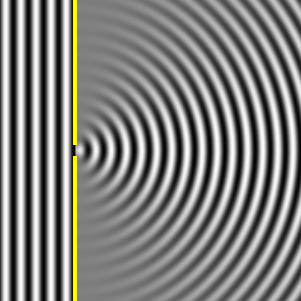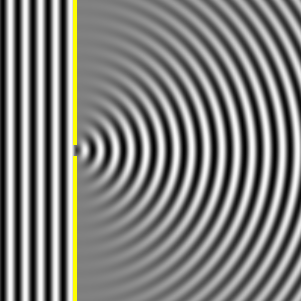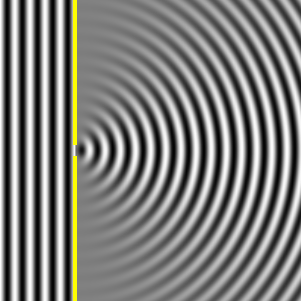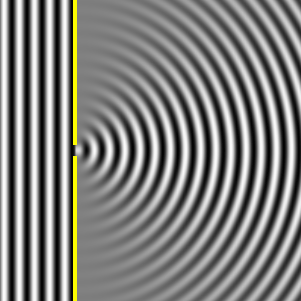 In 1678, Huygens proposed that every point reached by a luminous disturbance becomes a source of a spherical wave; the sum of these secondary waves determines the form of the wave at any subsequent time. He assumed that the secondary waves travelled only in the "forward" direction and it is not explained in the theory why this is the case. He was able to provide a qualitative explanation of linear and spherical wave propagation, and to derive the laws of reflection and refraction using this principle, but could not explain the deviations from rectilinear propagation that occur when light encounters edges, apertures and screens, commonly known as
In 1678, Huygens proposed that every point reached by a luminous disturbance becomes a source of a spherical wave; the sum of these secondary waves determines the form of the wave at any subsequent time. He assumed that the secondary waves travelled only in the "forward" direction and it is not explained in the theory why this is the case. He was able to provide a qualitative explanation of linear and spherical wave propagation, and to derive the laws of reflection and refraction using this principle, but could not explain the deviations from rectilinear propagation that occur when light encounters edges, apertures and screens, commonly known as
 Consider the case of a point source located at a point P0, vibrating at a
Consider the case of a point source located at a point P0, vibrating at a
Dutch
Dutch commonly refers to:
* Something of, from, or related to the Netherlands
* Dutch people ()
* Dutch language ()
Dutch may also refer to:
Places
* Dutch, West Virginia, a community in the United States
* Pennsylvania Dutch Country
People E ...
physicist
A physicist is a scientist who specializes in the field of physics, which encompasses the interactions of matter and energy at all length and time scales in the physical universe.
Physicists generally are interested in the root or ultimate caus ...
Christiaan Huygens
Christiaan Huygens, Lord of Zeelhem, ( , , ; also spelled Huyghens; la, Hugenius; 14 April 1629 – 8 July 1695) was a Dutch mathematician, physicist, engineer, astronomer, and inventor, who is regarded as one of the greatest scientists of ...
and French physicist Augustin-Jean Fresnel
Augustin-Jean Fresnel (10 May 1788 – 14 July 1827) was a French civil engineer and physicist whose research in optics led to the almost unanimous acceptance of the wave theory of light, excluding any remnant of Newton's corpuscular th ...
) states that every point on a wavefront
In physics, the wavefront of a time-varying '' wave field'' is the set ( locus) of all points having the same '' phase''. The term is generally meaningful only for fields that, at each point, vary sinusoidally in time with a single temporal fr ...
is itself the source of spherical wavelets, and the secondary wavelets emanating from different points mutually interfere. The sum of these spherical wavelets forms a new wavefront. As such, the Huygens-Fresnel principle is a method of analysis applied to problems of luminous wave propagation
Wave propagation is any of the ways in which waves travel. Single wave propagation can be calculated by 2nd order wave equation ( standing wavefield) or 1st order one-way wave equation.
With respect to the direction of the oscillation relative to ...
both in the far-field limit and in near-field diffraction
Diffraction is defined as the interference or bending of waves around the corners of an obstacle or through an aperture into the region of geometrical shadow of the obstacle/aperture. The diffracting object or aperture effectively becomes a s ...
as well as reflection Reflection or reflexion may refer to:
Science and technology
* Reflection (physics), a common wave phenomenon
** Specular reflection, reflection from a smooth surface
*** Mirror image, a reflection in a mirror or in water
** Signal reflection, in ...
.
History
 In 1678, Huygens proposed that every point reached by a luminous disturbance becomes a source of a spherical wave; the sum of these secondary waves determines the form of the wave at any subsequent time. He assumed that the secondary waves travelled only in the "forward" direction and it is not explained in the theory why this is the case. He was able to provide a qualitative explanation of linear and spherical wave propagation, and to derive the laws of reflection and refraction using this principle, but could not explain the deviations from rectilinear propagation that occur when light encounters edges, apertures and screens, commonly known as
In 1678, Huygens proposed that every point reached by a luminous disturbance becomes a source of a spherical wave; the sum of these secondary waves determines the form of the wave at any subsequent time. He assumed that the secondary waves travelled only in the "forward" direction and it is not explained in the theory why this is the case. He was able to provide a qualitative explanation of linear and spherical wave propagation, and to derive the laws of reflection and refraction using this principle, but could not explain the deviations from rectilinear propagation that occur when light encounters edges, apertures and screens, commonly known as diffraction
Diffraction is defined as the interference or bending of waves around the corners of an obstacle or through an aperture into the region of geometrical shadow of the obstacle/aperture. The diffracting object or aperture effectively becomes a s ...
effects. The resolution of this error was finally explained by David A. B. Miller in 1991. The resolution is that the source is a dipole (not the monopole assumed by Huygens), which cancels in the reflected direction.
In 1818, Fresnel showed that Huygens's principle, together with his own principle of interference
Interference is the act of interfering, invading, or poaching. Interference may also refer to:
Communications
* Interference (communication), anything which alters, modifies, or disrupts a message
* Adjacent-channel interference, caused by extr ...
could explain both the rectilinear propagation of light and also diffraction effects. To obtain agreement with experimental results, he had to include additional arbitrary assumptions about the phase and amplitude of the secondary waves, and also an obliquity factor. These assumptions have no obvious physical foundation but led to predictions that agreed with many experimental observations, including the Poisson spot.
Poisson was a member of the French Academy, which reviewed Fresnel's work. He used Fresnel's theory to predict that a bright spot ought to appear in the center of the shadow of a small disc, and deduced from this that the theory was incorrect. However, Arago, another member of the committee, performed the experiment and showed that the prediction was correct. (Lisle had observed this fifty years earlier.) This was one of the investigations that led to the victory of the wave theory of light over then predominant corpuscular theory
In optics, the corpuscular theory of light states that light is made up of small discrete particles called " corpuscles" (little particles) which travel in a straight line with a finite velocity and possess impetus. This was based on an alternate ...
.
In antenna theory
In radio engineering, an antenna or aerial is the interface between radio waves propagating through space and electric currents moving in metal conductors, used with a transmitter or receiver. In transmission, a radio transmitter supplies a ...
and engineering, the reformulation of the Huygens–Fresnel principle for radiating current sources is known as surface equivalence principle.
Huygens' principle as a microscopic model
The Huygens–Fresnel principle provides a reasonable basis for understanding and predicting the classical wave propagation of light. However, there are limitations to the principle, namely the same approximations done for deriving theKirchhoff's diffraction formula Kirchhoff's diffraction formula (also Fresnel–Kirchhoff diffraction formula)
can be used to model the propagation of light in a wide range of configurations, either analytically or using numerical modelling. It gives an expression for the wave d ...
and the approximations of near field due to Fresnel. These can be summarized in the fact that the wavelength of light is much smaller than the dimensions of any optical components encountered.
Kirchhoff's diffraction formula Kirchhoff's diffraction formula (also Fresnel–Kirchhoff diffraction formula)
can be used to model the propagation of light in a wide range of configurations, either analytically or using numerical modelling. It gives an expression for the wave d ...
provides a rigorous mathematical foundation for diffraction, based on the wave equation. The arbitrary assumptions made by Fresnel to arrive at the Huygens–Fresnel equation emerge automatically from the mathematics in this derivation.
A simple example of the operation of the principle can be seen when an open doorway connects two rooms and a sound is produced in a remote corner of one of them. A person in the other room will hear the sound as if it originated at the doorway. As far as the second room is concerned, the vibrating air in the doorway is the source of the sound.
Modern physics interpretations
Not all experts agree that the Huygens' principle is an accurate microscopic representation of reality. For instance,Melvin Schwartz
Melvin Schwartz (; November 2, 1932 – August 28, 2006) was an American physicist. He shared the 1988 Nobel Prize in Physics with Leon M. Lederman and Jack Steinberger for their development of the neutrino beam method and their demonstration of ...
argued that "Huygens' principle actually does give the right answer but for the wrong reasons".
This can be reflected in the following facts:
* The microscopic mechanics to create photons and of emission, in general, is essentially acceleration of electrons.
* The original analysis of Huygens included amplitudes only. It includes neither phases nor waves propagating at different speeds (due to diffraction within continuous media), and therefore does not take into account interference.
* The Huygens analysis also does not include polarization for light which imply a vector potential, where instead sound waves can be described with a scalar potential and there is no unique and natural translation between the two.
* In the Huygens description, there is no explanation of why we choose only the forward-going ( retarded wave or forward envelope of wave fronts) versus the backward-propagating advanced wave (backward envelope).
* In the Fresnel approximation there is a concept of non-local behavior due to the sum of spherical waves with different phases that comes from the different points of the wave front, and non local theories are subject of many debates (e.g., not being Lorentz covariant
In relativistic physics, Lorentz symmetry or Lorentz invariance, named after the Dutch physicist Hendrik Lorentz, is an equivalence of observation or observational symmetry due to special relativity implying that the laws of physics stay the same ...
) and of active research.
* The Fresnel approximation can be interpreted in a quantum probabilistic manner but is unclear how much this sum of states (i.e., wavelets on the wavefront) is a complete list of states that are meaningful physically or represents more of an approximation on a generic basis
Basis may refer to:
Finance and accounting
* Adjusted basis, the net cost of an asset after adjusting for various tax-related items
*Basis point, 0.01%, often used in the context of interest rates
* Basis trading, a trading strategy consisting ...
like in the linear combination of atomic orbitals
A linear combination of atomic orbitals or LCAO is a quantum superposition of atomic orbitals and a technique for calculating molecular orbitals in quantum chemistry. In quantum mechanics, electron configurations of atoms are described as wavefu ...
(LCAO) method.
The Huygens' principle is essentially compatible with quantum field theory in the far field approximation, considering effective fields in the center of scattering, considering small perturbations, and in the same sense that quantum optics
Quantum optics is a branch of atomic, molecular, and optical physics dealing with how individual quanta of light, known as photons, interact with atoms and molecules. It includes the study of the particle-like properties of photons. Photons have b ...
is compatible with classical optics
Optics is the branch of physics that studies the behaviour and properties of light, including its interactions with matter and the construction of instruments that use or detect it. Optics usually describes the behaviour of visible, ultravio ...
, other interpretations are subject of debates and active research.
The Feynman model where every point in an imaginary wave front as large as the room is generating a wavelet, shall also be interpreted in these approximations and in a probabilistic context, in this context remote points can only contribute minimally to the overall probability amplitude.
Quantum field theory does not include any microscopic model for photon creation and the concept of single photon is also put under scrutiny on a theoretical level.
Mathematical expression of the principle
frequency
Frequency is the number of occurrences of a repeating event per unit of time. It is also occasionally referred to as ''temporal frequency'' for clarity, and is distinct from ''angular frequency''. Frequency is measured in hertz (Hz) which is eq ...
''f''. The disturbance may be described by a complex variable ''U''0 known as the complex amplitude
Complex commonly refers to:
* Complexity, the behaviour of a system whose components interact in multiple ways so possible interactions are difficult to describe
** Complex system, a system composed of many components which may interact with each ...
. It produces a spherical wave with wavelength
In physics, the wavelength is the spatial period of a periodic wave—the distance over which the wave's shape repeats.
It is the distance between consecutive corresponding points of the same phase on the wave, such as two adjacent crests, tro ...
λ, wavenumber
In the physical sciences, the wavenumber (also wave number or repetency) is the ''spatial frequency'' of a wave, measured in cycles per unit distance (ordinary wavenumber) or radians per unit distance (angular wavenumber). It is analogous to temp ...
. Within a constant of proportionality, the complex amplitude of the primary wave at the point Q located at a distance ''r''0 from P0 is:
:
Note that magnitude
Magnitude may refer to:
Mathematics
*Euclidean vector, a quantity defined by both its magnitude and its direction
*Magnitude (mathematics), the relative size of an object
*Norm (mathematics), a term for the size or length of a vector
*Order of ...
decreases in inverse proportion to the distance travelled, and the phase changes as ''k'' times the distance travelled.
Using Huygens's theory and the principle of superposition of waves, the complex amplitude at a further point P is found by summing the contributions from each point on the sphere of radius ''r''0. In order to get agreement with experimental results, Fresnel found that the individual contributions from the secondary waves on the sphere had to be multiplied by a constant, −''i''/λ, and by an additional inclination factor, ''K''(χ). The first assumption means that the secondary waves oscillate at a quarter of a cycle out of phase with respect to the primary wave, and that the magnitude of the secondary waves are in a ratio of 1:λ to the primary wave. He also assumed that ''K''(χ) had a maximum value when χ = 0, and was equal to zero when χ = π/2, where χ is the angle between the normal of the primary wave front and the normal of the secondary wave front. The complex amplitude at P, due to the contribution of secondary waves, is then given by:
:
where ''S'' describes the surface of the sphere, and ''s'' is the distance between Q and P.
Fresnel used a zone construction method to find approximate values of ''K'' for the different zones, which enabled him to make predictions that were in agreement with experimental results. The integral theorem of Kirchhoff includes the basic idea of Huygens–Fresnel principle. Kirchhoff showed that in many cases, the theorem can be approximated to a simpler form that is equivalent to the formation of Fresnel's formulation.
For an aperture illumination consisting of a single expanding spherical wave, if the radius of the curvature of the wave is sufficiently large, Kirchhoff gave the following expression for ''K''(χ):
:
''K'' has a maximum value at χ = 0 as in the Huygens–Fresnel principle; however, ''K'' is not equal to zero at χ = π/2, but at χ = π.
Above derivation of ''K''(χ) assumed that the diffracting aperture is illuminated by a single spherical wave with a sufficiently large radius of curvature. However, the principle holds for more general illuminations. An arbitrary illumination can be decomposed into a collection of point sources, and the linearity of the wave equation can be invoked to apply the principle to each point source individually. ''K''(χ) can be generally expressed as:
:
In this case, ''K'' satisfies the conditions stated above (maximum value at χ = 0 and zero at χ = π/2).
Generalized Huygens' principle
Many books and references e.g. and refer to the Generalized Huygens' Principle as the one referred byFeynman
Richard Phillips Feynman (; May 11, 1918 – February 15, 1988) was an American theoretical physicist, known for his work in the path integral formulation of quantum mechanics, the theory of quantum electrodynamics, the physics of the superflu ...
in this publication.
Feynman defines the generalized principle in the following way:
This clarifies the fact that in this context the generalized principle reflects the linearity of quantum mechanics and the fact that the quantum mechanics equations are first order in time. Finally only in this case the superposition principle fully apply, i.e. the wave function in a point P can be expanded as a superposition of waves on a border surface enclosing P. Wave functions can be interpreted in the usual quantum mechanical sense as probability densities where the formalism of Green's functions
In mathematics, a Green's function is the impulse response of an inhomogeneous linear differential operator defined on a domain with specified initial conditions or boundary conditions.
This means that if \operatorname is the linear differential ...
and propagators apply. What is note-worthy is that this generalized principle is applicable for "matter waves" and not for light waves any more. The phase factor is now clarified as given by the action
Action may refer to:
* Action (narrative), a literary mode
* Action fiction, a type of genre fiction
* Action game, a genre of video game
Film
* Action film, a genre of film
* ''Action'' (1921 film), a film by John Ford
* ''Action'' (1980 fil ...
and there is no more confusion why the phases of the wavelets are different from the one of the original wave and modified by the additional Fresnel parameters.
As per Greiner the generalized principle can be expressed for in the form:
:
Where G is the usual Green function that propagates in time the wave function . This description resembles and generalize the initial Fresnel's formula of the classical model.
Huygens' theory, Feynman's path integral and the modern photon wave function
Huygens' theory served as a fundamental explanation of the wave nature of light interference and was further developed by Fresnel and Young but did not fully resolve all observations such as the low-intensitydouble-slit experiment
In modern physics, the double-slit experiment is a demonstration that light and matter can display characteristics of both classically defined waves and particles; moreover, it displays the fundamentally probabilistic nature of quantum mechanics ...
first performed by G. I. Taylor in 1909. It was not until the early and mid-1900s that quantum theory discussions, particularly the early discussions at the 1927 Brussels Solvay Conference
The Solvay Conferences (french: Conseils Solvay) have been devoted to outstanding preeminent open problems in both physics and chemistry. They began with the historic invitation-only 1911 Solvay Conference on Physics, considered a turning point i ...
, where Louis de Broglie
Louis Victor Pierre Raymond, 7th Duc de Broglie (, also , or ; 15 August 1892 – 19 March 1987) was a French physicist and aristocrat who made groundbreaking contributions to Old quantum theory, quantum theory. In his 1924 PhD thesis, he pos ...
proposed his de Broglie hypothesis that the photon is guided by a wave function.
The wave function presents a much different explanation of the observed light and dark bands in a double slit experiment. In this conception, the photon follows a path which is a probabilistic choice of one of many possible paths in the electromagnetic field. These probable paths form the pattern: in dark areas, no photons are landing, and in bright areas, many photons are landing. The set of possible photon paths is consistent with Richard Feynman's path integral theory, the paths determined by the surroundings: the photon's originating point (atom), the slit, and the screen and by tracking and summing phases. The wave function is a solution to this geometry. The wave function approach was further supported by additional double-slit experiments in Italy and Japan in the 1970s and 1980s with electrons.
Huygens' principle and quantum field theory
Huygens' principle can be seen as a consequence of thehomogeneity
Homogeneity and heterogeneity are concepts often used in the sciences and statistics relating to the Uniformity (chemistry), uniformity of a Chemical substance, substance or organism. A material or image that is homogeneous is uniform in compos ...
of space—space is uniform in all locations. Any disturbance created in a sufficiently small region of homogeneous space (or in a homogeneous medium) propagates from that region in all geodesic directions. The waves produced by this disturbance, in turn, create disturbances in other regions, and so on. The superposition of all the waves results in the observed pattern of wave propagation.
Homogeneity of space is fundamental to quantum field theory
In theoretical physics, quantum field theory (QFT) is a theoretical framework that combines classical field theory, special relativity, and quantum mechanics. QFT is used in particle physics to construct physical models of subatomic particles and ...
(QFT) where the wave function
A wave function in quantum physics is a mathematical description of the quantum state of an isolated quantum system. The wave function is a complex-valued probability amplitude, and the probabilities for the possible results of measurements mad ...
of any object propagates along all available unobstructed paths. When integrated along all possible paths, with a phase
Phase or phases may refer to:
Science
*State of matter, or phase, one of the distinct forms in which matter can exist
*Phase (matter), a region of space throughout which all physical properties are essentially uniform
* Phase space, a mathematic ...
factor proportional to the action
Action may refer to:
* Action (narrative), a literary mode
* Action fiction, a type of genre fiction
* Action game, a genre of video game
Film
* Action film, a genre of film
* ''Action'' (1921 film), a film by John Ford
* ''Action'' (1980 fil ...
, the interference of the wave-functions correctly predicts observable phenomena. Every point on the wavefront acts as the source of secondary wavelets that spread out in the light cone with the same speed as the wave. The new wavefront is found by constructing the surface tangent to the secondary wavelets.
In other spatial dimensions
In 1900,Jacques Hadamard
Jacques Salomon Hadamard (; 8 December 1865 – 17 October 1963) was a French mathematician who made major contributions in number theory, complex analysis, differential geometry and partial differential equations.
Biography
The son of a teac ...
observed that Huygens' principle was broken when the number of spatial dimensions is even. From this, he developed a set of conjectures that remain an active topic of research. In particular, it has been discovered that Huygens' principle holds on a large class of homogeneous space
In mathematics, particularly in the theories of Lie groups, algebraic groups and topological groups, a homogeneous space for a group ''G'' is a non-empty manifold or topological space ''X'' on which ''G'' acts transitively. The elements of ' ...
s derived from the Coxeter group
In mathematics, a Coxeter group, named after H. S. M. Coxeter, is an abstract group that admits a formal description in terms of reflections (or kaleidoscopic mirrors). Indeed, the finite Coxeter groups are precisely the finite Euclidean refl ...
(so, for example, the Weyl group
In mathematics, in particular the theory of Lie algebras, the Weyl group (named after Hermann Weyl) of a root system Φ is a subgroup of the isometry group of that root system. Specifically, it is the subgroup which is generated by reflections th ...
s of simple Lie algebra
In mathematics, a Lie algebra (pronounced ) is a vector space \mathfrak g together with an Binary operation, operation called the Lie bracket, an Alternating multilinear map, alternating bilinear map \mathfrak g \times \mathfrak g \rightarrow ...
s).
The traditional statement of Huygens' principle for the D'Alembertian
In special relativity, electromagnetism and wave theory, the d'Alembert operator (denoted by a box: \Box), also called the d'Alembertian, wave operator, box operator or sometimes quabla operator (''cf''. nabla symbol) is the Laplace operator of Mi ...
gives rise to the KdV hierarchy In mathematics, the KdV hierarchy is an infinite sequence of partial differential equations which starts with the Korteweg–de Vries equation.
Details
Let T be translation operator defined on real valued functions as T(g)(x)=g(x+1). Let \mathc ...
; analogously, the Dirac operator
In mathematics and quantum mechanics, a Dirac operator is a differential operator that is a formal square root, or half-iterate, of a second-order operator such as a Laplacian. The original case which concerned Paul Dirac was to factorise formall ...
gives rise to the AKNS hierarchy.
See also
*Fraunhofer diffraction
In optics, the Fraunhofer diffraction equation is used to model the diffraction of waves when plane waves are incident on a diffracting object, and the diffraction pattern is viewed at a sufficiently long distance (a distance satisfying Fraunhofer ...
* Kirchhoff's diffraction formula Kirchhoff's diffraction formula (also Fresnel–Kirchhoff diffraction formula)
can be used to model the propagation of light in a wide range of configurations, either analytically or using numerical modelling. It gives an expression for the wave d ...
* Green's function
In mathematics, a Green's function is the impulse response of an inhomogeneous linear differential operator defined on a domain with specified initial conditions or boundary conditions.
This means that if \operatorname is the linear differenti ...
* Green's theorem
In vector calculus, Green's theorem relates a line integral around a simple closed curve to a double integral over the plane region bounded by . It is the two-dimensional special case of Stokes' theorem.
Theorem
Let be a positively orient ...
* Green's identities
In mathematics, Green's identities are a set of three identities in vector calculus relating the bulk with the boundary of a region on which differential operators act. They are named after the mathematician George Green, who discovered Green's ...
* Near-field diffraction pattern
* Double-slit experiment
In modern physics, the double-slit experiment is a demonstration that light and matter can display characteristics of both classically defined waves and particles; moreover, it displays the fundamentally probabilistic nature of quantum mechanics ...
* Knife-edge effect
Diffraction is defined as the interference or bending of waves around the corners of an obstacle or through an aperture into the region of geometrical shadow of the obstacle/aperture. The diffracting object or aperture effectively becomes a s ...
* Fermat's principle
Fermat's principle, also known as the principle of least time, is the link between ray optics and wave optics. In its original "strong" form, Fermat's principle states that the path taken by a ray between two given points is the pat ...
* Fourier optics Fourier optics is the study of classical optics using Fourier transforms (FTs), in which the waveform being considered is regarded as made up of a combination, or '' superposition'', of plane waves. It has some parallels to the Huygens–Fresnel pr ...
* Surface equivalence principle
* Wave field synthesis
Wave field synthesis (WFS) is a spatial audio rendering technique, characterized by creation of virtual acoustic space, acoustic environments. It produces ''artificial'' wavefronts synthesized by a large number of individually driven loudspeake ...
References
Further reading
* Stratton, Julius Adams: ''Electromagnetic Theory'', McGraw-Hill, 1941. (Reissued by Wiley – IEEE Press, ). * B.B. Baker and E.T. Copson, ''The Mathematical Theory of Huygens' Principle'', Oxford, 1939, 1950; AMS Chelsea, 1987. {{DEFAULTSORT:Huygens-Fresnel Principle Wave mechanics Diffraction Christiaan Huygens