Gauss' Theorem on:
[Wikipedia]
[Google]
[Amazon]
In
 Suppose is a subset of (in the case of represents a volume in
Suppose is a subset of (in the case of represents a volume in
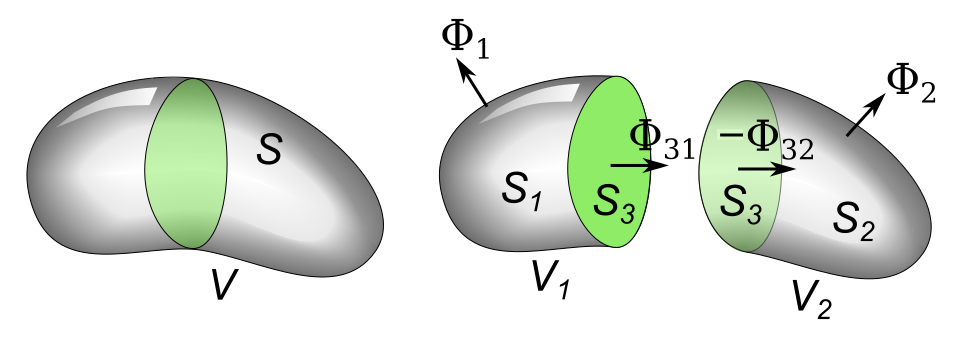 See the diagram. A closed, bounded volume is divided into two volumes and by a surface ''(green)''. The flux out of each component region is equal to the sum of the flux through its two faces, so the sum of the flux out of the two parts is
:
where and are the flux out of surfaces and , is the flux through out of volume 1, and is the flux through out of volume 2. The point is that surface is part of the surface of both volumes. The "outward" direction of the
See the diagram. A closed, bounded volume is divided into two volumes and by a surface ''(green)''. The flux out of each component region is equal to the sum of the flux through its two faces, so the sum of the flux out of the two parts is
:
where and are the flux out of surfaces and , is the flux through out of volume 1, and is the flux through out of volume 2. The point is that surface is part of the surface of both volumes. The "outward" direction of the
 This principle applies to a volume divided into any number of parts, as shown in the diagram. Since the integral over each internal partition ''(green surfaces)'' appears with opposite signs in the flux of the two adjacent volumes they cancel out, and the only contribution to the flux is the integral over the external surfaces ''(grey)''. Since the external surfaces of all the component volumes equal the original surface.
:
This principle applies to a volume divided into any number of parts, as shown in the diagram. Since the integral over each internal partition ''(green surfaces)'' appears with opposite signs in the flux of the two adjacent volumes they cancel out, and the only contribution to the flux is the integral over the external surfaces ''(grey)''. Since the external surfaces of all the component volumes equal the original surface.
:
 The flux out of each volume is the surface integral of the vector field over the surface
:
The goal is to divide the original volume into infinitely many infinitesimal volumes. As the volume is divided into smaller and smaller parts, the surface integral on the right, the flux out of each subvolume, approaches zero because the surface area approaches zero. However, from the definition of
The flux out of each volume is the surface integral of the vector field over the surface
:
The goal is to divide the original volume into infinitely many infinitesimal volumes. As the volume is divided into smaller and smaller parts, the surface integral on the right, the flux out of each subvolume, approaches zero because the surface area approaches zero. However, from the definition of
/ref> :: :The last term on the right vanishes for constant or any divergence free (solenoidal) vector field, e.g. Incompressible flows without sources or sinks such as phase change or chemical reactions etc. In particular, taking to be constant: :: * With for vector field and constant vector c: :: : By reordering the

 Suppose we wish to evaluate
:
where is the
Suppose we wish to evaluate
:
where is the
, and
).
Differential Operators and the Divergence Theorem
at MathPages
The Divergence (Gauss) Theorem
by Nick Bykov,
vector calculus
Vector calculus, or vector analysis, is concerned with differentiation and integration of vector fields, primarily in 3-dimensional Euclidean space \mathbb^3. The term "vector calculus" is sometimes used as a synonym for the broader subjec ...
, the divergence theorem, also known as Gauss's theorem or Ostrogradsky's theorem, reprinted in is a theorem
In mathematics, a theorem is a statement that has been proved, or can be proved. The ''proof'' of a theorem is a logical argument that uses the inference rules of a deductive system to establish that the theorem is a logical consequence of ...
which relates the ''flux
Flux describes any effect that appears to pass or travel (whether it actually moves or not) through a surface or substance. Flux is a concept in applied mathematics and vector calculus which has many applications to physics. For transport ...
'' of a vector field through a closed surface
A surface, as the term is most generally used, is the outermost or uppermost layer of a physical object or space. It is the portion or region of the object that can first be perceived by an observer using the senses of sight and touch, and is t ...
to the ''divergence
In vector calculus, divergence is a vector operator that operates on a vector field, producing a scalar field giving the quantity of the vector field's source at each point. More technically, the divergence represents the volume density of t ...
'' of the field in the volume enclosed.
More precisely, the divergence theorem states that the surface integral
In mathematics, particularly multivariable calculus, a surface integral is a generalization of multiple integrals to integration over surfaces. It can be thought of as the double integral analogue of the line integral. Given a surface, on ...
of a vector field over a closed surface, which is called the "flux" through the surface, is equal to the volume integral
In mathematics (particularly multivariable calculus), a volume integral (∭) refers to an integral over a 3-dimensional domain; that is, it is a special case of multiple integrals. Volume integrals are especially important in physics for many ...
of the divergence over the region inside the surface. Intuitively, it states that "the sum of all sources of the field in a region (with sinks regarded as negative sources) gives the net flux out of the region".
The divergence theorem is an important result for the mathematics of physics
Physics is the natural science that studies matter, its fundamental constituents, its motion and behavior through space and time, and the related entities of energy and force. "Physical science is that department of knowledge which rel ...
and engineering
Engineering is the use of scientific method, scientific principles to design and build machines, structures, and other items, including bridges, tunnels, roads, vehicles, and buildings. The discipline of engineering encompasses a broad rang ...
, particularly in electrostatics
Electrostatics is a branch of physics that studies electric charges at rest (static electricity).
Since classical times, it has been known that some materials, such as amber, attract lightweight particles after rubbing. The Greek word for am ...
and fluid dynamics
In physics and engineering, fluid dynamics is a subdiscipline of fluid mechanics that describes the flow of fluids—liquids and gases. It has several subdisciplines, including '' aerodynamics'' (the study of air and other gases in motion) ...
. In these fields, it is usually applied in three dimensions. However, it generalizes to any number of dimensions. In one dimension, it is equivalent to integration by parts
In calculus, and more generally in mathematical analysis, integration by parts or partial integration is a process that finds the integral of a product of functions in terms of the integral of the product of their derivative and antiderivative. ...
. In two dimensions, it is equivalent to Green's theorem
In vector calculus, Green's theorem relates a line integral around a simple closed curve to a double integral over the plane region bounded by . It is the two-dimensional special case of Stokes' theorem.
Theorem
Let be a positively ori ...
.
Explanation using liquid flow
Vector fields are often illustrated using the example of thevelocity
Velocity is the directional speed of an object in motion as an indication of its rate of change in position as observed from a particular frame of reference and as measured by a particular standard of time (e.g. northbound). Velocity i ...
field of a fluid
In physics, a fluid is a liquid, gas, or other material that continuously deforms (''flows'') under an applied shear stress, or external force. They have zero shear modulus, or, in simpler terms, are substances which cannot resist any shea ...
, such as a gas or liquid. A moving liquid has a velocity—a speed and a direction—at each point, which can be represented by a vector
Vector most often refers to:
*Euclidean vector, a quantity with a magnitude and a direction
*Vector (epidemiology), an agent that carries and transmits an infectious pathogen into another living organism
Vector may also refer to:
Mathematic ...
, so that the velocity of the liquid at any moment forms a vector field. Consider an imaginary closed surface ''S'' inside a body of liquid, enclosing a volume of liquid. The flux
Flux describes any effect that appears to pass or travel (whether it actually moves or not) through a surface or substance. Flux is a concept in applied mathematics and vector calculus which has many applications to physics. For transport ...
of liquid out of the volume is equal to the volume rate of fluid crossing this surface, i.e., the surface integral
In mathematics, particularly multivariable calculus, a surface integral is a generalization of multiple integrals to integration over surfaces. It can be thought of as the double integral analogue of the line integral. Given a surface, on ...
of the velocity over the surface.
Since liquids are incompressible, the amount of liquid inside a closed volume is constant; if there are no sources or sinks inside the volume then the flux of liquid out of ''S'' is zero. If the liquid is moving, it may flow into the volume at some points on the surface ''S'' and out of the volume at other points, but the amounts flowing in and out at any moment are equal, so the ''net'' flux of liquid out of the volume is zero.
However if a ''source'' of liquid is inside the closed surface, such as a pipe through which liquid is introduced, the additional liquid will exert pressure on the surrounding liquid, causing an outward flow in all directions. This will cause a net outward flow through the surface ''S''. The flux outward through ''S'' equals the volume rate of flow of fluid into ''S'' from the pipe. Similarly if there is a ''sink'' or drain inside ''S'', such as a pipe which drains the liquid off, the external pressure of the liquid will cause a velocity throughout the liquid directed inward toward the location of the drain. The volume rate of flow of liquid inward through the surface ''S'' equals the rate of liquid removed by the sink.
If there are multiple sources and sinks of liquid inside ''S'', the flux through the surface can be calculated by adding up the volume rate of liquid added by the sources and subtracting the rate of liquid drained off by the sinks. The volume rate of flow of liquid through a source or sink (with the flow through a sink given a negative sign) is equal to the ''divergence
In vector calculus, divergence is a vector operator that operates on a vector field, producing a scalar field giving the quantity of the vector field's source at each point. More technically, the divergence represents the volume density of t ...
'' of the velocity field at the pipe mouth, so adding up (integrating) the divergence of the liquid throughout the volume enclosed by ''S'' equals the volume rate of flux through ''S''. This is the divergence theorem.
The divergence theorem is employed in any conservation law
In physics, a conservation law states that a particular measurable property of an isolated physical system does not change as the system evolves over time. Exact conservation laws include conservation of energy, conservation of linear momentum, ...
which states that the total volume of all sinks and sources, that is the volume integral of the divergence, is equal to the net flow across the volume's boundary.
Mathematical statement
three-dimensional space
Three-dimensional space (also: 3D space, 3-space or, rarely, tri-dimensional space) is a geometric setting in which three values (called ''parameters'') are required to determine the position of an element (i.e., point). This is the informal ...
) which is compact
Compact as used in politics may refer broadly to a pact or treaty; in more specific cases it may refer to:
* Interstate compact
* Blood compact, an ancient ritual of the Philippines
* Compact government, a type of colonial rule utilized in British ...
and has a piecewise
In mathematics, a piecewise-defined function (also called a piecewise function, a hybrid function, or definition by cases) is a function defined by multiple sub-functions, where each sub-function applies to a different interval in the domain. P ...
smooth boundary (also indicated with ). If is a continuously differentiable vector field defined on a neighborhood of , then:
:
The left side is a volume integral
In mathematics (particularly multivariable calculus), a volume integral (∭) refers to an integral over a 3-dimensional domain; that is, it is a special case of multiple integrals. Volume integrals are especially important in physics for many ...
over the volume , the right side is the surface integral
In mathematics, particularly multivariable calculus, a surface integral is a generalization of multiple integrals to integration over surfaces. It can be thought of as the double integral analogue of the line integral. Given a surface, on ...
over the boundary of the volume . The closed manifold is oriented by outward-pointing normals, and is the outward pointing unit normal at each point on the boundary . ( may be used as a shorthand for .) In terms of the intuitive description above, the left-hand side of the equation represents the total of the sources in the volume , and the right-hand side represents the total flow across the boundary .
Informal derivation
The divergence theorem follows from the fact that if a volume is partitioned into separate parts, theflux
Flux describes any effect that appears to pass or travel (whether it actually moves or not) through a surface or substance. Flux is a concept in applied mathematics and vector calculus which has many applications to physics. For transport ...
out of the original volume is equal to the sum of the flux out of each component volume. This is true despite the fact that the new subvolumes have surfaces that were not part of the original volume's surface, because these surfaces are just partitions between two of the subvolumes and the flux through them just passes from one volume to the other and so cancels out when the flux out of the subvolumes is summed.
 See the diagram. A closed, bounded volume is divided into two volumes and by a surface ''(green)''. The flux out of each component region is equal to the sum of the flux through its two faces, so the sum of the flux out of the two parts is
:
where and are the flux out of surfaces and , is the flux through out of volume 1, and is the flux through out of volume 2. The point is that surface is part of the surface of both volumes. The "outward" direction of the
See the diagram. A closed, bounded volume is divided into two volumes and by a surface ''(green)''. The flux out of each component region is equal to the sum of the flux through its two faces, so the sum of the flux out of the two parts is
:
where and are the flux out of surfaces and , is the flux through out of volume 1, and is the flux through out of volume 2. The point is that surface is part of the surface of both volumes. The "outward" direction of the normal vector
In geometry, a normal is an object such as a line, ray, or vector that is perpendicular to a given object. For example, the normal line to a plane curve at a given point is the (infinite) line perpendicular to the tangent line to the curve ...
is opposite for each volume, so the flux out of one through is equal to the negative of the flux out of the other
:
so these two fluxes cancel in the sum. Therefore
:
Since the union of surfaces and is
:
 This principle applies to a volume divided into any number of parts, as shown in the diagram. Since the integral over each internal partition ''(green surfaces)'' appears with opposite signs in the flux of the two adjacent volumes they cancel out, and the only contribution to the flux is the integral over the external surfaces ''(grey)''. Since the external surfaces of all the component volumes equal the original surface.
:
This principle applies to a volume divided into any number of parts, as shown in the diagram. Since the integral over each internal partition ''(green surfaces)'' appears with opposite signs in the flux of the two adjacent volumes they cancel out, and the only contribution to the flux is the integral over the external surfaces ''(grey)''. Since the external surfaces of all the component volumes equal the original surface.
:
 The flux out of each volume is the surface integral of the vector field over the surface
:
The goal is to divide the original volume into infinitely many infinitesimal volumes. As the volume is divided into smaller and smaller parts, the surface integral on the right, the flux out of each subvolume, approaches zero because the surface area approaches zero. However, from the definition of
The flux out of each volume is the surface integral of the vector field over the surface
:
The goal is to divide the original volume into infinitely many infinitesimal volumes. As the volume is divided into smaller and smaller parts, the surface integral on the right, the flux out of each subvolume, approaches zero because the surface area approaches zero. However, from the definition of divergence
In vector calculus, divergence is a vector operator that operates on a vector field, producing a scalar field giving the quantity of the vector field's source at each point. More technically, the divergence represents the volume density of t ...
, the ratio of flux to volume, , the part in parentheses below, does not in general vanish but approaches the divergence
In vector calculus, divergence is a vector operator that operates on a vector field, producing a scalar field giving the quantity of the vector field's source at each point. More technically, the divergence represents the volume density of t ...
as the volume approaches zero.
:
As long as the vector field has continuous derivatives, the sum above holds even in the limit
Limit or Limits may refer to:
Arts and media
* ''Limit'' (manga), a manga by Keiko Suenobu
* ''Limit'' (film), a South Korean film
* Limit (music), a way to characterize harmony
* "Limit" (song), a 2016 single by Luna Sea
* "Limits", a 2019 ...
when the volume is divided into infinitely small increments
:
As approaches zero volume, it becomes the infinitesimal , the part in parentheses becomes the divergence, and the sum becomes a volume integral
In mathematics (particularly multivariable calculus), a volume integral (∭) refers to an integral over a 3-dimensional domain; that is, it is a special case of multiple integrals. Volume integrals are especially important in physics for many ...
over
Since this derivation is coordinate free, it shows that the divergence does not depend on the coordinates used.
Proofs
For bounded open subsets of Euclidean space
We are going to prove the following: Proof of Theorem. (1) The first step is to reduce to the case where . Pick such that on . Note that and on . Hence it suffices to prove the theorem for . Hence we may assume that . (2) Let be arbitrary. The assumption that has boundary means that there is an open neighborhood of in such that is the graph of a function with lying on one side of this graph. More precisely, this means that after a translation and rotation of , there are and and a function , such that with the notation it holds that and for , Since is compact, we can cover with finitely many neighborhoods of the above form. Note that is an open cover of . By using apartition of unity
In mathematics, a partition of unity of a topological space is a set of continuous functions from to the unit interval ,1such that for every point x\in X:
* there is a neighbourhood of where all but a finite number of the functions of are ...
subordinate to this cover, it suffices to prove the theorem in the case where either has compact support
Support may refer to:
Arts, entertainment, and media
* Supporting character
Business and finance
* Support (technical analysis)
* Child support
* Customer support
* Income Support
Construction
* Support (structure), or lateral support, a ...
in or has compact support in some . If has compact support in , then for all , by the fundamental theorem of calculus, and since vanishes on a neighborhood of . Thus the theorem holds for with compact support in . Thus we have reduced to the case where has compact support in some .
(3) So assume has compact support in some . The last step now is to show that the theorem is true by direct computation. Change notation to , and bring in the notation from (2) used to describe . Note that this means that we have rotated and translated . This is a valid reduction since the theorem is invariant under rotations and translations of coordinates. Since for and for , we have for each that
For we have by the fundamental theorem of calculus that
Now fix . Note that
Define by . By the chain rule,
But since has compact support, we can integrate out first to deduce that
Thus
In summary, with we have
Recall that the outward unit normal to the graph of at a point is and that the surface element is given by . Thus
This completes the proof.
For compact Riemannian manifolds with boundary
We are going to prove the following: Proof of Theorem. We use the Einstein summation convention. By using a partition of unity, we may assume that and have compact support in a coordinate patch . First consider the case where the patch is disjoint from . Then is identified with an open subset of and integration by parts produces no boundary terms: In the last equality we used the Voss-Weyl coordinate formula for the divergence, although the preceding identity could be used to define as the formal adjoint of . Now suppose intersects . Then is identified with an open set in . We zero extend and to and perform integration by parts to obtain where . By a variant of thestraightening theorem for vector fields In differential calculus, the domain-straightening theorem states that, given a vector field X on a manifold
In mathematics, a manifold is a topological space that locally resembles Euclidean space near each point. More precisely, an n-dimens ...
, we may choose so that is the inward unit normal at . In this case is the volume element on and the above formula reads
This completes the proof.
Corollaries
By replacing in the divergence theorem with specific forms, other useful identities can be derived (cf.vector identities
The following are important identities involving derivatives and integrals in vector calculus.
Operator notation
Gradient
For a function f(x, y, z) in three-dimensional Cartesian coordinate variables, the gradient is the vector field:
...
).
* With for a scalar function and a vector field ,
::
:A special case of this is , in which case the theorem is the basis for Green's identities
In mathematics, Green's identities are a set of three identities in vector calculus relating the bulk with the boundary of a region on which differential operators act. They are named after the mathematician George Green, who discovered Green's ...
.
* With for two vector fields and , where denotes a cross product,
::
* With for two vector fields and , where denotes a dot product,
::
* With for a scalar function and vector field c:MathWorld/ref> :: :The last term on the right vanishes for constant or any divergence free (solenoidal) vector field, e.g. Incompressible flows without sources or sinks such as phase change or chemical reactions etc. In particular, taking to be constant: :: * With for vector field and constant vector c: :: : By reordering the
triple product
In geometry and algebra, the triple product is a product of three 3- dimensional vectors, usually Euclidean vectors. The name "triple product" is used for two different products, the scalar-valued scalar triple product and, less often, the vector ...
on the right hand side and taking out the constant vector of the integral,
::
: Hence,
::
Example

unit sphere
In mathematics, a unit sphere is simply a sphere of radius one around a given center. More generally, it is the set of points of distance 1 from a fixed central point, where different norms can be used as general notions of "distance". A u ...
defined by
:
and is the vector field
:
The direct computation of this integral is quite difficult, but we can simplify the derivation of the result using the divergence theorem, because the divergence theorem says that the integral is equal to:
:
where is the unit ball
Unit may refer to:
Arts and entertainment
* UNIT, a fictional military organization in the science fiction television series ''Doctor Who''
* Unit of action, a discrete piece of action (or beat) in a theatrical presentation
Music
* ''Unit'' (al ...
:
:
Since the function is positive in one hemisphere of and negative in the other, in an equal and opposite way, its total integral over is zero. The same is true for :
:
Therefore,
:
because the unit ball has volume
Volume is a measure of occupied three-dimensional space. It is often quantified numerically using SI derived units (such as the cubic metre and litre) or by various imperial or US customary units (such as the gallon, quart, cubic inch). Th ...
.
Applications
Differential and integral forms of physical laws
As a result of the divergence theorem, a host of physical laws can be written in both a differential form (where one quantity is the divergence of another) and an integral form (where the flux of one quantity through a closed surface is equal to another quantity). Three examples areGauss's law
In physics and electromagnetism, Gauss's law, also known as Gauss's flux theorem, (or sometimes simply called Gauss's theorem) is a law relating the distribution of electric charge to the resulting electric field. In its integral form, it sta ...
(in electrostatics
Electrostatics is a branch of physics that studies electric charges at rest (static electricity).
Since classical times, it has been known that some materials, such as amber, attract lightweight particles after rubbing. The Greek word for am ...
), Gauss's law for magnetism
In physics, Gauss's law for magnetism is one of the four Maxwell's equations that underlie classical electrodynamics. It states that the magnetic field has divergence equal to zero, in other words, that it is a solenoidal vector field. It ...
, and Gauss's law for gravity
In physics, Gauss's law for gravity, also known as Gauss's flux theorem for gravity, is a law of physics that is equivalent to Newton's law of universal gravitation. It is named after Carl Friedrich Gauss. It states that the flux (surface integr ...
.
Continuity equations
Continuity equation
A continuity equation or transport equation is an equation that describes the transport of some quantity. It is particularly simple and powerful when applied to a conserved quantity, but it can be generalized to apply to any extensive quantity. ...
s offer more examples of laws with both differential and integral forms, related to each other by the divergence theorem. In fluid dynamics
In physics and engineering, fluid dynamics is a subdiscipline of fluid mechanics that describes the flow of fluids—liquids and gases. It has several subdisciplines, including '' aerodynamics'' (the study of air and other gases in motion) ...
, electromagnetism
In physics, electromagnetism is an interaction that occurs between particles with electric charge. It is the second-strongest of the four fundamental interactions, after the strong force, and it is the dominant force in the interactions o ...
, quantum mechanics
Quantum mechanics is a fundamental theory in physics that provides a description of the physical properties of nature at the scale of atoms and subatomic particles. It is the foundation of all quantum physics including quantum chemistry, q ...
, relativity theory
The theory of relativity usually encompasses two interrelated theories by Albert Einstein: special relativity and general relativity, proposed and published in 1905 and 1915, respectively. Special relativity applies to all physical phenomena in ...
, and a number of other fields, there are continuity equation
A continuity equation or transport equation is an equation that describes the transport of some quantity. It is particularly simple and powerful when applied to a conserved quantity, but it can be generalized to apply to any extensive quantity. ...
s that describe the conservation of mass, momentum, energy, probability, or other quantities. Generically, these equations state that the divergence of the flow of the conserved quantity is equal to the distribution of ''sources'' or ''sinks'' of that quantity. The divergence theorem states that any such continuity equation can be written in a differential form (in terms of a divergence) and an integral form (in terms of a flux).
Inverse-square laws
Any ''inverse-square law
In science, an inverse-square law is any scientific law stating that a specified physical quantity is inversely proportional to the square of the distance from the source of that physical quantity. The fundamental cause for this can be unde ...
'' can instead be written in a ''Gauss's law''-type form (with a differential and integral form, as described above). Two examples are Gauss's law
In physics and electromagnetism, Gauss's law, also known as Gauss's flux theorem, (or sometimes simply called Gauss's theorem) is a law relating the distribution of electric charge to the resulting electric field. In its integral form, it sta ...
(in electrostatics), which follows from the inverse-square Coulomb's law
Coulomb's inverse-square law, or simply Coulomb's law, is an experimental law of physics that quantifies the amount of force between two stationary, electrically charged particles. The electric force between charged bodies at rest is conventio ...
, and Gauss's law for gravity
In physics, Gauss's law for gravity, also known as Gauss's flux theorem for gravity, is a law of physics that is equivalent to Newton's law of universal gravitation. It is named after Carl Friedrich Gauss. It states that the flux (surface integr ...
, which follows from the inverse-square Newton's law of universal gravitation
Newton's law of universal gravitation is usually stated as that every particle attracts every other particle in the universe with a force that is proportional to the product of their masses and inversely proportional to the square of the distanc ...
. The derivation of the Gauss's law-type equation from the inverse-square formulation or vice versa is exactly the same in both cases; see either of those articles for details.
History
Joseph-Louis Lagrange
Joseph-Louis Lagrange (born Giuseppe Luigi LagrangiaMécanique Analytique
''Mécanique analytique'' (1788–89) is a two volume French treatise on analytical mechanics, written by Joseph-Louis Lagrange, and published 101 years following Isaac Newton's
''Philosophiæ Naturalis Principia Mathematica''. It consolidated i ...
.'' Lagrange employed surface integrals in his work on fluid mechanics. He discovered the divergence theorem in 1762.
Carl Friedrich Gauss
Johann Carl Friedrich Gauss (; german: Gauß ; la, Carolus Fridericus Gauss; 30 April 177723 February 1855) was a German mathematician and physicist who made significant contributions to many fields in mathematics and science. Sometimes refe ...
was also using surface integrals while working on the gravitational attraction of an elliptical spheroid in 1813, when he proved special cases of the divergence theorem. He proved additional special cases in 1833 and 1839. But it was Mikhail Ostrogradsky
Mikhail Vasilyevich Ostrogradsky (transcribed also ''Ostrogradskiy'', Ostrogradskiĭ) (russian: Михаи́л Васи́льевич Острогра́дский, ua, Миха́йло Васи́льович Острогра́дський; 24 Sep ...
, who gave the first proof of the general theorem, in 1826, as part of his investigation of heat flow. Special cases were proven by George Green in 1828 in ''An Essay on the Application of Mathematical Analysis to the Theories of Electricity and Magnetism'', Siméon Denis Poisson
Baron Siméon Denis Poisson FRS FRSE (; 21 June 1781 – 25 April 1840) was a French mathematician and physicist who worked on statistics, complex analysis, partial differential equations, the calculus of variations, analytical mechanics, electr ...
in 1824 in a paper on elasticity, and Frédéric Sarrus in 1828 in his work on floating bodies.
Worked examples
Example 1
To verify the planar variant of the divergence theorem for a region : : and the vector field: : The boundary of is the unit circle, , that can be represented parametrically by: : such that where units is the length arc from the point to the point on . Then a vector equation of is : At a point on : : Therefore, : Because , we can evaluate and because . Thus :Example 2
Let's say we wanted to evaluate the flux of the following vector field defined by bounded by the following inequalities: : By the divergence theorem, : We now need to determine the divergence of . If is a three-dimensional vector field, then the divergence of is given by . Thus, we can set up the following flux integral as follows: : Now that we have set up the integral, we can evaluate it. :Generalizations
Multiple dimensions
One can use the generalStokes' Theorem
Stokes's theorem, also known as the Kelvin–Stokes theorem Nagayoshi Iwahori, et al.:"Bi-Bun-Seki-Bun-Gaku" Sho-Ka-Bou(jp) 1983/12Written in Japanese)Atsuo Fujimoto;"Vector-Kai-Seki Gendai su-gaku rekucha zu. C(1)" :ja:培風館, Bai-Fu-Kan( ...
to equate the -dimensional volume integral of the divergence of a vector field over a region to the -dimensional surface integral of over the boundary of :
:
This equation is also known as the divergence theorem.
When , this is equivalent to Green's theorem
In vector calculus, Green's theorem relates a line integral around a simple closed curve to a double integral over the plane region bounded by . It is the two-dimensional special case of Stokes' theorem.
Theorem
Let be a positively ori ...
.
When , it reduces to the fundamental theorem of calculus
The fundamental theorem of calculus is a theorem that links the concept of differentiating a function (calculating its slopes, or rate of change at each time) with the concept of integrating a function (calculating the area under its graph, ...
, part 2.
Tensor fields
Writing the theorem inEinstein notation
In mathematics, especially the usage of linear algebra in Mathematical physics, Einstein notation (also known as the Einstein summation convention or Einstein summation notation) is a notational convention that implies summation over a set of ...
:
:
suggestively, replacing the vector field with a rank- tensor field , this can be generalized to:
:
where on each side, tensor contraction
In multilinear algebra, a tensor contraction is an operation on a tensor that arises from the natural pairing of a finite-dimensional vector space and its dual. In components, it is expressed as a sum of products of scalar components of the tensor ...
occurs for at least one index. This form of the theorem is still in 3d, each index takes values 1, 2, and 3. It can be generalized further still to higher (or lower) dimensions (for example to 4d spacetime
In physics, spacetime is a mathematical model that combines the three dimensions of space and one dimension of time into a single four-dimensional manifold. Spacetime diagrams can be used to visualize relativistic effects, such as why diffe ...
in general relativity
General relativity, also known as the general theory of relativity and Einstein's theory of gravity, is the geometric theory of gravitation published by Albert Einstein in 1915 and is the current description of gravitation in modern physics. ...
see for example: , and
).
See also
*Kelvin–Stokes theorem
Stokes's theorem, also known as the Kelvin–Stokes theoremNagayoshi Iwahori, et al.:"Bi-Bun-Seki-Bun-Gaku" Sho-Ka-Bou(jp) 1983/12Written in Japanese)Atsuo Fujimoto;"Vector-Kai-Seki Gendai su-gaku rekucha zu. C(1)" :ja:培風館, Bai-Fu-Kan(j ...
References
External links
*Differential Operators and the Divergence Theorem
at MathPages
The Divergence (Gauss) Theorem
by Nick Bykov,
Wolfram Demonstrations Project
The Wolfram Demonstrations Project is an organized, open-source collection of small (or medium-size) interactive programs called Demonstrations, which are meant to visually and interactively represent ideas from a range of fields. It is hos ...
.
* – ''This article was originally based on the GFDL
The GNU Free Documentation License (GNU FDL or simply GFDL) is a copyleft license for free documentation, designed by the Free Software Foundation (FSF) for the GNU Project. It is similar to the GNU General Public License, giving readers the r ...
article from PlanetMath
PlanetMath is a free, collaborative, mathematics online encyclopedia. The emphasis is on rigour, openness, pedagogy, real-time content, interlinked content, and also community of about 24,000 people with various maths interests. Intended to be c ...
at https://web.archive.org/web/20021029094728/http://planetmath.org/encyclopedia/Divergence.html ''
{{Calculus topics
Theorems in calculus