Evolvent on:
[Wikipedia]
[Google]
[Amazon]
 In
In
 In order to derive properties of a regular curve it is advantageous to suppose the arc length to be the parameter of the given curve, which lead to the following simplifications: and , with the
In order to derive properties of a regular curve it is advantageous to suppose the arc length to be the parameter of the given curve, which lead to the following simplifications: and , with the
 For a circle with parametric representation , one has
.
Hence , and the path length is .
Evaluating the above given equation of the involute, one gets
:
for the
For a circle with parametric representation , one has
.
Hence , and the path length is .
Evaluating the above given equation of the involute, one gets
:
for the 
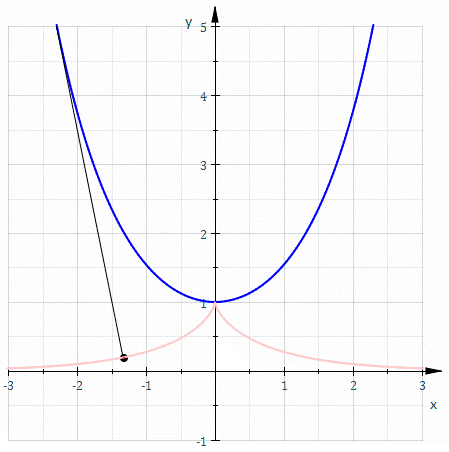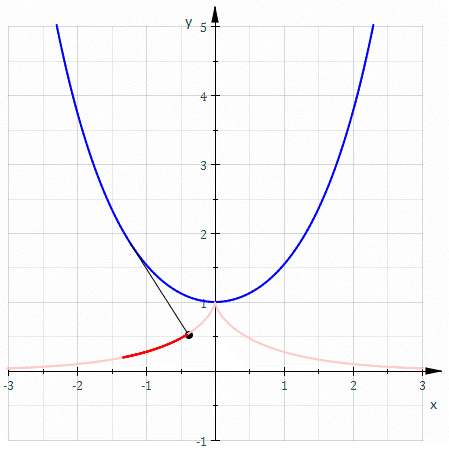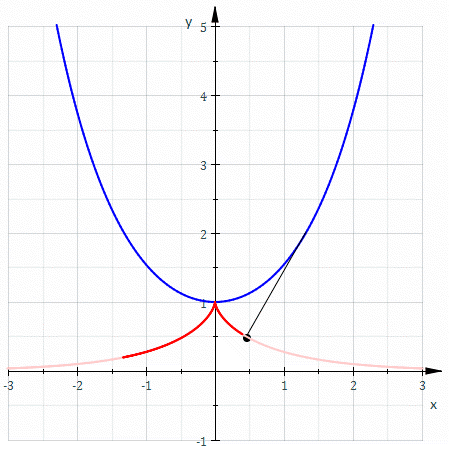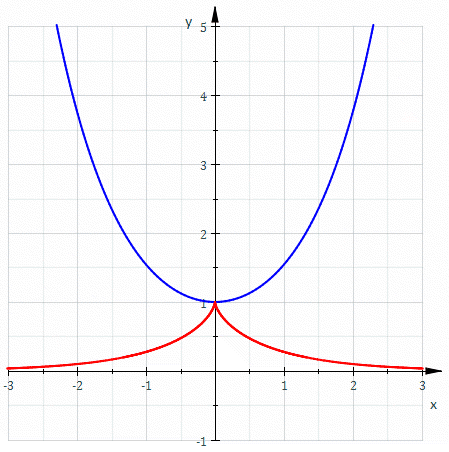
 The parametric representation describes a cycloid. From , one gets (after having used some trigonometric formulas)
:
and
:
Hence the equations of the corresponding involute are
:
:
which describe the shifted red cycloid of the diagram. Hence
* The involutes of the cycloid are parallel curves of the cycloid
:
(Parallel curves of a cycloid are not cycloids.)
The parametric representation describes a cycloid. From , one gets (after having used some trigonometric formulas)
:
and
:
Hence the equations of the corresponding involute are
:
:
which describe the shifted red cycloid of the diagram. Hence
* The involutes of the cycloid are parallel curves of the cycloid
:
(Parallel curves of a cycloid are not cycloids.)
Springerlink
(subscription required). The involute of a circle is also an important shape in gas compressing, as a
Involute
at MathWorld {{Differential transforms of plane curves Differential geometry Roulettes (curve)
mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics ...
, an involute (also known as an evolvent) is a particular type of curve
In mathematics, a curve (also called a curved line in older texts) is an object similar to a line (geometry), line, but that does not have to be Linearity, straight.
Intuitively, a curve may be thought of as the trace left by a moving point (ge ...
that is dependent on another shape or curve. An involute of a curve is the locus of a point on a piece of taut string as the string is either unwrapped from or wrapped around the curve.
It is a class of curves coming under the roulette
Roulette is a casino game named after the French word meaning ''little wheel'' which was likely developed from the Italian game Biribi''.'' In the game, a player may choose to place a bet on a single number, various groupings of numbers, the ...
family of curves.
The evolute of an involute is the original curve.
The notions of the involute and evolute of a curve were introduced by Christiaan Huygens
Christiaan Huygens, Lord of Zeelhem, ( , , ; also spelled Huyghens; la, Hugenius; 14 April 1629 – 8 July 1695) was a Dutch mathematician, physicist, engineer, astronomer, and inventor, who is regarded as one of the greatest scientists of ...
in his work titled '' Horologium oscillatorium sive de motu pendulorum ad horologia aptato demonstrationes geometricae'' (1673).
Involute of a parameterized curve
Let be aregular curve
In mathematics, a curve (also called a curved line in older texts) is an object similar to a line, but that does not have to be straight.
Intuitively, a curve may be thought of as the trace left by a moving point. This is the definition that ap ...
in the plane with its curvature
In mathematics, curvature is any of several strongly related concepts in geometry. Intuitively, the curvature is the amount by which a curve deviates from being a straight line, or a surface deviates from being a plane.
For curves, the canonic ...
nowhere 0 and , then the curve with the parametric representation
is an ''involute'' of the given curve.
Adding an arbitrary but fixed number to the integral results in an involute corresponding to a string extended by (like a ball of wool yarn having some length of thread already hanging before it is unwound). Hence, the involute can be varied by constant and/or adding a number to the integral (see Involutes of a semicubic parabola).
If one gets
:
Properties of involutes
 In order to derive properties of a regular curve it is advantageous to suppose the arc length to be the parameter of the given curve, which lead to the following simplifications: and , with the
In order to derive properties of a regular curve it is advantageous to suppose the arc length to be the parameter of the given curve, which lead to the following simplifications: and , with the curvature
In mathematics, curvature is any of several strongly related concepts in geometry. Intuitively, the curvature is the amount by which a curve deviates from being a straight line, or a surface deviates from being a plane.
For curves, the canonic ...
and the unit normal. One gets for the involute:
: and
:
and the statement:
*At point the involute is ''not regular'' (because ),
and from follows:
* The normal of the involute at point is the tangent of the given curve at point .
* The involutes are parallel curve
A parallel of a curve is the envelope of a family of congruent circles centered on the curve.
It generalises the concept of '' parallel (straight) lines''. It can also be defined as a curve whose points are at a constant ''normal distance'' fr ...
s, because of and the fact, that is the unit normal at .
Examples
Involutes of a circle
parametric equation
In mathematics, a parametric equation defines a group of quantities as functions of one or more independent variables called parameters. Parametric equations are commonly used to express the coordinates of the points that make up a geometric obj ...
of the involute of the circle.
The term is optional; it serves to set the start location of the curve on the circle. The figure shows involutes for (green), (red), (purple) and (light blue). The involutes look like Archimedean spiral
The Archimedean spiral (also known as the arithmetic spiral) is a spiral named after the 3rd-century BC Greek mathematician Archimedes. It is the locus corresponding to the locations over time of a point moving away from a fixed point with a con ...
s, but they are actually not.
The arc length for and of the involute is
:
Involutes of a semicubic parabola
Theparametric equation
In mathematics, a parametric equation defines a group of quantities as functions of one or more independent variables called parameters. Parametric equations are commonly used to express the coordinates of the points that make up a geometric obj ...
describes a semicubical parabola
In mathematics, a cuspidal cubic or semicubical parabola is an algebraic plane curve that has an implicit equation of the form
: y^2 - a^2 x^3 = 0
(with ) in some Cartesian coordinate system.
Solving for leads to the ''explicit form''
: y = \ ...
. From one gets and . Extending the string by extensively simplifies further calculation, and one gets
:
Eliminating yields showing that this involute is a parabola.
The other involutes are thus parallel curves of a parabola, and are not parabolas, as they are curves of degree six (See ).

Involutes of a catenary
For the catenary , the tangent vector is , and, as its length is . Thus the arc length from the point is Hence the involute starting from is parametrized by : and is thus a tractrix. The other involutes are not tractrices, as they are parallel curves of a tractrix.Involutes of a cycloid
Involute and evolute
The evolute of a given curve consists of the curvature centers of . Between involutes and evolutes the following statement holds: :''A curve is the evolute of any of its involutes.''Application
The most common profiles of modern gear teeth are involutes of a circle. In an involute gear system the teeth of two meshing gears contact at a single instantaneous point that follows along a single straight line of action. The forces exerted the contacting teeth exert on each other also follow this line, and are normal to the teeth. The involute gear system maintaining these conditions follows the fundamental law of gearing: the ratio of angular velocities between the two gears must remain constant throughout. With teeth of other shapes, the relative speeds and forces rise and fall as successive teeth engage, resulting in vibration, noise, and excessive wear. For this reason, nearly all modern planar gear systems are either involute or the relatedcycloidal gear
The cycloidal gear profile is a form of toothed gear used in mechanical clocks, rather than the involute gear form used for most other gears. The gear tooth profile is based on the epicycloid and hypocycloid curves, which are the curves generate ...
system.V. G. A. Goss (2013) "Application of analytical geometry to the shape of gear teeth", Resonance 18(9): 817 to 3Springerlink
(subscription required). The involute of a circle is also an important shape in gas compressing, as a
scroll compressor
A scroll compressor (also called ''spiral compressor'', scroll pump and scroll vacuum pump) is a device for compressing air or refrigerant. It is used in air conditioning equipment, as an automobile supercharger (where it is known as a scroll- ...
can be built based on this shape. Scroll compressors make less sound than conventional compressors and have proven to be quite efficient.
The High Flux Isotope Reactor
The High Flux Isotope Reactor (HFIR) is a nuclear research reactor at Oak Ridge National Laboratory (ORNL) in Oak Ridge, Tennessee, United States. Operating at 85 MW, HFIR is one of the highest flux reactor-based sources of neutrons for condense ...
uses involute-shaped fuel elements, since these allow a constant-width channel between them for coolant.
See also
* Evolute *Scroll compressor
A scroll compressor (also called ''spiral compressor'', scroll pump and scroll vacuum pump) is a device for compressing air or refrigerant. It is used in air conditioning equipment, as an automobile supercharger (where it is known as a scroll- ...
* Involute gear
* Roulette (curve)
* Envelope (mathematics)
References
External links
Involute
at MathWorld {{Differential transforms of plane curves Differential geometry Roulettes (curve)