Distance ladder on:
[Wikipedia]
[Google]
[Amazon]
 The cosmic distance ladder (also known as the extragalactic distance scale) is the succession of methods by which
The cosmic distance ladder (also known as the extragalactic distance scale) is the succession of methods by which
 At the base of the ladder are ''fundamental'' distance measurements, in which distances are determined directly, with no physical assumptions about the nature of the object in question. The precise measurement of stellar positions is part of the discipline of astrometry.
At the base of the ladder are ''fundamental'' distance measurements, in which distances are determined directly, with no physical assumptions about the nature of the object in question. The precise measurement of stellar positions is part of the discipline of astrometry.
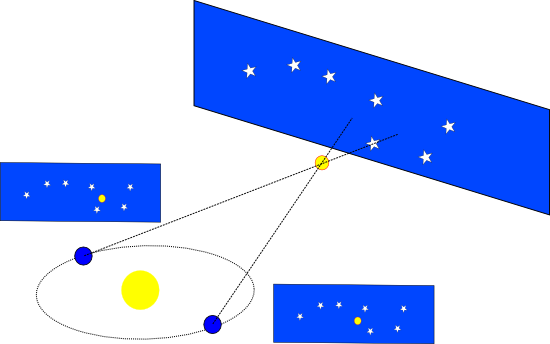 The most important fundamental distance measurements come from trigonometric parallax. As the Earth orbits the Sun, the position of nearby stars will appear to shift slightly against the more distant background. These shifts are angles in an isosceles
The most important fundamental distance measurements come from trigonometric parallax. As the Earth orbits the Sun, the position of nearby stars will appear to shift slightly against the more distant background. These shifts are angles in an isosceles  The motion of the Sun through space provides a longer baseline that will increase the accuracy of parallax measurements, known as secular parallax. For stars in the Milky Way disk, this corresponds to a mean baseline of 4 AU per year, while for halo stars the baseline is 40 AU per year. After several decades, the baseline can be orders of magnitude greater than the Earth–Sun baseline used for traditional parallax. However, secular parallax introduces a higher level of uncertainty because the relative velocity of observed stars is an additional unknown. When applied to samples of multiple stars, the uncertainty can be reduced; the uncertainty is inversely proportional to the
The motion of the Sun through space provides a longer baseline that will increase the accuracy of parallax measurements, known as secular parallax. For stars in the Milky Way disk, this corresponds to a mean baseline of 4 AU per year, while for halo stars the baseline is 40 AU per year. After several decades, the baseline can be orders of magnitude greater than the Earth–Sun baseline used for traditional parallax. However, secular parallax introduces a higher level of uncertainty because the relative velocity of observed stars is an additional unknown. When applied to samples of multiple stars, the uncertainty can be reduced; the uncertainty is inversely proportional to the
"Hubble Space Telescope Fine Guidance Sensor Parallaxes of Galactic Cepheid Variable Stars: Period-Luminosity Relations"
, ''The Astronomical Journal'', Volume 133, Issue 4, pp. 1810–1827 (2007) : Majaess, Daniel; Turner, David; Moni Bidin, Christian; Mauro, Francesco; Geisler, Douglas; Gieren, Wolfgang; Minniti, Dante; Chené, André-Nicolas; Lucas, Philip; Borissova, Jura; Kurtev, Radostn; Dékány, Istvan; Saito, Roberto K
"New Evidence Supporting Membership for TW Nor in Lyngå 6 and the Centaurus Spiral Arm"
, ''ApJ Letters'', Volume 741, Issue 2, article id. L2 (2011) Several problems complicate the use of Cepheids as standard candles and are actively debated, chief among them are: the nature and linearity of the period-luminosity relation in various passbands and the impact of metallicity on both the zero-point and slope of those relations, and the effects of photometric contamination (blending) and a changing (typically unknown) extinction law on Cepheid distances. These unresolved matters have resulted in cited values for the
 There are several different methods for which supernovae can be used to measure extragalactic distances.
There are several different methods for which supernovae can be used to measure extragalactic distances.
 The following method deals with the overall inherent properties of galaxies. These methods, though with varying error percentages, have the ability to make distance estimates beyond 100 Mpc, though it is usually applied more locally.
The
The following method deals with the overall inherent properties of galaxies. These methods, though with varying error percentages, have the ability to make distance estimates beyond 100 Mpc, though it is usually applied more locally.
The
The ABC's of distances (UCLA)
by Bill Keel
The Hubble Space Telescope Key Project on the Extragalactic Distance Scale
The Hubble Constant
a historical discussion
NASA Cosmic Distance Scale
The Astrophysical Journal
{{Authority control Astrometry Physical cosmology Standard candles Length, distance, or range measuring devices Concepts in astronomy
astronomer
An astronomer is a scientist in the field of astronomy who focuses their studies on a specific question or field outside the scope of Earth. They observe astronomical objects such as stars, planets, moons, comets and galaxies – in either ...
s determine the distance
Distance is a numerical or occasionally qualitative measurement of how far apart objects or points are. In physics or everyday usage, distance may refer to a physical length or an estimation based on other criteria (e.g. "two counties over"). ...
s to celestial objects. A ''direct'' distance measurement of an astronomical object is possible only for those objects that are "close enough" (within about a thousand parsec
The parsec (symbol: pc) is a unit of length used to measure the large distances to astronomical objects outside the Solar System, approximately equal to or (au), i.e. . The parsec unit is obtained by the use of parallax and trigonometry, an ...
s) to Earth. The techniques for determining distances to more distant objects are all based on various measured correlations between methods that work at close distances and methods that work at larger distances. Several methods rely on a standard candle, which is an astronomical object that has a known luminosity.
The ladder analogy arises because no single technique can measure distances at all ranges encountered in astronomy. Instead, one method can be used to measure nearby distances, a second can be used to measure nearby to intermediate distances, and so on. Each rung of the ladder provides information that can be used to determine the distances at the next higher rung.
Direct measurement
 At the base of the ladder are ''fundamental'' distance measurements, in which distances are determined directly, with no physical assumptions about the nature of the object in question. The precise measurement of stellar positions is part of the discipline of astrometry.
At the base of the ladder are ''fundamental'' distance measurements, in which distances are determined directly, with no physical assumptions about the nature of the object in question. The precise measurement of stellar positions is part of the discipline of astrometry.
Astronomical unit
Direct distance measurements are based upon theastronomical unit
The astronomical unit (symbol: au, or or AU) is a unit of length, roughly the distance from Earth to the Sun and approximately equal to or 8.3 light-minutes. The actual distance from Earth to the Sun varies by about 3% as Earth orbits ...
(AU), which is defined as the mean distance between the Earth
Earth is the third planet from the Sun and the only astronomical object known to harbor life. While large volumes of water can be found throughout the Solar System, only Earth sustains liquid surface water. About 71% of Earth's surfa ...
and the Sun
The Sun is the star at the center of the Solar System. It is a nearly perfect ball of hot plasma, heated to incandescence by nuclear fusion reactions in its core. The Sun radiates this energy mainly as light, ultraviolet, and infrared radi ...
.
Kepler's laws
In astronomy, Kepler's laws of planetary motion, published by Johannes Kepler between 1609 and 1619, describe the orbits of planets around the Sun. The laws modified the heliocentric theory of Nicolaus Copernicus, replacing its circular orbi ...
provide precise ratio
In mathematics, a ratio shows how many times one number contains another. For example, if there are eight oranges and six lemons in a bowl of fruit, then the ratio of oranges to lemons is eight to six (that is, 8:6, which is equivalent to the ...
s of the sizes of the orbits of objects orbiting the Sun, but provide no measurement of the overall scale of the orbit system. Radar
Radar is a detection system that uses radio waves to determine the distance ('' ranging''), angle, and radial velocity of objects relative to the site. It can be used to detect aircraft, ships, spacecraft, guided missiles, motor vehicles, we ...
is used to measure the distance between the orbits of the Earth and of a second body. From that measurement and the ratio of the two orbit sizes, the size of Earth's orbit is calculated. The Earth's orbit is known with an absolute precision of a few meters and a relative precision of a few parts in 100 billion ().
Historically, observations of transits of Venus
frameless, upright=0.5
A transit of Venus across the Sun takes place when the planet Venus passes directly between the Sun and a superior planet, becoming visible against (and hence obscuring a small portion of) the solar disk. During a tran ...
were crucial in determining the AU; in the first half of the 20th century, observations of asteroids
An asteroid is a minor planet of the inner Solar System. Sizes and shapes of asteroids vary significantly, ranging from 1-meter rocks to a dwarf planet almost 1000 km in diameter; they are rocky, metallic or icy bodies with no atmosphere. ...
were also important. Presently the orbit of Earth is determined with high precision using radar
Radar is a detection system that uses radio waves to determine the distance ('' ranging''), angle, and radial velocity of objects relative to the site. It can be used to detect aircraft, ships, spacecraft, guided missiles, motor vehicles, we ...
measurements of distances to Venus
Venus is the second planet from the Sun. It is sometimes called Earth's "sister" or "twin" planet as it is almost as large and has a similar composition. As an interior planet to Earth, Venus (like Mercury) appears in Earth's sky never f ...
and other nearby planets and asteroids, and by tracking interplanetary spacecraft
A spacecraft is a vehicle or machine designed to fly in outer space. A type of artificial satellite, spacecraft are used for a variety of purposes, including communications, Earth observation, meteorology, navigation, space colonization, p ...
in their orbits around the Sun through the Solar System
The Solar System Capitalization of the name varies. The International Astronomical Union, the authoritative body regarding astronomical nomenclature, specifies capitalizing the names of all individual astronomical objects but uses mixed "Solar ...
.
Parallax
 The most important fundamental distance measurements come from trigonometric parallax. As the Earth orbits the Sun, the position of nearby stars will appear to shift slightly against the more distant background. These shifts are angles in an isosceles
The most important fundamental distance measurements come from trigonometric parallax. As the Earth orbits the Sun, the position of nearby stars will appear to shift slightly against the more distant background. These shifts are angles in an isosceles triangle
A triangle is a polygon with three edges and three vertices. It is one of the basic shapes in geometry. A triangle with vertices ''A'', ''B'', and ''C'' is denoted \triangle ABC.
In Euclidean geometry, any three points, when non- colline ...
, with 2 AU (the distance between the extreme positions of Earth's orbit around the Sun) making the base leg of the triangle and the distance to the star being the long equal length legs. The amount of shift is quite small, even for the nearest stars, measuring 1 arcsecond for an object at 1 parsec
The parsec (symbol: pc) is a unit of length used to measure the large distances to astronomical objects outside the Solar System, approximately equal to or (au), i.e. . The parsec unit is obtained by the use of parallax and trigonometry, an ...
's distance (3.26 light-years), and thereafter decreasing in angular amount as the distance increases. Astronomers usually express distances in units of parsecs (parallax arcseconds); light-years are used in popular media.
Because parallax becomes smaller for a greater stellar distance, useful distances can be measured only for stars which are near enough to have a parallax larger than a few times the precision
Precision, precise or precisely may refer to:
Science, and technology, and mathematics Mathematics and computing (general)
* Accuracy and precision, measurement deviation from true value and its scatter
* Significant figures, the number of digit ...
of the measurement. In the 1990s, for example, the Hipparcos mission obtained parallaxes for over a hundred thousand stars with a precision of about a milliarcsecond
A minute of arc, arcminute (arcmin), arc minute, or minute arc, denoted by the symbol , is a unit of angular measurement equal to of one degree. Since one degree is of a turn (or complete rotation), one minute of arc is of a turn. The n ...
, providing useful distances for stars out to a few hundred parsecs. The Hubble telescope WFC3 now has the potential to provide a precision of 20 to 40 ''micro''arcseconds, enabling reliable distance measurements up to for small numbers of stars. In 2018, Data Release 2 from the Gaia space mission provides similarly accurate distances to most stars brighter than 15th magnitude.
Stars have a velocity relative to the Sun that causes proper motion (transverse across the sky) and radial velocity (motion toward or away from the Sun). The former is determined by plotting the changing position of the stars over many years, while the latter comes from measuring the Doppler shift of the star's spectrum caused by motion along the line of sight. For a group of stars with the same spectral class and a similar magnitude range, a mean parallax can be derived from statistical analysis of the proper motions relative to their radial velocities. This statistical parallax
The most important fundamental distance measurements in astronomy come from trigonometric parallax. As the Earth orbits the Sun, the position of nearby stars will appear to shift slightly against the more distant background. These shifts are ang ...
method is useful for measuring the distances of bright stars beyond 50 parsecs and giant variable star
A variable star is a star whose brightness as seen from Earth (its apparent magnitude) changes with time. This variation may be caused by a change in emitted light or by something partly blocking the light, so variable stars are classified as e ...
s, including Cepheids and the RR Lyrae variables.
 The motion of the Sun through space provides a longer baseline that will increase the accuracy of parallax measurements, known as secular parallax. For stars in the Milky Way disk, this corresponds to a mean baseline of 4 AU per year, while for halo stars the baseline is 40 AU per year. After several decades, the baseline can be orders of magnitude greater than the Earth–Sun baseline used for traditional parallax. However, secular parallax introduces a higher level of uncertainty because the relative velocity of observed stars is an additional unknown. When applied to samples of multiple stars, the uncertainty can be reduced; the uncertainty is inversely proportional to the
The motion of the Sun through space provides a longer baseline that will increase the accuracy of parallax measurements, known as secular parallax. For stars in the Milky Way disk, this corresponds to a mean baseline of 4 AU per year, while for halo stars the baseline is 40 AU per year. After several decades, the baseline can be orders of magnitude greater than the Earth–Sun baseline used for traditional parallax. However, secular parallax introduces a higher level of uncertainty because the relative velocity of observed stars is an additional unknown. When applied to samples of multiple stars, the uncertainty can be reduced; the uncertainty is inversely proportional to the square root
In mathematics, a square root of a number is a number such that ; in other words, a number whose ''square'' (the result of multiplying the number by itself, or ⋅ ) is . For example, 4 and −4 are square roots of 16, because .
...
of the sample size.
Moving cluster parallax is a technique where the motions of individual stars in a nearby star cluster can be used to find the distance to the cluster. Only open cluster
An open cluster is a type of star cluster made of up to a few thousand stars that were formed from the same giant molecular cloud and have roughly the same age. More than 1,100 open clusters have been discovered within the Milky Way galaxy, an ...
s are near enough for this technique to be useful. In particular the distance obtained for the Hyades Hyades may refer to:
* Hyades (band)
*Hyades (mythology)
*Hyades (star cluster)
The Hyades (; Greek Ὑάδες, also known as Caldwell 41, Collinder 50, or Melotte 25) is the nearest open cluster and one of the best-studied star clusters. Loca ...
has historically been an important step in the distance ladder.
Other individual objects can have fundamental distance estimates made for them under special circumstances. If the expansion of a gas cloud, like a supernova remnant
A supernova remnant (SNR) is the structure resulting from the explosion of a star in a supernova. The supernova remnant is bounded by an expanding shock wave, and consists of ejected material expanding from the explosion, and the interstellar mat ...
or planetary nebula
A planetary nebula (PN, plural PNe) is a type of emission nebula consisting of an expanding, glowing shell of ionized gas ejected from red giant stars late in their lives.
The term "planetary nebula" is a misnomer because they are unrelate ...
, can be observed over time, then an ''expansion parallax'' distance to that cloud can be estimated. Those measurements however suffer from uncertainties in the deviation of the object from sphericity. Binary stars which are both visual
The visual system comprises the sensory organ (the eye) and parts of the central nervous system (the retina containing photoreceptor cells, the optic nerve, the optic tract and the visual cortex) which gives organisms the sense of sight (th ...
and spectroscopic
Spectroscopy is the field of study that measures and interprets the electromagnetic spectra that result from the interaction between electromagnetic radiation and matter as a function of the wavelength or frequency of the radiation. Matter wa ...
binaries also can have their distance estimated by similar means, and don't suffer from the above geometric uncertainty. The common characteristic to these methods is that a measurement of angular motion is combined with a measurement of the absolute velocity
Velocity is the directional speed of an object in motion as an indication of its rate of change in position as observed from a particular frame of reference and as measured by a particular standard of time (e.g. northbound). Velocity i ...
(usually obtained via the Doppler effect). The distance estimate comes from computing how far the object must be to make its observed absolute velocity appear with the observed angular motion.
Expansion parallaxes in particular can give fundamental distance estimates for objects that are very far, because supernova ejecta have large expansion velocities and large sizes (compared to stars). Further, they can be observed with radio interferometers which can measure very small angular motions. These combine to provide fundamental distance estimates to supernovae in other galaxies. Though valuable, such cases are quite rare, so they serve as important consistency checks on the distance ladder rather than workhorse steps by themselves.
Standard candles
Almost all astronomical objects used as physical distance indicators belong to a class that has a known brightness. By comparing this known luminosity to an object's observed brightness, the distance to the object can be computed using the inverse-square law. These objects of known brightness are termed standard candles, coined byHenrietta Swan Leavitt
Henrietta Swan Leavitt (; July 4, 1868 – December 12, 1921) was an American astronomer. A graduate of Radcliffe College, she worked at the Harvard College Observatory as a "computer", tasked with examining photographic plates in order to measu ...
.
The brightness of an object can be expressed in terms of its absolute magnitude
Absolute magnitude () is a measure of the luminosity of a celestial object on an inverse logarithmic astronomical magnitude scale. An object's absolute magnitude is defined to be equal to the apparent magnitude that the object would have if it ...
. This quantity is derived from the logarithm of its luminosity as seen from a distance of 10 parsec
The parsec (symbol: pc) is a unit of length used to measure the large distances to astronomical objects outside the Solar System, approximately equal to or (au), i.e. . The parsec unit is obtained by the use of parallax and trigonometry, an ...
s. The apparent magnitude
Apparent magnitude () is a measure of the brightness of a star or other astronomical object observed from Earth. An object's apparent magnitude depends on its intrinsic luminosity, its distance from Earth, and any extinction of the object's ...
, the magnitude as seen by the observer (an instrument called a bolometer
A bolometer is a device for measuring radiant heat by means of a material having a temperature-dependent electrical resistance. It was invented in 1878 by the American astronomer Samuel Pierpont Langley.
Principle of operation
A bolometer ...
is used), can be measured and used with the absolute magnitude to calculate the distance ''d'' to the object in parsecs as follows:
:
or
:
where ''m'' is the apparent magnitude, and ''M'' the absolute magnitude. For this to be accurate, both magnitudes must be in the same frequency band and there can be no relative motion in the radial direction.
Some means of correcting for interstellar extinction
Extinction is the termination of a kind of organism or of a group of kinds (taxon), usually a species. The moment of extinction is generally considered to be the death of the last individual of the species, although the capacity to breed and ...
, which also makes objects appear fainter and more red, is needed, especially if the object lies within a dusty or gaseous region. The difference between an object's absolute and apparent magnitudes is called its distance modulus The distance modulus is a way of expressing distances that is often used in astronomy. It describes distances on a logarithmic scale based on the astronomical magnitude system.
Definition
The distance modulus \mu=m-M is the difference between th ...
, and astronomical distances, especially intergalactic ones, are sometimes tabulated in this way.
Problems
Two problems exist for any class of standard candle. The principal one iscalibration
In measurement technology and metrology, calibration is the comparison of measurement values delivered by a device under test with those of a calibration standard of known accuracy. Such a standard could be another measurement device of kno ...
, that is the determination of exactly what the absolute magnitude of the candle is. This includes defining the class well enough that members can be recognized, and finding enough members of that class with well-known distances to allow their true absolute magnitude to be determined with enough accuracy. The second problem lies in recognizing members of the class, and not mistakenly using a standard candle calibration on an object which does not belong to the class. At extreme distances, which is where one most wishes to use a distance indicator, this recognition problem can be quite serious.
A significant issue with standard candles is the recurring question of how standard they are. For example, all observations seem to indicate that Type Ia supernova
A Type Ia supernova (read: "type one-A") is a type of supernova that occurs in binary systems (two stars orbiting one another) in which one of the stars is a white dwarf. The other star can be anything from a giant star to an even smaller white ...
e that are of known distance have the same brightness (corrected by the shape of the light curve). The basis for this closeness in brightness is discussed below; however, the possibility exists that the distant Type Ia supernovae have different properties than nearby Type Ia supernovae. The use of Type Ia supernovae is crucial in determining the correct cosmological model. If indeed the properties of Type Ia supernovae are different at large distances, i.e. if the extrapolation of their calibration to arbitrary distances is not valid, ignoring this variation can dangerously bias the reconstruction of the cosmological parameters, in particular the reconstruction of the matter density parameter
The Friedmann equations are a set of equations in physical cosmology that govern the expansion of space in homogeneous and isotropic models of the universe within the context of general relativity. They were first derived by Alexander Friedma ...
.
(And references therein.)
That this is not merely a philosophical issue can be seen from the history of distance measurements using Cepheid variable
A Cepheid variable () is a type of star that pulsates radially, varying in both diameter and temperature and producing changes in brightness with a well-defined stable period and amplitude.
A strong direct relationship between a Cepheid vari ...
s. In the 1950s, Walter Baade
Wilhelm Heinrich Walter Baade (March 24, 1893 – June 25, 1960) was a German astronomer who worked in the United States from 1931 to 1959.
Biography
The son of a teacher, Baade finished school in 1912. He then studied maths, physics and astr ...
discovered that the nearby Cepheid variables used to calibrate the standard candle were of a different type than the ones used to measure distances to nearby galaxies. The nearby Cepheid variables were population I
During 1944, Walter Baade categorized groups of stars within the Milky Way into stellar populations.
In the abstract of the article by Baade, he recognizes that Jan Oort originally conceived this type of classification in 1926:
Baade noticed th ...
stars with much higher metal
A metal (from Greek μέταλλον ''métallon'', "mine, quarry, metal") is a material that, when freshly prepared, polished, or fractured, shows a lustrous appearance, and conducts electricity and heat relatively well. Metals are typicall ...
content than the distant population II
During 1944, Walter Baade categorized groups of stars within the Milky Way into stellar populations.
In the abstract of the article by Baade, he recognizes that Jan Oort originally conceived this type of classification in 1926:
Baade noticed ...
stars. As a result, the population II stars were actually much brighter than believed, and when corrected, this had the effect of doubling the distances to the globular clusters, the nearby galaxies, and the diameter of the Milky Way
The Milky Way is the galaxy that includes our Solar System, with the name describing the galaxy's appearance from Earth: a hazy band of light seen in the night sky formed from stars that cannot be individually distinguished by the naked eye. ...
.
Standard siren
Gravitational waves originating from the inspiral phase of compact binary systems, such asneutron star
A neutron star is the collapsed core of a massive supergiant star, which had a total mass of between 10 and 25 solar masses, possibly more if the star was especially metal-rich. Except for black holes and some hypothetical objects (e.g. w ...
s or black holes, have the useful property that energy emitted as gravitational radiation comes exclusively from the orbital energy
In the gravitational two-body problem, the specific orbital energy \varepsilon (or vis-viva energy) of two orbiting bodies is the constant sum of their mutual potential energy (\varepsilon_p) and their total kinetic energy (\varepsilon_k), divid ...
of the pair, and the resultant shrinking of their orbits is directly observable as an increase in the frequency of the emitted gravitational waves. To leading order, the rate of change of frequency is given by
:
where is the gravitational constant, is the speed of light
The speed of light in vacuum, commonly denoted , is a universal physical constant that is important in many areas of physics. The speed of light is exactly equal to ). According to the special theory of relativity, is the upper limit ...
, and is a single (therefore computable) number called the chirp mass In astrophysics the chirp mass of a compact binary system determines the leading-order orbital evolution of the system as a result of energy loss from emitting gravitational waves
Gravitational waves are waves of the intensity of gravity genera ...
of the system, a combination of the masses of the two objects
:
By observing the waveform, the chirp mass can be computed and thence the power
Power most often refers to:
* Power (physics), meaning "rate of doing work"
** Engine power, the power put out by an engine
** Electric power
* Power (social and political), the ability to influence people or events
** Abusive power
Power may a ...
(rate of energy emission) of the gravitational waves. Thus, such a gravitational wave source is a standard siren of known loudness.
Just as with standard candles, given the emitted and received amplitudes, the inverse-square law determines the distance to the source. There are some differences with standard candles, however. Gravitational waves are not emitted isotropically, but measuring the polarisation of the wave provides enough information to determine the angle of emission. Gravitational wave detectors also have anisotropic antenna patterns, so the position of the source on the sky relative to the detectors is needed to determine the angle of reception. Generally, if a wave is detected by a network of three detectors at different locations, the network will measure enough information to make these corrections and obtain the distance. Also unlike standard candles, gravitational waves need no calibration against other distance measures. The measurement of distance does of course require the calibration of the gravitational wave detectors, but then the distance is fundamentally given as a multiple of the wavelength of the laser light being used in the gravitational wave interferometer.
There are other considerations that limit the accuracy of this distance, besides detector calibration. Fortunately, gravitational waves are not subject to extinction
Extinction is the termination of a kind of organism or of a group of kinds (taxon), usually a species. The moment of extinction is generally considered to be the death of the last individual of the species, although the capacity to breed and ...
due to an intervening absorbing medium. But they ''are'' subject to gravitational lens
A gravitational lens is a distribution of matter (such as a cluster of galaxies) between a distant light source and an observer that is capable of bending the light from the source as the light travels toward the observer. This effect is known ...
ing, in the same way as light. If a signal is strongly lensed, then it might be received as multiple events, separated in time (the analogue of multiple images of a quasar, for example). Less easy to discern and control for is the effect of weak lensing, where the signal's path through space is affected by many small magnification and demagnification events. This will be important for signals originating at cosmological redshifts greater than 1. Finally, it is difficult for detector networks to measure the polarization of a signal accurately if the binary system is observed nearly face-on; such signals suffer significantly larger errors in the distance measurement. Unfortunately, binaries radiate most strongly perpendicular to the orbital plane, so face-on signals are intrinsically stronger and the most commonly observed.
If the binary consists of a pair of neutron stars, their merger will be accompanied by a kilonova
A kilonova (also called a macronova) is a transient astronomical event that occurs in a compact binary system when two neutron stars or a neutron star and a black hole merge. These mergers are thought to produce gamma-ray bursts and emit bright e ...
/hypernova
A hypernova (sometimes called a collapsar) is a very energetic supernova thought to result from an extreme core-collapse scenario. In this case, a massive star (>30 solar masses) collapses to form a rotating black hole emitting twin energetic je ...
explosion that may allow the position to be accurately identified by electromagnetic telescopes. In such cases, the redshift of the host galaxy allows a determination of the Hubble constant
Hubble's law, also known as the Hubble–Lemaître law, is the observation in physical cosmology that galaxies are moving away from Earth at speeds proportional to their distance. In other words, the farther they are, the faster they are moving ...
. This was the case for GW170817
GW 170817 was a gravitational wave (GW) signal observed by the LIGO and Virgo detectors on 17 August 2017, originating from the shell elliptical galaxy . The signal was produced by the last minutes of a binary pair of neutron stars' insp ...
, which was used to make the first such measurement. Even if no electromagnetic counterpart can be identified for an ensemble of signals, it is possible to use a statistical method to infer the value of .
Standard ruler
Another class of physical distance indicator is the standard ruler. In 2008, galaxy diameters have been proposed as a possible standard ruler for cosmological parameter determination. More recently the physical scale imprinted bybaryon acoustic oscillations
In cosmology, baryon acoustic oscillations (BAO) are fluctuations in the density of the visible baryonic matter (normal matter) of the universe, caused by acoustic density waves in the primordial plasma of the early universe. In the same way ...
(BAO) in the early universe has been used.
In the early universe (before recombination) the baryons and photons scatter off each other, and form a tightly-coupled fluid that can support sound waves. The waves are sourced by primordial density perturbations, and travel at speed that can be predicted from the baryon density and other cosmological parameters. The total distance that these sound waves can travel before recombination determines a fixed scale, which simply expands with the universe after recombination. BAO therefore provide a standard ruler that can be measured in galaxy surveys from the effect of baryons on the clustering of galaxies. The method requires an extensive galaxy survey in order to make this scale visible, but has been measured with percent-level precision (see baryon acoustic oscillations
In cosmology, baryon acoustic oscillations (BAO) are fluctuations in the density of the visible baryonic matter (normal matter) of the universe, caused by acoustic density waves in the primordial plasma of the early universe. In the same way ...
). The scale does depend on cosmological parameters like the baryon and matter densities, and the number of neutrinos, so distances based on BAO are more dependent on cosmological model than those based on local measurements.
Light echo
309x309px, Reflected light following path B arrives shortly after the direct flash following path A but before light following path C. B and C have the same apparent distance from the star as seen from Earth.">Earth.html" ;"title="apparent distan ...
s can be also used as standard rulers, although it is challenging to correctly measure the source geometry.
Galactic distance indicators
With few exceptions, distances based on direct measurements are available only out to about a thousand parsecs, which is a modest portion of our own Galaxy. For distances beyond that, measures depend upon physical assumptions, that is, the assertion that one recognizes the object in question, and the class of objects is homogeneous enough that its members can be used for meaningful estimation of distance. Physical distance indicators, used on progressively larger distance scales, include: *Dynamical parallax
In astronomy, the distance to a visual binary star may be estimated from the masses of its two components, the size of their orbit, and the period of their orbit about one another. A dynamical parallax is an (annual) parallax which is computed fr ...
, uses orbital parameters of visual binaries to measure the mass of the system, and hence use the mass–luminosity relation In astrophysics, the mass–luminosity relation is an equation giving the relationship between a star's mass and its luminosity, first noted by Jakob Karl Ernst Halm. The relationship is represented by the equation:
:\frac = \left(\frac\right)^a
...
to determine the luminosity
**Eclipsing binaries
A binary star is a system of two stars that are gravitationally bound to and in orbit around each other. Binary stars in the night sky that are seen as a single object to the naked eye are often resolved using a telescope as separate stars, in wh ...
— In the last decade, measurement of eclipsing binaries' fundamental parameters has become possible with 8-meter class telescopes. This makes it feasible to use them as indicators of distance. Recently, they have been used to give direct distance estimates to the Large Magellanic Cloud (LMC), Small Magellanic Cloud (SMC), Andromeda Galaxy
The Andromeda Galaxy (IPA: ), also known as Messier 31, M31, or NGC 224 and originally the Andromeda Nebula, is a barred spiral galaxy with the diameter of about approximately from Earth and the nearest large galaxy to the Milky Way. The gal ...
and Triangulum Galaxy
The Triangulum Galaxy is a spiral galaxy 2.73 million light-years (ly) from Earth in the constellation Triangulum. It is catalogued as Messier 33 or NGC (''New General Catalogue)'' 598. With the D25 isophotal diameter of , the Triangulum Ga ...
. Eclipsing binaries offer a direct method to gauge the distance to galaxies to a new improved 5% level of accuracy which is feasible with current technology to a distance of around 3 Mpc (3 million parsecs).
* RR Lyrae variables — used for measuring distances within the galaxy and in nearby globular cluster
A globular cluster is a spheroidal conglomeration of stars. Globular clusters are bound together by gravity, with a higher concentration of stars towards their centers. They can contain anywhere from tens of thousands to many millions of membe ...
s.
* The following four indicators all use stars in the old stellar populations (Population II
During 1944, Walter Baade categorized groups of stars within the Milky Way into stellar populations.
In the abstract of the article by Baade, he recognizes that Jan Oort originally conceived this type of classification in 1926:
Baade noticed ...
):
** Tip of the red-giant branch
Tip of the red-giant branch (TRGB) is a primary distance indicator used in astronomy. It uses the luminosity of the brightest red-giant-branch stars in a galaxy as a standard candle to gauge the distance to that galaxy. It has been used in conjun ...
(TRGB) distance indicator.
** Planetary nebula luminosity function
Planetary nebula luminosity function (PNLF) is a secondary distance indicator used in astronomy. It makes use of the IIIλ5007 forbidden line found in all planetary nebula (PNe) which are members of the old stellar populations ( Populati ...
(PNLF)
** Globular cluster luminosity function
A globular cluster is a spheroidal conglomeration of stars. Globular clusters are bound together by gravity, with a higher concentration of stars towards their centers. They can contain anywhere from tens of thousands to many millions of memb ...
(GCLF)
** Surface brightness fluctuation
Surface brightness fluctuation (SBF) is a secondary distance indicator used to estimate distances to galaxies. It is useful to 100 Mpc (parsec). The method measures the variance in a galaxy's light distribution arising from fluctuations in the n ...
(SBF)
* In galactic astronomy, X-ray burst
An X-ray, or, much less commonly, X-radiation, is a penetrating form of high-energy electromagnetic radiation. Most X-rays have a wavelength ranging from 10 picometers to 10 nanometers, corresponding to frequencies in the range 30 ...
s (thermonuclear flashes on the surface of a neutron star
A neutron star is the collapsed core of a massive supergiant star, which had a total mass of between 10 and 25 solar masses, possibly more if the star was especially metal-rich. Except for black holes and some hypothetical objects (e.g. w ...
) are used as standard candles. Observations of X-ray burst sometimes show X-ray spectra indicating radius expansion. Therefore, the X-ray flux at the peak of the burst should correspond to Eddington luminosity, which can be calculated once the mass of the neutron star is known (1.5 solar masses is a commonly used assumption). This method allows distance determination of some low-mass X-ray binaries
X-ray binaries are a class of binary stars that are luminous in X-rays.
The X-rays are produced by matter falling from one component, called the ''donor'' (usually a relatively normal star), to the other component, called the ''accretor'', which ...
. Low-mass X-ray binaries are very faint in the optical, making their distances extremely difficult to determine.
* Interstellar masers can be used to derive distances to galactic and some extragalactic objects that have maser emission.
* Cepheids and novae
* The Tully–Fisher relation
In astronomy, the Tully–Fisher relation (TFR) is an empirical relationship between the mass or intrinsic luminosity of a spiral galaxy and its asymptotic rotation velocity or emission line width. It was first published in 1977 by astronomer ...
* The Faber–Jackson relation
The Faber–Jackson relation provided the first empirical power-law relation between the luminosity L and the central stellar velocity dispersion \sigma of elliptical galaxy, and was presented by the astronomers Sandra M. Faber and Robert Earl ...
* Type Ia supernova
A Type Ia supernova (read: "type one-A") is a type of supernova that occurs in binary systems (two stars orbiting one another) in which one of the stars is a white dwarf. The other star can be anything from a giant star to an even smaller white ...
e that have a very well-determined maximum absolute magnitude as a function of the shape of their light curve
In astronomy, a light curve is a graph of light intensity of a celestial object or region as a function of time, typically with the magnitude of light received on the y axis and with time on the x axis. The light is usually in a particular frequ ...
and are useful in determining extragalactic distances up to a few hundred Mpc. A notable exception is SN 2003fg
SN 2003fg, nicknamed the Champagne Supernova, was an unusual Type Ia supernova. It was discovered in 2003, with the Canada-France-Hawaii Telescope and the Keck Telescope, both on Mauna Kea in Hawaii, and announced by researchers at the Univ ...
, the "Champagne Supernova", a Type Ia supernova of unusual nature.
* Redshifts and Hubble's law
Hubble's law, also known as the Hubble–Lemaître law, is the observation in physical cosmology that galaxies are moving away from Earth at speeds proportional to their distance. In other words, the farther they are, the faster they are moving a ...
Main sequence fitting
When the absolute magnitude for a group of stars is plotted against thespectral classification
In astronomy, stellar classification is the classification of stars based on their spectral characteristics. Electromagnetic radiation from the star is analyzed by splitting it with a prism or diffraction grating into a spectrum exhibiting the ...
of the star, in a Hertzsprung–Russell diagram, evolutionary patterns are found that relate to the mass, age and composition of the star. In particular, during their hydrogen burning period, stars lie along a curve in the diagram called the main sequence. By measuring these properties from a star's spectrum, the position of a main sequence star on the H–R diagram can be determined, and thereby the star's absolute magnitude estimated. A comparison of this value with the apparent magnitude allows the approximate distance to be determined, after correcting for interstellar extinction
Extinction is the termination of a kind of organism or of a group of kinds (taxon), usually a species. The moment of extinction is generally considered to be the death of the last individual of the species, although the capacity to breed and ...
of the luminosity because of gas and dust.
In a gravitationally-bound star cluster such as the Hyades Hyades may refer to:
* Hyades (band)
*Hyades (mythology)
*Hyades (star cluster)
The Hyades (; Greek Ὑάδες, also known as Caldwell 41, Collinder 50, or Melotte 25) is the nearest open cluster and one of the best-studied star clusters. Loca ...
, the stars formed at approximately the same age and lie at the same distance. This allows relatively accurate main sequence fitting, providing both age and distance determination.
Extragalactic distance scale
The extragalactic distance scale is a series of techniques used today by astronomers to determine the distance of cosmological bodies beyond our own galaxy, which are not easily obtained with traditional methods. Some procedures utilize properties of these objects, such as stars,globular cluster
A globular cluster is a spheroidal conglomeration of stars. Globular clusters are bound together by gravity, with a higher concentration of stars towards their centers. They can contain anywhere from tens of thousands to many millions of membe ...
s, nebulae, and galaxies as a whole. Other methods are based more on the statistics and probabilities of things such as entire galaxy cluster
A galaxy cluster, or a cluster of galaxies, is a structure that consists of anywhere from hundreds to thousands of galaxies that are bound together by gravity, with typical masses ranging from 1014 to 1015 solar masses. They are the second-lar ...
s.
Wilson–Bappu effect
Discovered in 1956 by Olin Wilson and M.K. Vainu Bappu, theWilson–Bappu effect
The Ca II K line in cool stars is among the strongest emission lines which originates in the star's chromosphere. In 1957, Olin C. Wilson and M. K. Vainu Bappu reported on the remarkable correlation between the measured width of the aforemen ...
utilizes the effect known as spectroscopic parallax Spectroscopic parallax or main sequence fitting is an astronomical method for measuring the distances to stars.
Despite its name, it does not rely on the geometric parallax effect. The spectroscopic parallax technique can be applied to any main se ...
. Many stars have features in their spectra, such as the calcium K-line
In physics and optics, the Fraunhofer lines are a set of spectral absorption lines named after the German physicist Joseph von Fraunhofer (1787–1826). The lines were originally observed as dark features (absorption lines) in the optical spectru ...
, that indicate their absolute magnitude
Absolute magnitude () is a measure of the luminosity of a celestial object on an inverse logarithmic astronomical magnitude scale. An object's absolute magnitude is defined to be equal to the apparent magnitude that the object would have if it ...
. The distance to the star can then be calculated from its apparent magnitude
Apparent magnitude () is a measure of the brightness of a star or other astronomical object observed from Earth. An object's apparent magnitude depends on its intrinsic luminosity, its distance from Earth, and any extinction of the object's ...
using the distance modulus The distance modulus is a way of expressing distances that is often used in astronomy. It describes distances on a logarithmic scale based on the astronomical magnitude system.
Definition
The distance modulus \mu=m-M is the difference between th ...
.
There are major limitations to this method for finding stellar distances. The calibration of the spectral line strengths has limited accuracy and it requires a correction for interstellar extinction
In astronomy, extinction is the absorption and scattering of electromagnetic radiation by dust and gas between an emitting astronomical object and the observer. Interstellar extinction was first documented as such in 1930 by Robert Julius Trump ...
. Though in theory this method has the ability to provide reliable distance calculations to stars up to 7 megaparsecs (Mpc), it is generally only used for stars at hundreds of kiloparsecs (kpc).
Classical Cepheids
Beyond the reach of theWilson–Bappu effect
The Ca II K line in cool stars is among the strongest emission lines which originates in the star's chromosphere. In 1957, Olin C. Wilson and M. K. Vainu Bappu reported on the remarkable correlation between the measured width of the aforemen ...
, the next method relies on the period-luminosity relation
In astronomy, a period-luminosity relation is a relationship linking the luminosity of pulsating variable stars with their pulsation period.
The best-known relation is the direct proportionality law holding for Classical Cepheid variables, sometim ...
of classical Cepheid variable
A Cepheid variable () is a type of star that pulsates radially, varying in both diameter and temperature and producing changes in brightness with a well-defined stable period and amplitude.
A strong direct relationship between a Cepheid vari ...
stars. The following relation can be used to calculate the distance to Galactic and extragalactic classical Cepheids:
: Benedict, G. Fritz et al"Hubble Space Telescope Fine Guidance Sensor Parallaxes of Galactic Cepheid Variable Stars: Period-Luminosity Relations"
, ''The Astronomical Journal'', Volume 133, Issue 4, pp. 1810–1827 (2007) : Majaess, Daniel; Turner, David; Moni Bidin, Christian; Mauro, Francesco; Geisler, Douglas; Gieren, Wolfgang; Minniti, Dante; Chené, André-Nicolas; Lucas, Philip; Borissova, Jura; Kurtev, Radostn; Dékány, Istvan; Saito, Roberto K
"New Evidence Supporting Membership for TW Nor in Lyngå 6 and the Centaurus Spiral Arm"
, ''ApJ Letters'', Volume 741, Issue 2, article id. L2 (2011) Several problems complicate the use of Cepheids as standard candles and are actively debated, chief among them are: the nature and linearity of the period-luminosity relation in various passbands and the impact of metallicity on both the zero-point and slope of those relations, and the effects of photometric contamination (blending) and a changing (typically unknown) extinction law on Cepheid distances. These unresolved matters have resulted in cited values for the
Hubble constant
Hubble's law, also known as the Hubble–Lemaître law, is the observation in physical cosmology that galaxies are moving away from Earth at speeds proportional to their distance. In other words, the farther they are, the faster they are moving ...
ranging between 60 km/s/Mpc and 80 km/s/Mpc. Resolving this discrepancy is one of the foremost problems in astronomy since some cosmological parameters of the Universe may be constrained significantly better by supplying a precise value of the Hubble constant.
Cepheid variable stars were the key instrument in Edwin Hubble's 1923 conclusion that M31 (Andromeda) was an external galaxy, as opposed to a smaller nebula within the Milky Way
The Milky Way is the galaxy that includes our Solar System, with the name describing the galaxy's appearance from Earth: a hazy band of light seen in the night sky formed from stars that cannot be individually distinguished by the naked eye. ...
. He was able to calculate the distance of M31 to 285 Kpc, today's value being 770 Kpc.
As detected thus far, NGC 3370, a spiral galaxy in the constellation Leo, contains the farthest Cepheids yet found at a distance of 29 Mpc. Cepheid variable stars are in no way perfect distance markers: at nearby galaxies they have an error of about 7% and up to a 15% error for the most distant.
Supernovae
 There are several different methods for which supernovae can be used to measure extragalactic distances.
There are several different methods for which supernovae can be used to measure extragalactic distances.
Measuring a supernova's photosphere
We can assume that a supernova expands in a spherically symmetric manner. If the supernova is close enough such that we can measure the angular extent, ''θ''(''t''), of its photosphere, we can use the equation : where ''ω'' is angular velocity, ''θ'' is angular extent. In order to get an accurate measurement, it is necessary to make two observations separated by time Δ''t''. Subsequently, we can use : where d is the distance to the supernova, ''Vej'' is the supernova's ejecta's radial velocity (it can be assumed that ''Vej'' equals ''Vθ'' if spherically symmetric). This method works only if the supernova is close enough to be able to measure accurately the photosphere. Similarly, the expanding shell of gas is in fact not perfectly spherical nor a perfect blackbody. Also interstellar extinction can hinder the accurate measurements of the photosphere. This problem is further exacerbated by core-collapse supernova. All of these factors contribute to the distance error of up to 25%.Type Ia light curves
Type Ia supernovae are some of the best ways to determine extragalactic distances. Ia's occur when a binary white dwarf star begins to accrete matter from its companion star. As the white dwarf gains matter, eventually it reaches itsChandrasekhar limit
The Chandrasekhar limit () is the maximum mass of a stable white dwarf star. The currently accepted value of the Chandrasekhar limit is about ().
White dwarfs resist gravitational collapse primarily through electron degeneracy pressure, compa ...
of .
Once reached, the star becomes unstable and undergoes a runaway nuclear fusion reaction. Because all Type Ia supernovae explode at about the same mass, their absolute magnitudes are all the same. This makes them very useful as standard candles. All Type Ia supernovae have a standard blue and visual magnitude of
:
Therefore, when observing a Type Ia supernova, if it is possible to determine what its peak magnitude was, then its distance can be calculated. It is not intrinsically necessary to capture the supernova directly at its peak magnitude; using the multicolor light curve shape method (MLCS), the shape of the light curve (taken at any reasonable time after the initial explosion) is compared to a family of parameterized curves that will determine the absolute magnitude at the maximum brightness. This method also takes into effect interstellar extinction/dimming from dust and gas.
Similarly, the stretch method fits the particular supernovae magnitude light curves to a template light curve. This template, as opposed to being several light curves at different wavelengths (MLCS) is just a single light curve that has been stretched (or compressed) in time. By using this ''Stretch Factor'', the peak magnitude can be determined.
Using Type Ia supernovae is one of the most accurate methods, particularly since supernova explosions can be visible at great distances (their luminosities rival that of the galaxy in which they are situated), much farther than Cepheid Variables (500 times farther). Much time has been devoted to the refining of this method. The current uncertainty approaches a mere 5%, corresponding to an uncertainty of just 0.1 magnitudes.
Novae in distance determinations
Novae can be used in much the same way as supernovae to derive extragalactic distances. There is a direct relation between a nova's max magnitude and the time for its visible light to decline by two magnitudes. This relation is shown to be: : Where is the time derivative of the nova's mag, describing the average rate of decline over the first 2 magnitudes. After novae fade, they are about as bright as the most luminous Cepheid variable stars, therefore both these techniques have about the same max distance: ~ 20 Mpc. The error in this method produces an uncertainty in magnitude of about ±0.4Globular cluster luminosity function
Based on the method of comparing the luminosities of globular clusters (located in galactic halos) from distant galaxies to that of theVirgo Cluster
The Virgo Cluster is a large cluster of galaxies whose center is 53.8 ± 0.3 Mly (16.5 ± 0.1 Mpc) away in the constellation Virgo. Comprising approximately 1,300 (and possibly up to 2,000) member galaxies, the cluster forms the heart of the la ...
, the globular cluster luminosity function
A globular cluster is a spheroidal conglomeration of stars. Globular clusters are bound together by gravity, with a higher concentration of stars towards their centers. They can contain anywhere from tens of thousands to many millions of memb ...
carries an uncertainty of distance of about 20% (or 0.4 magnitudes).
US astronomer William Alvin Baum first attempted to use globular clusters to measure distant elliptical galaxies. He compared the brightest globular clusters in Virgo A galaxy with those in Andromeda, assuming the luminosities of the clusters were the same in both. Knowing the distance to Andromeda, Baum has assumed a direct correlation and estimated Virgo A's distance.
Baum used just a single globular cluster, but individual formations are often poor standard candles. Canadian astronomer René Racine assumed the use of the globular cluster luminosity function (GCLF) would lead to a better approximation. The number of globular clusters as a function of magnitude is given by:
:
where ''m''0 is the turnover magnitude, ''M''0 is the magnitude of the Virgo cluster, and sigma is the dispersion ~ 1.4 mag.
It is assumed that globular clusters all have roughly the same luminosities within the universe
The universe is all of space and time and their contents, including planets, stars, galaxies, and all other forms of matter and energy. The Big Bang theory is the prevailing cosmological description of the development of the universe. ...
. There is no universal globular cluster luminosity function that applies to all galaxies.
Planetary nebula luminosity function
Like the GCLF method, a similar numerical analysis can be used forplanetary nebula
A planetary nebula (PN, plural PNe) is a type of emission nebula consisting of an expanding, glowing shell of ionized gas ejected from red giant stars late in their lives.
The term "planetary nebula" is a misnomer because they are unrelate ...
e within far off galaxies. The planetary nebula luminosity function
Planetary nebula luminosity function (PNLF) is a secondary distance indicator used in astronomy. It makes use of the IIIλ5007 forbidden line found in all planetary nebula (PNe) which are members of the old stellar populations ( Populati ...
(PNLF) was first proposed in the late 1970s by Holland Cole and David Jenner. They suggested that all planetary nebulae might all have similar maximum intrinsic brightness, now calculated to be M = −4.53. This would therefore make them potential standard candles for determining extragalactic distances.
Astronomer George Howard Jacoby and his colleagues later proposed that the PNLF function equaled:
:
Where N(M) is number of planetary nebula, having absolute magnitude M. M* is equal to the nebula with the brightest magnitude.
Surface brightness fluctuation method
 The following method deals with the overall inherent properties of galaxies. These methods, though with varying error percentages, have the ability to make distance estimates beyond 100 Mpc, though it is usually applied more locally.
The
The following method deals with the overall inherent properties of galaxies. These methods, though with varying error percentages, have the ability to make distance estimates beyond 100 Mpc, though it is usually applied more locally.
The surface brightness fluctuation
Surface brightness fluctuation (SBF) is a secondary distance indicator used to estimate distances to galaxies. It is useful to 100 Mpc (parsec). The method measures the variance in a galaxy's light distribution arising from fluctuations in the n ...
(SBF) method takes advantage of the use of CCD cameras on telescopes. Because of spatial fluctuations in a galaxy's surface brightness, some pixels on these cameras will pick up more stars than others. However, as distance increases the picture will become increasingly smoother. Analysis of this describes a magnitude of the pixel-to-pixel variation, which is directly related to a galaxy's distance.
Sigma-D relation
TheSigma-D relation The Sigma-D relation, or Σ-D Relation, is the radio surface brightness to diameter relation of a supernova remnant.Urošević, D. et al. (2009Sigma-D relation for supernova remnants and its dependent on the density of the interstellar medium ''Ast ...
(or Σ-D relation), used in elliptical galaxies
An elliptical galaxy is a type of galaxy with an approximately ellipsoidal shape and a smooth, nearly featureless image. They are one of the four main classes of galaxy described by Edwin Hubble in his Hubble sequence and 1936 work ''The Real ...
, relates the angular diameter (D) of the galaxy to its velocity dispersion
In astronomy, the velocity dispersion (''σ'') is the statistical dispersion of velocities about the mean velocity for a group of astronomical objects, such as an open cluster, globular cluster, galaxy, galaxy cluster, or supercluster. By measurin ...
. It is important to describe exactly what D represents, in order to understand this method. It is, more precisely, the galaxy's angular diameter out to the surface brightness
In astronomy, surface brightness (SB) quantifies the apparent brightness or flux density per unit angular area of a spatially extended object such as a galaxy or nebula, or of the night sky background. An object's surface brightness depends on it ...
level of 20.75 B-mag arcsec−2. This surface brightness is independent of the galaxy's actual distance from us. Instead, D is inversely proportional to the galaxy's distance, represented as d. Thus, this relation does not employ standard candles. Rather, D provides a standard ruler. This relation between D and Σ is
:
where C is a constant which depends on the distance to the galaxy clusters.
This method has the potential to become one of the strongest methods of galactic distance calculators, perhaps exceeding the range of even the Tully–Fisher method. As of today, however, elliptical galaxies are not bright enough to provide a calibration for this method through the use of techniques such as Cepheids. Instead, calibration is done using more crude methods.
Overlap and scaling
A succession of distance indicators, which is the distance ladder, is needed for determining distances to other galaxies. The reason is that objects bright enough to be recognized and measured at such distances are so rare that few or none are present nearby, so there are too few examples close enough with reliable trigonometric parallax to calibrate the indicator. For example, Cepheid variables, one of the best indicators for nearbyspiral galaxies
Spiral galaxies form a class of galaxy originally described by Edwin Hubble in his 1936 work ''The Realm of the Nebulae''elliptical galaxies
An elliptical galaxy is a type of galaxy with an approximately ellipsoidal shape and a smooth, nearly featureless image. They are one of the four main classes of galaxy described by Edwin Hubble in his Hubble sequence and 1936 work ''The Real ...
usually have long ceased to have large-scale star formation, they will not have Cepheids. Instead, distance indicators whose origins are in an older stellar population (like novae and RR Lyrae variables) must be used. However, RR Lyrae variables are less luminous than Cepheids, and novae are unpredictable and an intensive monitoring program—and luck during that program—is needed to gather enough novae in the target galaxy for a good distance estimate.
Because the more distant steps of the cosmic distance ladder depend upon the nearer ones, the more distant steps include the effects of error
An error (from the Latin ''error'', meaning "wandering") is an action which is inaccurate or incorrect. In some usages, an error is synonymous with a mistake. The etymology derives from the Latin term 'errare', meaning 'to stray'.
In statistics ...
s in the nearer steps, both systematic and statistical ones. The result of these propagating errors means that distances in astronomy are rarely known to the same level of precision as measurements in the other sciences, and that the precision necessarily is poorer for more distant types of object.
Another concern, especially for the very brightest standard candles, is their "standardness": how homogeneous the objects are in their true absolute magnitude. For some of these different standard candles, the homogeneity is based on theories about the formation
Formation may refer to:
Linguistics
* Back-formation, the process of creating a new lexeme by removing or affixes
* Word formation, the creation of a new word by adding affixes
Mathematics and science
* Cave formation or speleothem, a secondar ...
and evolution
Evolution is change in the heritable characteristics of biological populations over successive generations. These characteristics are the expressions of genes, which are passed on from parent to offspring during reproduction. Variation ...
of stars and galaxies, and is thus also subject to uncertainties in those aspects. For the most luminous of distance indicators, the Type Ia supernovae, this homogeneity is known to be poor; however, no other class of object is bright enough to be detected at such large distances, so the class is useful simply because there is no real alternative.
The observational result of Hubble's Law, the proportional relationship between distance and the speed with which a galaxy is moving away from us (usually referred to as redshift) is a product of the cosmic distance ladder. Edwin Hubble observed that fainter galaxies are more redshifted. Finding the value of the Hubble constant was the result of decades of work by many astronomers, both in amassing the measurements of galaxy redshifts and in calibrating the steps of the distance ladder. Hubble's Law is the primary means we have for estimating the distances of quasars and distant galaxies in which individual distance indicators cannot be seen.
See also
* Araucaria Project *Distance measure
Distance measures are used in physical cosmology to give a natural notion of the distance between two objects or events in the universe. They are often used to tie some ''observable'' quantity (such as the luminosity of a distant quasar, the red ...
* Orders of magnitude (length)#Astronomical
* Standard ruler
Footnotes
References
Bibliography
* *''Measuring the Universe The Cosmological Distance Ladder'', Stephen Webb, copyright 2001. * *''The Astrophysical Journal'', ''The Globular Cluster Luminosity Function as a Distance Indicator: Dynamical Effects'', Ostriker and Gnedin, May 5, 1997. *''An Introduction to Distance Measurement in Astronomy'', Richard de Grijs, Chichester: John Wiley & Sons, 2011, .External links
The ABC's of distances (UCLA)
by Bill Keel
The Hubble Space Telescope Key Project on the Extragalactic Distance Scale
The Hubble Constant
a historical discussion
NASA Cosmic Distance Scale
The Astrophysical Journal
{{Authority control Astrometry Physical cosmology Standard candles Length, distance, or range measuring devices Concepts in astronomy