Dedekind-complete

In mathematics, the least-upper-bound property (sometimes called completeness or supremum property or l.u.b. property) is a fundamental property of the real numbers. More generally, a partially ordered set has the least-upper-bound property if every non-empty subset of with an upper bound has a ''least'' upper bound (supremum) in . Not every (partially) ordered set has the least upper bound property. For example, the set $\mathbb$ of all rational numbers with its natural order does ''not'' have the least upper bound property.

The least-upper-bound property is one form of the completeness axiom for the real numbers, and is sometimes referred to as Dedekind completeness.Willard says that an ordered space "X is Dedekind complete if every subset of X having an upper bound has a least upper bound." (pp. 124-5, Problem 17E.) It can be used to prove many of the fundamental results of real analysis, such as the intermediate value theorem, the Bolzano–Weierstrass theorem, the extreme value theorem, and the Heine–Borel theorem. It is usually taken as an axiom in synthetic constructions of the real numbers, and it is also intimately related to the construction of the real numbers using Dedekind cuts.

In order theory, this property can be generalized to a notion of completeness for any partially ordered set. A linearly ordered set that is dense and has the least upper bound property is called a linear continuum.

Statement of the property

Statement for real numbers

Let be a non-empty set of real numbers.
* A real number is called an upper bound for if for all .
* A real number is the least upper bound (or supremum) for if is an upper bound for and for every upper bound of .
The least-upper-bound property states that any non-empty set of real numbers that has an upper bound must have a least upper bound in ''real numbers''.

Generalization to ordered sets

More generally, one may define upper bound and least upper bound for any subset of a partially ordered set , with “real number” replaced by “element of ”. In this case, we say that has the least-upper-bound property if every non-empty subset of with an upper bound has a least upper bound in .

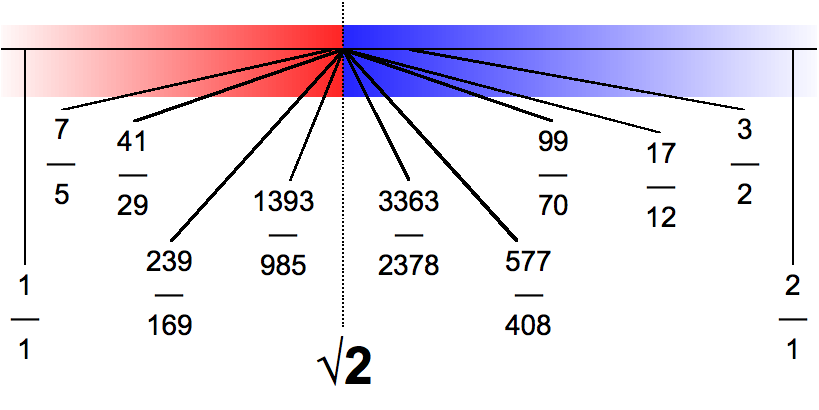
For example, the set of rational numbers does not have the least-upper-bound property under the usual order. For instance, the set

: $\left\ = \mathbf \cap \left(-\sqrt, \sqrt\right)$

has an upper bound in , but does not have a least upper bound in (since the square root of two is irrational). The construction of the real numbers using Dedekind cuts takes advantage of this failure by defining the irrational numbers as the least upper bounds of certain subsets of the rationals.

Proof

Logical status

The least-upper-bound property is equivalent to other forms of the completeness axiom, such as the convergence of Cauchy sequences or the nested intervals theorem. The logical status of the property depends on the construction of the real numbers used: in the synthetic approach, the property is usually taken as an axiom for the real numbers (see least upper bound axiom); in a constructive approach, the property must be proved as a theorem, either directly from the construction or as a consequence of some other form of completeness.

Proof using Cauchy sequences

It is possible to prove the least-upper-bound property using the assumption that every Cauchy sequence of real numbers converges. Let be a nonempty set of real numbers. If has exactly one element, then its only element is a least upper bound. So consider with more than one element, and suppose that has an upper bound . Since is nonempty and has more than one element, there exists a real number that is not an upper bound for . Define sequences and recursively as follows:
# Check whether is an upper bound for .
# If it is, let and let .
# Otherwise there must be an element in so that . Let and let .
Then and as . It follows that both sequences are Cauchy and have the same limit , which must be the least upper bound for .

Applications

The least-upper-bound property of can be used to prove many of the main foundational theorems in real analysis.

Intermediate value theorem

Let be a continuous function, and suppose that and . In this case, the intermediate value theorem states that must have a root in the interval . This theorem can be proved by considering the set
:.
That is, is the initial segment of that takes negative values under . Then is an upper bound for , and the least upper bound must be a root of .

Bolzano–Weierstrass theorem

The Bolzano–Weierstrass theorem for states that every sequence of real numbers in a closed interval must have a convergent subsequence. This theorem can be proved by considering the set
:
Clearly,
$a\in S$, and is not empty.
In addition, is an upper bound for , so has a least upper bound .
Then must be a limit point of the sequence , and it follows that has a subsequence that converges to .

Extreme value theorem

Let be a continuous function and let , where if has no upper bound. The extreme value theorem states that is finite and for some . This can be proved by considering the set
:.
By definition of , , and by its own definition, is bounded by .
If is the least upper bound of , then it follows from continuity that .

Heine–Borel theorem

Let be a closed interval in , and let be a collection of open sets that covers . Then the Heine–Borel theorem states that some finite subcollection of covers as well. This statement can be proved by considering the set
:.
The set obviously contains , and is bounded by by construction.
By the least-upper-bound property, has a least upper bound .
Hence, is itself an element of some open set , and it follows for that can be covered by finitely many for some sufficiently small .
This proves that and is not an upper bound for .
Consequently, .

History

The importance of the least-upper-bound property was first recognized by Bernard Bolzano in his 1817 paper ''Rein analytischer Beweis des Lehrsatzes dass zwischen je zwey Werthen, die ein entgegengesetztes Resultat gewäahren, wenigstens eine reelle Wurzel der Gleichung liege''.

See also

* List of real analysis topics

Notes

References

*
*

*
*
*
*
*
*{{cite book , last=Willard , first=Stephen , title=General Topology , isbn=9780486434797 , orig-year=1970, year=2004 , location=Mineola, N.Y. , publisher=Dover Publications

Real analysis
Order theory
Articles containing proofs