Astroid on:
[Wikipedia]
[Google]
[Amazon]

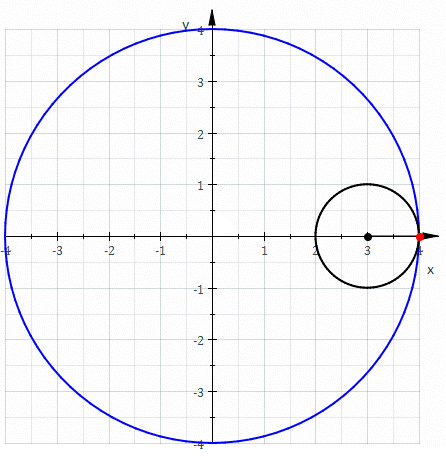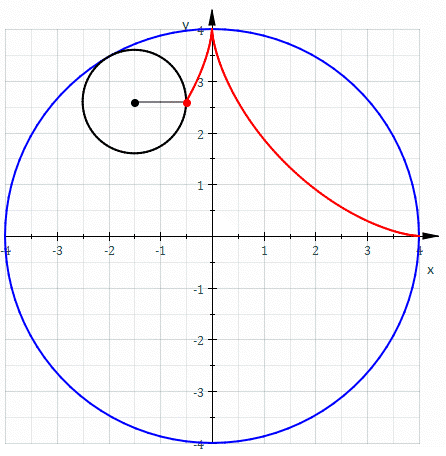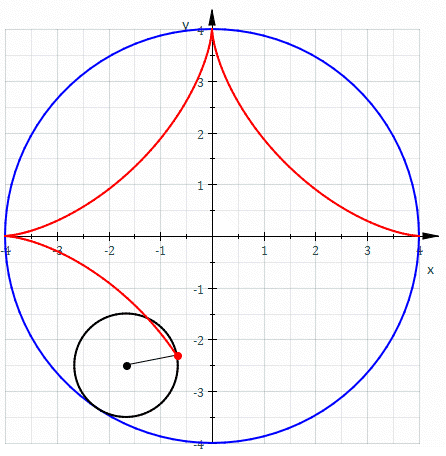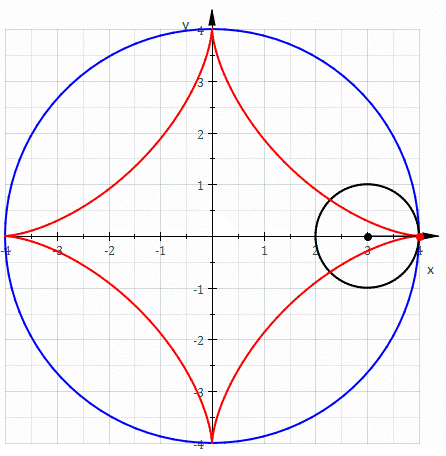

 In mathematics, an astroid is a particular type of roulette curve: a
In mathematics, an astroid is a particular type of roulette curve: a
"Astroid" at The MacTutor History of Mathematics archive
"Astroid" at The Encyclopedia of Remarkable Mathematical Forms
Bars of an Astroid
by Sándor Kabai,

hypocycloid
In geometry, a hypocycloid is a special plane curve generated by the trace of a fixed point on a small circle that rolls within a larger circle. As the radius of the larger circle is increased, the hypocycloid becomes more like the cycloid ...
with four cusps. Specifically, it is the locus
Locus (plural loci) is Latin for "place". It may refer to:
Entertainment
* Locus (comics), a Marvel Comics mutant villainess, a member of the Mutant Liberation Front
* ''Locus'' (magazine), science fiction and fantasy magazine
** ''Locus Award ...
of a point on a circle as it rolls inside a fixed circle with four times the radius
In classical geometry, a radius ( : radii) of a circle or sphere is any of the line segments from its center to its perimeter, and in more modern usage, it is also their length. The name comes from the latin ''radius'', meaning ray but also the ...
. By double generation, it is also the locus of a point on a circle as it rolls inside a fixed circle with 4/3 times the radius. It can also be defined as the envelope
An envelope is a common packaging item, usually made of thin, flat material. It is designed to contain a flat object, such as a letter or card.
Traditional envelopes are made from sheets of paper cut to one of three shapes: a rhombus, a ...
of a line segment of fixed length that moves while keeping an end point on each of the axes. It is therefore the envelope
An envelope is a common packaging item, usually made of thin, flat material. It is designed to contain a flat object, such as a letter or card.
Traditional envelopes are made from sheets of paper cut to one of three shapes: a rhombus, a ...
of the moving bar in the Trammel of Archimedes.
Its modern name comes from the Greek word for "star
A star is an astronomical object comprising a luminous spheroid of plasma held together by its gravity. The nearest star to Earth is the Sun. Many other stars are visible to the naked eye at night, but their immense distances from Earth make ...
". It was proposed, originally in the form of "Astrois", by Joseph Johann von Littrow in 1838. The curve had a variety of names, including tetracuspid (still used), cubocycloid, and paracycle. It is nearly identical in form to the evolute of an ellipse.
Equations
If the radius of the fixed circle is ''a'' then the equation is given by : This implies that an astroid is also asuperellipse
A superellipse, also known as a Lamé curve after Gabriel Lamé, is a closed curve resembling the ellipse, retaining the geometric features of semi-major axis and semi-minor axis, and symmetry about them, but a different overall shape.
In the C ...
.
Parametric equation
In mathematics, a parametric equation defines a group of quantities as functions of one or more independent variables called parameters. Parametric equations are commonly used to express the coordinates of the points that make up a geometric ...
s are
:
The pedal equation with respect to the origin is
:
the Whewell equation is
:
and the Cesàro equation In geometry, the Cesàro equation of a plane curve is an equation relating the curvature () at a point of the curve to the arc length () from the start of the curve to the given point. It may also be given as an equation relating the radius of curv ...
is
:
The polar equation is
:
The astroid is a real locus of a plane algebraic curve
In mathematics, an affine algebraic plane curve is the zero set of a polynomial in two variables. A projective algebraic plane curve is the zero set in a projective plane of a homogeneous polynomial in three variables. An affine algebraic plane ...
of genus
Genus ( plural genera ) is a taxonomic rank used in the biological classification of living and fossil organisms as well as viruses. In the hierarchy of biological classification, genus comes above species and below family. In binomial n ...
zero. It has the equation
:
The astroid is, therefore, a real algebraic curve of degree six.
Derivation of the polynomial equation
The polynomial equation may be derived from Leibniz's equation by elementary algebra: : Cube both sides: : : : Cube both sides again: : But since: : It follows that : Therefore: : or :Metric properties
;Area enclosed : ;Length of curve : ;Volume of the surface of revolution of the enclose area about the ''x''-axis. : ;Area of surface of revolution about the ''x''-axis :Properties
The astroid has four cusp singularities in the real plane, the points on the star. It has two more complex cusp singularities at infinity, and four complex double points, for a total of ten singularities. The dual curve to the astroid is the cruciform curve with equation Theevolute
In the differential geometry of curves, the evolute of a curve is the locus of all its centers of curvature. That is to say that when the center of curvature of each point on a curve is drawn, the resultant shape will be the evolute of that c ...
of an astroid is an astroid twice as large.
The astroid has only one tangent line in each oriented direction, making it an example of a hedgehog
A hedgehog is a spiny mammal of the subfamily Erinaceinae, in the eulipotyphlan family Erinaceidae. There are seventeen species of hedgehog in five genera found throughout parts of Europe, Asia, and Africa, and in New Zealand by introduct ...
.
See also
*Cardioid
In geometry, a cardioid () is a plane curve traced by a point on the perimeter of a circle that is rolling around a fixed circle of the same radius. It can also be defined as an epicycloid having a single cusp. It is also a type of sinusoida ...
(epicycloid with one cusp)
* Nephroid (epicycloid with two cusps)
* Deltoid (hypocycloid with three cusps)
* Stoner–Wohlfarth astroid a use of this curve in magnetics.
* Spirograph
Spirograph is a geometric drawing device that produces mathematical roulette curves of the variety technically known as hypotrochoids and epitrochoids. The well-known toy version was developed by British engineer Denys Fisher and first sold ...
References
* * *External links
* * {{MathWorld , urlname=Astroid , title=Astroid"Astroid" at The MacTutor History of Mathematics archive
"Astroid" at The Encyclopedia of Remarkable Mathematical Forms
Bars of an Astroid
by Sándor Kabai,
The Wolfram Demonstrations Project
The Wolfram Demonstrations Project is an organized, open-source collection of small (or medium-size) interactive programs called Demonstrations, which are meant to visually and interactively represent ideas from a range of fields. It is hos ...
.
Sextic curves
Roulettes (curve)