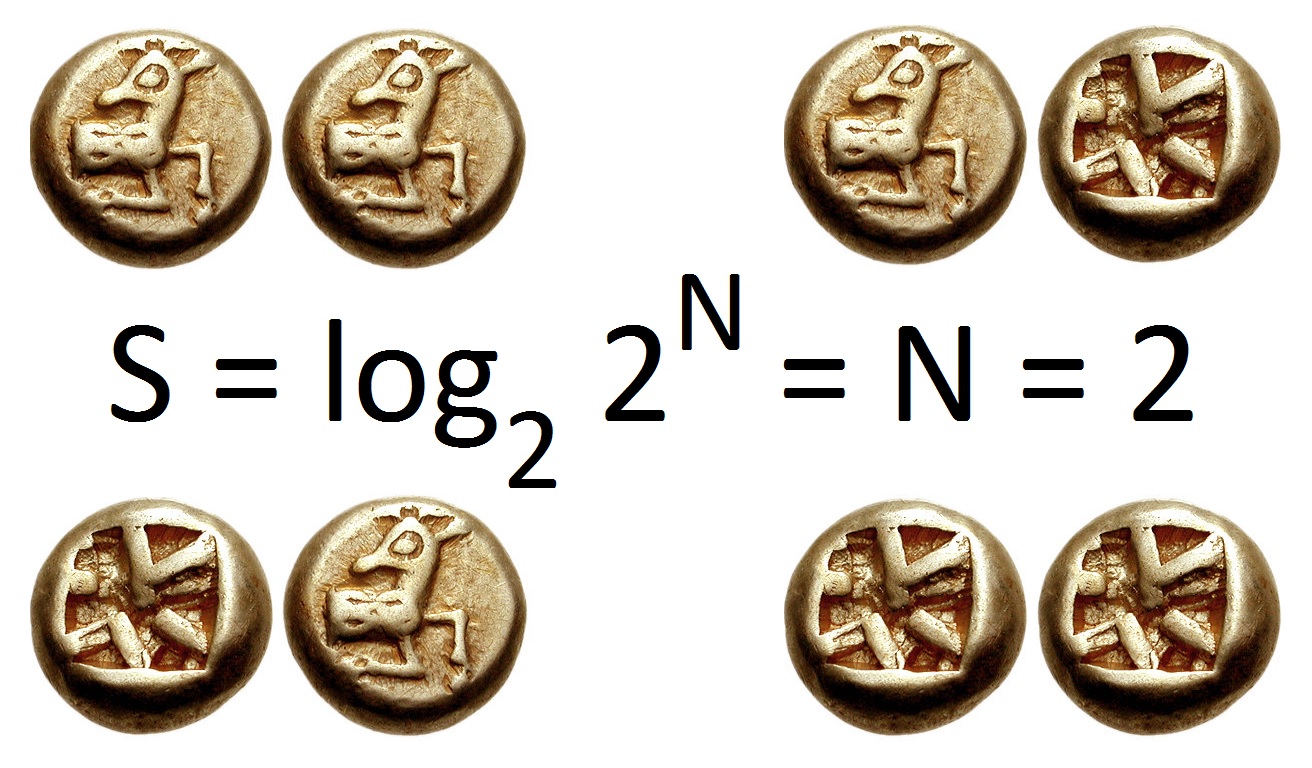|
Von Mises Distribution
In probability theory and directional statistics, the von Mises distribution (also known as the circular normal distribution or Tikhonov distribution) is a continuous probability distribution on the circle. It is a close approximation to the wrapped normal distribution, which is the circular analogue of the normal distribution. A freely diffusing angle \theta on a circle is a wrapped normally distributed random variable with an unwrapped variance that grows linearly in time. On the other hand, the von Mises distribution is the stationary distribution of a drift and diffusion process on the circle in a harmonic potential, i.e. with a preferred orientation. The von Mises distribution is the maximum entropy distribution for circular data when the real and imaginary parts of the first circular moment are specified. The von Mises distribution is a special case of the von Mises–Fisher distribution on the ''N''-dimensional sphere. Definition The von Mises probability density fu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Uniform Convergence
In the mathematical field of analysis, uniform convergence is a mode of convergence of functions stronger than pointwise convergence. A sequence of functions (f_n) converges uniformly to a limiting function f on a set E if, given any arbitrarily small positive number \epsilon, a number N can be found such that each of the functions f_N, f_,f_,\ldots differs from f by no more than \epsilon ''at every point'' x ''in'' E. Described in an informal way, if f_n converges to f uniformly, then the rate at which f_n(x) approaches f(x) is "uniform" throughout its domain in the following sense: in order to guarantee that f_n(x) falls within a certain distance \epsilon of f(x), we do not need to know the value of x\in E in question — there can be found a single value of N=N(\epsilon) ''independent of x'', such that choosing n\geq N will ensure that f_n(x) is within \epsilon of f(x) ''for all x\in E''. In contrast, pointwise convergence of f_n to f merely guarantees that for any x\in E ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Incomplete Bessel Function
In mathematics, the incomplete Bessel functions are types of special functions which act as a type of extension from the complete-type of Bessel functions. Definition The incomplete Bessel functions are defined as the same delay differential equations of the complete-type Bessel functions: :J_(z,w)-J_(z,w)=2\dfracJ_v(z,w) :Y_(z,w)-Y_(z,w)=2\dfracY_v(z,w) :I_(z,w)+I_(z,w)=2\dfracI_v(z,w) :K_(z,w)+K_(z,w)=-2\dfracK_v(z,w) :H_^(z,w)-H_^(z,w)=2\dfracH_v^(z,w) :H_^(z,w)-H_^(z,w)=2\dfracH_v^(z,w) And the following suitable extension forms of delay differential equations from that of the complete-type Bessel functions: :J_(z,w)+J_(z,w)=\dfracJ_v(z,w)-\dfrac\dfracJ_v(z,w) :Y_(z,w)+Y_(z,w)=\dfracY_v(z,w)-\dfrac\dfracY_v(z,w) :I_(z,w)-I_(z,w)=\dfracI_v(z,w)-\dfrac\dfracI_v(z,w) :K_(z,w)-K_(z,w)=-\dfracK_v(z,w)+\dfrac\dfracK_v(z,w) :H_^(z,w)+H_^(z,w)=\dfracH_v^(z,w)-\dfrac\dfracH_v^(z,w) :H_^(z,w)+H_^(z,w)=\dfracH_v^(z,w)-\dfrac\dfracH_v^(z,w) Where the new parameter w defines the integ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dover Publications
Dover Publications, also known as Dover Books, is an American book publisher founded in 1941 by Hayward and Blanche Cirker. It primarily reissues books that are out of print from their original publishers. These are often, but not always, books in the public domain. The original published editions may be scarce or historically significant. Dover republishes these books, making them available at a significantly reduced cost. Classic reprints Dover reprints classic works of literature, classical sheet music, and public-domain images from the 18th and 19th centuries. Dover also publishes an extensive collection of mathematical, scientific, and engineering texts. It often targets its reprints at a niche market, such as woodworking. Starting in 2015, the company branched out into graphic novel reprints, overseen by Dover acquisitions editor and former comics writer and editor Drew Ford. Most Dover reprints are photo facsimiles of the originals, retaining the original pagination an ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Abramowitz And Stegun
''Abramowitz and Stegun'' (''AS'') is the informal name of a 1964 mathematical reference work edited by Milton Abramowitz and Irene Stegun of the United States National Bureau of Standards (NBS), now the ''National Institute of Standards and Technology'' (NIST). Its full title is ''Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables''. A digital successor to the Handbook was released as the " Digital Library of Mathematical Functions" (DLMF) on 11 May 2010, along with a printed version, the '' NIST Handbook of Mathematical Functions'', published by Cambridge University Press. Overview Since it was first published in 1964, the 1046 page ''Handbook'' has been one of the most comprehensive sources of information on special functions, containing definitions, identities, approximations, plots, and tables of values of numerous functions used in virtually all fields of applied mathematics. The notation used in the ''Handbook'' is the ''de facto'' stand ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kent Distribution
In directional statistics, the Kent distribution, also known as the 5-parameter Fisher–Bingham distribution (named after John T. Kent, Ronald Fisher, and Christopher Bingham), is a probability distribution on the unit sphere (2-sphere ''S''2 in 3-space R3). It is the analogue on ''S''2 of the bivariate normal distribution with an unconstrained covariance matrix. The Kent distribution was proposed by John T. Kent in 1982, and is used in geology as well as bioinformatics. Definition The probability density function f(\mathbf)\, of the Kent distribution is given by: : f(\mathbf)=\frac\exp\ where \mathbf\, is a three-dimensional unit vector, (\cdot)^ denotes the transpose of (\cdot), and the normalizing constant \textrm(\kappa,\beta)\, is: : c(\kappa,\beta)=2\pi\sum_^\infty\frac\beta^\left(\frac\kappa\right)^ I_(\kappa) Where I_v(\kappa) is the modified Bessel function and \Gamma(\cdot) is the gamma function. Note that c(0,0) = 4\pi and c(\kappa,0)=4\pi(\kappa^)\sinh(\ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Von Mises–Fisher Distribution
In directional statistics, the von Mises–Fisher distribution (named after Richard von Mises and Ronald Fisher), is a probability distribution on the (p-1)-sphere in \mathbb^. If p=2 the distribution reduces to the von Mises distribution on the circle. Definition The probability density function of the von Mises–Fisher distribution for the random ''p''-dimensional unit vector \mathbf is given by: :f_(\mathbf; \boldsymbol, \kappa) = C_(\kappa) \exp \left( \right), where \kappa \ge 0, \left \Vert \boldsymbol \right \Vert = 1 and the normalization constant C_(\kappa) is equal to : C_(\kappa)=\frac , where I_ denotes the modified Bessel function of the first kind at order v. If p = 3, the normalization constant reduces to : C_(\kappa) = \frac = \frac . The parameters \boldsymbol and \kappa are called the ''mean direction'' and '' concentration parameter'', respectively. The greater the value of \kappa, the higher the concentration of the distribution around the mean ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bivariate Von Mises Distribution
In probability theory and statistics, the bivariate von Mises distribution is a probability distribution describing values on a torus. It may be thought of as an analogue on the torus of the bivariate normal distribution. The distribution belongs to the field of directional statistics. The general bivariate von Mises distribution was first proposed by Kanti Mardia in 1975. One of its variants is today used in the field of bioinformatics to formulate a probabilistic model of protein structure in atomic detail, such as backbone-dependent rotamer libraries. Definition The bivariate von Mises distribution is a probability distribution defined on the torus, S^1 \times S^1 in \mathbb^3. The probability density function of the general bivariate von Mises distribution for the angles \phi, \psi \in , 2\pi/math> is given by : f(\phi, \psi) \propto \exp \kappa_1 \cos(\phi - \mu) + \kappa_2 \cos(\psi - \nu) + (\cos(\phi-\mu), \sin(\phi-\mu)) \mathbf (\cos(\psi - \nu), \sin(\psi - ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Circular Variance
Directional statistics (also circular statistics or spherical statistics) is the subdiscipline of statistics that deals with directions (unit vectors in Euclidean space, R''n''), axes ( lines through the origin in R''n'') or rotations in R''n''. More generally, directional statistics deals with observations on compact Riemannian manifolds including the Stiefel manifold. The fact that 0 degrees and 360 degrees are identical angles, so that for example 180 degrees is not a sensible mean of 2 degrees and 358 degrees, provides one illustration that special statistical methods are required for the analysis of some types of data (in this case, angular data). Other examples of data that may be regarded as directional include statistics involving temporal periods (e.g. time of day, week, month, year, etc.), compass directions, dihedral angles in molecules, orientations, rotations and so on. Circular distributions Any probability density function (pdf) \ p(x) on the line can be "wrap ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Circular Mean
In mathematics and statistics, a circular mean or angular mean is a mean designed for angles and similar cyclic quantities, such as daytimes, and fractional parts of real numbers. This is necessary since most of the usual means may not be appropriate on angle-like quantities. For example, the arithmetic mean of 0° and 360° is 180°, which is misleading because 360° equals 0° modulo a full cycle.Christopher M. Bishop: ''Pattern Recognition and Machine Learning (Information Science and Statistics)'', As another example, the "average time" between 11 PM and 1 AM is either midnight or noon, depending on whether the two times are part of a single night or part of a single calendar day. The circular mean is one of the simplest examples of circular statistics and of statistics of non-Euclidean spaces. This computation produces a different result than the arithmetic mean, with the difference being greater when the angles are widely distributed. For example, the arithmetic mean of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Entropy (information Theory)
In information theory, the entropy of a random variable is the average level of "information", "surprise", or "uncertainty" inherent to the variable's possible outcomes. Given a discrete random variable X, which takes values in the alphabet \mathcal and is distributed according to p: \mathcal\to , 1/math>: \Eta(X) := -\sum_ p(x) \log p(x) = \mathbb \log p(X), where \Sigma denotes the sum over the variable's possible values. The choice of base for \log, the logarithm, varies for different applications. Base 2 gives the unit of bits (or " shannons"), while base ''e'' gives "natural units" nat, and base 10 gives units of "dits", "bans", or " hartleys". An equivalent definition of entropy is the expected value of the self-information of a variable. The concept of information entropy was introduced by Claude Shannon in his 1948 paper "A Mathematical Theory of Communication",PDF archived froherePDF archived frohere and is also referred to as Shannon entropy. Shannon's theory def ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Circular Uniform Distribution
In probability theory and directional statistics, a circular uniform distribution is a probability distribution on the unit circle whose density is uniform for all angles. Description Definition The probability density function (pdf) of the circular uniform distribution, e.g. with \theta\in[0,2\pi), is: : f_(\theta)=\frac. Moments with respect to a parametrization We consider the circular variable z=e^ with z=1 at base angle \theta=0. In these terms, the circular moments of the circular uniform distribution are all zero, except for m_0: :\langle z^n\rangle=\delta_n where \delta_n is the Kronecker delta symbol. Descriptive statistics Here the mean angle is undefined, and the length of the mean resultant is zero. : R=, \langle z^n\rangle, =0\, Distribution of the mean The sample mean of a set of ''N'' measurements z_n=e^ drawn from a circular uniform distribution is defined as: : \overline = \frac\sum_^N z_n = \overline+i\overline = \overlinee^ where the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |