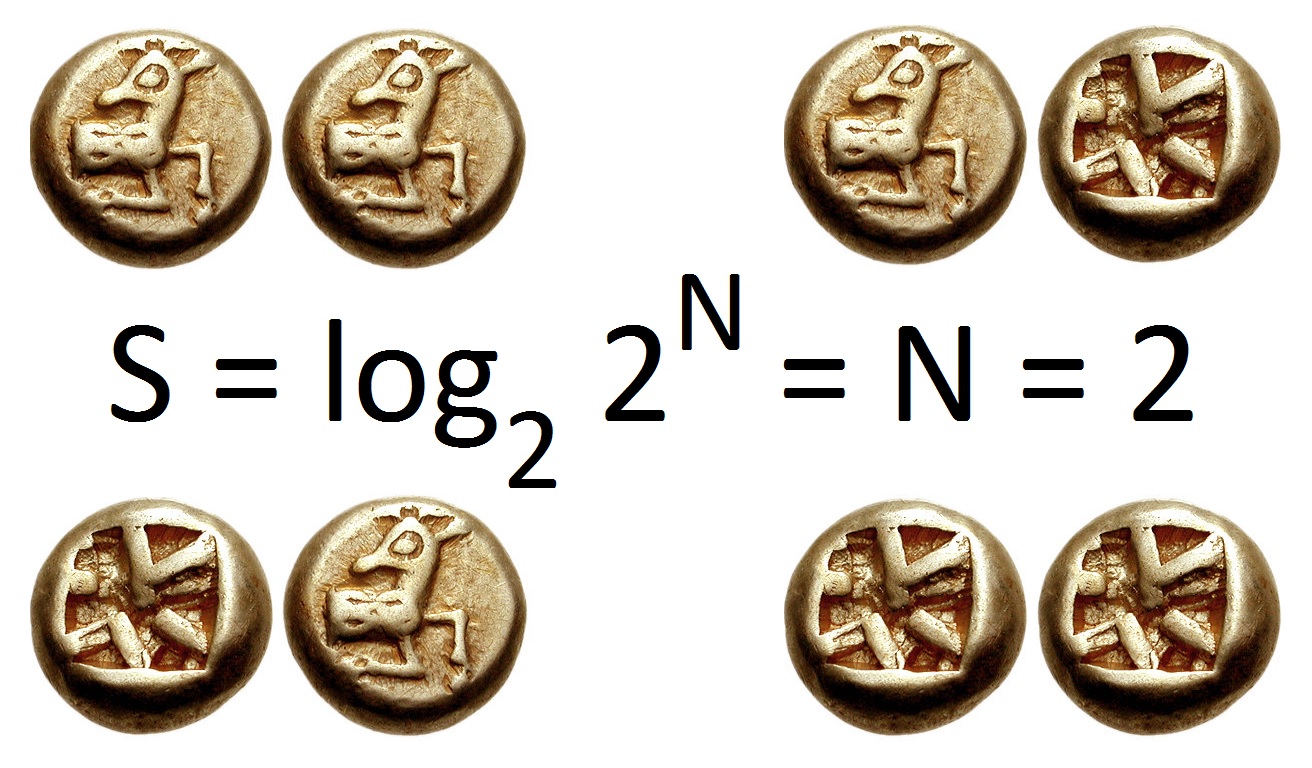|
True Diversity
A diversity index is a quantitative measure that reflects how many different types (such as species) there are in a dataset (a community), and that can simultaneously take into account the phylogenetic relations among the individuals distributed among those types, such as ''richness'', ''divergence'' or ''evenness''. These indices are statistical representations of biodiversity in different aspects ( richness, evenness, and dominance). Effective number of species or Hill numbers When diversity indices are used in ecology, the types of interest are usually species, but they can also be other categories, such as genera, families, functional types, or haplotypes. The entities of interest are usually individual plants or animals, and the measure of abundance can be, for example, number of individuals, biomass or coverage. In demography, the entities of interest can be people, and the types of interest various demographic groups. In information science, the entities can be charact ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Species
In biology, a species is the basic unit of classification and a taxonomic rank of an organism, as well as a unit of biodiversity. A species is often defined as the largest group of organisms in which any two individuals of the appropriate sexes or mating types can produce fertile offspring, typically by sexual reproduction. Other ways of defining species include their karyotype, DNA sequence, morphology, behaviour or ecological niche. In addition, paleontologists use the concept of the chronospecies since fossil reproduction cannot be examined. The most recent rigorous estimate for the total number of species of eukaryotes is between 8 and 8.7 million. However, only about 14% of these had been described by 2011. All species (except viruses) are given a two-part name, a "binomial". The first part of a binomial is the genus to which the species belongs. The second part is called the specific name or the specific epithet (in botanical nomenclature, also sometimes i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fraction (mathematics)
A fraction (from la, fractus, "broken") represents a part of a whole or, more generally, any number of equal parts. When spoken in everyday English, a fraction describes how many parts of a certain size there are, for example, one-half, eight-fifths, three-quarters. A ''common'', ''vulgar'', or ''simple'' fraction (examples: \tfrac and \tfrac) consists of a numerator, displayed above a line (or before a slash like ), and a non-zero denominator, displayed below (or after) that line. Numerators and denominators are also used in fractions that are not ''common'', including compound fractions, complex fractions, and mixed numerals. In positive common fractions, the numerator and denominator are natural numbers. The numerator represents a number of equal parts, and the denominator indicates how many of those parts make up a unit or a whole. The denominator cannot be zero, because zero parts can never make up a whole. For example, in the fraction , the numerator 3 indicates that the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Shannon Information Content
In information theory, the information content, self-information, surprisal, or Shannon information is a basic quantity derived from the probability of a particular event occurring from a random variable. It can be thought of as an alternative way of expressing probability, much like odds or log-odds, but which has particular mathematical advantages in the setting of information theory. The Shannon information can be interpreted as quantifying the level of "surprise" of a particular outcome. As it is such a basic quantity, it also appears in several other settings, such as the length of a message needed to transmit the event given an optimal source coding of the random variable. The Shannon information is closely related to ''entropy'', which is the expected value of the self-information of a random variable, quantifying how surprising the random variable is "on average". This is the average amount of self-information an observer would expect to gain about a random variable when ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Entropy (information Theory)
In information theory, the entropy of a random variable is the average level of "information", "surprise", or "uncertainty" inherent to the variable's possible outcomes. Given a discrete random variable X, which takes values in the alphabet \mathcal and is distributed according to p: \mathcal\to , 1/math>: \Eta(X) := -\sum_ p(x) \log p(x) = \mathbb \log p(X), where \Sigma denotes the sum over the variable's possible values. The choice of base for \log, the logarithm, varies for different applications. Base 2 gives the unit of bits (or " shannons"), while base ''e'' gives "natural units" nat, and base 10 gives units of "dits", "bans", or " hartleys". An equivalent definition of entropy is the expected value of the self-information of a variable. The concept of information entropy was introduced by Claude Shannon in his 1948 paper "A Mathematical Theory of Communication",PDF archived froherePDF archived frohere and is also referred to as Shannon entropy. Shannon's theory defi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Claude Shannon
Claude Elwood Shannon (April 30, 1916 – February 24, 2001) was an American people, American mathematician, electrical engineering, electrical engineer, and cryptography, cryptographer known as a "father of information theory". As a 21-year-old master's degree student at the Massachusetts Institute of Technology (MIT), he wrote A Symbolic Analysis of Relay and Switching Circuits, his thesis demonstrating that electrical applications of Boolean algebra could construct any logical numerical relationship. Shannon contributed to the field of cryptanalysis for national defense of the United States during World War II, including his fundamental work on codebreaking and secure telecommunications. Biography Childhood The Shannon family lived in Gaylord, Michigan, and Claude was born in a hospital in nearby Petoskey, Michigan, Petoskey. His father, Claude Sr. (1862–1934), was a businessman and for a while, a judge of probate in Gaylord. His mother, Mabel Wolf Shannon (1890–1945), ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Global Ecology And Biogeography
''Global Ecology and Biogeography'' is a bimonthly peer-reviewed scientific journal that was established in 1991. It covers research in the field of macroecology. The current editor-in-chief is Brian McGill. According to its publisher, Wiley, the journal has an impact factor The impact factor (IF) or journal impact factor (JIF) of an academic journal is a scientometric index calculated by Clarivate that reflects the yearly mean number of citations of articles published in the last two years in a given journal, as i ... of 5.667, and in 2018 it was ranked 17/165 journals in "Ecology," and 2/50 in "Geography, Physical" by the ISI Journal Citation Reports Ranking: https://onlinelibrary.wiley.com/journal/14668238 External links * {{Official, 1=http://onlinelibrary.wiley.com/journal/10.1111/(ISSN)1466-8238 Bimonthly journals Publications established in 1993 English-language journals ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Warren Weaver
Warren Weaver (July 17, 1894 – November 24, 1978) was an American scientist, mathematician, and science administrator. He is widely recognized as one of the pioneers of machine translation and as an important figure in creating support for science in the United States. Career Weaver received three degrees from the University of Wisconsin–Madison: a Bachelor of Science in 1916, a civil engineering degree in 1917, and a Ph.D. in 1921. He became an assistant professor of mathematics at Throop College (now California Institute of Technology). He served as a second lieutenant in the Air Service during World War I. After the war, he returned to teach mathematics at Wisconsin (1920–32). Weaver was director of the Division of Natural Sciences at the Rockefeller Foundation (1932–55), and was science consultant (1947–51), trustee (1954), and vice president (from 1958) at the Sloan-Kettering Institute for Cancer Research. His chief researches were in the problems of communicat ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Norbert Wiener
Norbert Wiener (November 26, 1894 – March 18, 1964) was an American mathematician and philosopher. He was a professor of mathematics at the Massachusetts Institute of Technology (MIT). A child prodigy, Wiener later became an early researcher in stochastic and mathematical noise processes, contributing work relevant to electronic engineering, electronic communication, and control systems. Wiener is considered the originator of cybernetics, the science of communication as it relates to living things and machines, with implications for engineering, systems control, computer science, biology, neuroscience, philosophy, and the organization of society. Norbert Wiener is credited as being one of the first to theorize that all intelligent behavior was the result of feedback mechanisms, that could possibly be simulated by machines and was an important early step towards the development of modern artificial intelligence. Biography Youth Wiener was born in Columbia, Missouri, the first ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Infinity
Infinity is that which is boundless, endless, or larger than any natural number. It is often denoted by the infinity symbol . Since the time of the ancient Greeks, the philosophical nature of infinity was the subject of many discussions among philosophers. In the 17th century, with the introduction of the infinity symbol and the infinitesimal calculus, mathematicians began to work with infinite series and what some mathematicians (including l'Hôpital and Bernoulli) regarded as infinitely small quantities, but infinity continued to be associated with endless processes. As mathematicians struggled with the foundation of calculus, it remained unclear whether infinity could be considered as a number or magnitude and, if so, how this could be done. At the end of the 19th century, Georg Cantor enlarged the mathematical study of infinity by studying infinite sets and infinite numbers, showing that they can be of various sizes. For example, if a line is viewed as the set of all o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Arithmetic Mean
In mathematics and statistics, the arithmetic mean ( ) or arithmetic average, or just the ''mean'' or the ''average'' (when the context is clear), is the sum of a collection of numbers divided by the count of numbers in the collection. The collection is often a set of results of an experiment or an observational study, or frequently a set of results from a survey. The term "arithmetic mean" is preferred in some contexts in mathematics and statistics, because it helps distinguish it from other means, such as the geometric mean and the harmonic mean. In addition to mathematics and statistics, the arithmetic mean is used frequently in many diverse fields such as economics, anthropology and history, and it is used in almost every academic field to some extent. For example, per capita income is the arithmetic average income of a nation's population. While the arithmetic mean is often used to report central tendencies, it is not a robust statistic, meaning that it is greatly influe ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geometric Mean
In mathematics, the geometric mean is a mean or average which indicates a central tendency of a set of numbers by using the product of their values (as opposed to the arithmetic mean which uses their sum). The geometric mean is defined as the th root of the product of numbers, i.e., for a set of numbers , the geometric mean is defined as :\left(\prod_^n a_i\right)^\frac = \sqrt /math> or, equivalently, as the arithmetic mean in logscale: :\exp For instance, the geometric mean of two numbers, say 2 and 8, is just the square root of their product, that is, \sqrt = 4. As another example, the geometric mean of the three numbers 4, 1, and 1/32 is the cube root of their product (1/8), which is 1/2, that is, \sqrt = 1/2. The geometric mean applies only to positive numbers. The geometric mean is often used for a set of numbers whose values are meant to be multiplied together or are exponential in nature, such as a set of growth figures: values of the human population or inter ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Harmonic Mean
In mathematics, the harmonic mean is one of several kinds of average, and in particular, one of the Pythagorean means. It is sometimes appropriate for situations when the average rate is desired. The harmonic mean can be expressed as the reciprocal of the arithmetic mean of the reciprocals of the given set of observations. As a simple example, the harmonic mean of 1, 4, and 4 is : \left(\frac\right)^ = \frac = \frac = 2\,. Definition The harmonic mean ''H'' of the positive real numbers x_1, x_2, \ldots, x_n is defined to be :H = \frac = \frac = \left(\frac\right)^. The third formula in the above equation expresses the harmonic mean as the reciprocal of the arithmetic mean of the reciprocals. From the following formula: :H = \frac. it is more apparent that the harmonic mean is related to the arithmetic and geometric means. It is the reciprocal dual of the arithmetic mean for positive inputs: :1/H(1/x_1 \ldots 1/x_n) = A(x_1 \ldots x_n) The harmonic mean is a Schur-con ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |