|
Tetrahedron Packing
In geometry, tetrahedron packing is the problem of arranging identical regular tetrahedra throughout three-dimensional space so as to fill the maximum possible fraction of space. Currently, the best lower bound achieved on the optimal packing fraction of regular tetrahedra is 85.63%. Tetrahedra do not tile space, and an upper bound below 100% (namely, 1 − (2.6...)·10−25) has been reported. Historical results Aristotle claimed that tetrahedra could fill space completely. In 2006, Conway and Torquato showed that a packing fraction about 72% can be obtained by constructing a non-Bravais lattice packing of tetrahedra (with multiple particles with generally different orientations per repeating unit), and thus they showed that the best tetrahedron packing cannot be a lattice packing (with one particle per repeating unit such that each particle has a common orientation). These packing constructions almost doubled the optimal Bravais-lattice-packing fraction 36.73% obtained ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is called a '' geometer''. Until the 19th century, geometry was almost exclusively devoted to Euclidean geometry, which includes the notions of point, line, plane, distance, angle, surface, and curve, as fundamental concepts. During the 19th century several discoveries enlarged dramatically the scope of geometry. One of the oldest such discoveries is Carl Friedrich Gauss' ("remarkable theorem") that asserts roughly that the Gaussian curvature of a surface is independent from any specific embedding in a Euclidean space. This implies that surfaces can be studied ''intrinsically'', that is, as stand-alone spaces, and has been expanded into the theory of manifolds and Riemannian geometry. Later in the 19th century, it appeared that geom ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Monte Carlo Method
Monte Carlo methods, or Monte Carlo experiments, are a broad class of computational algorithms that rely on repeated random sampling to obtain numerical results. The underlying concept is to use randomness to solve problems that might be deterministic in principle. They are often used in physical and mathematical problems and are most useful when it is difficult or impossible to use other approaches. Monte Carlo methods are mainly used in three problem classes: optimization, numerical integration, and generating draws from a probability distribution. In physics-related problems, Monte Carlo methods are useful for simulating systems with many coupled degrees of freedom, such as fluids, disordered materials, strongly coupled solids, and cellular structures (see cellular Potts model, interacting particle systems, McKean–Vlasov processes, kinetic models of gases). Other examples include modeling phenomena with significant uncertainty in inputs such as the calculation of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cell-transitive
In geometry, a tessellation of dimension (a plane tiling) or higher, or a polytope of dimension (a polyhedron) or higher, is isohedral or face-transitive if all its faces are the same. More specifically, all faces must be not merely congruent but must be ''transitive'', i.e. must lie within the same ''symmetry orbit''. In other words, for any two faces and , there must be a symmetry of the ''entire'' figure by translations, rotations, and/or reflections that maps onto . For this reason, convex isohedral polyhedra are the shapes that will make fair dice. Isohedral polyhedra are called isohedra. They can be described by their face configuration. An isohedron has an even number of faces. The dual of an isohedral polyhedron is vertex-transitive, i.e. isogonal. The Catalan solids, the bipyramids, and the trapezohedra are all isohedral. They are the duals of the (isogonal) Archimedean solids, prisms, and antiprisms, respectively. The Platonic solids, which are either self-dual ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
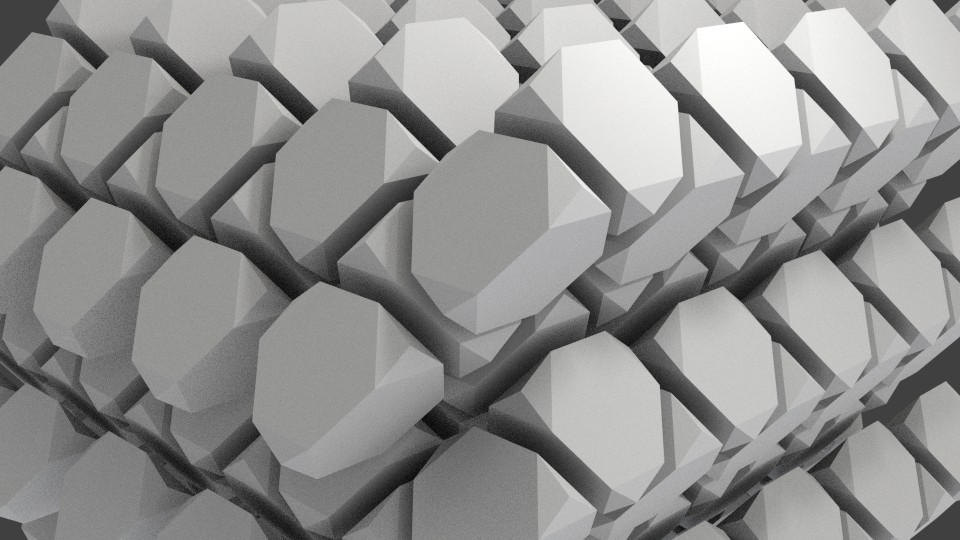
Triakis Truncated Tetrahedral Honeycomb
The triakis truncated tetrahedral honeycomb is a space-filling tessellation (or honeycomb) in Euclidean 3-space made up of triakis truncated tetrahedra. It was discovered in 1914. Voronoi tessellation It is the Voronoi tessellation of the carbon atoms in diamond, which lie in the diamond cubic crystal structure. Being composed entirely of triakis truncated tetrahedra, it is cell-transitive. Relation to quarter cubic honeycomb It can be seen as the uniform quarter cubic honeycomb where its tetrahedral cells are subdivided by the center point into 4 shorter tetrahedra, and each adjoined to the adjacent truncated tetrahedral cells. : See also *Disphenoid tetrahedral honeycomb The tetragonal disphenoid tetrahedral honeycomb is a space-filling tessellation (or honeycomb) in Euclidean 3-space made up of identical tetragonal disphenoidal cells. Cells are face-transitive with 4 identical isosceles triangle faces. John Horton ... References Honeycombs (geometry) Truncated tili ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Isohedral
In geometry, a tessellation of dimension (a plane tiling) or higher, or a polytope of dimension (a polyhedron) or higher, is isohedral or face-transitive if all its faces are the same. More specifically, all faces must be not merely congruent but must be ''transitive'', i.e. must lie within the same '' symmetry orbit''. In other words, for any two faces and , there must be a symmetry of the ''entire'' figure by translations, rotations, and/or reflections that maps onto . For this reason, convex isohedral polyhedra are the shapes that will make fair dice. Isohedral polyhedra are called isohedra. They can be described by their face configuration. An isohedron has an even number of faces. The dual of an isohedral polyhedron is vertex-transitive, i.e. isogonal. The Catalan solids, the bipyramids, and the trapezohedra are all isohedral. They are the duals of the (isogonal) Archimedean solids, prisms, and antiprisms, respectively. The Platonic solids, which are either sel ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Disphenoid Tetrahedral Honeycomb
The tetragonal disphenoid tetrahedral honeycomb is a space-filling tessellation (or honeycomb) in Euclidean 3-space made up of identical tetragonal disphenoidal cells. Cells are face-transitive with 4 identical isosceles triangle faces. John Horton Conway calls it an ''oblate tetrahedrille'' or shortened to ''obtetrahedrille''. Symmetry of Things, Table 21.1. Prime Architectonic and Catopric tilings of space, p. 293, 295. A cell can be seen as 1/12 of a translational cube, with its vertices centered on two faces and two edges. Four of its edges belong to 6 cells, and two edges belong to 4 cells. : The tetrahedral disphenoid honeycomb is the dual of the uniform bitruncated cubic honeycomb. Its vertices form the A / D lattice, which is also known as the body-centered cubic lattice. Geometry This honeycomb's vertex figure is a tetrakis cube: 24 disphenoids meet at each vertex. The union of these 24 disphenoids forms a rhombic dodecahedron. Each edge of the tessellation is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Packing Problem
Packing problems are a class of optimization problems in mathematics that involve attempting to pack objects together into containers. The goal is to either pack a single container as densely as possible or pack all objects using as few containers as possible. Many of these problems can be related to real-life packaging, storage and transportation issues. Each packing problem has a dual covering problem, which asks how many of the same objects are required to completely cover every region of the container, where objects are allowed to overlap. In a bin packing problem, you are given: * A ''container'', usually a two- or three-dimensional convex region, possibly of infinite size. Multiple containers may be given depending on the problem. * A set of ''objects'', some or all of which must be packed into one or more containers. The set may contain different objects with their sizes specified, or a single object of a fixed dimension that can be used repeatedly. Usually the pa ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ulam's Packing Conjecture
Ulam's packing conjecture, named for Stanislaw Ulam, is a conjecture about the highest possible packing density of identical convex solids in three-dimensional Euclidean space. The conjecture says that the optimal density for packing congruent spheres is smaller than that for any other convex body. That is, according to the conjecture, the ball is the convex solid which forces the largest fraction of space to remain empty in its optimal packing structure. This conjecture is therefore related to the Kepler conjecture about sphere packing. Since the solution to the Kepler conjecture establishes that identical balls must leave ≈25.95% of the space empty, Ulam's conjecture is equivalent to the statement that no other convex solid forces that much space to be left empty. Origin This conjecture was attributed posthumously to Ulam by Martin Gardner, who remarks in a postscript added to one of his ''Mathematical Games'' columns that Ulam communicated this conjecture to him in 1972. T ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sphere Packing
In geometry, a sphere packing is an arrangement of non-overlapping spheres within a containing space. The spheres considered are usually all of identical size, and the space is usually three- dimensional Euclidean space. However, sphere packing problems can be generalised to consider unequal spheres, spaces of other dimensions (where the problem becomes circle packing in two dimensions, or hypersphere packing in higher dimensions) or to non-Euclidean spaces such as hyperbolic space. A typical sphere packing problem is to find an arrangement in which the spheres fill as much of the space as possible. The proportion of space filled by the spheres is called the '' packing density'' of the arrangement. As the local density of a packing in an infinite space can vary depending on the volume over which it is measured, the problem is usually to maximise the average or asymptotic density, measured over a large enough volume. For equal spheres in three dimensions, the densest pac ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Minkowski Sum
In geometry, the Minkowski sum (also known as dilation) of two sets of position vectors ''A'' and ''B'' in Euclidean space is formed by adding each vector in ''A'' to each vector in ''B'', i.e., the set : A + B = \. Analogously, the Minkowski difference (or geometric difference) is defined using the complement operation as : A - B = \left(A^c + (-B)\right)^c In general A - B \ne A + (-B). For instance, in a one-dimensional case A = 2, 2/math> and B = 1, 1/math> the Minkowski difference A - B = 1, 1/math>, whereas A + (-B) = A + B = 3, 3 In a two-dimensional case, Minkowski difference is closely related to erosion (morphology) in image processing. The concept is named for Hermann Minkowski. Example For example, if we have two sets ''A'' and ''B'', each consisting of three position vectors (informally, three points), representing the vertices of two triangles in \mathbb^2, with coordinates :A = \ and :B = \ then their Minkowski sum is :A + B = \ which com ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Triangular Bipyramid
In geometry, the triangular bipyramid (or dipyramid) is a type of hexahedron, being the first in the infinite set of face-transitive bipyramids. It is the dual of the triangular prism with 6 isosceles triangle faces. As the name suggests, it can be constructed by joining two tetrahedra along one face. Although all its faces are congruent and the solid is face-transitive, it is not a Platonic solid because some vertices adjoin three faces and others adjoin four. The bipyramid whose six faces are all equilateral triangles is one of the Johnson solids, (). As a Johnson solid with all faces equilateral triangles, it is also a deltahedron. Formulae The following formulae for the height (H), surface area (A) and volume (V) can be used if all faces are regular, with edge length L: :H = L\cdot \frac \approx L\cdot 1.632993162 :A = L^2 \cdot \frac \approx L^2\cdot 2.598076211 :V = L^3 \cdot \frac \approx L^3\cdot 0.235702260 Dual polyhedron The dual polyhedron of th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Double Lattice
In mathematics, especially in geometry, a double lattice in is a discrete subgroup of the group of Euclidean motions that consists only of translations and point reflections and such that the subgroup of translations is a lattice. The orbit of any point under the action of a double lattice is a union of two Bravais lattices, related to each other by a point reflection. A double lattice in two dimensions is a p2 wallpaper group. In three dimensions, a double lattice is a space group of the type , as denoted by international notation. Double lattice packing A packing that can be described as the orbit of a body under the action of a double lattice is called a double lattice packing. In many cases the highest known packing density for a body is achieved by a double lattice. Examples include the regular pentagon, heptagon, and nonagon and the equilateral triangular bipyramid. Włodzimierz Kuperberg and Greg Kuperberg showed that all convex planar bodies can pack at a density o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |







_(cropped).jpg)