|
Sum Of Complementation
In music theory, ''complement'' refers to either traditional interval complementation, or the aggregate complementation of twelve-tone and serialism. In interval complementation a complement is the interval which, when added to the original interval, spans an octave in total. For example, a major 3rd is the complement of a minor 6th. The complement of any interval is also known as its ''inverse'' or ''inversion''. Note that the octave and the unison are each other's complements and that the tritone is its own complement (though the latter is "re-spelt" as either an augmented fourth or a diminished fifth, depending on the context). In the aggregate complementation of twelve-tone music and serialism the complement of one set of notes from the chromatic scale contains all the ''other'' notes of the scale. For example, A-B-C-D-E-F-G is ''complemented'' by B-C-E-F-A. Note that ''musical set theory'' broadens the definition of both senses somewhat. Interval complementation Rule o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Complement Trad
A complement is something that completes something else. Complement may refer specifically to: The arts * Complement (music), an interval that, when added to another, spans an octave ** Aggregate complementation, the separation of pitch-class collections into complementary sets * Complementary color, in the visual arts Biology and medicine *Complement system (immunology), a cascade of proteins in the blood that form part of innate immunity *Complementary DNA, DNA reverse transcribed from a mature mRNA template *Complementarity (molecular biology), a property whereby double stranded nucleic acids pair with each other *Complementation (genetics), a test to determine if independent recessive mutant phenotypes are caused by mutations in the same gene or in different genes Grammar and linguistics * Complement (linguistics), a word or phrase having a particular syntactic role ** Subject complement, a word or phrase adding to a clause's subject after a linking verb * Phonetic comp ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Perfect Fourth
A fourth is a musical interval encompassing four staff positions in the music notation of Western culture, and a perfect fourth () is the fourth spanning five semitones (half steps, or half tones). For example, the ascending interval from C to the next F is a perfect fourth, because the note F is the fifth semitone above C, and there are four staff positions between C and F. Diminished and augmented fourths span the same number of staff positions, but consist of a different number of semitones (four and six, respectively). The perfect fourth may be derived from the harmonic series as the interval between the third and fourth harmonics. The term ''perfect'' identifies this interval as belonging to the group of perfect intervals, so called because they are neither major nor minor. A perfect fourth in just intonation corresponds to a pitch ratio of 4:3, or about 498 cents (), while in equal temperament a perfect fourth is equal to five semitones, or 500 cents (see additive synth ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Complement (set Theory)
In set theory, the complement of a set , often denoted by (or ), is the set of elements not in . When all sets in the universe, i.e. all sets under consideration, are considered to be members of a given set , the absolute complement of is the set of elements in that are not in . The relative complement of with respect to a set , also termed the set difference of and , written B \setminus A, is the set of elements in that are not in . Absolute complement Definition If is a set, then the absolute complement of (or simply the complement of ) is the set of elements not in (within a larger set that is implicitly defined). In other words, let be a set that contains all the elements under study; if there is no need to mention , either because it has been previously specified, or it is obvious and unique, then the absolute complement of is the relative complement of in : A^\complement = U \setminus A. Or formally: A^\complement = \. The absolute complement of is u ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
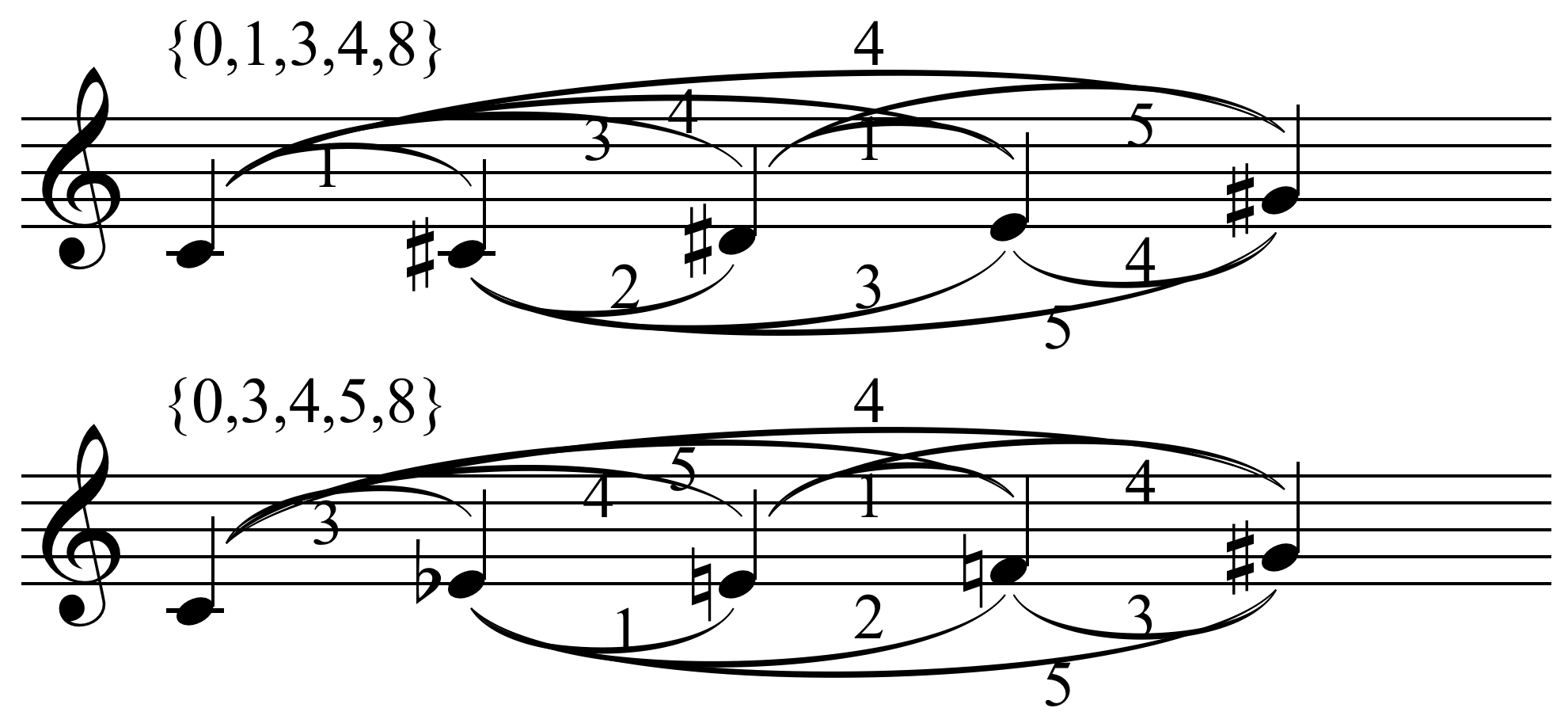
Set Theory (music)
Musical set theory provides concepts for categorizing musical objects and describing their relationships. Howard Hanson first elaborated many of the concepts for analyzing tonal music. Other theorists, such as Allen Forte, further developed the theory for analyzing atonal music, drawing on the twelve-tone theory of Milton Babbitt. The concepts of musical set theory are very general and can be applied to tonal and atonal styles in any equal temperament tuning system, and to some extent more generally than that. One branch of musical set theory deals with collections ( sets and permutations) of pitches and pitch classes (pitch-class set theory), which may be ordered or unordered, and can be related by musical operations such as transposition, melodic inversion, and complementation. Some theorists apply the methods of musical set theory to the analysis of rhythm as well. Mathematical set theory versus musical set theory Although musical set theory is often thought to inv ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Transposition (music)
In music, transposition refers to the process or operation of moving a collection of notes ( pitches or pitch classes) up or down in pitch by a constant interval. For example, one might transpose an entire piece of music into another key. Similarly, one might transpose a tone row or an unordered collection of pitches such as a chord so that it begins on another pitch. The transposition of a set ''A'' by ''n'' semitones is designated by ''T''''n''(''A''), representing the addition ( mod 12) of an integer ''n'' to each of the pitch class integers of the set ''A''. Thus the set (''A'') consisting of 0–1–2 transposed by 5 semitones is 5–6–7 (''T''5(''A'')) since , , and . Scalar transpositions In scalar transposition, every pitch in a collection is shifted up or down a fixed number of scale steps within some scale. The pitches remain in the same scale before and after the shift. This term covers both chromatic and diatonic transpositions as follows. Chromatic tr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Schoenberg - Moses Und Aron Combinatorial Tone Rows
Arnold Schoenberg or Schönberg (, ; ; 13 September 187413 July 1951) was an Austrian-American composer, music theorist, teacher, writer, and painter. He is widely considered one of the most influential composers of the 20th century. He was associated with the expressionist movement in German poetry and art, and leader of the Second Viennese School. As a Jewish composer, Schoenberg was targeted by the Nazi Party, which labeled his works as degenerate music and forbade them from being published. He immigrated to the United States in 1933, becoming an American citizen in 1941. Schoenberg's approach, bοth in terms of harmony and development, has shaped much of 20th-century musical thought. Many composers from at least three generations have consciously extended his thinking, whereas others have passionately reacted against it. Schoenberg was known early in his career for simultaneously extending the traditionally opposed German Romantic styles of Brahms and Wagner. Later, his n ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Permutation (music)
In music, a permutation (order) of a set is any ordering of the elements of that set. A specific arrangement of a set of discrete entities, or parameters, such as pitch, dynamics, or timbre. Different permutations may be related by transformation, through the application of zero or more ''operations'', such as transposition, inversion, retrogradation, circular permutation (also called ''rotation''), or multiplicative operations (such as the cycle of fourths and cycle of fifths transforms). These may produce reorderings of the members of the set, or may simply map the set onto itself. Order is particularly important in the theories of composition techniques originating in the 20th century such as the twelve-tone technique and serialism. Analytical techniques such as set theory take care to distinguish between ordered and unordered collections. In traditional theory concepts like voicing and form include ordering; for example, many musical forms, such as rondo, are defined by ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
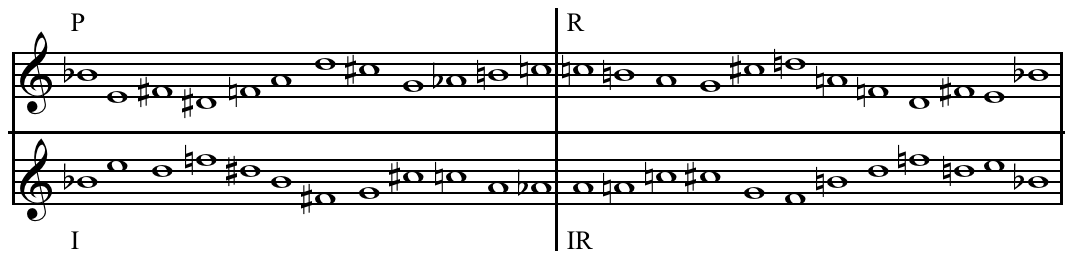
Tone Row
In music, a tone row or note row (german: Reihe or '), also series or set, is a non-repetitive ordering of a set of pitch-classes, typically of the twelve notes in musical set theory of the chromatic scale, though both larger and smaller sets are sometimes found. History and usage Tone rows are the basis of Arnold Schoenberg's twelve-tone technique and most types of serial music. Tone rows were widely used in 20th-century contemporary music, like Dmitri Shostakovich's use of twelve-tone rows, "without dodecaphonic transformations." A tone row has been identified in the A minor prelude, BWV 889, from book II of J.S. Bach's '' The Well-Tempered Clavier'' (1742) and by the late eighteenth century it is found in works such as Mozart's C major String Quartet, K. 157 (1772), String Quartet in E-flat major, K. 428, String Quintet in G minor, K. 516 (1790), and the Symphony in G minor, K. 550 (1788). Beethoven also used the technique but, on the whole, "Mozart seems to have ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Combinatoriality
In music using the twelve tone technique, combinatoriality is a quality shared by twelve-tone tone rows whereby each section of a row and a proportionate number of its transformations combine to form aggregates (all twelve tones). Whittall, Arnold. 2008. ''The Cambridge Introduction to Serialism. Cambridge Introductions to Music'', p. 272. New York: Cambridge University Press. (hardback) (pbk). Much as the pitches of an aggregate created by a tone row do not need to occur simultaneously, the pitches of a combinatorially created aggregate need not occur simultaneously. Arnold Schoenberg, creator of the twelve-tone technique, often combined P-0/I-5 to create "two aggregates, between the first hexachords of each, and the second hexachords of each, respectively." Combinatoriality is a side effect of derived rows, where the initial segment or set may be combined with its transformations (T,R,I,RI) to create an entire row. "Derivation refers to a process whereby, for instance, the in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hexachord
In music, a hexachord (also hexachordon) is a six-note series, as exhibited in a scale ( hexatonic or hexad) or tone row. The term was adopted in this sense during the Middle Ages and adapted in the 20th century in Milton Babbitt's serial theory. The word is taken from the gr, ἑξάχορδος, compounded from ἕξ (''hex'', six) and χορδή (''chordē'', string f the lyre whence "note"), and was also the term used in music theory up to the 18th century for the interval of a sixth ("hexachord major" being the major sixth and "hexachord minor" the minor sixth). Middle Ages The hexachord as a mnemonic device was first described by Guido of Arezzo, in his ''Epistola de ignoto cantu''. In each hexachord, all adjacent pitches are a whole tone apart, except for the middle two, which are separated by a semitone. These six pitches are named ''ut'', ''re'', ''mi'', ''fa'', ''sol'', and ''la'', with the semitone between ''mi'' and ''fa''. These six names are derived from the fir ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pitch Class
In music, a pitch class (p.c. or pc) is a set of all pitches that are a whole number of octaves apart; for example, the pitch class C consists of the Cs in all octaves. "The pitch class C stands for all possible Cs, in whatever octave position." Important to musical set theory, a pitch class is "all pitches related to each other by octave, enharmonic equivalence, or both." Thus, using scientific pitch notation, the pitch class "C" is the set : = . Although there is no formal upper or lower limit to this sequence, only a few of these pitches are audible to humans. Pitch class is important because human pitch-perception is periodic: pitches belonging to the same pitch class are perceived as having a similar quality or color, a property called " octave equivalence". Psychologists refer to the quality of a pitch as its "chroma". A ''chroma'' is an attribute of pitches (as opposed to ''tone height''), just like hue is an attribute of color. A ''pitch class'' is a set of al ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Side-slipping Complementation
In jazz improvisation, outside playing describes an approach where one plays over a scale, mode or chord that is harmonically distant from the given chord. There are several common techniques to playing outside, that include side-stepping or side-slipping, superimposition of Coltrane changes, and polytonality. Side-slipping The term side-slipping or side-stepping has been used to describe several similar yet distinct methods of playing outside. In one version, one plays only the five "'wrong'" non-scale notes for the given chord and none of the seven scale or three to four chord tones, given that there are twelve notes in the equal tempered scale and heptatonic scales are generally used. Another technique described as sideslipping is the addition of distant ii–V relationships, such as a half-step above the original ii–V. This increases chromatic tension as it first moves away and then towards the tonic. Lastly, side-slipping can be described as playing in a scale a half-st ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |