|
Projective Cone
A projective cone (or just cone) in projective geometry is the union of all lines that intersect a projective subspace ''R'' (the apex of the cone) and an arbitrary subset ''A'' (the basis) of some other subspace ''S'', disjoint from ''R''. In the special case that ''R'' is a single point, ''S'' is a plane, and ''A'' is a conic section on ''S'', the projective cone is a conical surface; hence the name. Definition Let ''X'' be a projective space over some field ''K'', and ''R'', ''S'' be disjoint subspaces of ''X''. Let ''A'' be an arbitrary subset of ''S''. Then we define ''RA'', the cone with top ''R'' and basis ''A'', as follows : * When ''A'' is empty, ''RA'' = ''A''. * When ''A'' is not empty, ''RA'' consists of all those points on a line connecting a point on ''R'' and a point on ''A''. Properties * As ''R'' and ''S'' are disjoint, one may deduce from linear algebra and the definition of a projective space that every point on ''RA'' not in ''R'' or ''A'' is on exactly ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Projective Geometry
In mathematics, projective geometry is the study of geometric properties that are invariant with respect to projective transformations. This means that, compared to elementary Euclidean geometry, projective geometry has a different setting (''projective space'') and a selective set of basic geometric concepts. The basic intuitions are that projective space has more points than Euclidean space, for a given dimension, and that geometric transformations are permitted that transform the extra points (called "Point at infinity, points at infinity") to Euclidean points, and vice versa. Properties meaningful for projective geometry are respected by this new idea of transformation, which is more radical in its effects than can be expressed by a transformation matrix and translation (geometry), translations (the affine transformations). The first issue for geometers is what kind of geometry is adequate for a novel situation. Unlike in Euclidean geometry, the concept of an angle does not ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Conic Section
A conic section, conic or a quadratic curve is a curve obtained from a cone's surface intersecting a plane. The three types of conic section are the hyperbola, the parabola, and the ellipse; the circle is a special case of the ellipse, though it was sometimes considered a fourth type. The ancient Greek mathematicians studied conic sections, culminating around 200 BC with Apollonius of Perga's systematic work on their properties. The conic sections in the Euclidean plane have various distinguishing properties, many of which can be used as alternative definitions. One such property defines a non-circular conic to be the set of those points whose distances to some particular point, called a '' focus'', and some particular line, called a ''directrix'', are in a fixed ratio, called the ''eccentricity''. The type of conic is determined by the value of the eccentricity. In analytic geometry, a conic may be defined as a plane algebraic curve of degree 2; that is, as the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Conical Surface
In geometry, a conical surface is an unbounded surface in three-dimensional space formed from the union of infinite lines that pass through a fixed point and a space curve. Definitions A (''general'') conical surface is the unbounded surface formed by the union of all the straight lines that pass through a fixed point — the ''apex'' or ''vertex'' — and any point of some fixed space curve — the ''directrix'' — that does not contain the apex. Each of those lines is called a ''generatrix'' of the surface. The directrix is often taken as a plane curve, in a plane not containing the apex, but this is not a requirement. In general, a conical surface consists of two congruent unbounded halves joined by the apex. Each half is called a nappe, and is the union of all the rays that start at the apex and pass through a point of some fixed space curve. Sometimes the term "conical surface" is used to mean just one nappe. Special cases If the directrix is a circl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Point (geometry)
In geometry, a point is an abstract idealization of an exact position, without size, in physical space, or its generalization to other kinds of mathematical spaces. As zero-dimensional objects, points are usually taken to be the fundamental indivisible elements comprising the space, of which one-dimensional curves, two-dimensional surfaces, and higher-dimensional objects consist. In classical Euclidean geometry, a point is a primitive notion, defined as "that which has no part". Points and other primitive notions are not defined in terms of other concepts, but only by certain formal properties, called axioms, that they must satisfy; for example, ''"there is exactly one straight line that passes through two distinct points"''. As physical diagrams, geometric figures are made with tools such as a compass, scriber, or pen, whose pointed tip can mark a small dot or prick a small hole representing a point, or can be drawn across a surface to represent a curve. A po ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Linear Algebra
Linear algebra is the branch of mathematics concerning linear equations such as :a_1x_1+\cdots +a_nx_n=b, linear maps such as :(x_1, \ldots, x_n) \mapsto a_1x_1+\cdots +a_nx_n, and their representations in vector spaces and through matrix (mathematics), matrices. Linear algebra is central to almost all areas of mathematics. For instance, linear algebra is fundamental in modern presentations of geometry, including for defining basic objects such as line (geometry), lines, plane (geometry), planes and rotation (mathematics), rotations. Also, functional analysis, a branch of mathematical analysis, may be viewed as the application of linear algebra to Space of functions, function spaces. Linear algebra is also used in most sciences and fields of engineering because it allows mathematical model, modeling many natural phenomena, and computing efficiently with such models. For nonlinear systems, which cannot be modeled with linear algebra, it is often used for dealing with first-order a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Finite Field
In mathematics, a finite field or Galois field (so-named in honor of Évariste Galois) is a field (mathematics), field that contains a finite number of Element (mathematics), elements. As with any field, a finite field is a Set (mathematics), set on which the operations of multiplication, addition, subtraction and division are defined and satisfy certain basic rules. The most common examples of finite fields are the integers mod n, integers mod p when p is a prime number. The ''order'' of a finite field is its number of elements, which is either a prime number or a prime power. For every prime number p and every positive integer k there are fields of order p^k. All finite fields of a given order are isomorphism, isomorphic. Finite fields are fundamental in a number of areas of mathematics and computer science, including number theory, algebraic geometry, Galois theory, finite geometry, cryptography and coding theory. Properties A finite field is a finite set that is a fiel ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
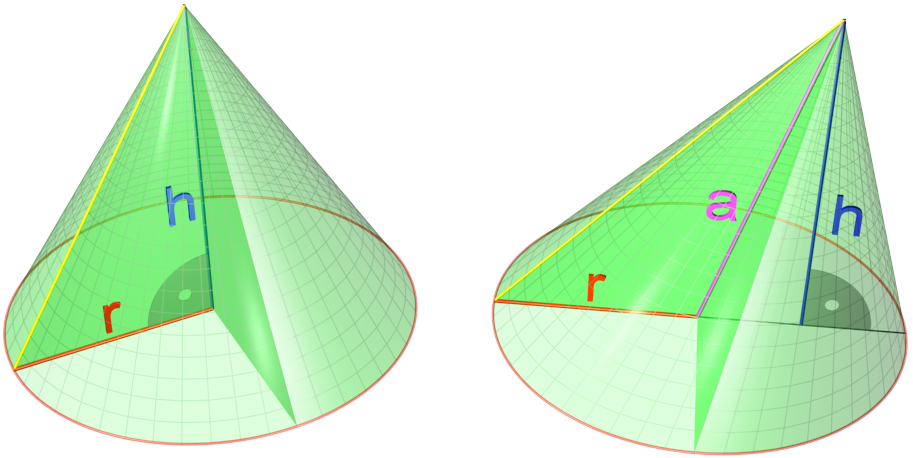
Cone (geometry)
In geometry, a cone is a three-dimensional figure that tapers smoothly from a flat base (typically a circle) to a point not contained in the base, called the '' apex'' or '' vertex''. A cone is formed by a set of line segments, half-lines, or lines connecting a common point, the apex, to all of the points on a base. In the case of line segments, the cone does not extend beyond the base, while in the case of half-lines, it extends infinitely far. In the case of lines, the cone extends infinitely far in both directions from the apex, in which case it is sometimes called a ''double cone''. Each of the two halves of a double cone split at the apex is called a ''nappe''. Depending on the author, the base may be restricted to a circle, any one-dimensional quadratic form in the plane, any closed one-dimensional figure, or any of the above plus all the enclosed points. If the enclosed points are included in the base, the cone is a solid object; otherwise it is an open surface ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Cone (algebraic Geometry)
In algebraic geometry, a cone is a generalization of a vector bundle. Specifically, given a scheme ''X'', the relative Spec :C = \operatorname_X R of a quasi-coherent graded ''O''''X''-algebra ''R'' is called the cone or affine cone of ''R''. Similarly, the relative Proj :\mathbb(C) = \operatorname_X R is called the projective cone of ''C'' or ''R''. Note: The cone comes with the \mathbb_m-action due to the grading of ''R''; this action is a part of the data of a cone (whence the terminology). Examples *If ''X'' = Spec ''k'' is a point and ''R'' is a homogeneous coordinate ring, then the affine cone of ''R'' is the (usual) affine cone over the projective variety corresponding to ''R''. *If R = \bigoplus_0^\infty I^n/I^ for some ideal sheaf ''I'', then \operatorname_X R is the normal cone to the closed scheme determined by ''I''. *If R = \bigoplus_0^\infty L^ for some line bundle ''L'', then \operatorname_X R is the total space of the dual of ''L''. *More generally, given a ve ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Cone (topology)
In topology, especially algebraic topology, the cone of a topological space X is intuitively obtained by stretching ''X'' into a cylinder and then collapsing one of its end faces to a point. The cone of X is denoted by CX or by \operatorname(X). Definitions Formally, the cone of ''X'' is defined as: :CX = (X \times ,1\cup_p v\ =\ \varinjlim \bigl( (X \times ,1 \hookleftarrow (X\times \) \xrightarrow v\bigr), where v is a point (called the vertex of the cone) and p is the projection to that point. In other words, it is the result of attaching the cylinder X \times ,1/math> by its face X\times\ to a point v along the projection p: \bigl( X\times\ \bigr)\to v. If X is a non-empty compact subspace of Euclidean space, the cone on X is homeomorphic to the union of segments from X to any fixed point v \not\in X such that these segments intersect only in v itself. That is, the topological cone agrees with the geometric cone for compact spaces when the latter is defined. H ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Cone (linear Algebra)
In linear algebra, a cone—sometimes called a linear cone to distinguish it from other sorts of cones—is a subset of a real vector space that is closed under positive scalar multiplication; that is, C is a cone if x\in C implies sx\in C for every . This is a broad generalization of the standard cone in Euclidean space. A convex cone is a cone that is also closed under addition, or, equivalently, a subset of a vector space that is closed under linear combinations with positive coefficients. It follows that convex cones are convex sets. The definition of a convex cone makes sense in a vector space over any ordered field, although the field of real numbers is used most often. Definition A subset C of a vector space is a cone if x\in C implies sx\in C for every s>0. Here s>0 refers to (strict) positivity in the scalar field. Competing definitions Some other authors require ,\infty)C\subset C or even 0\in C. Some require a cone to be convex and/or satisfy C\cap-C\subset\. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Conic Section
A conic section, conic or a quadratic curve is a curve obtained from a cone's surface intersecting a plane. The three types of conic section are the hyperbola, the parabola, and the ellipse; the circle is a special case of the ellipse, though it was sometimes considered a fourth type. The ancient Greek mathematicians studied conic sections, culminating around 200 BC with Apollonius of Perga's systematic work on their properties. The conic sections in the Euclidean plane have various distinguishing properties, many of which can be used as alternative definitions. One such property defines a non-circular conic to be the set of those points whose distances to some particular point, called a '' focus'', and some particular line, called a ''directrix'', are in a fixed ratio, called the ''eccentricity''. The type of conic is determined by the value of the eccentricity. In analytic geometry, a conic may be defined as a plane algebraic curve of degree 2; that is, as the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Ruled Surface
In geometry, a Differential geometry of surfaces, surface in 3-dimensional Euclidean space is ruled (also called a scroll) if through every Point (geometry), point of , there is a straight line that lies on . Examples include the plane (mathematics), plane, the lateral surface of a cylinder (geometry), cylinder or cone (geometry), cone, a conical surface with ellipse, elliptical directrix (rational normal scroll), directrix, the right conoid, the helicoid, and the tangent developable of a smooth curve in space. A ruled surface can be described as the set of points swept by a moving straight line. For example, a cone is formed by keeping one point of a line fixed whilst moving another point along a circle. A surface is doubly ruled if through every one of its points there are two distinct lines that lie on the surface. The hyperbolic paraboloid and the hyperboloid of one sheet are doubly ruled surfaces. The plane is the only surface which contains at least three distinct lines thr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |