|
Prime-counting Function
In mathematics, the prime-counting function is the function counting the number of prime numbers less than or equal to some real number ''x''. It is denoted by (''x'') (unrelated to the number ). History Of great interest in number theory is the growth rate of the prime-counting function. It was conjectured in the end of the 18th century by Gauss and by Legendre to be approximately : \frac x where log is the natural logarithm, in the sense that :\lim_ \frac=1. This statement is the prime number theorem. An equivalent statement is :\lim_\pi(x) / \operatorname(x)=1 where li is the logarithmic integral function. The prime number theorem was first proved in 1896 by Jacques Hadamard and by Charles de la Vallée Poussin independently, using properties of the Riemann zeta function introduced by Riemann in 1859. Proofs of the prime number theorem not using the zeta function or complex analysis were found around 1948 by Atle Selberg and by Paul Erdős (for the most ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics with the major subdisciplines of number theory, algebra, geometry, and analysis, respectively. There is no general consensus among mathematicians about a common definition for their academic discipline. Most mathematical activity involves the discovery of properties of abstract objects and the use of pure reason to prove them. These objects consist of either abstractions from nature orin modern mathematicsentities that are stipulated to have certain properties, called axioms. A ''proof'' consists of a succession of applications of deductive rules to already established results. These results include previously proved theorems, axioms, andin case of abstraction from naturesome basic properties that are considered true starting poin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Complex Analysis
Complex analysis, traditionally known as the theory of functions of a complex variable, is the branch of mathematical analysis that investigates functions of complex numbers. It is helpful in many branches of mathematics, including algebraic geometry, number theory, analytic combinatorics, applied mathematics; as well as in physics, including the branches of hydrodynamics, thermodynamics, and particularly quantum mechanics. By extension, use of complex analysis also has applications in engineering fields such as nuclear, aerospace, mechanical and electrical engineering. As a differentiable function of a complex variable is equal to its Taylor series (that is, it is analytic), complex analysis is particularly concerned with analytic functions of a complex variable (that is, holomorphic functions). History Complex analysis is one of the classical branches in mathematics, with roots in the 18th century and just prior. Important mathematicians associated with comple ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
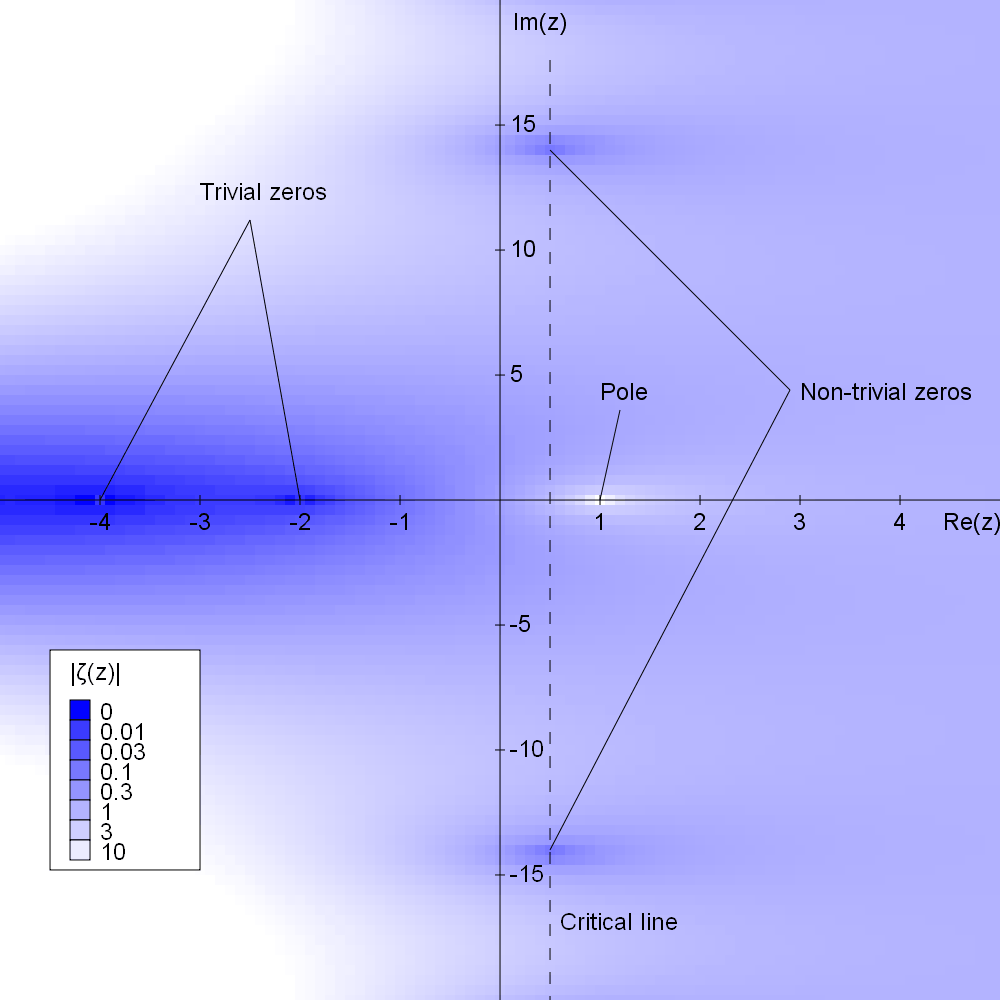
Riemann Hypothesis
In mathematics, the Riemann hypothesis is the conjecture that the Riemann zeta function has its zeros only at the negative even integers and complex numbers with real part . Many consider it to be the most important unsolved problem in pure mathematics. It is of great interest in number theory because it implies results about the distribution of prime numbers. It was proposed by , after whom it is named. The Riemann hypothesis and some of its generalizations, along with Goldbach's conjecture and the twin prime conjecture, make up Hilbert's eighth problem in David Hilbert's list of twenty-three unsolved problems; it is also one of the Clay Mathematics Institute's Millennium Prize Problems, which offers a million dollars to anyone who solves any of them. The name is also used for some closely related analogues, such as the Riemann hypothesis for curves over finite fields. The Riemann zeta function ζ(''s'') is a function whose argument ''s'' may be any complex number ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematics Of Computation
''Mathematics of Computation'' is a bimonthly mathematics journal focused on computational mathematics. It was established in 1943 as ''Mathematical Tables and other Aids to Computation'', obtaining its current name in 1960. Articles older than five years are available electronically free of charge. Abstracting and indexing The journal is abstracted and indexed in Mathematical Reviews ''Mathematical Reviews'' is a journal published by the American Mathematical Society (AMS) that contains brief synopses, and in some cases evaluations, of many articles in mathematics, statistics, and theoretical computer science. The AMS also pu ..., Zentralblatt MATH, Science Citation Index, CompuMath Citation Index, and Current Contents/Physical, Chemical & Earth Sciences. According to the '' Journal Citation Reports'', the journal has a 2020 impact factor of 2.417. References External links * Delayed open access journals English-language journals Mathematics journals Public ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Exponential Integral
In mathematics, the exponential integral Ei is a special function on the complex plane. It is defined as one particular definite integral of the ratio between an exponential function and its argument. Definitions For real non-zero values of ''x'', the exponential integral Ei(''x'') is defined as : \operatorname(x) = -\int_^\infty \fract\,dt = \int_^x \fract\,dt. The Risch algorithm shows that Ei is not an elementary function. The definition above can be used for positive values of ''x'', but the integral has to be understood in terms of the Cauchy principal value due to the singularity of the integrand at zero. For complex values of the argument, the definition becomes ambiguous due to branch points at 0 and Instead of Ei, the following notation is used, :E_1(z) = \int_z^\infty \frac\, dt,\qquad, (z), 0. Properties Several properties of the exponential integral below, in certain cases, allow one to avoid its explicit evaluation through the definition above. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Branch Cut
In the mathematical field of complex analysis, a branch point of a multi-valued function (usually referred to as a "multifunction" in the context of complex analysis) is a point such that if the function is n-valued (has n values) at that point, all of its neighborhoods contain a point that has more than n values. Multi-valued functions are rigorously studied using Riemann surfaces, and the formal definition of branch points employs this concept. Branch points fall into three broad categories: algebraic branch points, transcendental branch points, and logarithmic branch points. Algebraic branch points most commonly arise from functions in which there is an ambiguity in the extraction of a root, such as solving the equation ''w''2 = ''z'' for ''w'' as a function of ''z''. Here the branch point is the origin, because the analytic continuation of any solution around a closed loop containing the origin will result in a different function: there is non-trivial monodromy. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Logarithmic Integral Function
In mathematics, the logarithmic integral function or integral logarithm li(''x'') is a special function. It is relevant in problems of physics and has number theoretic significance. In particular, according to the prime number theorem, it is a very good approximation to the prime-counting function, which is defined as the number of prime numbers less than or equal to a given value x. Integral representation The logarithmic integral has an integral representation defined for all positive real numbers ≠ 1 by the definite integral : \operatorname(x) = \int_0^x \frac. Here, denotes the natural logarithm. The function has a singularity at , and the integral for is interpreted as a Cauchy principal value, : \operatorname(x) = \lim_ \left( \int_0^ \frac + \int_^x \frac \right). Offset logarithmic integral The offset logarithmic integral or Eulerian logarithmic integral is defined as : \operatorname(x) = \int_2^x \frac = \operatorname(x) - \operatorname(2). As ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Möbius Function
The Möbius function is a multiplicative function in number theory introduced by the German mathematician August Ferdinand Möbius (also transliterated ''Moebius'') in 1832. It is ubiquitous in elementary and analytic number theory and most often appears as part of its namesake the Möbius inversion formula. Following work of Gian-Carlo Rota in the 1960s, generalizations of the Möbius function were introduced into combinatorics, and are similarly denoted . Definition For any positive integer , define as the sum of the primitive th roots of unity. It has values in depending on the factorization of into prime factors: * if is a square-free positive integer with an even number of prime factors. * if is a square-free positive integer with an odd number of prime factors. * if has a squared prime factor. The Möbius function can alternatively be represented as : \mu(n) = \delta_ \lambda(n), where is the Kronecker delta, is the Liouville function, is the numb ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
On The Number Of Primes Less Than A Given Magnitude
" die Anzahl der Primzahlen unter einer gegebenen " (usual English translation: "On the Number of Primes Less Than a Given Magnitude") is seminal9-page paper by Bernhard Riemann published in the November 1859 edition of the ''Monatsberichte der Königlich Preußischen Akademie der Wissenschaften zu Berlin''. Overview This paper studies the prime-counting function using analytic methods. Although it is the only paper Riemann ever published on number theory, it contains ideas which influenced thousands of researchers during the late 19th century and up to the present day. The paper consists primarily of definitions, heuristic arguments, sketches of proofs, and the application of powerful analytic methods; all of these have become essential concepts and tools of modern analytic number theory. Among the new definitions, ideas, and notation introduced: *The use of the Greek letter zeta (ζ) for a function previously mentioned by Euler *The analytic continuation of this zet ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Skewes' Number
In number theory, Skewes's number is any of several large numbers used by the South African mathematician Stanley Skewes as upper bounds for the smallest natural number x for which :\pi(x) > \operatorname(x), where is the prime-counting function and is the logarithmic integral function. Skewes's number is much larger, but it is now known that there is a crossing between \pi(x) \operatorname(x) near e^ < 1.397 \times 10^. It is not known whether it is the smallest crossing. Skewes's numbers , who was Skewes's research supervisor, had proved in that there is such a number (and so, a first such number); ...[...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kevin Ford (mathematician)
Kevin B. Ford (born 22 December 1967) is an American mathematician working in analytic number theory. Education and career He has been a professor in the department of mathematics of the University of Illinois at Urbana-Champaign since 2001. Prior to this appointment, he was a faculty member at the University of South Carolina. Ford received a Bachelor of Science in Computer Science and Mathematics in 1990 from the California State University, Chico. He then attended the University of Illinois at Urbana-Champaign, where he completed his doctoral studies in 1994 under the supervision of Heini Halberstam. Research Ford's early work focused on the distribution of Euler's totient function. In 1998, he published a paper that studied in detail the range of this function and established that Carmichael's totient function conjecture is true for all integers up to 10^. In 1999, he settled Sierpinski’s conjecture. In August 2014, Kevin Ford, in collaboration with Green, Konyagi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Big O Notation
Big ''O'' notation is a mathematical notation that describes the limiting behavior of a function when the argument tends towards a particular value or infinity. Big O is a member of a family of notations invented by Paul Bachmann, Edmund Landau, and others, collectively called Bachmann–Landau notation or asymptotic notation. The letter O was chosen by Bachmann to stand for '' Ordnung'', meaning the order of approximation. In computer science, big O notation is used to classify algorithms according to how their run time or space requirements grow as the input size grows. In analytic number theory, big O notation is often used to express a bound on the difference between an arithmetical function and a better understood approximation; a famous example of such a difference is the remainder term in the prime number theorem. Big O notation is also used in many other fields to provide similar estimates. Big O notation characterizes functions according to their growth rates: d ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |