|
Polytropic Index
A polytropic process is a thermodynamic process that obeys the relation: p V^ = C where ''p'' is the pressure, ''V'' is volume, ''n'' is the polytropic index, and ''C'' is a constant. The polytropic process equation describes expansion and compression processes which include heat transfer. Particular cases Some specific values of ''n'' correspond to particular cases: * n=0 for an isobaric process, * n=+\infty for an isochoric process. In addition, when the ideal gas law applies: * n=1 for an isothermal process, * n=\gamma for an isentropic process. Where \gamma is the ratio of the heat capacity at constant pressure (C_P) to heat capacity at constant volume (C_V). Equivalence between the polytropic coefficient and the ratio of energy transfers For an ideal gas in a closed system undergoing a slow process with negligible changes in kinetic and potential energy the process is polytropic, such that p v^ = C where ''C'' is a constant, K = \frac, \gamma = \frac, and with the poly ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Biogeography
Biogeography is the study of the distribution of species and ecosystems in geographic space and through geological time. Organisms and biological communities often vary in a regular fashion along geographic gradients of latitude, elevation, isolation and habitat area.Brown University, "Biogeography." Accessed February 24, 2014. . Phytogeography is the branch of biogeography that studies the distribution of plants. Zoogeography is the branch that studies distribution of animals. Mycogeography is the branch that studies distribution of fungi, such as mushrooms. Knowledge of spatial variation in the numbers and types of organisms is as vital to us today as it was to our early human ancestors, as we adapt to heterogeneous but geographically predictable environments. Biogeography is an integrative field of inquiry that unites concepts and information from ecology, evolutionary biology, taxonomy, geology, physical geography, palaeontology, and climatology.Dansereau, Pierre. 1957 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Explosion
An explosion is a rapid expansion in volume associated with an extreme outward release of energy, usually with the generation of high temperatures and release of high-pressure gases. Supersonic explosions created by high explosives are known as detonations and travel through shock waves. Subsonic explosions are created by low explosives through a slower combustion process known as deflagration. Causes Explosions can occur in nature due to a large influx of energy. Most natural explosions arise from volcanic or stellar processes of various sorts. Explosive volcanic eruptions occur when magma rises from below, it has very dissolved gas in it. The reduction of pressure as the magma rises and causes the gas to bubble out of solution, resulting in a rapid increase in volume. Explosions also occur as a result of impact events and in phenomena such as hydrothermal explosions (also due to volcanic processes). Explosions can also occur outside of Earth in the universe in events s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Isentropic Process
In thermodynamics, an isentropic process is an idealized thermodynamic process that is both adiabatic and reversible. The work transfers of the system are frictionless, and there is no net transfer of heat or matter. Such an idealized process is useful in engineering as a model of and basis of comparison for real processes. This process is idealized because reversible processes do not occur in reality; thinking of a process as both adiabatic and reversible would show that the initial and final entropies are the same, thus, the reason it is called isentropic (entropy does not change). Thermodynamic processes are named based on the effect they would have on the system (ex. isovolumetric: constant volume, isenthalpic: constant enthalpy). Even though in reality it is not necessarily possible to carry out an isentropic process, some may be approximated as such. The word "isentropic" can be interpreted in another way, since its meaning is deducible from its etymology. It means a pro ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Internal Combustion Engine
An internal combustion engine (ICE or IC engine) is a heat engine in which the combustion of a fuel occurs with an oxidizer (usually air) in a combustion chamber that is an integral part of the working fluid flow circuit. In an internal combustion engine, the expansion of the high-temperature and high-pressure gases produced by combustion applies direct force to some component of the engine. The force is typically applied to pistons ( piston engine), turbine blades (gas turbine), a rotor (Wankel engine), or a nozzle ( jet engine). This force moves the component over a distance, transforming chemical energy into kinetic energy which is used to propel, move or power whatever the engine is attached to. This replaced the external combustion engine for applications where the weight or size of an engine was more important. The first commercially successful internal combustion engine was created by Étienne Lenoir around 1860, and the first modern internal combustion engine, known ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Compressor
A compressor is a mechanical device that increases the pressure of a gas by reducing its volume. An air compressor is a specific type of gas compressor. Compressors are similar to pumps: both increase the pressure on a fluid and both can transport the fluid through a pipe. The main distinction is that the focus of a compressor is to change the density or volume of the fluid, which is mostly only achievable on gases. Gases are compressible, while liquids are relatively incompressible, so compressors are rarely used for liquids. The main action of a pump is to pressurize and transport liquids. Many compressors can be staged, that is, the fluid is compressed several times in steps or stages, to increase discharge pressure. Often, the second stage is physically smaller than the primary stage, to accommodate the already compressed gas without reducing its pressure. Each stage further compresses the gas and increases its pressure and also temperature (if inter cooling between stages i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Adiabatic Process
In thermodynamics, an adiabatic process (Greek: ''adiábatos'', "impassable") is a type of thermodynamic process that occurs without transferring heat or mass between the thermodynamic system and its environment. Unlike an isothermal process, an adiabatic process transfers energy to the surroundings only as work.. A translation may be founhere. Also a mostly reliabltranslation is to be foundin As a key concept in thermodynamics, the adiabatic process supports the theory that explains the first law of thermodynamics. Some chemical and physical processes occur too rapidly for energy to enter or leave the system as heat, allowing a convenient "adiabatic approximation".Bailyn, M. (1994), pp. 52–53. For example, the adiabatic flame temperature uses this approximation to calculate the upper limit of flame temperature by assuming combustion loses no heat to its surroundings. In meteorology and oceanography, adiabatic cooling produces condensation of moisture or salinity, oversatu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Polytrope
In astrophysics, a polytrope refers to a solution of the Lane–Emden equation in which the pressure depends upon the density in the form :P = K \rho^, where is pressure, is density and is a constant of proportionality. The constant is known as the polytropic index; note however that the polytropic index has an alternative definition as with ''n'' as the exponent. This relation need not be interpreted as an equation of state, which states ''P'' as a function of both ρ and ''T'' (the temperature); however in the particular case described by the polytrope equation there are other additional relations between these three quantities, which together determine the equation. Thus, this is simply a relation that expresses an assumption about the change of pressure with radius in terms of the change of density with radius, yielding a solution to the Lane–Emden equation. Sometimes the word ''polytrope'' may refer to an equation of state that looks similar to the thermodyna ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
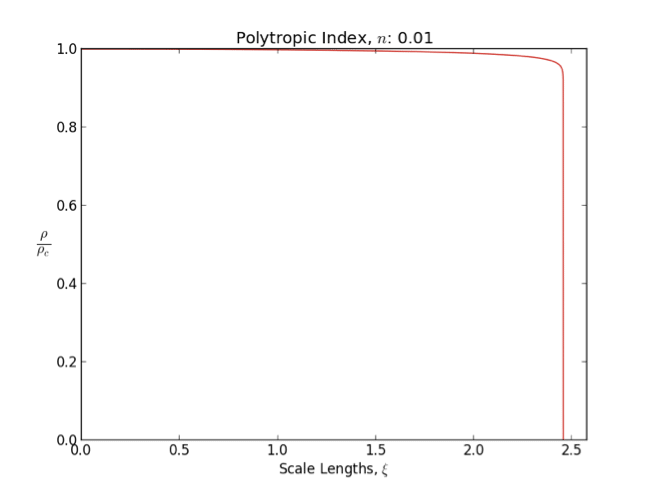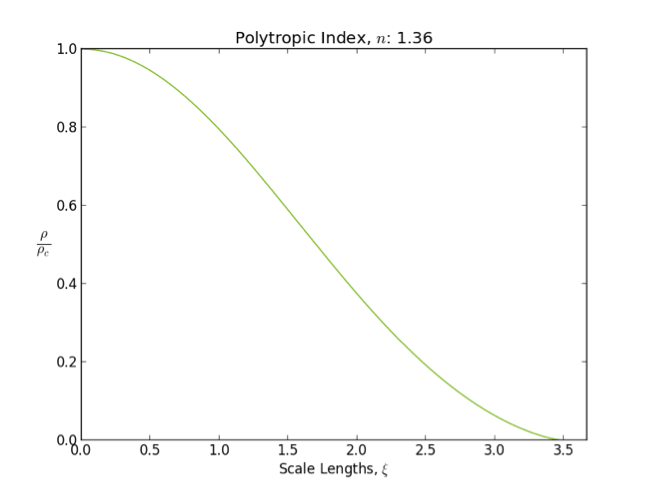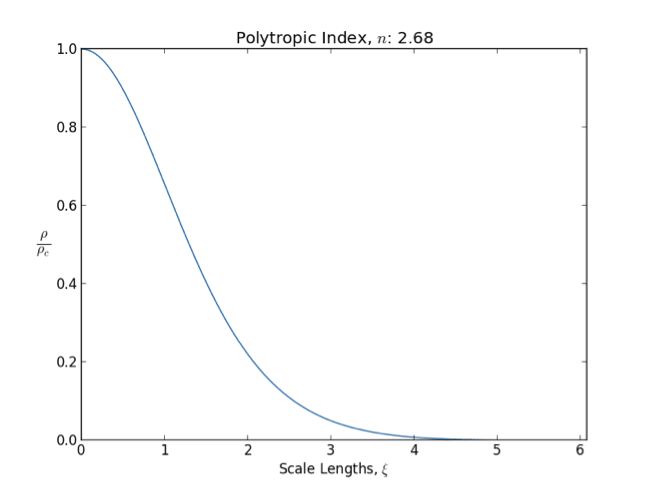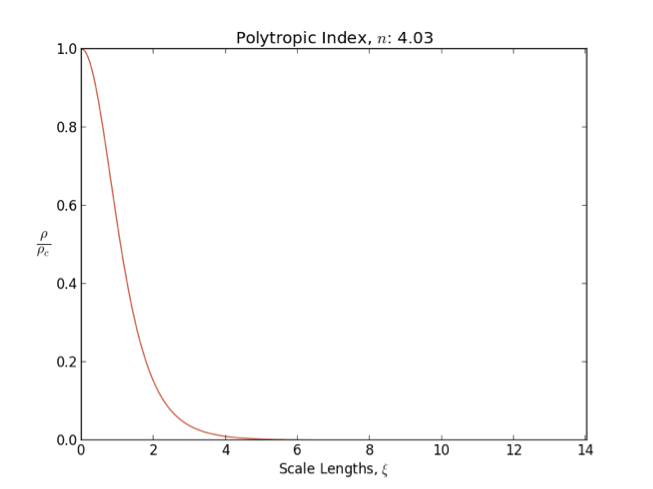
Lane–Emden Equation
In astrophysics, the Lane–Emden equation is a dimensionless form of Poisson's equation for the gravitational potential of a Newtonian self-gravitating, spherically symmetric, polytropic fluid. It is named after astrophysicists Jonathan Homer Lane and Robert Emden. The equation reads : \frac \frac \left(\right) + \theta^n = 0, where \xi is a dimensionless radius and \theta is related to the density, and thus the pressure, by \rho=\rho_c\theta^n for central density \rho_c. The index n is the polytropic index that appears in the polytropic equation of state, : P = K \rho^\, where P and \rho are the pressure and density, respectively, and K is a constant of proportionality. The standard boundary conditions are \theta(0)=1 and \theta'(0)=0. Solutions thus describe the run of pressure and density with radius and are known as ''polytropes'' of index n. If an isothermal fluid (polytropic index tends to infinity) is used instead of a polytropic fluid, one obtains the Emden–Chan ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mayer's Relation
In the 19th century, German chemist and physicist Julius von Mayer derived a relation between specific heat at constant pressure and the specific heat at constant volume for an ideal gas. Mayer's relation states that :C_ - C_ = R, where is the molar specific heat at constant pressure, is the molar specific heat at constant volume and is the gas constant. For more general homogeneous substances, not just ideal gases, the difference takes the form, :C_ - C_= V_ T\frac\, (see relations between heat capacities), where V_ is the molar volume, T is the temperature, \alpha_ is the thermal expansion coefficient and \beta is the isothermal compressibility. From this latter relation, several inferences can be made: * Since the isothermal compressibility \beta_ is positive for nearly all phases, and the square of thermal expansion coefficient is always either a positive quantity or zero, the specific heat at constant pressure is nearly always greater than or equal to specific heat at c ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bounded Function
In mathematics, a function ''f'' defined on some set ''X'' with real or complex values is called bounded if the set of its values is bounded. In other words, there exists a real number ''M'' such that :, f(x), \le M for all ''x'' in ''X''. A function that is ''not'' bounded is said to be unbounded. If ''f'' is real-valued and ''f''(''x'') ≤ ''A'' for all ''x'' in ''X'', then the function is said to be bounded (from) above by ''A''. If ''f''(''x'') ≥ ''B'' for all ''x'' in ''X'', then the function is said to be bounded (from) below by ''B''. A real-valued function is bounded if and only if it is bounded from above and below. An important special case is a bounded sequence, where ''X'' is taken to be the set N of natural numbers. Thus a sequence ''f'' = (''a''0, ''a''1, ''a''2, ...) is bounded if there exists a real number ''M'' such that :, a_n, \le M for every natural number ''n''. The set of all bounded sequences forms the sequence space l^\infty. The definition of bound ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Volume (thermodynamics)
In thermodynamics, the volume of a system is an important extensive parameter for describing its thermodynamic state. The specific volume, an intensive property, is the system's volume per unit of mass. Volume is a function of state and is interdependent with other thermodynamic properties such as pressure and temperature. For example, volume is related to the pressure and temperature of an ideal gas by the ideal gas law. The physical volume of a system may or may not coincide with a control volume used to analyze the system. Overview The volume of a thermodynamic system typically refers to the volume of the working fluid, such as, for example, the fluid within a piston. Changes to this volume may be made through an application of work, or may be used to produce work. An isochoric process however operates at a constant-volume, thus no work can be produced. Many other thermodynamic processes will result in a change in volume. A polytropic process, in particular, causes change ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
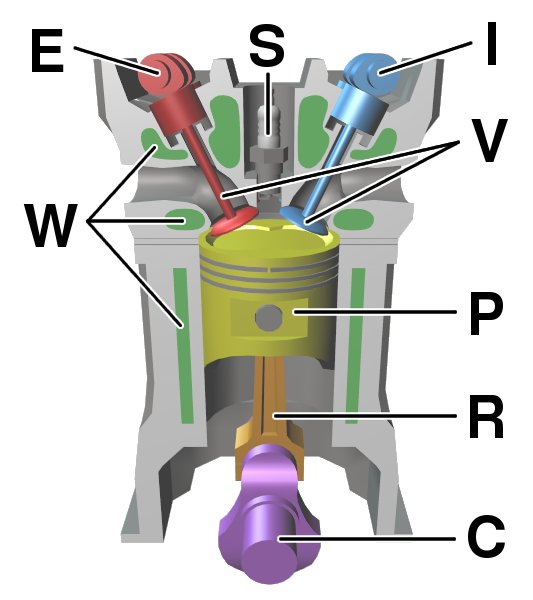
Internal Combustion Engine
An internal combustion engine (ICE or IC engine) is a heat engine in which the combustion of a fuel occurs with an oxidizer (usually air) in a combustion chamber that is an integral part of the working fluid flow circuit. In an internal combustion engine, the expansion of the high-temperature and high-pressure gases produced by combustion applies direct force to some component of the engine. The force is typically applied to pistons ( piston engine), turbine blades (gas turbine), a rotor (Wankel engine), or a nozzle ( jet engine). This force moves the component over a distance, transforming chemical energy into kinetic energy which is used to propel, move or power whatever the engine is attached to. This replaced the external combustion engine for applications where the weight or size of an engine was more important. The first commercially successful internal combustion engine was created by Étienne Lenoir around 1860, and the first modern internal combustion engine, known ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
