|
Photon Sphere
A photon sphere or photon circle is an area or region of space where gravity is so strong that photons are forced to travel in orbits, which is also sometimes called the last photon orbit. The radius of the photon sphere, which is also the lower bound for any stable orbit, is, for a Schwarzschild black hole, : r = \frac = \frac, where is the gravitational constant, is the black-hole mass, and is the speed of light in vacuum and is the Schwarzschild radius (the radius of the event horizon); see below for a derivation of this result. This equation entails that photon spheres can only exist in the space surrounding an extremely compact object (a black hole or possibly an "ultracompact" neutron star). The photon sphere is located farther from the center of a black hole than the event horizon. Within a photon sphere, it is possible to imagine a photon that's emitted from the back of one's head, orbiting the black hole, only then to be intercepted by the person's eyes, allowin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pseudoscientific
Pseudoscience consists of statements, beliefs, or practices that claim to be both scientific and factual but are incompatible with the scientific method. Pseudoscience is often characterized by contradictory, exaggerated or unfalsifiable claims; reliance on confirmation bias rather than rigorous attempts at refutation; lack of openness to evaluation by other experts; absence of systematic practices when developing hypotheses; and continued adherence long after the pseudoscientific hypotheses have been experimentally discredited. The demarcation between science and pseudoscience has scientific, philosophical, and political implications. Philosophers debate the nature of science and the general criteria for drawing the line between scientific theories and pseudoscientific beliefs, but there is general agreement on examples such as ancient astronauts, climate change denial, dowsing, evolution denial, Holocaust denialism, astrology, alchemy, alternative medicine, occulti ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Semi-major Axis
In geometry, the major axis of an ellipse is its longest diameter: a line segment that runs through the center and both foci, with ends at the two most widely separated points of the perimeter. The semi-major axis (major semiaxis) is the longest semidiameter or one half of the major axis, and thus runs from the centre, through a focus, and to the perimeter. The semi-minor axis (minor semiaxis) of an ellipse or hyperbola is a line segment that is at right angles with the semi-major axis and has one end at the center of the conic section. For the special case of a circle, the lengths of the semi-axes are both equal to the radius of the circle. The length of the semi-major axis of an ellipse is related to the semi-minor axis's length through the eccentricity and the semi-latus rectum \ell, as follows: The semi-major axis of a hyperbola is, depending on the convention, plus or minus one half of the distance between the two branches. Thus it is the distance from the cen ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Boyer–Lindquist Coordinates
In the mathematical description of general relativity, the Boyer–Lindquist coordinates are a generalization of the coordinates used for the metric of a Schwarzschild black hole that can be used to express the metric of a Kerr black hole. The Hamiltonian for test particle motion in Kerr spacetime is separable in Boyer–Lindquist coordinates. Using Hamilton–Jacobi theory one can derive a fourth constant of the motion known as Carter's constant. The 1967 paper introducing Boyer–Lindquist coordinates was a posthumous publication for Robert H. Boyer, who was killed in the 1966 University of Texas tower shooting. Line element The line element for a black hole with a total mass equivalent M, angular momentum J, and charge Q in Boyer–Lindquist coordinates and natural units (G=c=1) is : ds^2 = -\frac\left(dt - a \sin^2\theta \,d\phi \right)^2 +\frac\Big(\left(r^2+a^2\right)\,d\phi - a \,dt\Big)^2 + \fracdr^2 + \rho^2 \,d\theta^2 where :\Delta = r^2 - 2Mr + a^2 + Q^2, called th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
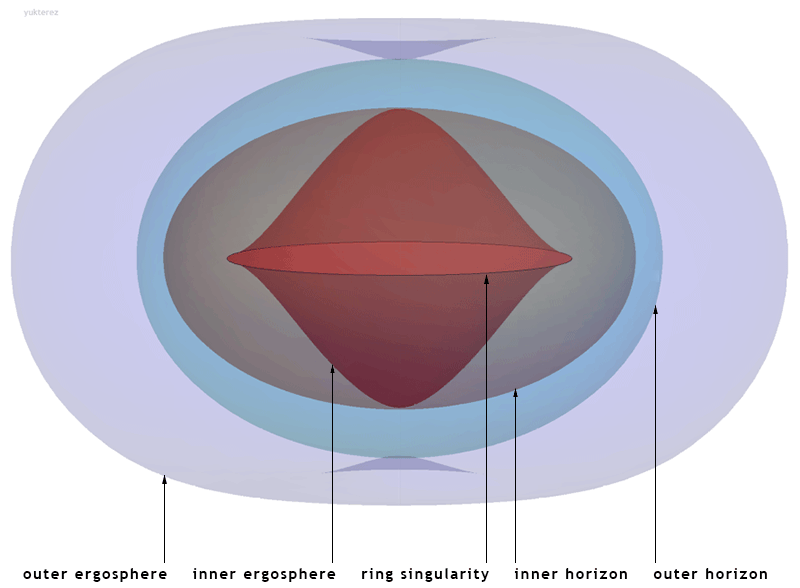
Kerr Black Hole
The Kerr metric or Kerr geometry describes the geometry of empty spacetime around a rotating uncharged axially symmetric black hole with a quasispherical event horizon. The Kerr metric is an exact solution of the Einstein field equations of general relativity; these equations are highly non-linear, which makes exact solutions very difficult to find. Overview The Kerr metric is a generalization to a rotating body of the Schwarzschild metric, discovered by Karl Schwarzschild in 1915, which described the geometry of spacetime around an uncharged, spherically symmetric, and non-rotating body. The corresponding solution for a ''charged'', spherical, non-rotating body, the Reissner–Nordström metric, was discovered soon afterwards (1916–1918). However, the exact solution for an uncharged, ''rotating'' black hole, the Kerr metric, remained unsolved until 1963, when it was discovered by Roy Kerr.Melia, Fulvio (2009). "Cracking the Einstein code: relativity and the birth of b ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kerr Photon Orbits With Orbital Inclination Thumbnail
{{Disambiguation, geo ...
Kerr may refer to: People *Kerr (surname) *Kerr (given name) Places ;United States *Kerr Township, Champaign County, Illinois *Kerr, Montana, A US census-designated place *Kerr, Ohio, an unincorporated community *Kerr County, Texas Other uses *KERR, A US radio station *Kerr, a brand of food Mason jars and lids *Clan Kerr, a Scottish clan * Kerr's, a Canadian candy company See also * * *Ker (other) Ker or KER may refer to: People * Ker (surname) * Ker, family name of the Dukes of Roxburghe * Ker, a spelling of the Guo surname * Frederick Ker, the appellant in the U.S. Supreme Court case ''Ker v. Illinois'' * Ker, a clan of the Bharwad p ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geodesic Equation
In geometry, a geodesic () is a curve representing in some sense the shortest path (arc) between two points in a surface, or more generally in a Riemannian manifold. The term also has meaning in any differentiable manifold with a connection. It is a generalization of the notion of a " straight line". The noun ''geodesic'' and the adjective '' geodetic'' come from ''geodesy'', the science of measuring the size and shape of Earth, though many of the underlying principles can be applied to any ellipsoidal geometry. In the original sense, a geodesic was the shortest route between two points on the Earth's surface. For a spherical Earth, it is a segment of a great circle (see also great-circle distance). The term has since been generalized to more abstract mathematical spaces; for example, in graph theory, one might consider a geodesic between two vertices/nodes of a graph. In a Riemannian manifold or submanifold, geodesics are characterised by the property of having van ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Schwarzschild Metric
In Einstein's theory of general relativity, the Schwarzschild metric (also known as the Schwarzschild solution) is an exact solution to the Einstein field equations that describes the gravitational field outside a spherical mass, on the assumption that the electric charge of the mass, angular momentum of the mass, and universal cosmological constant are all zero. The solution is a useful approximation for describing slowly rotating astronomical objects such as many stars and planets, including Earth and the Sun. It was found by Karl Schwarzschild in 1916, and around the same time independently by Johannes Droste, who published his more complete and modern-looking discussion four months after Schwarzschild. According to Birkhoff's theorem, the Schwarzschild metric is the most general spherically symmetric vacuum solution of the Einstein field equations. A Schwarzschild black hole or static black hole is a black hole that has neither electric charge nor angular momentum. A Sch ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Angular Velocity
In physics, angular velocity or rotational velocity ( or ), also known as angular frequency vector,(UP1) is a pseudovector representation of how fast the angular position or orientation of an object changes with time (i.e. how quickly an object rotates or revolves relative to a point or axis). The magnitude of the pseudovector represents the ''angular speed'', the rate at which the object rotates or revolves, and its direction is normal to the instantaneous plane of rotation or angular displacement. The orientation of angular velocity is conventionally specified by the right-hand rule.(EM1) There are two types of angular velocity. * Orbital angular velocity refers to how fast a point object revolves about a fixed origin, i.e. the time rate of change of its angular position relative to the origin. * Spin angular velocity refers to how fast a rigid body rotates with respect to its center of rotation and is independent of the choice of origin, in contrast to orbital angular ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Frame-dragging
Frame-dragging is an effect on spacetime, predicted by Albert Einstein's general theory of relativity, that is due to non-static stationary distributions of mass–energy. A stationary field is one that is in a steady state, but the masses causing that field may be non-static — rotating, for instance. More generally, the subject that deals with the effects caused by mass–energy currents is known as gravitoelectromagnetism, which is analogous to the magnetism of classical electromagnetism. The first frame-dragging effect was derived in 1918, in the framework of general relativity, by the Austrian physicists Josef Lense and Hans Thirring, and is also known as the Lense–Thirring effect. They predicted that the rotation of a massive object would distort the spacetime metric, making the orbit of a nearby test particle precess. This does not happen in Newtonian mechanics for which the gravitational field of a body depends only on its mass, not on its rotation. Th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rotating Black Hole
A rotating black hole is a black hole that possesses angular momentum. In particular, it rotates about one of its axes of symmetry. All celestial objects – planets, stars ( Sun), galaxies, black holes – spin. Types of black holes There are four known, exact, black hole solutions to the Einstein field equations, which describe gravity in general relativity. Two of those rotate: the Kerr and Kerr–Newman black holes. It is generally believed that every black hole decays rapidly to a stable black hole; and, by the no-hair theorem, that (except for quantum fluctuations) stable black holes can be completely described at any moment in time by these 11 numbers: * mass-energy ''M'', * linear momentum ''P'' (three components), * angular momentum ''J'' (three components), * position ''X'' (three components), * electric charge ''Q''. These numbers represent the conserved attributes of an object which can be determined from a distance by examining its electromagnetic and grav ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Centripetal Force
A centripetal force (from Latin ''centrum'', "center" and ''petere'', "to seek") is a force that makes a body follow a curved path. Its direction is always orthogonal to the motion of the body and towards the fixed point of the instantaneous center of curvature of the path. Isaac Newton described it as "a force by which bodies are drawn or impelled, or in any way tend, towards a point as to a centre". In Newtonian mechanics, gravity provides the centripetal force causing astronomical orbits. One common example involving centripetal force is the case in which a body moves with uniform speed along a circular path. The centripetal force is directed at right angles to the motion and also along the radius towards the centre of the circular path. The mathematical description was derived in 1659 by the Dutch physicist Christiaan Huygens. Formula The magnitude of the centripetal force on an object of mass ''m'' moving at tangential speed ''v'' along a path with radius of curvatu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Centrifugal Force
In Newtonian mechanics, the centrifugal force is an inertial force (also called a "fictitious" or "pseudo" force) that appears to act on all objects when viewed in a rotating frame of reference. It is directed away from an axis which is parallel to the axis of rotation and passing through the coordinate system's origin. If the axis of rotation passes through the coordinate system's origin, the centrifugal force is directed radially outwards from that axis. The magnitude of centrifugal force ''F'' on an object of mass ''m'' at the distance ''r'' from the origin of a frame of reference rotating with angular velocity is: F = m\omega^2 r The concept of centrifugal force can be applied in rotating devices, such as centrifuges, centrifugal pumps, centrifugal governors, and centrifugal clutches, and in centrifugal railways, planetary orbits and banked curves, when they are analyzed in a rotating coordinate system. Confusingly, the term has sometimes also been used for the rea ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |

.png)

.gif)
