|
Pentacube
image:tetracube_categories.svg, upAll 8 one-sided tetracubes – if chirality is ignored, the bottom 2 in grey are considered the same, giving 7 free tetracubes in total image:9L cube puzzle solution.svg, A puzzle involving arranging nine L tricubes into a 3×3 cube A polycube is a solid figure formed by joining one or more equal cube (geometry), cubes face to face. Polycubes are the three-dimensional analogues of the planar polyominoes. The Soma cube, the Bedlam cube, the Diabolical cube, the Slothouber–Graatsma puzzle, and the Conway puzzle are examples of packing problems based on polycubes. Enumerating polycubes image:AGK-pentacube.png, A Chirality (mathematics), chiral pentacube Like polyominoes, polycubes can be enumerated in two ways, depending on whether Chirality (mathematics), chiral pairs of polycubes are counted as one polycube or two. For example, 6 tetracubes have Reflection symmetry, mirror symmetry and one is Chirality (mathematics), chiral, giving a coun ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tetracube Categories
image:tetracube_categories.svg, upAll 8 one-sided tetracubes – if chirality is ignored, the bottom 2 in grey are considered the same, giving 7 free tetracubes in total image:9L cube puzzle solution.svg, A puzzle involving arranging nine L tricubes into a 3×3 cube A polycube is a solid figure formed by joining one or more equal cube (geometry), cubes face to face. Polycubes are the three-dimensional analogues of the planar polyominoes. The Soma cube, the Bedlam cube, the Diabolical cube, the Slothouber–Graatsma puzzle, and the Conway puzzle are examples of packing problems based on polycubes. Enumerating polycubes image:AGK-pentacube.png, A Chirality (mathematics), chiral pentacube Like polyominoes, polycubes can be enumerated in two ways, depending on whether Chirality (mathematics), chiral pairs of polycubes are counted as one polycube or two. For example, 6 tetracubes have Reflection symmetry, mirror symmetry and one is Chirality (mathematics), chiral, giving a coun ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pentomino
Derived from the Greek word for ' 5', and "domino", a pentomino (or 5-omino) is a polyomino of order 5, that is, a polygon in the plane made of 5 equal-sized squares connected edge-to-edge. When rotations and reflections are not considered to be distinct shapes, there are 12 different '' free'' pentominoes. When reflections are considered distinct, there are 18 '' one-sided'' pentominoes. When rotations are also considered distinct, there are 63 ''fixed'' pentominoes. Pentomino tiling puzzles and games are popular in recreational mathematics. Usually, video games such as ''Tetris'' imitations and ''Rampart'' consider mirror reflections to be distinct, and thus use the full set of 18 one-sided pentominoes. Each of the twelve pentominoes satisfies the Conway criterion; hence every pentomino is capable of tiling the plane. Each chiral pentomino can tile the plane without being reflected. History The earliest puzzle containing a complete set of pentominoes appeared in Henry D ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Soma Cube
The Soma cube is a solid dissection puzzle invented by Danish polymath Piet Hein in 1933 during a lecture on quantum mechanics conducted by Werner Heisenberg. Seven pieces made out of unit cubes must be assembled into a 3×3×3 cube. The pieces can also be used to make a variety of other 3D shapes. The pieces of the Soma cube consist of all possible combinations of three or four unit cubes, joined at their faces, such that at least one inside corner is formed. There is one combination of three cubes that satisfies this condition, and six combinations of four cubes that satisfy this condition, of which two are mirror images of each other (see Chirality). Thus, 3 + (6 × 4) is 27, which is exactly the number of cells in a 3×3×3 cube. The Soma cube was popularized by Martin Gardner in the September 1958 Mathematical Games column in ''Scientific American.'' The book '' Winning Ways for your Mathematical Plays'' also contains a detailed analysis of the Soma cube problem. Ther ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bedlam Cube
The Bedlam cube is a solid dissection puzzle invented by British puzzle expert Bruce Bedlam. Design The puzzle consists of thirteen polycubic pieces: twelve pentacubes and one tetracube. The objective is to assemble these pieces into a 4 x 4 x 4 cube. There are 19,186 distinct ways of doing so, up to rotations and reflections. The Bedlam cube is one unit per side larger than the 3 x 3 x 3 Soma cube, and is much more difficult to solve. History Two of the BBC's 'Dragons' from ''Dragons' Den'', Rachel Elnaugh and Theo Paphitis, were to invest in the Bedlam cube during the 2005 series. They offered £100,000 for a 30% share of equity in Bedlam Puzzles. Danny Bamping (the entrepreneur behind Bedlam cube) finally chose a bank loan instead of their investment, as seen in the relevant "Where Are They Now" episode of ''Dragons' Den''. Records According to ''Guinness World Records'', the official world record for assembling the Bedlam Cube is 11.03 seconds by Danny Bamping ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Diabolical Cube
The diabolical cube is a three-dimensional dissection puzzle consisting of six polycubes (shapes formed by gluing cubes together face to face) that can be assembled together to form a single 3 × 3 × 3 cube.. The six pieces are: one dicube, one tricube, one tetracube, one pentacube, one hexacube and one heptacube, that is, polycubes of 2, 3, 4, 5, 6 and 7 cubes. There are many similar variations of this type of puzzle, including the Soma cube and the Slothouber–Graatsma puzzle, two other dissections of a 3 × 3 × 3 cube into polycubes which use seven and nine pieces respectively. However, writes that the diabolical cube appears to be the oldest puzzle of this type, first appearing in an 1893 book ''Puzzles Old and New'' by Professor Hoffmann Professor Hoffmann (1839–1919) was the pseudonym of Angelo John Lewis, an English-born barrister and writer who has been described as "the most prolific and influential magic author and transla ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Salvador Dalí
Salvador Domingo Felipe Jacinto Dalí i Domènech, Marquess of Dalí of Púbol (; ; ; 11 May 190423 January 1989) was a Spanish Surrealism, surrealist artist renowned for his technical skill, precise draftsmanship, and the striking and bizarre images in his work. Born in Figueres, Catalonia, Spain, Dalí received his formal education in fine arts in Madrid. Influenced by Impressionism and the Renaissance art, Renaissance masters from a young age he became increasingly attracted to Cubism and avant-garde movements. He moved closer to Surrealism in the late 1920s and joined the Surrealist group in 1929, soon becoming one of its leading exponents. His best-known work, ''The Persistence of Memory'', was completed in August 1931, and is one of the most famous Surrealist paintings. Dalí lived in France throughout the Spanish Civil War (1936 to 1939) before leaving for the United States in 1940 where he achieved commercial success. He returned to Spain in 1948 where he announced his ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hexomino
A hexomino (or 6-omino) is a polyomino of order 6, that is, a polygon in the plane made of 6 equal-sized squares connected edge-to-edge. The name of this type of figure is formed with the prefix hex(a)-. When rotations and reflections are not considered to be distinct shapes, there are 35 different ''free'' hexominoes. When reflections are considered distinct, there are 60 ''one-sided'' hexominoes. When rotations are also considered distinct, there are 216 ''fixed'' hexominoes. Symmetry The figure above shows all 35 possible free hexominoes, coloured according to their symmetry groups: * The twenty grey hexominoes have no symmetry. Their symmetry group consists only of the identity mapping. * The six red hexominoes have an axis of mirror symmetry parallel to the gridlines. Their symmetry group has two elements, the identity and a reflection in a line parallel to the sides of the squares. * The two green hexominoes have an axis of mirror symmetry at 45° to the gridlin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Latin Cross
A Latin cross or ''crux immissa'' is a type of cross in which the vertical beam sticks above the crossbeam, with the three upper arms either equally long or with the vertical topmost arm shorter than the two horizontal arms, and always with a much longer bottom arm. If displayed upside down it is called St. Peter's Cross, because he was reputedly executed on this type of cross.Joyce Mori, ''Crosses of Many Cultures'' (Harrisburg, PA: Morehouse Publishing, 1998), p. 32 When displayed sideways it is called St. Philip's cross for the same reason. History In a broad sense, the Latin cross is used to represent all of Christianity and Christendom, given that it teaches that Jesus sacrificed himself for humanity upon it, atoning for the sins of the world. It is especially used among the denominations of Western Christianity, including the Roman Catholic tradition and several Protestant traditions, such as Lutheranism, Moravianism, Anglicanism, Methodism, and Reformed Christianity, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Two-barred Cross
A two-barred cross is similar to a Latin cross but with an extra bar added. The lengths and placement of the bars (or "arms") vary, and most of the variations are interchangeably called the cross of Lorraine, the patriarchal cross, the Orthodox cross or the archiepiscopal cross. The two bars The two bars can be placed tight together (condensed) or far apart. They can be symmetrically spaced either around the middle, or above or below the middle. One asymmetrical variation has one bar near the top and the other just below the middle. Finally the bars can be of equal length, or with one shorter than the other. Decorations The ends of the arms can be decorated according to different styles. A style with round or rounded ends is called treflée or botonée (from French bouton) in heraldic use. The same style is called budded, apostles' or cathedral cross in religious use. A straight and pointy style called pattée also includes maltese cross variations, and finally a pointed style ca ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Robert A
The name Robert is an ancient Germanic given name, from Proto-Germanic "fame" and "bright" (''Hrōþiberhtaz''). Compare Old Dutch ''Robrecht'' and Old High German ''Hrodebert'' (a compound of '' Hruod'' ( non, Hróðr) "fame, glory, honour, praise, renown" and ''berht'' "bright, light, shining"). It is the second most frequently used given name of ancient Germanic origin. It is also in use as a surname. Another commonly used form of the name is Rupert. After becoming widely used in Continental Europe it entered England in its Old French form ''Robert'', where an Old English cognate form (''Hrēodbēorht'', ''Hrodberht'', ''Hrēodbēorð'', ''Hrœdbœrð'', ''Hrœdberð'', ''Hrōðberχtŕ'') had existed before the Norman Conquest. The feminine version is Roberta. The Italian, Portuguese, and Spanish form is Roberto. Robert is also a common name in many Germanic languages, including English, German, Dutch, Norwegian, Swedish, Scots, Danish, and Icelandic. It can be use ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Crucifixion (Corpus Hypercubus)
''Crucifixion (Corpus Hypercubus)'' is a 1954 oil-on-canvas painting by Salvador Dalí. A nontraditional, surrealist portrayal of the Crucifixion, it depicts Christ on a polyhedron net of a tesseract (hypercube). It is one of his best-known paintings from the later period of his career. Background During the 1940s and 1950s Dalí's interest in traditional surrealism diminished and he became fascinated with nuclear science, feeling that "thenceforth, the atom was isfavorite food for thought". The atomic bombing at the end of World War II left a lasting impression; his 1951 essay "Mystical Manifesto" introduced an art theory he called "nuclear mysticism" that combined his interests in Catholicism, mathematics, science, and Catalan culture in an effort to reestablish classical values and techniques, which he extensively utilized in ''Corpus Hypercubus''. That same year, to promote nuclear mysticism and explain the "return to spiritual classicism movement" in modern art, he tra ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Nature (journal)
''Nature'' is a British weekly scientific journal founded and based in London, England. As a multidisciplinary publication, ''Nature'' features peer-reviewed research from a variety of academic disciplines, mainly in science and technology. It has core editorial offices across the United States, continental Europe, and Asia under the international scientific publishing company Springer Nature. ''Nature'' was one of the world's most cited scientific journals by the Science Edition of the 2019 ''Journal Citation Reports'' (with an ascribed impact factor of 42.778), making it one of the world's most-read and most prestigious academic journals. , it claimed an online readership of about three million unique readers per month. Founded in autumn 1869, ''Nature'' was first circulated by Norman Lockyer and Alexander Macmillan as a public forum for scientific innovations. The mid-20th century facilitated an editorial expansion for the journal; ''Nature'' redoubled its efforts in exp ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |

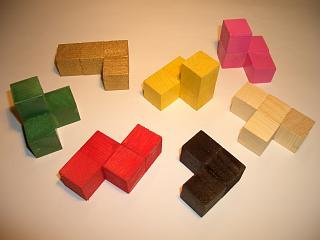

.jpg)
