|
Oper (mathematics)
In mathematics, an Oper is a principal connection, or in more elementary terms a type of differential operator. They were first defined and used by Vladimir Drinfeld and Vladimir Sokolov to study how the KdV equation and related integrable PDEs correspond to algebraic structures known as Kac–Moody algebras. Their modern formulation is due to Drinfeld and Alexander Beilinson. History Opers were first defined, although not named, in a 1981 Russian paper by Drinfeld and Sokolov on ''Equations of Korteweg–de Vries type, and simple Lie algebras''. They were later generalized by Drinfeld and Beilinson in 1993, later published as an e-print in 2005. Formulation Abstract Let G be a connected reductive group over the complex plane \mathbb, with a distinguished Borel subgroup B = B_G \subset G. Set N = ,B/math>, so that H = B/N is the Cartan group. Denote by \mathfrak < \mathfrak < \mathfrak and the corresponding |
Mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics with the major subdisciplines of number theory, algebra, geometry, and analysis, respectively. There is no general consensus among mathematicians about a common definition for their academic discipline. Most mathematical activity involves the discovery of properties of abstract objects and the use of pure reason to prove them. These objects consist of either abstractions from nature orin modern mathematicsentities that are stipulated to have certain properties, called axioms. A ''proof'' consists of a succession of applications of deductive rules to already established results. These results include previously proved theorems, axioms, andin case of abstraction from naturesome basic properties that are considered true starting points of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
One-form
In differential geometry, a one-form on a differentiable manifold is a smooth section of the cotangent bundle. Equivalently, a one-form on a manifold M is a smooth mapping of the total space of the tangent bundle of M to \R whose restriction to each fibre is a linear functional on the tangent space. Symbolically, \alpha : TM \rightarrow ,\quad \alpha_x = \alpha, _: T_xM \rightarrow , where \alpha_x is linear. Often one-forms are described locally, particularly in local coordinates. In a local coordinate system, a one-form is a linear combination of the differentials of the coordinates: \alpha_x = f_1(x) \, dx_1 + f_2(x) \, dx_2 + \cdots + f_n(x) \, dx_n , where the f_i are smooth functions. From this perspective, a one-form has a covariant transformation law on passing from one coordinate system to another. Thus a one-form is an order 1 covariant tensor field. Examples The most basic non-trivial differential one-form is the "change in angle" form d\theta. This is defined as ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Algebraic Variety
Algebraic varieties are the central objects of study in algebraic geometry, a sub-field of mathematics. Classically, an algebraic variety is defined as the set of solutions of a system of polynomial equations over the real or complex numbers. Modern definitions generalize this concept in several different ways, while attempting to preserve the geometric intuition behind the original definition. Conventions regarding the definition of an algebraic variety differ slightly. For example, some definitions require an algebraic variety to be irreducible, which means that it is not the union of two smaller sets that are closed in the Zariski topology. Under this definition, non-irreducible algebraic varieties are called algebraic sets. Other conventions do not require irreducibility. The fundamental theorem of algebra establishes a link between algebra and geometry by showing that a monic polynomial (an algebraic object) in one variable with complex number coefficients is determined ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Langlands Dual
In representation theory, a branch of mathematics, the Langlands dual ''L''''G'' of a reductive algebraic group ''G'' (also called the ''L''-group of ''G'') is a group that controls the representation theory of ''G''. If ''G'' is defined over a field ''k'', then ''L''''G'' is an extension of the absolute Galois group of ''k'' by a complex Lie group. There is also a variation called the Weil form of the ''L''-group, where the Galois group is replaced by a Weil group. Here, the letter ''L'' in the name also indicates the connection with the theory of L-functions, particularly the ''automorphic'' L-functions. The Langlands dual was introduced by in a letter to A. Weil. The ''L''-group is used heavily in the Langlands conjectures of Robert Langlands. It is used to make precise statements from ideas that automorphic forms are in a sense functorial in the group ''G'', when ''k'' is a global field. It is not exactly ''G'' with respect to which automorphic forms and representations are ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Gaudin Model
In physics, the Gaudin model, sometimes known as the ''quantum'' Gaudin model, is a model, or a large class of models, in statistical mechanics first described in its simplest case by Michel Gaudin. They are exactly solvable models, and are also examples of quantum spin chains. History The simplest case was first described by Michel Gaudin in 1976, with the associated Lie algebra taken to be \mathfrak_2, the two-dimensional special linear group. Mathematical formulation Let \mathfrak be a semi-simple Lie algebra of finite dimension d. Let N be a positive integer. On the complex plane \mathbb, choose N different points, z_i. Denote by V_\lambda the finite-dimensional irreducible representation of \mathfrak corresponding to the dominant integral element \lambda. Let (\boldsymbol) := (\lambda_1, \cdots, \lambda_N) be a set of dominant integral weights of \mathfrak. Define the tensor product V_:=V_\otimes \cdots \otimes V_. The model is then specified by a set of operators ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Spectrum (functional Analysis)
In mathematics, particularly in functional analysis, the spectrum of a bounded linear operator (or, more generally, an unbounded linear operator) is a generalisation of the set of eigenvalues of a matrix. Specifically, a complex number \lambda is said to be in the spectrum of a bounded linear operator T if T-\lambda I is not invertible, where I is the identity operator. The study of spectra and related properties is known as spectral theory, which has numerous applications, most notably the mathematical formulation of quantum mechanics. The spectrum of an operator on a finite-dimensional vector space is precisely the set of eigenvalues. However an operator on an infinite-dimensional space may have additional elements in its spectrum, and may have no eigenvalues. For example, consider the right shift operator ''R'' on the Hilbert space ℓ2, :(x_1, x_2, \dots) \mapsto (0, x_1, x_2, \dots). This has no eigenvalues, since if ''Rx''=''λx'' then by expanding this expression we see ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Nicolai Reshetikhin
Nicolai Yuryevich Reshetikhin (russian: Николай Юрьевич Решетихин, born October 10, 1958 in Leningrad, Soviet Union) is a mathematical physicist, currently a professor of mathematics at Tsinghua University, China and a professor of mathematical physics at the University of Amsterdam (Korteweg-de Vries Institute for Mathematics). He is also a professor emeritus at the University of California, Berkeley. His research is in the fields of low-dimensional topology, representation theory, and quantum groups. His major contributions are in the theory of quantum integrable systems, in representation theory of quantum groups and in quantum topology. He and Vladimir Turaev constructed invariants of 3-manifolds which are expected to describe quantum Chern-Simons field theory introduced by Edward Witten. He earned his bachelor's degree and master's degree from Leningrad State University in 1982, and his Ph.D. from the Steklov Mathematical Institute in 1984. He gave a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Edward Frenkel
Edward Vladimirovich Frenkel (; born May 2, 1968) is a Russian-American mathematician working in representation theory, algebraic geometry, and mathematical physics. He is a professor of mathematics at University of California, Berkeley, a member of the American Academy of Arts and Sciences, and author of the bestselling book ''Edward Frenkel#Love and Math, Love and Math''. Biography Edward Frenkel was born on May 2, 1968, in Kolomna, Russia, which was then part of the Soviet Union. His father is of Jewish descent and his mother is Russian. As a high school student he studied higher mathematics privately with Evgeny Evgenievich Petrov, although his initial interest was in quantum physics rather than mathematics. He was not admitted to Moscow State University because of discrimination against Jews and enrolled instead in the applied mathematics program at the Gubkin Russian State University of Oil and Gas, Gubkin University of Oil and Gas. While a student there, he attended the sem ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Boris Feigin
Boris Lvovich Feigin (russian: Бори́с Льво́вич Фе́йгин) (born 20 November 1953) is a Russian mathematician. His research has spanned representation theory, mathematical physics, algebraic geometry, Lie groups and Lie algebras, conformal field theory, homological and homotopical algebra. In 1969 Feigin graduated from the Moscow Mathematical School No. 2 (Andrei Zelevinsky was among his classmates). From 1969 until 1974 he was a student in the Faculty of Mechanics and Mathematics at Moscow State University (MSU) under joint supervision of Dmitry Fuchs and Israel Gelfand. His diploma thesis was dedicated to characteristic classes of flags of foliations. Feigin was not accepted to the graduate school of MSU due to increasingly anti-semitic policies at that institution at that time. After working as a computer programmer in industry for some time, he was accepted in 1976 to the graduate school of Yaroslavl State University and defended his thesis "Cohomology ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Gauge Transformation
In physics, a gauge theory is a type of field theory in which the Lagrangian (and hence the dynamics of the system itself) does not change (is invariant) under local transformations according to certain smooth families of operations (Lie groups). The term ''gauge'' refers to any specific mathematical formalism to regulate redundant degrees of freedom in the Lagrangian of a physical system. The transformations between possible gauges, called ''gauge transformations'', form a Lie group—referred to as the ''symmetry group'' or the ''gauge group'' of the theory. Associated with any Lie group is the Lie algebra of group generators. For each group generator there necessarily arises a corresponding field (usually a vector field) called the ''gauge field''. Gauge fields are included in the Lagrangian to ensure its invariance under the local group transformations (called ''gauge invariance''). When such a theory is quantized, the quanta of the gauge fields are called ''gauge bosons' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lie Group
In mathematics, a Lie group (pronounced ) is a group that is also a differentiable manifold. A manifold is a space that locally resembles Euclidean space, whereas groups define the abstract concept of a binary operation along with the additional properties it must have to be thought of as a "transformation" in the abstract sense, for instance multiplication and the taking of inverses (division), or equivalently, the concept of addition and the taking of inverses (subtraction). Combining these two ideas, one obtains a continuous group where multiplying points and their inverses are continuous. If the multiplication and taking of inverses are smooth (differentiable) as well, one obtains a Lie group. Lie groups provide a natural model for the concept of continuous symmetry, a celebrated example of which is the rotational symmetry in three dimensions (given by the special orthogonal group \text(3)). Lie groups are widely used in many parts of modern mathematics and physics. Lie ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
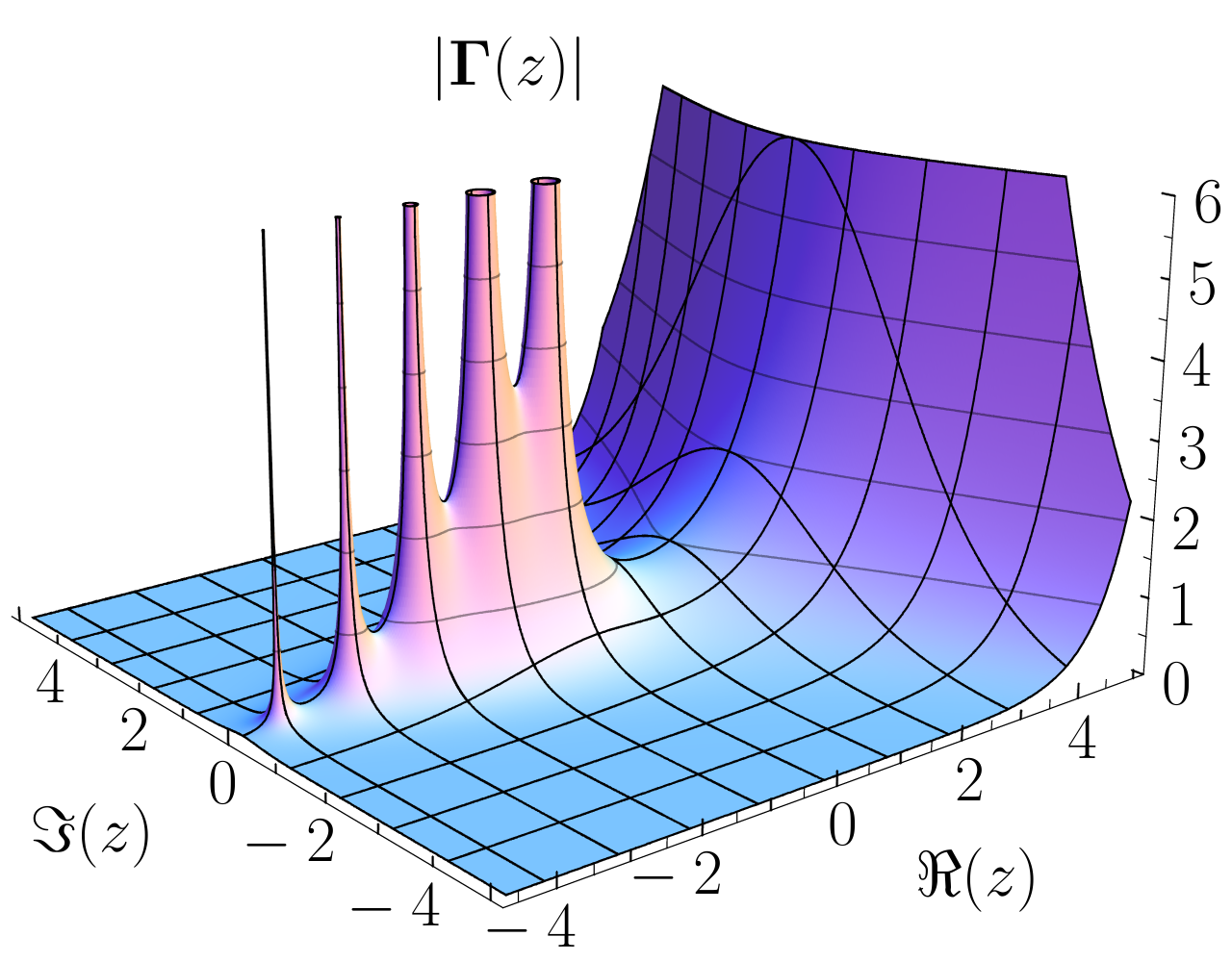
Meromorphic
In the mathematical field of complex analysis, a meromorphic function on an open subset ''D'' of the complex plane is a function that is holomorphic on all of ''D'' ''except'' for a set of isolated points, which are pole (complex analysis), poles of the function. The term comes from the Greek ''meros'' ( μέρος), meaning "part". Every meromorphic function on ''D'' can be expressed as the ratio between two holomorphic functions (with the denominator not constant 0) defined on ''D'': any pole must coincide with a zero of the denominator. Heuristic description Intuitively, a meromorphic function is a ratio of two well-behaved (holomorphic) functions. Such a function will still be well-behaved, except possibly at the points where the denominator of the fraction is zero. If the denominator has a zero at ''z'' and the numerator does not, then the value of the function will approach infinity; if both parts have a zero at ''z'', then one must compare the multiplicity of these zeros ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |