|
Kinematic Overflow
Kinematics is a subfield of physics, developed in classical mechanics, that describes the motion of points, bodies (objects), and systems of bodies (groups of objects) without considering the forces that cause them to move. Kinematics, as a field of study, is often referred to as the "geometry of motion" and is occasionally seen as a branch of mathematics. A kinematics problem begins by describing the geometry of the system and declaring the initial conditions of any known values of position, velocity and/or acceleration of points within the system. Then, using arguments from geometry, the position, velocity and acceleration of any unknown parts of the system can be determined. The study of how forces act on bodies falls within kinetics, not kinematics. For further details, see analytical dynamics. Kinematics is used in astrophysics to describe the motion of celestial bodies and collections of such bodies. In mechanical engineering, robotics, and biomechanics kinematics is used ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Physics
Physics is the natural science that studies matter, its fundamental constituents, its motion and behavior through space and time, and the related entities of energy and force. "Physical science is that department of knowledge which relates to the order of nature, or, in other words, to the regular succession of events." Physics is one of the most fundamental scientific disciplines, with its main goal being to understand how the universe behaves. "Physics is one of the most fundamental of the sciences. Scientists of all disciplines use the ideas of physics, including chemists who study the structure of molecules, paleontologists who try to reconstruct how dinosaurs walked, and climatologists who study how human activities affect the atmosphere and oceans. Physics is also the foundation of all engineering and technology. No engineer could design a flat-screen TV, an interplanetary spacecraft, or even a better mousetrap without first understanding the basic laws of physic ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Human Skeleton
The human skeleton is the internal framework of the human body. It is composed of around 270 bones at birth – this total decreases to around 206 bones by adulthood after some bones get fused together. The bone mass in the skeleton makes up about 14% of the total body weight (ca. 10–11 kg for an average person) and reaches maximum density around age 21. The human skeleton can be divided into the axial skeleton and the appendicular skeleton. The axial skeleton is formed by the vertebral column, the rib cage, the skull and other associated bones. The appendicular skeleton, which is attached to the axial skeleton, is formed by the shoulder girdle, the pelvic girdle and the bones of the upper and lower limbs. The human skeleton performs six major functions: support, movement, protection, production of blood cells, storage of minerals, and endocrine regulation. The human skeleton is not as sexually dimorphic as that of many other primate species, but subtle differences bet ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kinematics
Kinematics is a subfield of physics, developed in classical mechanics, that describes the Motion (physics), motion of points, Physical object, bodies (objects), and systems of bodies (groups of objects) without considering the forces that cause them to move. Kinematics, as a field of study, is often referred to as the "geometry of motion" and is occasionally seen as a branch of mathematics. A kinematics problem begins by describing the geometry of the system and declaring the initial conditions of any known values of position, velocity and/or acceleration of points within the system. Then, using arguments from geometry, the position, velocity and acceleration of any unknown parts of the system can be determined. The study of how forces act on bodies falls within kinetics (physics), kinetics, not kinematics. For further details, see analytical dynamics. Kinematics is used in astrophysics to describe the motion of celestial bodies and collections of such bodies. In mechanical engin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ancient Greek Language
Ancient Greek includes the forms of the Greek language used in ancient Greece and the classical antiquity, ancient world from around 1500 BC to 300 BC. It is often roughly divided into the following periods: Mycenaean Greek (), Greek Dark Ages, Dark Ages (), the Archaic Greece, Archaic period (), and the Classical Greece, Classical period (). Ancient Greek was the language of Homer and of fifth-century Athens, fifth-century Athenian historians, playwrights, and Ancient Greek philosophy, philosophers. It has contributed many words to English vocabulary and has been a standard subject of study in educational institutions of the Western world since the Renaissance. This article primarily contains information about the Homeric Greek, Epic and Classical periods of the language. From the Hellenistic period (), Ancient Greek was followed by Koine Greek, which is regarded as a separate historical stage, although its earliest form closely resembles Attic Greek and its latest form a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
André-Marie Ampère
André-Marie Ampère (, ; ; 20 January 177510 June 1836) was a French physicist and mathematician who was one of the founders of the science of classical electromagnetism, which he referred to as "electrodynamics". He is also the inventor of numerous applications, such as the solenoid (a term coined by him) and the electrical telegraph. As an autodidact, Ampère was a member of the French Academy of Sciences and professor at the École polytechnique and the Collège de France. The SI unit of measurement of electric current, the ampere, is named after him. His name is also one of the 72 names inscribed on the Eiffel Tower. Early life André-Marie Ampère was born on 20 January 1775 to Jean-Jacques Ampère, a prosperous businessman, and Jeanne Antoinette Desutières-Sarcey Ampère, during the height of the French Enlightenment. He spent his childhood and adolescence at the family property at Poleymieux-au-Mont-d'Or near Lyon. Jean-Jacques Ampère, a successful merchant, was an ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mechanical Advantage
Mechanical advantage is a measure of the force amplification achieved by using a tool, mechanical device or machine system. The device trades off input forces against movement to obtain a desired amplification in the output force. The model for this is the ''law of the lever.'' Machine components designed to manage forces and movement in this way are called mechanisms. An ideal mechanism transmits power without adding to or subtracting from it. This means the ideal machine does not include a power source, is frictionless, and is constructed from rigid bodies that do not deflect or wear. The performance of a real system relative to this ideal is expressed in terms of efficiency factors that take into account departures from the ideal. Lever The lever is a movable bar that pivots on a fulcrum attached to or positioned on or across a fixed point. The lever operates by applying forces at different distances from the fulcrum, or pivot. The location of the fulcrum determines ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Algebraic Geometry
Algebraic geometry is a branch of mathematics, classically studying zeros of multivariate polynomials. Modern algebraic geometry is based on the use of abstract algebraic techniques, mainly from commutative algebra, for solving geometrical problems about these sets of zeros. The fundamental objects of study in algebraic geometry are algebraic varieties, which are geometric manifestations of solutions of systems of polynomial equations. Examples of the most studied classes of algebraic varieties are: plane algebraic curves, which include lines, circles, parabolas, ellipses, hyperbolas, cubic curves like elliptic curves, and quartic curves like lemniscates and Cassini ovals. A point of the plane belongs to an algebraic curve if its coordinates satisfy a given polynomial equation. Basic questions involve the study of the points of special interest like the singular points, the inflection points and the points at infinity. More advanced questions involve the topology of the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Four-bar Linkage
In the study of mechanisms, a four-bar linkage, also called a four-bar, is the simplest closed- chain movable linkage. It consists of four bodies, called ''bars'' or ''links'', connected in a loop by four joints. Generally, the joints are configured so the links move in parallel planes, and the assembly is called a ''planar four-bar linkage''. Spherical and spatial four-bar linkages also exist and are used in practice. Planar four-bar linkage Planar four-bar linkages are constructed from four links connected in a loop by four one- degree-of-freedom joints. A joint may be either a revolute joint – also known as a pin joint or hinged joint – denoted by R, or a prismatic joint – also known as a sliding pair – denoted by P. A link that are fixed in place relative to the viewer is called a ''ground link.'' A link connecting to the ground by a revolute joint that can perform a complete revolution is called a '' crank link.'' A link connecting to the ground by a revolute join ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
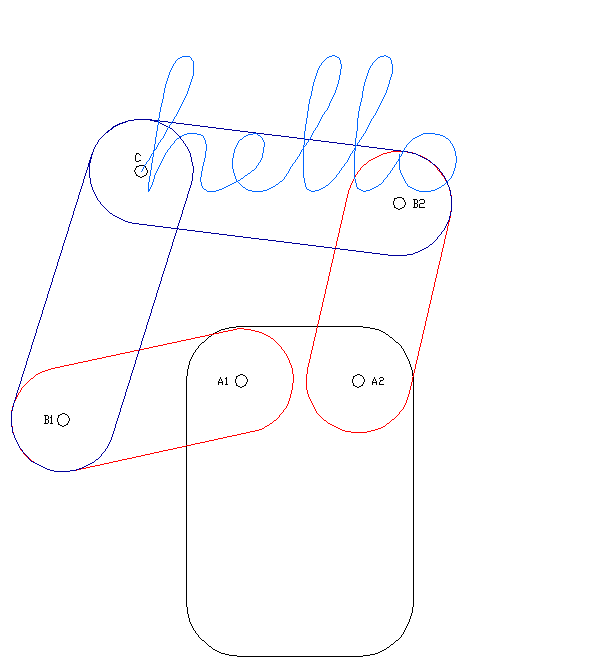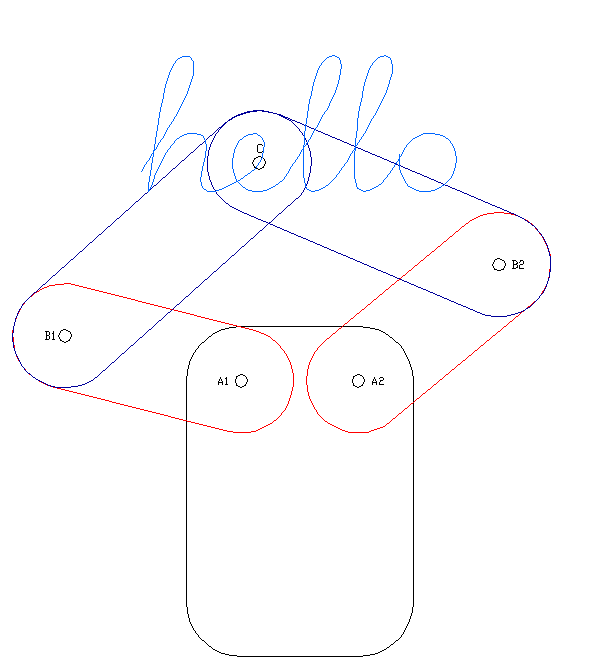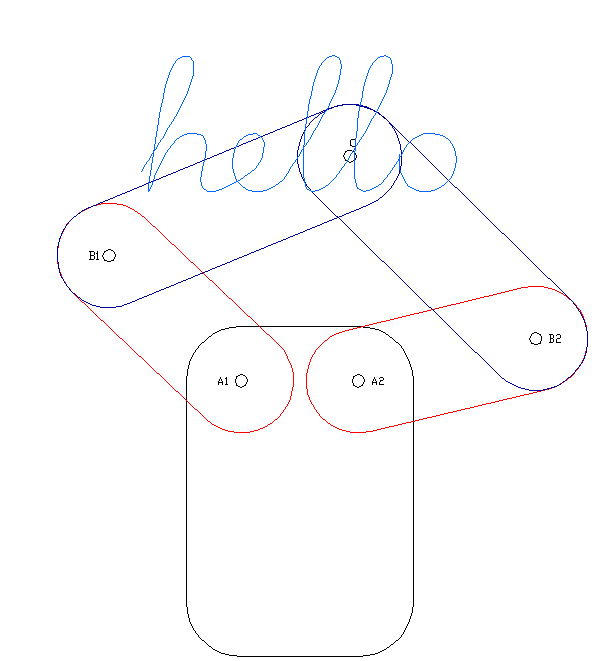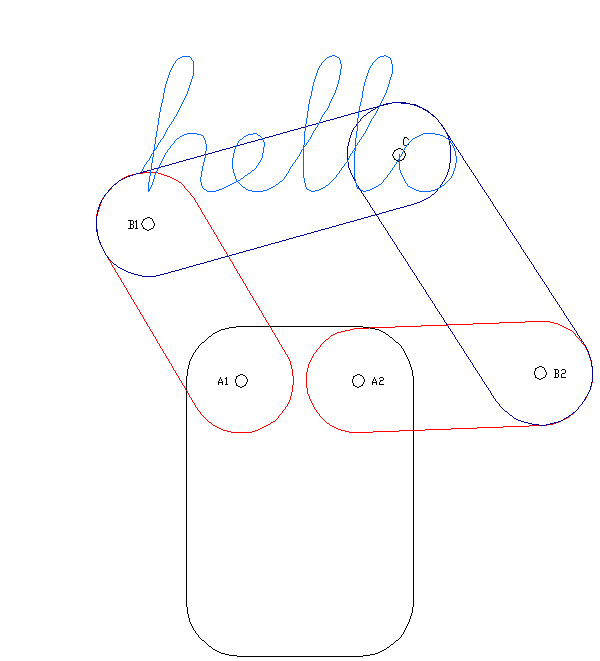
Burmester Theory
In kinematics, Burmester theory comprises geometric techniques for synthesis of linkages. It was introduced in the late 19th century by Ludwig Burmester (1840–1927). His approach was to compute the geometric constraints of the linkage directly from the inventor's desired movement for a floating link. From this point of view a four-bar linkage is a floating link that has two points constrained to lie on two circles. Burmester began with a set of locations, often called ''poses'', for the floating link, which are viewed as snapshots of the constrained movement of this floating link in the device that is to be designed. The design of a crank for the linkage now becomes finding a point in the moving floating link that when viewed in each of these specified positions has a trajectory that lies on a circle. The dimension of the crank is the distance from the point in the floating link, called the circling point, to the center of the circle it travels on, called the center point. Two ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mechanism (engineering)
In engineering, a mechanism is a Machine, device that transforms input forces and movement into a desired set of output forces and movement. Mechanisms generally consist of moving components which may include: * Gears and gear trains; * belt drive, Belts and chain drives; * Cams and cam follower, followers; * Linkage (mechanical), Linkages; * Friction devices, such as brakes or clutches; * Structural components such as a frame, fasteners, bearings, springs, or lubricants; * Various machine elements, such as splines, pins, or keys. The German scientist Franz Reuleaux defines ''machine'' as "a combination of resistant bodies so arranged that by their means the mechanical forces of nature can be compelled to do work accompanied by certain determinate motion". In this context, his use of ''machine'' is generally interpreted to mean ''mechanism''. The combination of force and movement defines Power (physics), power, and a mechanism manages power to achieve a desired set of forces and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Physical Quantity
A physical quantity is a physical property of a material or system that can be quantified by measurement. A physical quantity can be expressed as a ''value'', which is the algebraic multiplication of a ' Numerical value ' and a ' Unit '. For example, the physical quantity of mass can be quantified as '32.3 kg ', where '32.3' is the numerical value and 'kg' is the Unit. A physical quantity possesses at least two characteristics in common. # Numerical magnitude. # Units Symbols and nomenclature International recommendations for the use of symbols for quantities are set out in ISO/IEC 80000, the IUPAP red book and the IUPAC green book. For example, the recommended symbol for the physical quantity ''mass'' is ''m'', and the recommended symbol for the quantity ''electric charge'' is ''Q''. Subscripts and indices Subscripts are used for two reasons, to simply attach a name to the quantity or associate it with another quantity, or index a specific component (e.g., row or colum ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Robot Kinematics
In robotics, robot kinematics applies geometry to the study of the movement of multi-degree of freedom kinematic chains that form the structure of robotic systems. The emphasis on geometry means that the links of the robot are modeled as rigid bodies and its joints are assumed to provide pure rotation or translation. Robot kinematics studies the relationship between the dimensions and connectivity of kinematic chains and the position, velocity and acceleration of each of the links in the robotic system, in order to plan and control movement and to compute actuator forces and torques. The relationship between mass and inertia properties, motion, and the associated forces and torques is studied as part of robot dynamics. Kinematic equations A fundamental tool in robot kinematics is the kinematics equations of the kinematic chains that form the robot. These non-linear equations are used to map the joint parameters to the configuration of the robot system. Kinematics equatio ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |