|
Harmonic Series (music)
A harmonic series (also overtone series) is the sequence of harmonics, musical tones, or pure tones whose frequency is an integer multiple of a ''fundamental frequency''. Pitched musical instruments are often based on an acoustic resonator such as a string or a column of air, which oscillates at numerous modes simultaneously. At the frequencies of each vibrating mode, waves travel in both directions along the string or air column, reinforcing and canceling each other to form standing waves. Interaction with the surrounding air causes audible sound waves, which travel away from the instrument. Because of the typical spacing of the resonances, these frequencies are mostly limited to integer multiples, or harmonics, of the lowest frequency, and such multiples form the harmonic series. The musical pitch of a note is usually perceived as the lowest partial present (the fundamental frequency), which may be the one created by vibration over the full length of the string or air co ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Harmonic Partials On Strings
A harmonic is a wave with a frequency that is a positive integer multiple of the ''fundamental frequency'', the frequency of the original periodic signal, such as a sinusoidal wave. The original signal is also called the ''1st harmonic'', the other harmonics are known as ''higher harmonics''. As all harmonics are periodic at the fundamental frequency, the sum of harmonics is also periodic at that frequency. The set of harmonics forms a '' harmonic series''. The term is employed in various disciplines, including music, physics, acoustics, electronic power transmission, radio technology, and other fields. For example, if the fundamental frequency is 50 Hz, a common AC power supply frequency, the frequencies of the first three higher harmonics are 100 Hz (2nd harmonic), 150 Hz (3rd harmonic), 200 Hz (4th harmonic) and any addition of waves with these frequencies is periodic at 50 Hz. In music, harmonics are used on string instruments and wind instrum ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pitch (music)
Pitch is a perceptual property of sounds that allows their ordering on a frequency-related scale, or more commonly, pitch is the quality that makes it possible to judge sounds as "higher" and "lower" in the sense associated with musical melodies. Pitch is a major auditory attribute of musical tones, along with duration, loudness, and timbre. Pitch may be quantified as a frequency, but pitch is not a purely objective physical property; it is a subjective psychoacoustical attribute of sound. Historically, the study of pitch and pitch perception has been a central problem in psychoacoustics, and has been instrumental in forming and testing theories of sound representation, processing, and perception in the auditory system. Perception Pitch and frequency Pitch is an auditory sensation in which a listener assigns musical tones to relative positions on a musical scale based primarily on their perception of the frequency of vibration. Pitch is closely related to frequency, but ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Inharmonicity
In music, inharmonicity is the degree to which the frequencies of overtones (also known as partials or partial tones) depart from whole multiples of the fundamental frequency ( harmonic series). Acoustically, a note perceived to have a single distinct pitch in fact contains a variety of additional overtones. Many percussion instruments, such as cymbals, tam-tams, and chimes, create complex and inharmonic sounds. Music harmony and intonation depends strongly on the harmonicity of tones. An ideal, homogeneous, infinitesimally thin or infinitely flexible string or column of air has exactly harmonic modes of vibration. In any real musical instrument, the resonant body that produces the music tone—typically a string, wire, or column of air—deviates from this ideal and has some small or large amount of inharmonicity. For instance, a very thick string behaves less as an ideal string and more like a cylinder (a tube of mass), which has natural resonances that are not whole numb ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
John R
John R. (born John Richbourg, August 20, 1910 - February 15, 1986) was an American radio disc jockey who attained fame in the 1950s and 1960s for playing rhythm and blues music on Nashville radio station WLAC. He was also a notable record producer and artist manager. Richbourg was arguably the most popular and charismatic of the four announcers at WLAC who showcased popular African-American music in nightly programs from the late 1940s to the early 1970s. (The other three were Gene Nobles, Herman Grizzard, and Bill "Hoss" Allen.) Later rock music disc jockeys, such as Alan Freed and Wolfman Jack, mimicked Richbourg's practice of using speech that simulated African-American street language of the mid-twentieth century. Richbourg's highly stylized approach to on-air presentation of both music and advertising earned him popularity, but it also created identity confusion. Because Richbourg and fellow disc jockey Allen used African-American speech patterns, many listeners thought that ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hermann Von Helmholtz
Hermann Ludwig Ferdinand von Helmholtz (31 August 1821 – 8 September 1894) was a German physicist and physician who made significant contributions in several scientific fields, particularly hydrodynamic stability. The Helmholtz Association, the largest German association of research institutions, is named in his honor. In the fields of physiology and psychology, Helmholtz is known for his mathematics concerning the eye, theories of vision, ideas on the visual perception of space, color vision research, the sensation of tone, perceptions of sound, and empiricism in the physiology of perception. In physics, he is known for his theories on the conservation of energy, work in electrodynamics, chemical thermodynamics, and on a mechanical foundation of thermodynamics. As a philosopher, he is known for his philosophy of science, ideas on the relation between the laws of perception and the laws of nature, the science of aesthetics, and ideas on the civilizing power of science. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Alexander John Ellis
Alexander John Ellis, (14 June 1814 – 28 October 1890), was an English mathematician, philologist and early phonetician who also influenced the field of musicology. He changed his name from his father's name, Sharpe, to his mother's maiden name, Ellis, in 1825 as a condition of receiving significant financial support from a relative on his mother's side. He is buried in Kensal Green Cemetery, London. Biography He was born Alexander John Sharpe in Hoxton, Middlesex to a wealthy family. His father, James Birch Sharpe, was a notable artist and physician who was later appointed Esquire of Windlesham. His mother, Ann Ellis, was from a noble background, but it is not known how her family made its fortune. Alexander's brother James Birch Sharpe junior died at the Battle of Inkerman during the Crimean War. His other brother, William Henry Sharpe, served with the Lancashire Fusiliers after moving north with his family to Cumberland, due to military work. Alexander was educated at Sh ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fourier Analysis
In mathematics, Fourier analysis () is the study of the way general functions may be represented or approximated by sums of simpler trigonometric functions. Fourier analysis grew from the study of Fourier series, and is named after Joseph Fourier, who showed that representing a function as a sum of trigonometric functions greatly simplifies the study of heat transfer. The subject of Fourier analysis encompasses a vast spectrum of mathematics. In the sciences and engineering, the process of decomposing a function into oscillatory components is often called Fourier analysis, while the operation of rebuilding the function from these pieces is known as Fourier synthesis. For example, determining what component frequencies are present in a musical note would involve computing the Fourier transform of a sampled musical note. One could then re-synthesize the same sound by including the frequency components as revealed in the Fourier analysis. In mathematics, the term ''Fourier ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
William Forde Thompson
William Forde "Bill" Thompson is an academic who has worked in Canada, Sweden and Australia. He is a distinguished professor at Macquarie University in Sydney, Australia, where he was chair of the psychology department between 2009 and 2013. His research focuses on music, emotion, expertise, and performance. From 2007 to 2009, he was president of the Society for Music Perception and Cognition. He was an associate editor at ''Music Perception'', former editor of '' Empirical Musicology Review'' (2008–2010), and chief investigator of the ARC Centre of Excellence in Cognition and its Disorders. He is a fellow of the Association for Psychological Science. Born in Middletown, Connecticut, US, Thompson holds a BSc in psychology from McGill University (Montreal, Canada) and an MA and PhD in psychology from Queen's University (Kingston, Canada). He is the author of '' Music, Thought, and Feeling: Understanding the Psychology of Music'', Oxford University Press S 2009 (1st edition), ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
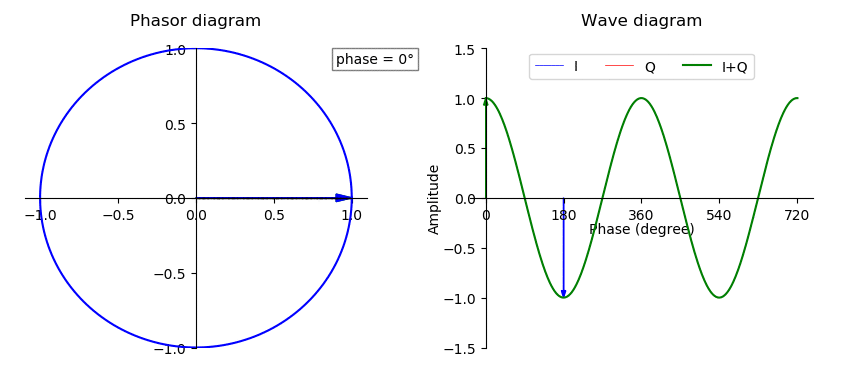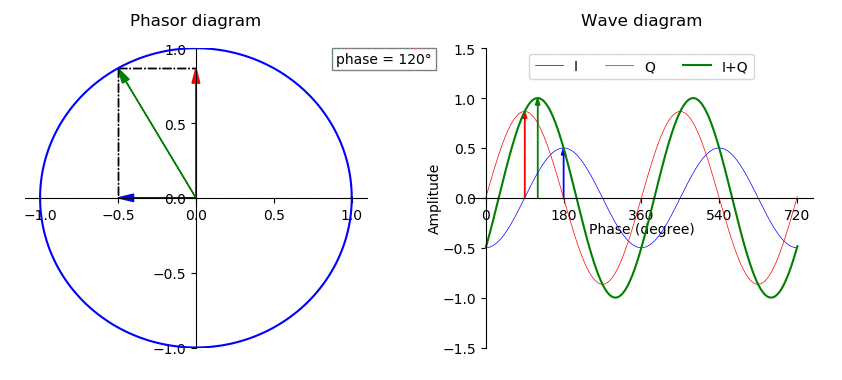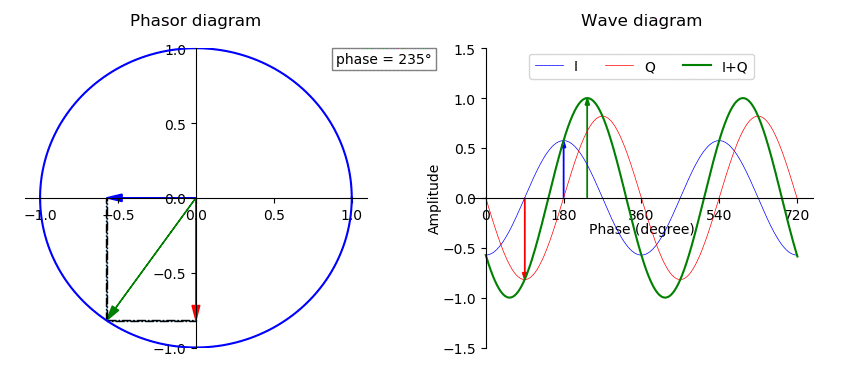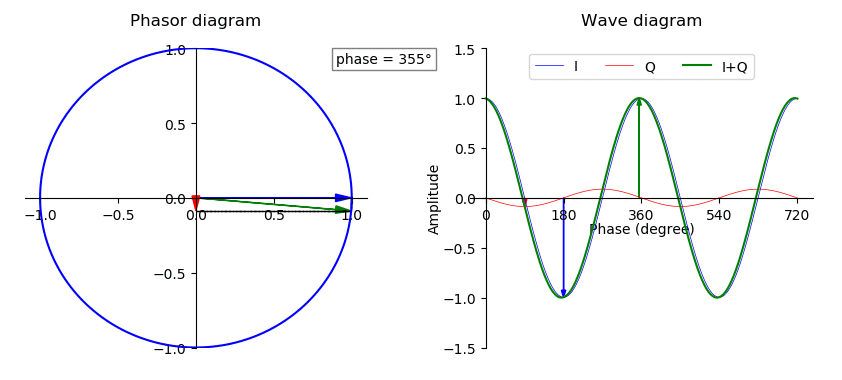
Phase (waves)
In physics and mathematics, the phase of a periodic function F of some real variable t (such as time) is an angle-like quantity representing the fraction of the cycle covered up to t. It is denoted \phi(t) and expressed in such a scale that it varies by one full turn as the variable t goes through each period (and F(t) goes through each complete cycle). It may be measured in any angular unit such as degrees or radians, thus increasing by 360° or 2\pi as the variable t completes a full period. This convention is especially appropriate for a sinusoidal function, since its value at any argument t then can be expressed as \phi(t), the sine of the phase, multiplied by some factor (the amplitude of the sinusoid). (The cosine may be used instead of sine, depending on where one considers each period to start.) Usually, whole turns are ignored when expressing the phase; so that \phi(t) is also a periodic function, with the same period as F, that repeatedly scans the same range of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Amplitude
The amplitude of a periodic variable is a measure of its change in a single period (such as time or spatial period). The amplitude of a non-periodic signal is its magnitude compared with a reference value. There are various definitions of amplitude (see below), which are all functions of the magnitude of the differences between the variable's extreme values. In older texts, the phase of a periodic function is sometimes called the amplitude. Definitions Peak amplitude & semi-amplitude For symmetric periodic waves, like sine waves, square waves or triangle waves ''peak amplitude'' and ''semi amplitude'' are the same. Peak amplitude In audio system measurements, telecommunications and others where the measurand is a signal that swings above and below a reference value but is not sinusoidal, peak amplitude is often used. If the reference is zero, this is the maximum absolute value of the signal; if the reference is a mean value (DC component), the peak amplitude is the maximu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sine Wave
A sine wave, sinusoidal wave, or just sinusoid is a curve, mathematical curve defined in terms of the ''sine'' trigonometric function, of which it is the graph of a function, graph. It is a type of continuous wave and also a Smoothness, smooth periodic function. It occurs often in mathematics, as well as in physics, engineering, signal processing and many other fields. Formulation Its most basic form as a function of time (''t'') is: y(t) = A\sin(2 \pi f t + \varphi) = A\sin(\omega t + \varphi) where: * ''A'', ''amplitude'', the peak deviation of the function from zero. * ''f'', ''frequency, ordinary frequency'', the ''Real number, number'' of oscillations (cycles) that occur each second of time. * ''ω'' = 2''f'', ''angular frequency'', the rate of change of the function argument in units of radians per second. * \varphi, ''phase (waves), phase'', specifies (in radians) where in its cycle the oscillation is at ''t'' = 0. When \varphi is non-zero, the entire waveform appears to ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Wave
In physics, mathematics, and related fields, a wave is a propagating dynamic disturbance (change from equilibrium) of one or more quantities. Waves can be periodic, in which case those quantities oscillate repeatedly about an equilibrium (resting) value at some frequency. When the entire waveform moves in one direction, it is said to be a ''traveling wave''; by contrast, a pair of superimposed periodic waves traveling in opposite directions makes a '' standing wave''. In a standing wave, the amplitude of vibration has nulls at some positions where the wave amplitude appears smaller or even zero. Waves are often described by a ''wave equation'' (standing wave field of two opposite waves) or a one-way wave equation for single wave propagation in a defined direction. Two types of waves are most commonly studied in classical physics. In a ''mechanical wave'', stress and strain fields oscillate about a mechanical equilibrium. A mechanical wave is a local deformation (strain) in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
.png "Click for more on -> Fourier Analysis")
