|
Flexural Strength
Flexural strength, also known as modulus of rupture, or bend strength, or transverse rupture strength is a material property, defined as the stress in a material just before it yields in a flexure test. The transverse bending test is most frequently employed, in which a specimen having either a circular or rectangular cross-section is bent until fracture or yielding using a three-point flexural test technique. The flexural strength represents the highest stress experienced within the material at its moment of yield. It is measured in terms of stress, here given the symbol \sigma. Introduction When an object is formed of a single material, like a wooden beam or a steel rod, is bent (Fig. 1), it experiences a range of stresses across its depth (Fig. 2). At the edge of the object on the inside of the bend (concave face) the stress will be at its maximum compressive stress value. At the outside of the bend (convex face) the stress will be at its maximum tensile value. These in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Flexural Strength
Flexural strength, also known as modulus of rupture, or bend strength, or transverse rupture strength is a material property, defined as the stress in a material just before it yields in a flexure test. The transverse bending test is most frequently employed, in which a specimen having either a circular or rectangular cross-section is bent until fracture or yielding using a three-point flexural test technique. The flexural strength represents the highest stress experienced within the material at its moment of yield. It is measured in terms of stress, here given the symbol \sigma. Introduction When an object is formed of a single material, like a wooden beam or a steel rod, is bent (Fig. 1), it experiences a range of stresses across its depth (Fig. 2). At the edge of the object on the inside of the bend (concave face) the stress will be at its maximum compressive stress value. At the outside of the bend (convex face) the stress will be at its maximum tensile value. These in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Stress (mechanics)
In continuum mechanics, stress is a physical quantity. It is a quantity that describes the magnitude of forces that cause deformation. Stress is defined as ''force per unit area''. When an object is pulled apart by a force it will cause elongation which is also known as deformation, like the stretching of an elastic band, it is called tensile stress. But, when the forces result in the compression of an object, it is called compressive stress. It results when forces like tension or compression act on a body. The greater this force and the smaller the cross-sectional area of the body on which it acts, the greater the stress. Therefore, stress is measured in newton per square meter (N/m2) or pascal (Pa). Stress expresses the internal forces that neighbouring particles of a continuous material exert on each other, while strain is the measure of the deformation of the material. For example, when a solid vertical bar is supporting an overhead weight, each particle in the bar pushe ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Yield (engineering)
In materials science and engineering, the yield point is the point on a stress-strain curve that indicates the limit of elastic behavior and the beginning of plastic behavior. Below the yield point, a material will deform elastically and will return to its original shape when the applied stress is removed. Once the yield point is passed, some fraction of the deformation will be permanent and non-reversible and is known as plastic deformation. The yield strength or yield stress is a material property and is the stress corresponding to the yield point at which the material begins to deform plastically. The yield strength is often used to determine the maximum allowable load in a mechanical component, since it represents the upper limit to forces that can be applied without producing permanent deformation. In some materials, such as aluminium, there is a gradual onset of non-linear behavior, making the precise yield point difficult to determine. In such a case, the offset yiel ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
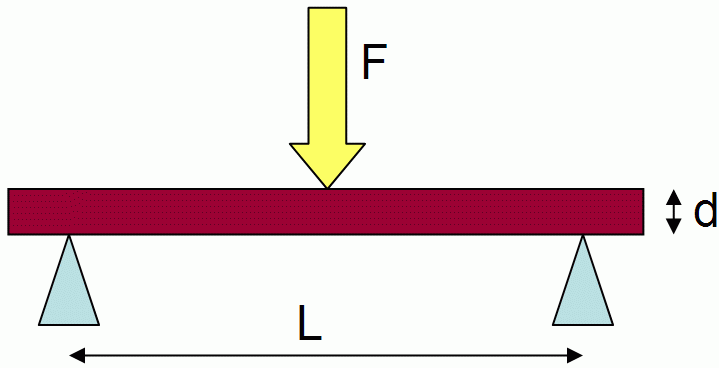
Three-point Flexural Test
The three-point bending flexural test provides values for the modulus of elasticity in bending E_f, flexural stress \sigma_f, flexural strain \epsilon_f and the flexural stress–strain response of the material. This test is performed on a universal testing machine (tensile testing machine or tensile tester) with a three-point or four-point bend fixture. The main advantage of a three-point flexural test is the ease of the specimen preparation and testing. However, this method has also some disadvantages: the results of the testing method are sensitive to specimen and loading geometry and strain rate. Testing method The test method for conducting the test usually involves a specified test fixture on a universal testing machine. Details of the test preparation, conditioning, and conduct affect the test results. The sample is placed on two supporting pins a set distance apart. Calculation of the flexural stress \sigma_f :\sigma_f = \frac for a rectangular cross section :\si ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tensile Strength
Ultimate tensile strength (UTS), often shortened to tensile strength (TS), ultimate strength, or F_\text within equations, is the maximum stress that a material can withstand while being stretched or pulled before breaking. In brittle materials the ultimate tensile strength is close to the yield point, whereas in ductile materials the ultimate tensile strength can be higher. The ultimate tensile strength is usually found by performing a tensile test and recording the engineering stress versus strain. The highest point of the stress–strain curve is the ultimate tensile strength and has units of stress. The equivalent point for the case of compression, instead of tension, is called the compressive strength. Tensile strengths are rarely of any consequence in the design of ductile members, but they are important with brittle members. They are tabulated for common materials such as alloys, composite materials, ceramics, plastics, and wood. Definition The ultimate tensile streng ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Homogeneous
Homogeneity and heterogeneity are concepts often used in the sciences and statistics relating to the uniformity of a substance or organism. A material or image that is homogeneous is uniform in composition or character (i.e. color, shape, size, weight, height, distribution, texture, language, income, disease, temperature, radioactivity, architectural design, etc.); one that is heterogeneous is distinctly nonuniform in at least one of these qualities. Heterogeneous Mixtures, in chemistry, is where certain elements are unwillingly combined and, when given the option, will separate. Etymology and spelling The words ''homogeneous'' and ''heterogeneous'' come from Medieval Latin ''homogeneus'' and ''heterogeneus'', from Ancient Greek ὁμογενής (''homogenēs'') and ἑτερογενής (''heterogenēs''), from ὁμός (''homos'', “same”) and ἕτερος (''heteros'', “other, another, different”) respectively, followed by γένος (''genos'', “kind”); - ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Beam 3pt
Beam may refer to: Streams of particles or energy *Light beam, or beam of light, a directional projection of light energy **Laser beam *Particle beam, a stream of charged or neutral particles **Charged particle beam, a spatially localized group of electrically charged particles ***Cathode ray, or electron beam or e-beam, streams of electrons observed in discharge tubes ***X-ray beam, a penetrating form of high-energy electromagnetic radiation **Molecular beam, a beam of particles moving at approximately equal velocities Arts, entertainment and media * Beam (music), a connection line in musical notation * Beam, to transport matter using the Transporter in the ''Star Trek'' fictional universe * Beam (rapper), American hip hop artist * BEAM.TV, an online digital delivery and content management platform * BEAM Channel 31, a Philippines television network * Beam (website), later Mixer, a former video game live streaming platform * BeamNG.drive, an open-world vehicle simulation vid ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Beam 4pt
Beam may refer to: Streams of particles or energy *Light beam, or beam of light, a directional projection of light energy **Laser beam *Particle beam, a stream of charged or neutral particles **Charged particle beam, a spatially localized group of electrically charged particles ***Cathode ray, or electron beam or e-beam, streams of electrons observed in discharge tubes ***X-ray beam, a penetrating form of high-energy electromagnetic radiation **Molecular beam, a beam of particles moving at approximately equal velocities Arts, entertainment and media * Beam (music), a connection line in musical notation * Beam, to transport matter using the Transporter in the ''Star Trek'' fictional universe * Beam (rapper), American hip hop artist * BEAM.TV, an online digital delivery and content management platform * BEAM Channel 31, a Philippines television network * Beam (website), later Mixer, a former video game live streaming platform * BeamNG.drive, an open-world vehicle simulation vid ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Flexural Modulus
In mechanics, the flexural modulus or bending modulus is an intensive property that is computed as the ratio of stress to strain in flexural deformation, or the tendency for a material to resist bending. It is determined from the slope of a stress-strain curve produced by a flexural test (such as the ASTM D790), and uses units of force per area. The flexural modulus defined using the 2-point (cantilever) and 3-point bend tests assumes a linear stress strain response. For a 3-point test of a rectangular beam behaving as an isotropic linear material, where ''w'' and ''h'' are the width and height of the beam, ''I'' is the second moment of area of the beam's cross-section, ''L'' is the distance between the two outer supports, and ''d'' is the deflection due to the load ''F'' applied at the middle of the beam, the flexural modulus: : E_ = \frac From elastic beam theory :d = \frac and for rectangular beam : I = \fracwh^3 thus E_ = E (Elastic modulus) For very small strains ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Three-point Flexural Test
The three-point bending flexural test provides values for the modulus of elasticity in bending E_f, flexural stress \sigma_f, flexural strain \epsilon_f and the flexural stress–strain response of the material. This test is performed on a universal testing machine (tensile testing machine or tensile tester) with a three-point or four-point bend fixture. The main advantage of a three-point flexural test is the ease of the specimen preparation and testing. However, this method has also some disadvantages: the results of the testing method are sensitive to specimen and loading geometry and strain rate. Testing method The test method for conducting the test usually involves a specified test fixture on a universal testing machine. Details of the test preparation, conditioning, and conduct affect the test results. The sample is placed on two supporting pins a set distance apart. Calculation of the flexural stress \sigma_f :\sigma_f = \frac for a rectangular cross section :\si ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Four-point Flexural Test
The four-point flexural test provides values for the modulus of elasticity in bending E_f, flexural stress \sigma_f, flexural strain \varepsilon_f and the flexural stress-strain response of the material. This test is very similar to the three-point bending flexural test. The major difference being that with the addition of a fourth bearing the portion of the beam between the two loading points is put under maximum stress, as opposed to only the material right under the central bearing in the case of three-point bending. This difference is of prime importance when studying brittle materials, where the number and severity of flaws exposed to the maximum stress is directly related to the flexural strength and crack initiation. Compared to the three-point bending flexural test, there are no shear forces in the four-point bending flexural test in the area between the two loading pins. The four-point bending test is therefore particularly suitable for brittle materials that cannot withs ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |