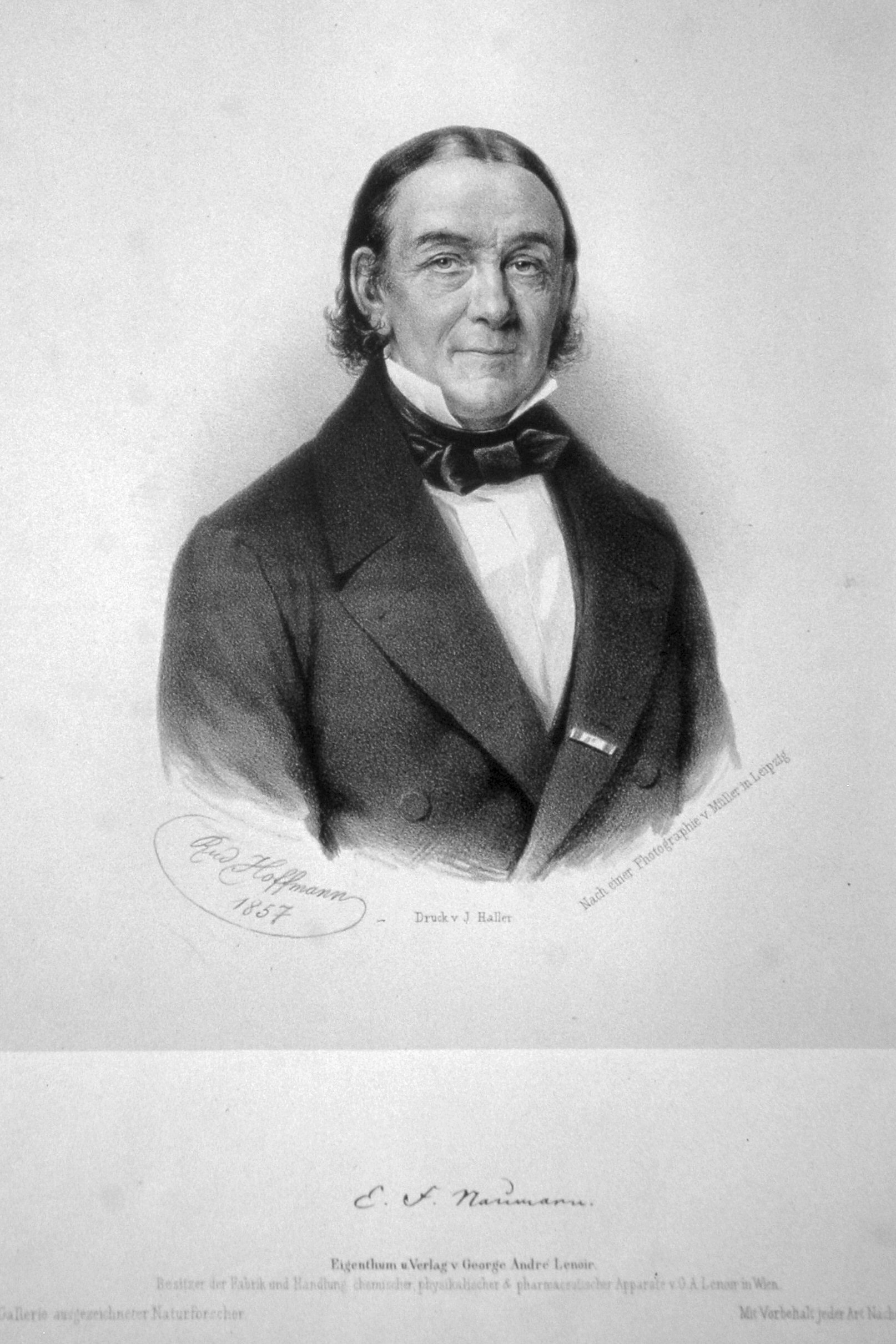|
Conchospiral
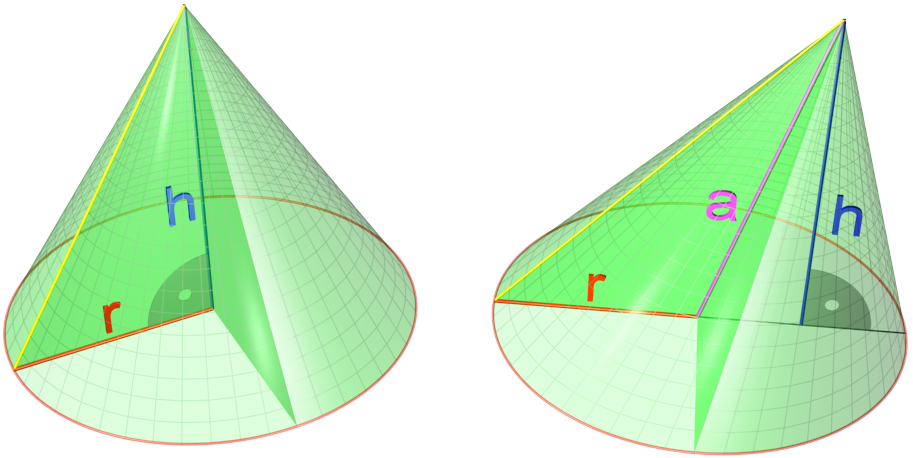
In mathematics, a conchospiral a specific type of space spiral on the surface of a cone (a '' conical spiral''), whose floor projection is a logarithmic spiral. Conchospirals are used in biology for modelling snail shells, and flight paths of insects and in electrical engineering for the construction of antennas. Parameterization In cylindrical coordinates, the conchospiral is described by the parametric equations: :r=\mu^t a :\theta=t :z=\mu^t c. The projection of a conchospiral on the (r,\theta) plane is a logarithmic spiral. The parameter \mu controls the opening angle of the projected spiral, while the parameter c controls the slope of the cone on which the curve lies. History The name "conchospiral" was given to these curves by 19th-century German mineralogist Georg Amadeus Carl Friedrich Naumann, in his study of the shapes of sea shells. Applications The conchospiral has been used in the design for radio antenna In radio engineering, an antenna or aerial is the int ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Conchospiral
In mathematics, a conchospiral a specific type of space spiral on the surface of a cone (a '' conical spiral''), whose floor projection is a logarithmic spiral. Conchospirals are used in biology for modelling snail shells, and flight paths of insects and in electrical engineering for the construction of antennas. Parameterization In cylindrical coordinates, the conchospiral is described by the parametric equations: :r=\mu^t a :\theta=t :z=\mu^t c. The projection of a conchospiral on the (r,\theta) plane is a logarithmic spiral. The parameter \mu controls the opening angle of the projected spiral, while the parameter c controls the slope of the cone on which the curve lies. History The name "conchospiral" was given to these curves by 19th-century German mineralogist Georg Amadeus Carl Friedrich Naumann, in his study of the shapes of sea shells. Applications The conchospiral has been used in the design for radio antenna In radio engineering, an antenna or aerial is the int ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics with the major subdisciplines of number theory, algebra, geometry, and analysis, respectively. There is no general consensus among mathematicians about a common definition for their academic discipline. Most mathematical activity involves the discovery of properties of abstract objects and the use of pure reason to prove them. These objects consist of either abstractions from nature orin modern mathematicsentities that are stipulated to have certain properties, called axioms. A ''proof'' consists of a succession of applications of deductive rules to already established results. These results include previously proved theorems, axioms, andin case of abstraction from naturesome basic properties that are considered true starting points of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Space Spiral
In mathematics, a spiral is a curve which emanates from a point, moving farther away as it revolves around the point. Helices Two major definitions of "spiral" in the American Heritage Dictionary are:Spiral ''American Heritage Dictionary of the English Language'', Houghton Mifflin Company, Fourth Edition, 2009. # a curve on a plane that winds around a fixed center point at a continuously increasing or decreasing distance from the point. # a three-dimensional curve that turns around an axis at a constant or continuously varying distance while moving parallel to the axis; a . The first definition describes a |
Cone
A cone is a three-dimensional geometric shape that tapers smoothly from a flat base (frequently, though not necessarily, circular) to a point called the apex or vertex. A cone is formed by a set of line segments, half-lines, or lines connecting a common point, the apex, to all of the points on a base that is in a plane that does not contain the apex. Depending on the author, the base may be restricted to be a circle, any one-dimensional quadratic form in the plane, any closed one-dimensional figure, or any of the above plus all the enclosed points. If the enclosed points are included in the base, the cone is a solid object; otherwise it is a two-dimensional object in three-dimensional space. In the case of a solid object, the boundary formed by these lines or partial lines is called the ''lateral surface''; if the lateral surface is unbounded, it is a conical surface. In the case of line segments, the cone does not extend beyond the base, while in the case of half-lin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Conical Spiral
In mathematics, a conical spiral, also known as a conical helix, is a space curve on a right circular cone, whose floor plan is a plane spiral. If the floor plan is a logarithmic spiral, it is called ''conchospiral'' (from conch). Parametric representation In the x-y-plane a spiral with parametric representation : x=r(\varphi)\cos\varphi \ ,\qquad y=r(\varphi)\sin\varphi a third coordinate z(\varphi) can be added such that the space curve lies on the cone with equation \;m^2(x^2+y^2)=(z-z_0)^2\ ,\ m>0\; : * x=r(\varphi)\cos\varphi \ ,\qquad y=r(\varphi)\sin\varphi\ , \qquad \color \ . Such curves are called conical spirals. They were known to Pappos. Parameter m is the slope of the cone's lines with respect to the x-y-plane. A conical spiral can instead be seen as the orthogonal projection of the floor plan spiral onto the cone. Examples : 1) Starting with an ''archimedean spiral'' \;r(\varphi)=a\varphi\; gives the conical spiral (see diagram) : x=a\varphi\cos\varph ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Floor Plan
In architecture and building engineering, a floor plan is a technical drawing to scale, showing a view from above, of the relationships between rooms, spaces, traffic patterns, and other physical features at one level of a structure. Dimensions are usually drawn between the walls to specify room sizes and wall lengths. Floor plans may also include details of fixtures like sinks, water heaters, furnaces, etc. Floor plans may include notes for construction to specify finishes, construction methods, or symbols for electrical items. It is also called a ''plan'' which is a measured plane typically projected at the floor height of , as opposed to an ''elevation'' which is a measured plane projected from the side of a building, along its height, or a section or ''cross section'' where a building is cut along an axis to reveal the interior structure. Overview Similar to a map, the orientation of the view is downward from above, but unlike a conventional map, a plan is drawn at a part ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Logarithmic Spiral
A logarithmic spiral, equiangular spiral, or growth spiral is a self-similar spiral curve that often appears in nature. The first to describe a logarithmic spiral was Albrecht Dürer (1525) who called it an "eternal line" ("ewige Linie"). More than a century later, the curve was discussed by Descartes (1638), and later extensively investigated by Jacob Bernoulli, who called it ''Spira mirabilis'', "the marvelous spiral". The logarithmic spiral can be distinguished from the Archimedean spiral by the fact that the distances between the turnings of a logarithmic spiral increase in geometric progression, while in an Archimedean spiral these distances are constant. Definition In polar coordinates (r, \varphi) the logarithmic spiral can be written as r = ae^,\quad \varphi \in \R, or \varphi = \frac \ln \frac, with e being the base of natural logarithms, and a > 0, k\ne 0 being real constants. In Cartesian coordinates The logarithmic spiral with the polar equation r = a e^ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Snail Shell
The gastropod shell is part of the body of a gastropod or snail, a kind of mollusc. The shell is an exoskeleton, which protects from predators, mechanical damage, and dehydration, but also serves for muscle attachment and calcium storage. Some gastropods appear shell-less (slugs) but may have a remnant within the mantle, or in some cases the shell is reduced such that the body cannot be retracted within it (semi-slug). Some snails also possess an operculum that seals the opening of the shell, known as the aperture, which provides further protection. The study of mollusc shells is known as conchology. The biological study of gastropods, and other molluscs in general, is malacology. Shell morphology terms vary by species group. Shell layers The gastropod shell has three major layers secreted by the mantle. The calcareous central layer, tracum, is typically made of calcium carbonate precipitated into an organic matrix known as conchiolin. The outermost layer is the periostra ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Electrical Engineering
Electrical engineering is an engineering discipline concerned with the study, design, and application of equipment, devices, and systems which use electricity, electronics, and electromagnetism. It emerged as an identifiable occupation in the latter half of the 19th century after commercialization of the electric telegraph, the telephone, and electrical power generation, distribution, and use. Electrical engineering is now divided into a wide range of different fields, including computer engineering, systems engineering, power engineering, telecommunications, radio-frequency engineering, signal processing, instrumentation, photovoltaic cells, electronics, and optics and photonics. Many of these disciplines overlap with other engineering branches, spanning a huge number of specializations including hardware engineering, power electronics, electromagnetics and waves, microwave engineering, nanotechnology, electrochemistry, renewable energies, mechatronics/control, and electrical m ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Antenna (radio)
In radio engineering, an antenna or aerial is the interface between radio waves propagating through space and electric currents moving in metal conductors, used with a transmitter or receiver. In transmission, a radio transmitter supplies an electric current to the antenna's terminals, and the antenna radiates the energy from the current as electromagnetic wave In physics, electromagnetic radiation (EMR) consists of waves of the electromagnetic (EM) field, which propagate through space and carry momentum and electromagnetic radiant energy. It includes radio waves, microwaves, infrared, (visib ...s (radio waves). In Receiver (radio), reception, an antenna intercepts some of the power of a radio wave in order to produce an electric current at its terminals, that is applied to a receiver to be Amplifier, amplified. Antennas are essential components of all radio equipment. An antenna is an array of conductor (material), conductors (Driven element, elements), elect ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cylindrical Coordinates
A cylindrical coordinate system is a three-dimensional coordinate system that specifies point positions by the distance from a chosen reference axis ''(axis L in the image opposite)'', the direction from the axis relative to a chosen reference direction ''(axis A)'', and the distance from a chosen reference plane perpendicular to the axis ''(plane containing the purple section)''. The latter distance is given as a positive or negative number depending on which side of the reference plane faces the point. The ''origin'' of the system is the point where all three coordinates can be given as zero. This is the intersection between the reference plane and the axis. The axis is variously called the ''cylindrical'' or ''longitudinal'' axis, to differentiate it from the ''polar axis'', which is the ray that lies in the reference plane, starting at the origin and pointing in the reference direction. Other directions perpendicular to the longitudinal axis are called ''radial lines''. The ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Georg Amadeus Carl Friedrich Naumann
Georg Amadeus Carl Friedrich Naumann (30 May 1797 – 26 November 1873), also known as Karl Friedrich Naumann, was a German mineralogist and geologist. The crater Naumann on the Moon is named after him. Life Naumann was born at Dresden, the son of a distinguished musician and composer. He received his early education at Pforta, studied at Freiberg under Werner, and afterwards at Leipzig and Jena. He graduated at Jena, and was occupied in 1823 in teaching in that town and in 1824 at Leipzig. In 1826 he succeeded Mohs as professor of crystallography, in 1835 he became professor also of geognosy at Freiberg; and in 1842 he was appointed professor of mineralogy and geognosy in the University of Leipzig. At Freiberg he was charged with the preparation of a geological map of Saxony, which he carried out with the aid of Bernhard von Cotta in 1846. Naumann was a man of encyclopedic knowledge, lucid and fluent as a teacher. Early in life (1821-1822) he traveled in Norway, and his ob ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
