|
Composite Field
In quantum field theory, a composite field is a field defined in terms of other more "elementary" fields. It might describe a composite particle (bound state) or it might not. It might be Principle of locality, local, or it might be quantum nonlocality, nonlocal. Noether fields are often composite fields and they are local. In the generalized LSZ formalism, composite fields, which are usually nonlocal, are used to model asymptotic bound states. See also * Fermionic field * Bosonic field * Auxiliary field Quantum field theory {{Quantum-stub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quantum Field Theory
In theoretical physics, quantum field theory (QFT) is a theoretical framework that combines classical field theory, special relativity, and quantum mechanics. QFT is used in particle physics to construct physical models of subatomic particles and in condensed matter physics to construct models of quasiparticles. QFT treats particles as excited states (also called Quantum, quanta) of their underlying quantum field (physics), fields, which are more fundamental than the particles. The equation of motion of the particle is determined by minimization of the Lagrangian, a functional of fields associated with the particle. Interactions between particles are described by interaction terms in the Lagrangian (field theory), Lagrangian involving their corresponding quantum fields. Each interaction can be visually represented by Feynman diagrams according to perturbation theory (quantum mechanics), perturbation theory in quantum mechanics. History Quantum field theory emerged from the wo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Composite Particle
This is a list of known and hypothesized particles. Elementary particles Elementary particles are particles with no measurable internal structure; that is, it is unknown whether they are composed of other particles. They are the fundamental objects of quantum field theory. Many families and sub-families of elementary particles exist. Elementary particles are classified according to their spin. Fermions have half-integer spin while bosons have integer spin. All the particles of the Standard Model have been experimentally observed, including the Higgs boson in 2012. Many other hypothetical elementary particles, such as the graviton, have been proposed, but not observed experimentally. Fermions Fermions are one of the two fundamental classes of particles, the other being bosons. Fermion particles are described by Fermi–Dirac statistics and have quantum numbers described by the Pauli exclusion principle. They include the quarks and leptons, as well as any composite particles ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bound State
Bound or bounds may refer to: Mathematics * Bound variable * Upper and lower bounds, observed limits of mathematical functions Physics * Bound state, a particle that has a tendency to remain localized in one or more regions of space Geography *Bound Brook (Raritan River), a tributary of the Raritan River in New Jersey * Bound Brook, New Jersey, a borough in Somerset County People *Bound (surname) *Bounds (surname) Arts, entertainment, and media Films * ''Bound'' (1996 film), an American neo-noir film by the Wachowskis * ''Bound'' (2015 film), an American erotic thriller film by Jared Cohn * ''Bound'' (2018 film), a Nigerian romantic drama film by Frank Rajah Arase Television * "Bound" (''Fringe''), an episode of ''Fringe'' * "Bound" (''The Secret Circle''), an episode of ''The Secret Circle'' * "Bound" (''Star Trek: Enterprise''), an episode of ''Star Trek: Enterprise'' Other arts, entertainment, and media * ''Bound'' (video game), a PlayStation 4 game * "Bound", a song ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Principle Of Locality
In physics, the principle of locality states that an object is influenced directly only by its immediate surroundings. A theory that includes the principle of locality is said to be a "local theory". This is an alternative to the concept of instantaneous "action at a distance". Locality evolved out of the field theories of classical physics. The concept is that for an action at one point to have an influence at another point, something in the space between those points must mediate the action. To exert an influence, something, such as a wave or particle, must travel through the space between the two points, carrying the influence. The special theory of relativity limits the speed at which all such influences can travel to the speed of light, c. Therefore, the principle of locality implies that an event at one point cannot cause a simultaneous result at another point. An event at point A cannot cause a result at point B in a time less than T=D/c, where D is the distance between ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
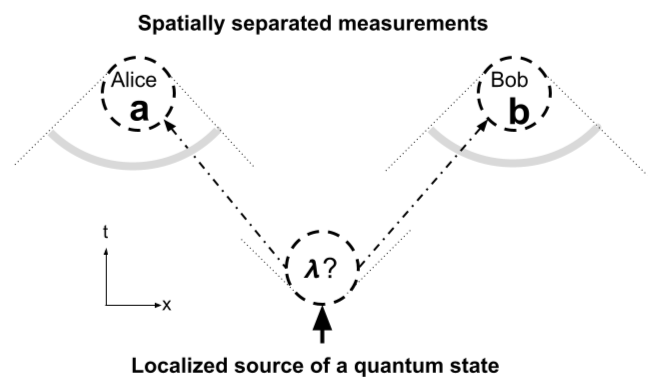
Quantum Nonlocality
In theoretical physics, quantum nonlocality refers to the phenomenon by which the measurement statistics of a multipartite quantum system do not admit an interpretation in terms of a local realistic theory. Quantum nonlocality has been experimentally verified under different physical assumptions. Any physical theory that aims at superseding or replacing quantum theory should account for such experiments and therefore cannot fulfill local realism; quantum nonlocality is a property of the universe that is independent of our description of nature. Quantum nonlocality does not allow for faster-than-light communication, and hence is compatible with special relativity and its universal speed limit of objects. Thus, quantum theory is local in the strict sense defined by special relativity and, as such, the term "quantum nonlocality" is sometimes considered a misnomer. Still, it prompts many of the foundational discussions concerning quantum theory. History Einstein, Podolsky and Rose ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Noether Field
Noether is the family name of several mathematicians (particularly, the Noether family), and the name given to some of their mathematical contributions: * Max Noether (1844–1921), father of Emmy and Fritz Noether, and discoverer of: ** Noether inequality ** Max Noether's theorem, several theorems * Emmy Noether (1882–1935), professor at the University of Göttingen and at Bryn Mawr College ** Noether's theorem (or Noether's first theorem) ** Noether's second theorem ** Noether normalization lemma ** Noetherian rings ** Nöther crater, on the far side of the moon, named after Emmy Noether * Fritz Noether (1884–1941), professor at the University of Tomsk * Gottfried E. Noether (1915–1991), son of Fritz Noether, statistician at the University of Connecticut See also * Noether's theorem (other) * List of things named after Emmy Noether Emmy Noether was a German mathematician who flourished in the early 20th century. This article is dedicated to the things ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
LSZ Formalism
In quantum field theory, the LSZ reduction formula is a method to calculate ''S''-matrix elements (the scattering amplitudes) from the time-ordered correlation functions of a quantum field theory. It is a step of the path that starts from the Lagrangian of some quantum field theory and leads to prediction of measurable quantities. It is named after the three German physicists Harry Lehmann, Kurt Symanzik and Wolfhart Zimmermann. Although the LSZ reduction formula cannot handle bound states, massless particles and topological solitons, it can be generalized to cover bound states, by use of composite fields which are often nonlocal. Furthermore, the method, or variants thereof, have turned out to be also fruitful in other fields of theoretical physics. For example, in statistical physics they can be used to get a particularly general formulation of the fluctuation-dissipation theorem. In and out fields ''S''-matrix elements are amplitudes of transitions between ''in'' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Asymptotic
In analytic geometry, an asymptote () of a curve is a line such that the distance between the curve and the line approaches zero as one or both of the ''x'' or ''y'' coordinates tends to infinity. In projective geometry and related contexts, an asymptote of a curve is a line which is tangent to the curve at a point at infinity. The word asymptote is derived from the Greek ἀσύμπτωτος (''asumptōtos'') which means "not falling together", from ἀ priv. + σύν "together" + πτωτ-ός "fallen". The term was introduced by Apollonius of Perga in his work on conic sections, but in contrast to its modern meaning, he used it to mean any line that does not intersect the given curve. There are three kinds of asymptotes: ''horizontal'', ''vertical'' and ''oblique''. For curves given by the graph of a function , horizontal asymptotes are horizontal lines that the graph of the function approaches as ''x'' tends to Vertical asymptotes are vertical lines near which the fu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fermionic Field
In quantum field theory, a fermionic field is a quantum field whose quanta are fermions; that is, they obey Fermi–Dirac statistics. Fermionic fields obey canonical anticommutation relations rather than the canonical commutation relations of bosonic fields. The most prominent example of a fermionic field is the Dirac field, which describes fermions with spin-1/2: electrons, protons, quarks, etc. The Dirac field can be described as either a 4-component spinor or as a pair of 2-component Weyl spinors. Spin-1/2 Majorana fermions, such as the hypothetical neutralino, can be described as either a dependent 4-component Majorana spinor or a single 2-component Weyl spinor. It is not known whether the neutrino is a Majorana fermion or a Dirac fermion; observing neutrinoless double-beta decay experimentally would settle this question. Basic properties Free (non-interacting) fermionic fields obey canonical anticommutation relations; i.e., involve the anticommutators = ''ab'' + ''ba ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bosonic Field
In quantum field theory, a bosonic field is a quantum field whose quanta are bosons; that is, they obey Bose–Einstein statistics. Bosonic fields obey canonical commutation relations, as distinct from the canonical anticommutation relations obeyed by fermionic fields. Examples include scalar fields, describing spin-0 particles such as the Higgs boson, and gauge fields, describing spin-1 particles such as the photon. Basic properties Free (non-interacting) bosonic fields obey canonical commutation relations. Those relations also hold for interacting bosonic fields in the interaction picture, where the fields evolve in time as if free and the effects of the interaction are encoded in the evolution of the states. It is these commutation relations that imply Bose–Einstein statistics for the field quanta. Examples Examples of bosonic fields include scalar fields, gauge fields, and symmetric 2-tensor fields, which are characterized by their covariance under Lorentz transformations ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Auxiliary Field
In physics, and especially quantum field theory, an auxiliary field is one whose equations of motion admit a single solution. Therefore, the Lagrangian describing such a field A contains an algebraic quadratic term and an arbitrary linear term, while it contains no kinetic terms (derivatives of the field): :\mathcal_\text = \frac(A, A) + (f(\varphi), A). The equation of motion for A is :A(\varphi) = -f(\varphi), and the Lagrangian becomes :\mathcal_\text = -\frac(f(\varphi), f(\varphi)). Auxiliary fields generally do not propagate, and hence the content of any theory can remain unchanged in many circumstances by adding such fields by hand. If we have an initial Lagrangian \mathcal_0 describing a field \varphi, then the Lagrangian describing both fields is :\mathcal = \mathcal_0(\varphi) + \mathcal_\text = \mathcal_0(\varphi) - \frac\big(f(\varphi), f(\varphi)\big). Therefore, auxiliary fields can be employed to cancel quadratic terms in \varphi in \mathcal_0 and linearize the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
