|
Centrosymmetric
In crystallography, a centrosymmetric point group contains an inversion center as one of its symmetry elements. In such a point group, for every point (x, y, z) in the unit cell there is an indistinguishable point (-x, -y, -z). Such point groups are also said to have ''inversion'' symmetry. Point reflection is a similar term used in geometry. Crystals with an inversion center cannot display certain properties, such as the piezoelectric effect. The following space groups have inversion symmetry: the triclinic space group 2, the monoclinic 10-15, the orthorhombic 47-74, the tetragonal 83-88 and 123-142, the trigonal 147, 148 and 162-167, the hexagonal 175, 176 and 191-194, the cubic 200-206 and 221-230. Point groups lacking an inversion center (non-centrosymmetric) can be '' polar'', ''chiral'', both, or neither. A '' polar'' point group is one whose symmetry operations leave more than one common point unmoved. A polar point group has no unique origin because each of those un ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Centrosymmetric Matrix
In mathematics, especially in linear algebra and matrix theory, a centrosymmetric matrix is a matrix which is symmetric about its center. More precisely, an ''n''×''n'' matrix ''A'' = 'A''''i'',''j''is centrosymmetric when its entries satisfy :''A''''i'',''j'' = ''A''''n''−''i'' + 1,''n''−''j'' + 1 for ''i'', ''j'' ∊. If ''J'' denotes the ''n''×''n'' exchange matrix with 1 on the antidiagonal and 0 elsewhere (that is, ''J''''i'',''n'' + 1 − ''i'' = 1; ''J''''i'',''j'' = 0 if ''j'' ≠ ''n'' +1− ''i''), then a matrix ''A'' is centrosymmetric if and only if ''AJ'' = ''JA''. Examples * All 2×2 centrosymmetric matrices have the form \begin a & b \\ b & a \end. * All 3×3 centrosymmetric matrices have the form \begin a & b & c \\ d & e & d \\ c & b & a \end. * Symmetric Toeplitz matrices are centrosymmetric. Algebraic structure and properties *If ''A'' and ''B'' are centrosymmetric matrices over a field ''F'', then so are ''A'' + ''B'' and ''cA'' for any ''c'' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Point Reflection
In geometry, a point reflection (point inversion, central inversion, or inversion through a point) is a type of isometry of Euclidean space. An object that is invariant under a point reflection is said to possess point symmetry; if it is invariant under point reflection through its center, it is said to possess central symmetry or to be centrally symmetric. Point reflection can be classified as an affine transformation. Namely, it is an isometric involutive affine transformation, which has exactly one fixed point, which is the point of inversion. It is equivalent to a homothetic transformation with scale factor equal to −1. The point of inversion is also called homothetic center. Terminology The term ''reflection'' is loose, and considered by some an abuse of language, with ''inversion'' preferred; however, ''point reflection'' is widely used. Such maps are involutions, meaning that they have order 2 – they are their own inverse: applying them twice yields the identi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Piezoelectric Effect
Piezoelectricity (, ) is the electric charge that accumulates in certain solid materials—such as crystals, certain ceramics, and biological matter such as bone, DNA, and various proteins—in response to applied mechanical stress. The word ''piezoelectricity'' means electricity resulting from pressure and latent heat. It is derived from the Greek word ; ''piezein'', which means to squeeze or press, and ''ēlektron'', which means amber, an ancient source of electric charge. The piezoelectric effect results from the linear electromechanical interaction between the mechanical and electrical states in crystalline materials with no inversion symmetry. The piezoelectric effect is a reversible process: materials exhibiting the piezoelectric effect also exhibit the reverse piezoelectric effect, the internal generation of a mechanical strain resulting from an applied electrical field. For example, lead zirconate titanate crystals will generate measurable piezoelectricity when th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rule Of Mutual Exclusion
The rule of mutual exclusion in molecular spectroscopy relates the observation of molecular vibrations to molecular symmetry. It states that no normal modes can be both Infrared and Raman active in a molecule that possesses a centre of symmetry. This is a powerful application of group theory to vibrational spectroscopy, and allows one to easily detect the presence of this symmetry element by comparison of the IR and Raman spectra generated by the same molecule. The rule arises because in a centrosymmetric point group, IR active modes, which must transform according to the same irreducible representation generated by one of the components of the dipole moment vector (x, y or z), must be of ''ungerade'' (u) symmetry, i.e. their character under inversion is -1, while Raman active modes, which transform according to the symmetry of the polarizability tensor (product of two coordinates), must be of ''gerade'' (g) symmetry since their character under inversion is +1. Thus, in the char ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Crystallography
Crystallography is the experimental science of determining the arrangement of atoms in crystalline solids. Crystallography is a fundamental subject in the fields of materials science and solid-state physics (condensed matter physics). The word "crystallography" is derived from the Greek word κρύσταλλος (''krystallos'') "clear ice, rock-crystal", with its meaning extending to all solids with some degree of transparency, and γράφειν (''graphein'') "to write". In July 2012, the United Nations recognised the importance of the science of crystallography by proclaiming that 2014 would be the International Year of Crystallography. denote a direction vector (in real space). * Coordinates in ''angle brackets'' or ''chevrons'' such as <100> denote a ''family'' of directions which are related by symmetry operations. In the cubic crystal system for example, would mean 00 10 01/nowiki> or the negative of any of those directions. * Miller indices in ''parentheses ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Point Group
In geometry, a point group is a mathematical group of symmetry operations ( isometries in a Euclidean space) that have a fixed point in common. The coordinate origin of the Euclidean space is conventionally taken to be a fixed point, and every point group in dimension ''d'' is then a subgroup of the orthogonal group O(''d''). Point groups are used to describe the symmetries of geometric figures and physical objects such as molecules. Each point group can be represented as sets of orthogonal matrices ''M'' that transform point ''x'' into point ''y'' according to Each element of a point group is either a rotation (determinant of ''M'' = 1), or it is a reflection or improper rotation (determinant of ''M'' = −1). The geometric symmetries of crystals are described by space groups, which allow translations and contain point groups as subgroups. Discrete point groups in more than one dimension come in infinite families, but from the crystallographic restriction theore ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Symmetry
Symmetry (from grc, συμμετρία "agreement in dimensions, due proportion, arrangement") in everyday language refers to a sense of harmonious and beautiful proportion and balance. In mathematics, "symmetry" has a more precise definition, and is usually used to refer to an object that is invariant under some transformations; including translation, reflection, rotation or scaling. Although these two meanings of "symmetry" can sometimes be told apart, they are intricately related, and hence are discussed together in this article. Mathematical symmetry may be observed with respect to the passage of time; as a spatial relationship; through geometric transformations; through other kinds of functional transformations; and as an aspect of abstract objects, including theoretic models, language, and music. This article describes symmetry from three perspectives: in mathematics, including geometry, the most familiar type of symmetry for many people; in science and n ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
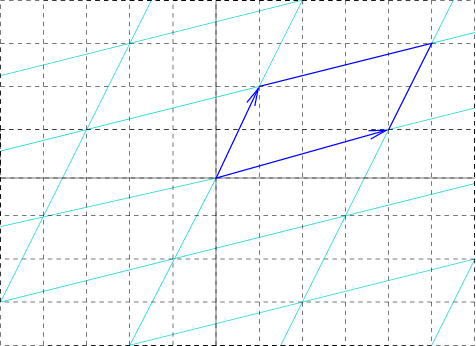
Unit Cell
In geometry, biology, mineralogy and solid state physics, a unit cell is a repeating unit formed by the vectors spanning the points of a lattice. Despite its suggestive name, the unit cell (unlike a unit vector, for example) does not necessarily have unit size, or even a particular size at all. Rather, the primitive cell is the closest analogy to a unit vector, since it has a determined size for a given lattice and is the basic building block from which larger cells are constructed. The concept is used particularly in describing crystal structure in two and three dimensions, though it makes sense in all dimensions. A lattice can be characterized by the geometry of its unit cell, which is a section of the tiling (a parallelogram or parallelepiped) that generates the whole tiling using only translations. There are two special cases of the unit cell: the primitive cell and the conventional cell. The primitive cell is a unit cell corresponding to a single lattice point, it is th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Space Group
In mathematics, physics and chemistry, a space group is the symmetry group of an object in space, usually in three dimensions. The elements of a space group (its symmetry operations) are the rigid transformations of an object that leave it unchanged. In three dimensions, space groups are classified into 219 distinct types, or 230 types if chiral copies are considered distinct. Space groups are discrete cocompact groups of isometries of an oriented Euclidean space in any number of dimensions. In dimensions other than 3, they are sometimes called Bieberbach groups. In crystallography, space groups are also called the crystallographic or Fedorov groups, and represent a description of the symmetry of the crystal. A definitive source regarding 3-dimensional space groups is the ''International Tables for Crystallography'' . History Space groups in 2 dimensions are the 17 wallpaper groups which have been known for several centuries, though the proof that the list was compl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Polar Point Group
In geometry, a polar point group is a point group in which there is more than one point that every symmetry operation leaves unmoved. The unmoved points will constitute a line, a plane, or all of space. While the simplest point group, C1, leaves all points invariant, most polar point groups will move some, but not all points. To describe the points which are unmoved by the symmetry operations of the point group, we draw a straight line joining two unmoved points. This line is called a polar direction. The electric polarization must be parallel to a polar direction. In polar point groups of high symmetry, the polar direction can be a unique axis of rotation, but if the symmetry operations do not allow any rotation at all, such as mirror symmetry, there can be an infinite number of such axes: in that case the only restriction on the polar direction is that it must be parallel to any mirror planes. A point group with more than one axis of rotation or with a mirror plane perpendicular ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Chirality (mathematics)
In geometry, a figure is chiral (and said to have chirality) if it is not identical to its mirror image, or, more precisely, if it cannot be mapped to its mirror image by rotations and translations alone. An object that is not chiral is said to be ''achiral''. A chiral object and its mirror image are said to be enantiomorphs. The word ''chirality'' is derived from the Greek (cheir), the hand, the most familiar chiral object; the word ''enantiomorph'' stems from the Greek (enantios) 'opposite' + (morphe) 'form'. Examples Some chiral three-dimensional objects, such as the helix, can be assigned a right or left handedness, according to the right-hand rule. Many other familiar objects exhibit the same chiral symmetry of the human body, such as gloves and shoes. Right shoes differ from left shoes only by being mirror images of each other. In contrast thin gloves may not be considered chiral if you can wear them inside-out. The J, L, S and Z-shaped '' tetrominoes'' of the pop ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |