|
Capillarity
Capillary action (sometimes called capillarity, capillary motion, capillary rise, capillary effect, or wicking) is the process of a liquid flowing in a narrow space without the assistance of, or even in opposition to, any external forces like gravity. The effect can be seen in the drawing up of liquids between the hairs of a paint-brush, in a thin tube, in porous materials such as paper and plaster, in some non-porous materials such as sand and liquefied carbon fiber, or in a biological cell. It occurs because of intermolecular forces between the liquid and surrounding solid surfaces. If the diameter of the tube is sufficiently small, then the combination of surface tension (which is caused by cohesion within the liquid) and adhesive forces between the liquid and container wall act to propel the liquid. Etymology Capillary comes from the Latin word capillaris, meaning "of or resembling hair." The meaning stems from the tiny, hairlike diameter of a capillary. While capill ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Surface Tension
Surface tension is the tendency of liquid surfaces at rest to shrink into the minimum surface area possible. Surface tension is what allows objects with a higher density than water such as razor blades and insects (e.g. water striders) to float on a water surface without becoming even partly submerged. At liquid–air interfaces, surface tension results from the greater attraction of liquid molecules to each other (due to cohesion) than to the molecules in the air (due to adhesion). There are two primary mechanisms in play. One is an inward force on the surface molecules causing the liquid to contract. Second is a tangential force parallel to the surface of the liquid. This ''tangential'' force is generally referred to as the surface tension. The net effect is the liquid behaves as if its surface were covered with a stretched elastic membrane. But this analogy must not be taken too far as the tension in an elastic membrane is dependent on the amount of deformation of th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Capillarity
Capillary action (sometimes called capillarity, capillary motion, capillary rise, capillary effect, or wicking) is the process of a liquid flowing in a narrow space without the assistance of, or even in opposition to, any external forces like gravity. The effect can be seen in the drawing up of liquids between the hairs of a paint-brush, in a thin tube, in porous materials such as paper and plaster, in some non-porous materials such as sand and liquefied carbon fiber, or in a biological cell. It occurs because of intermolecular forces between the liquid and surrounding solid surfaces. If the diameter of the tube is sufficiently small, then the combination of surface tension (which is caused by cohesion within the liquid) and adhesive forces between the liquid and container wall act to propel the liquid. Etymology Capillary comes from the Latin word capillaris, meaning "of or resembling hair." The meaning stems from the tiny, hairlike diameter of a capillary. While capill ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Young–Laplace Equation
In physics, the Young–Laplace equation () is an algebraic equation that describes the capillary pressure difference sustained across the interface between two static fluids, such as water and air, due to the phenomenon of surface tension or wall tension, although use of the latter is only applicable if assuming that the wall is very thin. The Young–Laplace equation relates the pressure difference to the shape of the surface or wall and it is fundamentally important in the study of static capillary surfaces. It's a statement of normal stress balance for static fluids meeting at an interface, where the interface is treated as a surface (zero thickness): \begin \Delta p &= -\gamma \nabla \cdot \hat n \\ &= -2\gamma H_f \\ &= -\gamma \left(\frac + \frac\right) \end where \Delta p is the Laplace pressure, the pressure difference across the fluid interface (the exterior pressure minus the interior pressure), \gamma is the surface tension (or wall tension), \hat n is the unit no ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
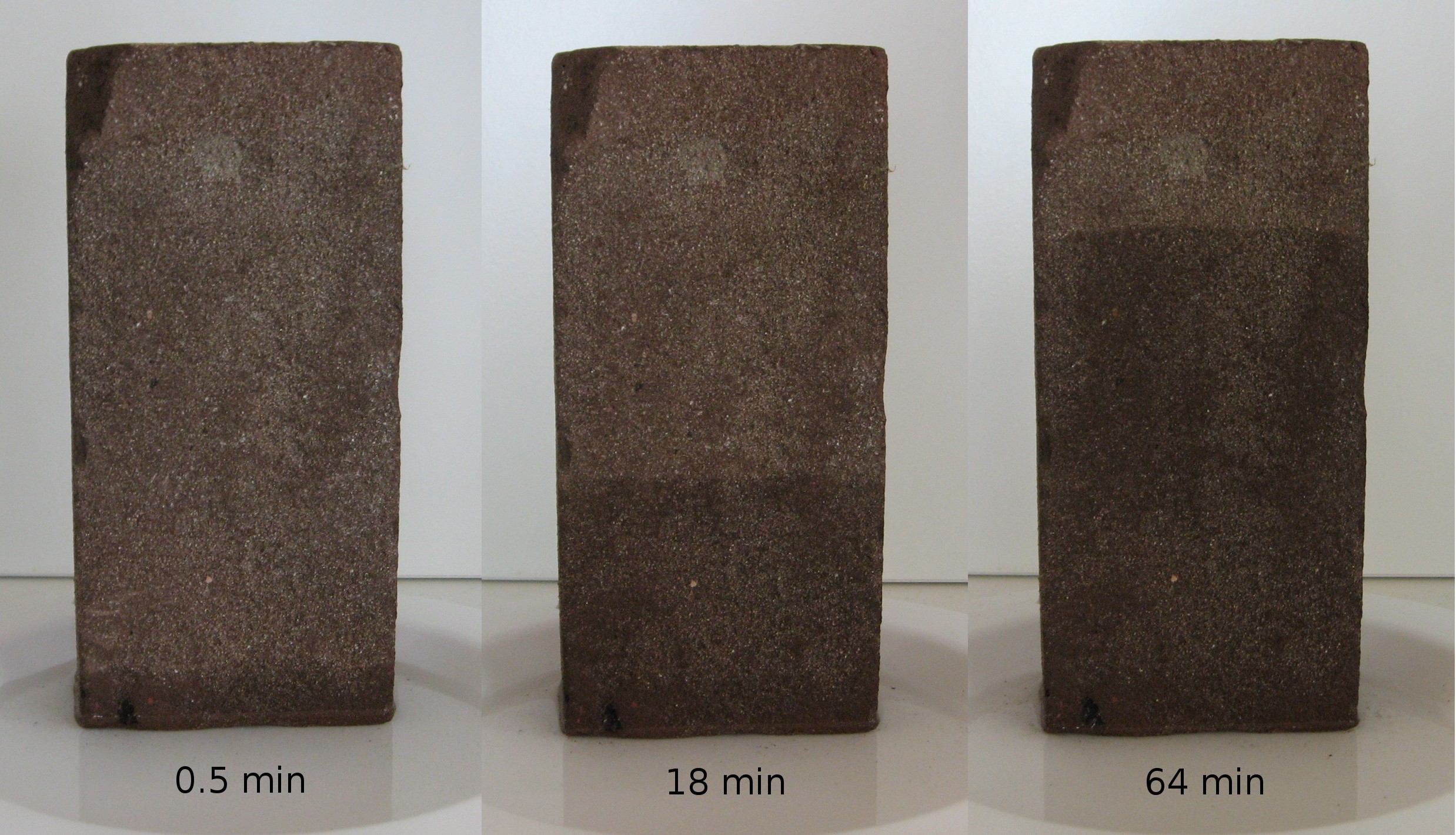
Capillary Flow Brick
A capillary is a small blood vessel from 5 to 10 micrometres (μm) in diameter. Capillaries are composed of only the tunica intima, consisting of a thin wall of simple squamous endothelial cells. They are the smallest blood vessels in the body: they convey blood between the arterioles and venules. These microvessels are the site of exchange of many substances with the interstitial fluid surrounding them. Substances which cross capillaries include water, oxygen, carbon dioxide, urea, glucose, uric acid, lactic acid and creatinine. Lymph capillaries connect with larger lymph vessels to drain lymphatic fluid collected in the microcirculation. During early embryonic development, new capillaries are formed through vasculogenesis, the process of blood vessel formation that occurs through a '' de novo'' production of endothelial cells that then form vascular tubes. The term '' angiogenesis'' denotes the formation of new capillaries from pre-existing blood vessels and already present en ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kelvin Equation
The Kelvin equation describes the change in vapour pressure due to a curved liquid–vapor interface, such as the surface of a droplet. The vapor pressure at a convex curved surface is higher than that at a flat surface. The Kelvin equation is dependent upon thermodynamic principles and does not allude to special properties of materials. It is also used for determination of pore size distribution of a porous medium using adsorption porosimetry. The equation is named in honor of William Thomson, also known as Lord Kelvin. Formulation The original form of the Kelvin equation, published in 1871, is: p(r_1 , r_2) = P - \frac \left ( \frac + \frac \right ), where: * p(r) = vapor pressure at a curved interface of radius r * P = vapor pressure at flat interface ( r = \infty ) = p_ * \gamma = surface tension * \rho _ = density of vapor * \rho _ = density of liquid * r_1 , r_2 = radii of curvature along the principal sections of the curved interface. This may ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Vapor Pressure
Vapor pressure (or vapour pressure in English-speaking countries other than the US; see spelling differences) or equilibrium vapor pressure is defined as the pressure exerted by a vapor in thermodynamic equilibrium with its condensed phases (solid or liquid) at a given temperature in a closed system. The equilibrium vapor pressure is an indication of a liquid's evaporation rate. It relates to the tendency of particles to escape from the liquid (or a solid). A substance with a high vapor pressure at normal temperatures is often referred to as '' volatile''. The pressure exhibited by vapor present above a liquid surface is known as vapor pressure. As the temperature of a liquid increases, the kinetic energy of its molecules also increases. As the kinetic energy of the molecules increases, the number of molecules transitioning into a vapor also increases, thereby increasing the vapor pressure. The vapor pressure of any substance increases non-linearly with temperature accord ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Meniscus (liquid)
The meniscus (plural: ''menisci'', from the Greek for "crescent") is the curve in the upper surface of a liquid close to the surface of the container or another object, produced by surface tension. A concave meniscus occurs when the attraction between the particles of the liquid and the container (adhesion) is more than half the attraction of the particles of the liquid to each other ( cohesion), causing the liquid to climb the walls of the container (see surface tension#Causes). This occurs between water and glass. Water-based fluids like sap, honey, and milk also have a concave meniscus in glass or other wettable containers. Conversely, a convex meniscus occurs when the adhesion energy is less than half the cohesion energy. Convex menisci occur, for example, between mercury and glass in barometers and thermometers. In general, the shape of the surface of a liquid can be complex. For a sufficiently small circular tube, the shape of the meniscus will approximate a section ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
William Thomson, 1st Baron Kelvin
William Thomson, 1st Baron Kelvin, (26 June 182417 December 1907) was a British mathematician, mathematical physicist and engineer born in Belfast. Professor of Natural Philosophy at the University of Glasgow for 53 years, he did important work in the mathematical analysis of electricity and formulation of the first and second laws of thermodynamics, and did much to unify the emerging discipline of physics in its contemporary form. He received the Royal Society's Copley Medal in 1883, was its president 1890–1895, and in 1892 was the first British scientist to be elevated to the House of Lords. Absolute temperatures are stated in units of kelvin in his honour. While the existence of a coldest possible temperature ( absolute zero) was known prior to his work, Kelvin is known for determining its correct value as approximately −273.15 degrees Celsius or −459.67 degrees Fahrenheit. The Joule–Thomson effect is also named in his honour. He worked closely with math ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Carl Friedrich Gauss
Johann Carl Friedrich Gauss (; german: Gauß ; la, Carolus Fridericus Gauss; 30 April 177723 February 1855) was a German mathematician and physicist who made significant contributions to many fields in mathematics and science. Sometimes referred to as the ''Princeps mathematicorum'' () and "the greatest mathematician since antiquity", Gauss had an exceptional influence in many fields of mathematics and science, and he is ranked among history's most influential mathematicians. Also available at Retrieved 23 February 2014. Comprehensive biographical article. Biography Early years Johann Carl Friedrich Gauss was born on 30 April 1777 in Brunswick (Braunschweig), in the Duchy of Brunswick-Wolfenbüttel (now part of Lower Saxony, Germany), to poor, working-class parents. His mother was illiterate and never recorded the date of his birth, remembering only that he had been born on a Wednesday, eight days before the Feast of the Ascension (which occurs 39 days after Easter). ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pierre-Simon Laplace
Pierre-Simon, marquis de Laplace (; ; 23 March 1749 – 5 March 1827) was a French scholar and polymath whose work was important to the development of engineering, mathematics, statistics, physics, astronomy, and philosophy. He summarized and extended the work of his predecessors in his five-volume Traité de mécanique céleste, ''Mécanique céleste'' (''Celestial Mechanics'') (1799–1825). This work translated the geometric study of classical mechanics to one based on calculus, opening up a broader range of problems. In statistics, the Bayesian probability, Bayesian interpretation of probability was developed mainly by Laplace. Laplace formulated Laplace's equation, and pioneered the Laplace transform which appears in many branches of mathematical physics, a field that he took a leading role in forming. The Laplace operator, Laplacian differential operator, widely used in mathematics, is also named after him. He restated and developed the nebular hypothesis of the origi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Thomas Young (scientist)
Thomas Young FRS (13 June 177310 May 1829) was a British polymath who made notable contributions to the fields of vision, light, solid mechanics, energy, physiology, language, musical harmony, and Egyptology. He was instrumental in the decipherment of Egyptian hieroglyphs, specifically the Rosetta Stone. Young has been described as " The Last Man Who Knew Everything". His work influenced that of William Herschel, Hermann von Helmholtz, James Clerk Maxwell, and Albert Einstein. Young is credited with establishing the wave theory of light, in contrast to the particle theory of Isaac Newton. Young's work was subsequently supported by the work of Augustin-Jean Fresnel. Personal life Young belonged to a Quaker family of Milverton, Somerset, where he was born in 1773, the eldest of ten children. At the age of fourteen Young had learned Greek and Latin. Young began to study medicine in London at St Bartholomew's Hospital in 1792, moved to the University of Edinburgh Medi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Alexis Clairaut
Alexis Claude Clairaut (; 13 May 1713 – 17 May 1765) was a French mathematician, astronomer, and geophysicist. He was a prominent Newtonian whose work helped to establish the validity of the principles and results that Sir Isaac Newton had outlined in the '' Principia'' of 1687. Clairaut was one of the key figures in the expedition to Lapland that helped to confirm Newton's theory for the figure of the Earth. In that context, Clairaut worked out a mathematical result now known as " Clairaut's theorem". He also tackled the gravitational three-body problem, being the first to obtain a satisfactory result for the apsidal precession of the Moon's orbit. In mathematics he is also credited with Clairaut's equation and Clairaut's relation. Biography Childhood and early life Clairaut was born in Paris, France, to Jean-Baptiste and Catherine Petit Clairaut. The couple had 20 children, however only a few of them survived childbirth. His father taught mathematics. Alexis was a prodigy ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |




.jpg)

