|
Beta-binomial Model
In probability theory and statistics, the beta-binomial distribution is a family of discrete probability distributions on a finite support of non-negative integers arising when the probability of success in each of a fixed or known number of Bernoulli trials is either unknown or random. The beta-binomial distribution is the binomial distribution in which the probability of success at each of ''n'' trials is not fixed but randomly drawn from a beta distribution. It is frequently used in Bayesian statistics, empirical Bayes methods and classical statistics to capture overdispersion in binomial type distributed data. The beta-binomial is a one-dimensional version of the Dirichlet-multinomial distribution as the binomial and beta distributions are univariate versions of the multinomial and Dirichlet distributions respectively. The special case where ''α'' and ''β'' are integers is also known as the negative hypergeometric distribution. Motivation and derivation As a compound dis ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Beta-binomial Distribution Pmf
In probability theory and statistics, the beta-binomial distribution is a family of discrete probability distributions on a finite Support (mathematics), support of non-negative integers arising when the probability of success in each of a fixed or known number of Bernoulli trials is either unknown or random. The beta-binomial distribution is the binomial distribution in which the probability of success at each of ''n'' trials is not fixed but randomly drawn from a beta distribution. It is frequently used in Bayesian statistics, empirical Bayes methods and classical statistics to capture overdispersion in binomial type distributed data. The beta-binomial is a one-dimensional version of the Dirichlet-multinomial distribution as the binomial and beta distributions are univariate versions of the multinomial distribution, multinomial and Dirichlet distributions respectively. The special case where ''α'' and ''β'' are integers is also known as the negative hypergeometric distribution ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Overdispersion
In statistics, overdispersion is the presence of greater variability (statistical dispersion) in a data set than would be expected based on a given statistical model. A common task in applied statistics is choosing a parametric model to fit a given set of empirical observations. This necessitates an assessment of the fit of the chosen model. It is usually possible to choose the model parameters in such a way that the theoretical population mean of the model is approximately equal to the sample mean. However, especially for simple models with few parameters, theoretical predictions may not match empirical observations for higher moments. When the observed variance is higher than the variance of a theoretical model, overdispersion has occurred. Conversely, underdispersion means that there was less variation in the data than predicted. Overdispersion is a very common feature in applied data analysis because in practice, populations are frequently heterogeneous (non-uniform) contrary ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Kurtosis
In probability theory and statistics, kurtosis (from el, κυρτός, ''kyrtos'' or ''kurtos'', meaning "curved, arching") is a measure of the "tailedness" of the probability distribution of a real-valued random variable. Like skewness, kurtosis describes a particular aspect of a probability distribution. There are different ways to quantify kurtosis for a theoretical distribution, and there are corresponding ways of estimating it using a sample from a population. Different measures of kurtosis may have different interpretations. The standard measure of a distribution's kurtosis, originating with Karl Pearson, is a scaled version of the fourth moment of the distribution. This number is related to the tails of the distribution, not its peak; hence, the sometimes-seen characterization of kurtosis as "peakedness" is incorrect. For this measure, higher kurtosis corresponds to greater extremity of deviations (or outliers), and not the configuration of data near the mean. It is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Moment (mathematics)
In mathematics, the moments of a function are certain quantitative measures related to the shape of the function's graph. If the function represents mass density, then the zeroth moment is the total mass, the first moment (normalized by total mass) is the center of mass, and the second moment is the moment of inertia. If the function is a probability distribution, then the first moment is the expected value, the second central moment is the variance, the third standardized moment is the skewness, and the fourth standardized moment is the kurtosis. The mathematical concept is closely related to the concept of moment in physics. For a distribution of mass or probability on a bounded interval, the collection of all the moments (of all orders, from to ) uniquely determines the distribution (Hausdorff moment problem). The same is not true on unbounded intervals (Hamburger moment problem). In the mid-nineteenth century, Pafnuty Chebyshev became the first person to think systematic ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
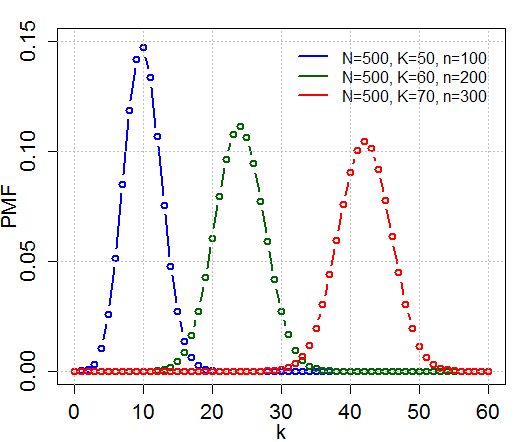
Hypergeometric Distribution
In probability theory and statistics, the hypergeometric distribution is a discrete probability distribution that describes the probability of k successes (random draws for which the object drawn has a specified feature) in n draws, ''without'' replacement, from a finite population of size N that contains exactly K objects with that feature, wherein each draw is either a success or a failure. In contrast, the binomial distribution describes the probability of k successes in n draws ''with'' replacement. Definitions Probability mass function The following conditions characterize the hypergeometric distribution: * The result of each draw (the elements of the population being sampled) can be classified into one of two mutually exclusive categories (e.g. Pass/Fail or Employed/Unemployed). * The probability of a success changes on each draw, as each draw decreases the population (''sampling without replacement'' from a finite population). A random variable X follows the hyperg ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pólya Urn Model
In statistics, a Pólya urn model (also known as a Pólya urn scheme or simply as Pólya's urn), named after George Pólya, is a type of statistical model used as an idealized mental exercise framework, unifying many treatments. In an urn model, objects of real interest (such as atoms, people, cars, etc.) are represented as colored balls in an urn or other container. In the basic Pólya urn model, the urn contains ''x'' white and ''y'' black balls; one ball is drawn randomly from the urn and its color observed; it is then returned in the urn, and an additional ball of the same color is added to the urn, and the selection process is repeated. Questions of interest are the evolution of the urn population and the sequence of colors of the balls drawn out. This endows the urn with a self-reinforcing property sometimes expressed as ''the rich get richer''. Note that in some sense, the Pólya urn model is the "opposite" of the model of sampling without replacement, where every time a p ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Integer
An integer is the number zero (), a positive natural number (, , , etc.) or a negative integer with a minus sign (−1, −2, −3, etc.). The negative numbers are the additive inverses of the corresponding positive numbers. In the language of mathematics, the set of integers is often denoted by the boldface or blackboard bold \mathbb. The set of natural numbers \mathbb is a subset of \mathbb, which in turn is a subset of the set of all rational numbers \mathbb, itself a subset of the real numbers \mathbb. Like the natural numbers, \mathbb is countably infinite. An integer may be regarded as a real number that can be written without a fractional component. For example, 21, 4, 0, and −2048 are integers, while 9.75, , and are not. The integers form the smallest group and the smallest ring containing the natural numbers. In algebraic number theory, the integers are sometimes qualified as rational integers to distinguish them from the more general algebraic integers ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Urn Model
In probability and statistics, an urn problem is an idealized mental exercise in which some objects of real interest (such as atoms, people, cars, etc.) are represented as colored balls in an urn or other container. One pretends to remove one or more balls from the urn; the goal is to determine the probability of drawing one color or another, or some other properties. A number of important variations are described below. An urn model is either a set of probabilities that describe events within an urn problem, or it is a probability distribution, or a family of such distributions, of random variables associated with urn problems.Dodge, Yadolah (2003) ''Oxford Dictionary of Statistical Terms'', OUP. History In ''Ars Conjectandi'' (1713), Jacob Bernoulli considered the problem of determining, given a number of pebbles drawn from an urn, the proportions of different colored pebbles within the urn. This problem was known as the ''inverse probability'' problem, and was a topic of res ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Compound Distribution
In probability and statistics, a compound probability distribution (also known as a mixture distribution or contagious distribution) is the probability distribution that results from assuming that a random variable is distributed according to some parametrized distribution, with (some of) the parameters of that distribution themselves being random variables. If the parameter is a scale parameter, the resulting mixture is also called a scale mixture. The compound distribution ("unconditional distribution") is the result of marginalizing (integrating) over the ''latent'' random variable(s) representing the parameter(s) of the parametrized distribution ("conditional distribution"). Definition A compound probability distribution is the probability distribution that results from assuming that a random variable X is distributed according to some parametrized distribution F with an unknown parameter \theta that is again distributed according to some other distribution G. The resulting ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Conjugate Prior
In Bayesian probability theory, if the posterior distribution p(\theta \mid x) is in the same probability distribution family as the prior probability distribution p(\theta), the prior and posterior are then called conjugate distributions, and the prior is called a conjugate prior for the likelihood function p(x \mid \theta). A conjugate prior is an algebraic convenience, giving a closed-form expression for the posterior; otherwise, numerical integration may be necessary. Further, conjugate priors may give intuition by more transparently showing how a likelihood function updates a prior distribution. The concept, as well as the term "conjugate prior", were introduced by Howard Raiffa and Robert Schlaifer in their work on Bayesian decision theory.Howard Raiffa and Robert Schlaifer. ''Applied Statistical Decision Theory''. Division of Research, Graduate School of Business Administration, Harvard University, 1961. A similar concept had been discovered independently by George Alfred ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Beta Distribution
In probability theory and statistics, the beta distribution is a family of continuous probability distributions defined on the interval , 1in terms of two positive parameters, denoted by ''alpha'' (''α'') and ''beta'' (''β''), that appear as exponents of the random variable and control the shape of the distribution. The beta distribution has been applied to model the behavior of random variables limited to intervals of finite length in a wide variety of disciplines. The beta distribution is a suitable model for the random behavior of percentages and proportions. In Bayesian inference, the beta distribution is the conjugate prior probability distribution for the Bernoulli, binomial, negative binomial and geometric distributions. The formulation of the beta distribution discussed here is also known as the beta distribution of the first kind, whereas ''beta distribution of the second kind'' is an alternative name for the beta prime distribution. The generalization to mult ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
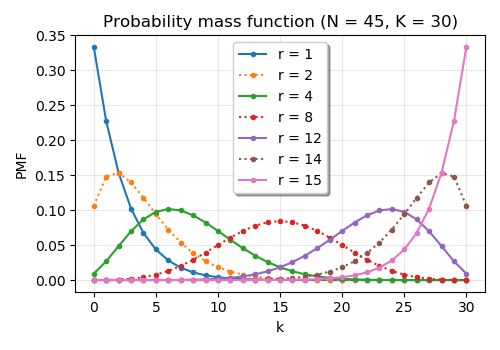
Negative Hypergeometric Distribution
In probability theory and statistics, the negative hypergeometric distribution describes probabilities for when sampling from a finite population without replacement in which each sample can be classified into two mutually exclusive categories like Pass/Fail or Employed/Unemployed. As random selections are made from the population, each subsequent draw decreases the population causing the probability of success to change with each draw. Unlike the standard hypergeometric distribution, which describes the number of successes in a fixed sample size, in the negative hypergeometric distribution, samples are drawn until r failures have been found, and the distribution describes the probability of finding k successes in such a sample. In other words, the negative hypergeometric distribution describes the likelihood of k successes in a sample with exactly r failures. Definition There are N elements, of which K are defined as "successes" and the rest are "failures". Elements are drawn ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |