|
Witting Polytope
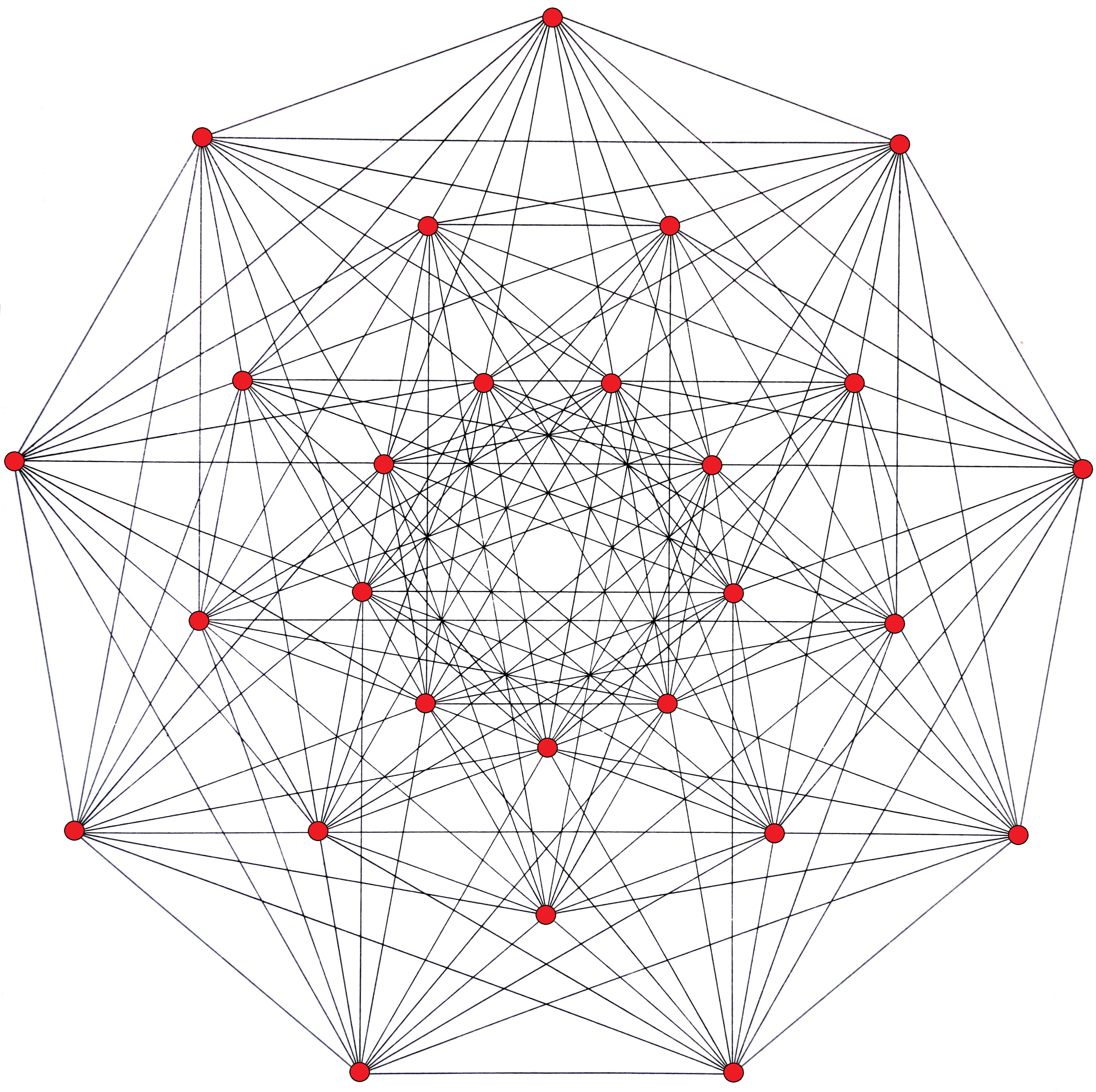
In 4-dimensional complex geometry, the Witting polytope is a regular complex polytope, named as: 3333, and Coxeter diagram . It has 240 vertices, 2160 3 edges, 2160 Möbius–Kantor polygon, 33 faces, and 240 Hessian polyhedron, 333 cells. It is self-dual. Each vertex belongs to 27 edges, 72 faces, and 27 cells, corresponding to the Hessian polyhedron vertex figure. Symmetry Its symmetry by 3[3]3[3]3[3]3 or , order 155,520. It has 240 copies of , order 648 at each cell. Structure The Configuration (polytope), configuration matrix is: \left [\begin240&27&72&27\\3&2160&8&8\\8&8&2160&3\\27&72&27&240\end\right ] The number of vertices, edges, faces, and cells are seen in the diagonal of the matrix. These are computed by the order of the group divided by the order of the subgroup, by removing certain complex reflections, shown with X below. The number of elements of the k-faces are seen in rows below the diagonal. The number of elements in the vertex figure, etc., are given in rows ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Witting Polytope
In 4-dimensional complex geometry, the Witting polytope is a regular complex polytope, named as: 3333, and Coxeter diagram . It has 240 vertices, 2160 3 edges, 2160 Möbius–Kantor polygon, 33 faces, and 240 Hessian polyhedron, 333 cells. It is self-dual. Each vertex belongs to 27 edges, 72 faces, and 27 cells, corresponding to the Hessian polyhedron vertex figure. Symmetry Its symmetry by 3[3]3[3]3[3]3 or , order 155,520. It has 240 copies of , order 648 at each cell. Structure The Configuration (polytope), configuration matrix is: \left [\begin240&27&72&27\\3&2160&8&8\\8&8&2160&3\\27&72&27&240\end\right ] The number of vertices, edges, faces, and cells are seen in the diagonal of the matrix. These are computed by the order of the group divided by the order of the subgroup, by removing certain complex reflections, shown with X below. The number of elements of the k-faces are seen in rows below the diagonal. The number of elements in the vertex figure, etc., are given in rows ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Regular Complex Polytope
In geometry, a complex polytope is a generalization of a polytope in real space to an analogous structure in a complex Hilbert space, where each real dimension is accompanied by an imaginary one. A complex polytope may be understood as a collection of complex points, lines, planes, and so on, where every point is the junction of multiple lines, every line of multiple planes, and so on. Precise definitions exist only for the regular complex polytopes, which are configurations. The regular complex polytopes have been completely characterized, and can be described using a symbolic notation developed by Coxeter. Some complex polytopes which are not fully regular have also been described. Definitions and introduction The complex line \mathbb^1 has one dimension with real coordinates and another with imaginary coordinates. Applying real coordinates to both dimensions is said to give it two dimensions over the real numbers. A real plane, with the imaginary axis labelled as such, is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Complex Polytope
In geometry, a complex polytope is a generalization of a polytope in real space to an analogous structure in a complex Hilbert space, where each real dimension is accompanied by an imaginary one. A complex polytope may be understood as a collection of complex points, lines, planes, and so on, where every point is the junction of multiple lines, every line of multiple planes, and so on. Precise definitions exist only for the regular complex polytopes, which are configurations. The regular complex polytopes have been completely characterized, and can be described using a symbolic notation developed by Coxeter. Some complex polytopes which are not fully regular have also been described. Definitions and introduction The complex line \mathbb^1 has one dimension with real number, real coordinates and another with imaginary coordinates. Applying real coordinates to both dimensions is said to give it two dimensions over the real numbers. A real plane, with the imaginary axis labelled ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Trion (geometry)
In geometry, a complex polytope is a generalization of a polytope in real space to an analogous structure in a complex Hilbert space, where each real dimension is accompanied by an imaginary one. A complex polytope may be understood as a collection of complex points, lines, planes, and so on, where every point is the junction of multiple lines, every line of multiple planes, and so on. Precise definitions exist only for the regular complex polytopes, which are configurations. The regular complex polytopes have been completely characterized, and can be described using a symbolic notation developed by Coxeter. Some complex polytopes which are not fully regular have also been described. Definitions and introduction The complex line \mathbb^1 has one dimension with real coordinates and another with imaginary coordinates. Applying real coordinates to both dimensions is said to give it two dimensions over the real numbers. A real plane, with the imaginary axis labelled as such, is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Van Oss Polygon
In geometry, a complex polytope is a generalization of a polytope in real space to an analogous structure in a complex Hilbert space, where each real dimension is accompanied by an imaginary one. A complex polytope may be understood as a collection of complex points, lines, planes, and so on, where every point is the junction of multiple lines, every line of multiple planes, and so on. Precise definitions exist only for the regular complex polytopes, which are configurations. The regular complex polytopes have been completely characterized, and can be described using a symbolic notation developed by Coxeter. Some complex polytopes which are not fully regular have also been described. Definitions and introduction The complex line \mathbb^1 has one dimension with real coordinates and another with imaginary coordinates. Applying real coordinates to both dimensions is said to give it two dimensions over the real numbers. A real plane, with the imaginary axis labelled as such, is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Harold Scott MacDonald Coxeter
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century. Biography Coxeter was born in Kensington to Harold Samuel Coxeter and Lucy (). His father had taken over the family business of Coxeter & Son, manufacturers of surgical instruments and compressed gases (including a mechanism for anaesthetising surgical patients with nitrous oxide), but was able to retire early and focus on sculpting and baritone singing; Lucy Coxeter was a portrait and landscape painter who had attended the Royal Academy of Arts. A maternal cousin was the architect Sir Giles Gilbert Scott. In his youth, Coxeter composed music and was an accomplished pianist at the age of 10. Roberts, Siobhan, ''King of Infinite Space: Donald Coxeter, The Man Who Saved Geometry'', Walker & Company, 2006, He felt that mathematics and music were intimately related, outlining his i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
F-vector
Polyhedral combinatorics is a branch of mathematics, within combinatorics and discrete geometry, that studies the problems of counting and describing the faces of convex polyhedra and higher-dimensional convex polytopes. Research in polyhedral combinatorics falls into two distinct areas. Mathematicians in this area study the combinatorics of polytopes; for instance, they seek inequalities that describe the relations between the numbers of vertices, edges, and faces of higher dimensions in arbitrary polytopes or in certain important subclasses of polytopes, and study other combinatorial properties of polytopes such as their connectivity and diameter (number of steps needed to reach any vertex from any other vertex). Additionally, many computer scientists use the phrase “polyhedral combinatorics” to describe research into precise descriptions of the faces of certain specific polytopes (especially 0-1 polytopes, whose vertices are subsets of a hypercube) arising from integer progr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
5 21 Honeycomb
In geometry, the 521 honeycomb is a uniform tessellation of 8-dimensional Euclidean space. The symbol 521 is from Coxeter, named for the length of the 3 branches of its Coxeter-Dynkin diagram.Coxeter, 1973, Chapter 5: The Kaleidoscope By putting spheres at its vertices one obtains the densest-possible packing of spheres in 8 dimensions. This was proven by Maryna Viazovska in 2016 using the theory of modular forms. Viazovska was awarded the Fields Medal for this work in 2022. This honeycomb was first studied by Gosset who called it a ''9-ic semi-regular figure'' (Gosset regarded honeycombs in ''n'' dimensions as degenerate ''n''+1 polytopes). Each vertex of the 521 honeycomb is surrounded by 2160 8-orthoplexes and 17280 8-simplicies. The vertex figure of Gosset's honeycomb is the semiregular 421 polytope. It is the final figure in the k21 family. This honeycomb is highly regular in the sense that its symmetry group (the affine _8 Weyl group) acts transitively on the ''k''- ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
4 21 Polytope
In 8-dimensional geometry, the 421 is a semiregular uniform 8-polytope, constructed within the symmetry of the E8 group. It was discovered by Thorold Gosset, published in his 1900 paper. He called it an ''8-ic semi-regular figure''.Gosset, 1900 Its Coxeter symbol is 421, describing its bifurcating Coxeter-Dynkin diagram, with a single ring on the end of the 4-node sequences, . The rectified 421 is constructed by points at the mid-edges of the 421. The birectified 421 is constructed by points at the triangle face centers of the 421. The trirectified 421 is constructed by points at the tetrahedral centers of the 421. These polytopes are part of a family of 255 = 28 − 1 convex uniform 8-polytopes, made of uniform 7-polytope facets and vertex figures, defined by all permutations of one or more rings in this Coxeter-Dynkin diagram: . 421 polytope The 421 polytope has 17,280 7-simplex and 2,160 7-orthoplex facets, and 240 vertices. Its vertex figure is the 321 po ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Alexander Witting
Carl Johann Adolf Alexander Witting (18 December 1861 – 29 November 1946) was a German mathematician. Family Witting was born in Dresden as the first child of the musician Carl Witting (1823–1907) and the painter Minna Witting, née Japha (1828–1882). Alexander Witting married the pianist Sophie Sebass (1864–1924) in 1889. They had two daughters and a son: Tillyta (1890–1970), Lotte (1894–1971) and the physicist Rudolf Witting (1899–1963). In view of the artistically affected family environment – father musician, mother painter, aunt Louise Japha (1826–1910) pianist, sister Agnes Witting (1863–1937) singer, brother Walther Witting painter – it does not surprise that Alexander Witting also painted sometimes and regularly made music, even beyond the narrow circle of family or colleagues. Education He successfully completed his final grammar school examinations at the Städtische Gymnasium zum Heiligen Kreuz (Kreuzschule) in Dresden at Easter 1880, served ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hyperplane
In geometry, a hyperplane is a subspace whose dimension is one less than that of its ''ambient space''. For example, if a space is 3-dimensional then its hyperplanes are the 2-dimensional planes, while if the space is 2-dimensional, its hyperplanes are the 1-dimensional lines. This notion can be used in any general space in which the concept of the dimension of a subspace is defined. In different settings, hyperplanes may have different properties. For instance, a hyperplane of an -dimensional affine space is a flat subset with dimension and it separates the space into two half spaces. While a hyperplane of an -dimensional projective space does not have this property. The difference in dimension between a subspace and its ambient space is known as the codimension of with respect to . Therefore, a necessary and sufficient condition for to be a hyperplane in is for to have codimension one in . Technical description In geometry, a hyperplane of an ''n''-dimensi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
K-face
In solid geometry, a face is a flat surface (a planar region) that forms part of the boundary of a solid object; a three-dimensional solid bounded exclusively by faces is a ''polyhedron''. In more technical treatments of the geometry of polyhedra and higher-dimensional polytopes, the term is also used to mean an element of any dimension of a more general polytope (in any number of dimensions).. Polygonal face In elementary geometry, a face is a polygon on the boundary of a polyhedron. Other names for a polygonal face include polyhedron side and Euclidean plane ''tile''. For example, any of the six squares that bound a cube is a face of the cube. Sometimes "face" is also used to refer to the 2-dimensional features of a 4-polytope. With this meaning, the 4-dimensional tesseract has 24 square faces, each sharing two of 8 cubic cells. Number of polygonal faces of a polyhedron Any convex polyhedron's surface has Euler characteristic :V - E + F = 2, where ''V'' is the number of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |