|
W. T. Tutte
William Thomas Tutte OC FRS FRSC (; 14 May 1917 – 2 May 2002) was an English and Canadian codebreaker and mathematician. During the Second World War, he made a brilliant and fundamental advance in cryptanalysis of the Lorenz cipher, a major Nazi Germany, Nazi German cipher system which was used for top-secret communications within the Wehrmacht High Command. The high-level, strategic nature of the intelligence obtained from Tutte's crucial breakthrough, in the bulk decrypting of Lorenz-enciphered messages specifically, contributed greatly, and perhaps even decisively, to the defeat of Nazi Germany. He also had a number of significant mathematical accomplishments, including foundation work in the fields of graph theory and matroid theory. Tutte's research in the field of graph theory proved to be of remarkable importance. At a time when graph theory was still a primitive subject, Tutte commenced the study of matroids and developed them into a theory by expanding from the wor ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Newmarket, Suffolk
Newmarket is a market town and civil parish in the West Suffolk district of Suffolk, England. Located (14 miles) west of Bury St Edmunds and (14 miles) northeast of Cambridge. It is considered the birthplace and global centre of thoroughbred horse racing. It is a major local business cluster, with annual investment rivalling that of the Cambridge Science Park, the other major cluster in the region. It is the largest racehorse training centre in Britain, the largest racehorse breeding centre in the country, home to most major British horseracing institutions, and a key global centre for horse health. Two Classic races, and an additional three British Champions Series races are held at Newmarket every year. The town has had close royal connections since the time of James I, who built a palace there, and was also a base for Charles I, Charles II, and most monarchs since. Elizabeth II visited the town often to see her horses in training. Newmarket has over fifty horse training stabl ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tutte Polynomial
The Tutte polynomial, also called the dichromate or the Tutte–Whitney polynomial, is a graph polynomial. It is a polynomial in two variables which plays an important role in graph theory. It is defined for every undirected graph G and contains information about how the graph is connected. It is denoted by T_G. The importance of this polynomial stems from the information it contains about G. Though originally studied in algebraic graph theory as a generalization of counting problems related to graph coloring and nowhere-zero flow, it contains several famous other specializations from other sciences such as the Jones polynomial from knot theory and the partition functions of the Potts model from statistical physics. It is also the source of several central computational problems in theoretical computer science. The Tutte polynomial has several equivalent definitions. It is equivalent to Whitney’s rank polynomial, Tutte’s own dichromatic polynomial and Fortuin–Kasteleyn ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Royal Society Of Canada
The Royal Society of Canada (RSC; french: Société royale du Canada, SRC), also known as the Academies of Arts, Humanities and Sciences of Canada (French: ''Académies des arts, des lettres et des sciences du Canada''), is the senior national, bilingual council of distinguished Canadian scholars, humanists, scientists and artists. The primary objective of the RSC is to promote learning and research in the arts, the humanities and the sciences. The RSC is Canada's National Academy and exists to promote Canadian research and scholarly accomplishment in both official languages, to recognize academic and artistic excellence, and to advise governments, non-governmental organizations and Canadians on matters of public interest. History In the late 1870s, the Governor General of Canada, the Marquis of Lorne, determined that Canada required a cultural institution to promote national scientific research and development. Since that time, succeeding Governor Generals have remained involved w ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Royal Society
The Royal Society, formally The Royal Society of London for Improving Natural Knowledge, is a learned society and the United Kingdom's national academy of sciences. The society fulfils a number of roles: promoting science and its benefits, recognising excellence in science, supporting outstanding science, providing scientific advice for policy, education and public engagement and fostering international and global co-operation. Founded on 28 November 1660, it was granted a royal charter by King Charles II as The Royal Society and is the oldest continuously existing scientific academy in the world. The society is governed by its Council, which is chaired by the Society's President, according to a set of statutes and standing orders. The members of Council and the President are elected from and by its Fellows, the basic members of the society, who are themselves elected by existing Fellows. , there are about 1,700 fellows, allowed to use the postnominal title FRS (Fellow of the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Order Of Canada
The Order of Canada (french: Ordre du Canada; abbreviated as OC) is a Canadian state order and the second-highest honour for merit in the system of orders, decorations, and medals of Canada, after the Order of Merit. To coincide with the centennial of Canadian Confederation, the three-tiered order was established in 1967 as a fellowship that recognizes the outstanding merit or distinguished service of Canadians who make a major difference to Canada through lifelong contributions in every field of endeavour, as well as the efforts by non-Canadians who have made the world better by their actions. Membership is accorded to those who exemplify the order's Latin motto, , meaning "they desire a better country", a phrase taken from Hebrews 11:16. The three tiers of the order are Companion, Officer, and Member; specific individuals may be given extraordinary membership and deserving non-Canadians may receive honorary appointment into each grade. , the reigning Canadian monarch, is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
CRM-Fields-PIMS Prize
The CRM-Fields-PIMS Prize is the premier Canadian research prize in the mathematical sciences. It is awarded in recognition of exceptional research achievement in the mathematical sciences and is given annually by three Canadian mathematics institutes: the Centre de Recherches Mathématiques (CRM), the Fields Institute, and the Pacific Institute for the Mathematical Sciences (PIMS). The prize was established in 1994 by the CRM and the Fields Institute as the CRM-Fields Prize. The prize took its current name when PIMS became a partner in 2005. The prize carries a monetary award of $10,000, funded jointly by the three institutes. The inaugural prize winner was H.S.M. Coxeter. Winners Source: Centre de recherches mathématiques *1995 – H. S. M. Coxeter *1996 – George A. Elliott *1997 – James Arthur *1998 – Robert V. Moody *1999 – Stephen A. Cook *2000 – Israel Michael Sigal *2001 – William T. Tutte *2002 – John B. Friedlander *2003 – John McKay and Edwin P ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Isaak-Walton-Killam Award
The Izaak Walton Killam Memorial Prize was established according to the will of Dorothy J. Killam to honour the memory of her husband Izaak Walton Killam. Five Killam Prizes, each having a value of $100,000, are annually awarded by the Canada Council for the Arts to eminent Canadian researchers who distinguish themselves in the fields of social, human, natural, or health sciences. In August 2021, the Canada Council announced it would transition the administration of the Killam program to the National Research Council Canada (NRC) by March 2022. The restructured Killam Program was launched under the administration of the NRC in April 2022. It is now called the National Killam Program and consists of the Killam Prizes and the Dorothy Killam Fellowships. Recipients See also * List of medicine awards * List of social sciences awards This list of social sciences awards is an index to articles about notable awards given for contributions to social sciences in general. It exclude ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Henry Marshall Tory Medal
The Henry Marshall Tory Medal is an award of the Royal Society of Canada "for outstanding research in a branch of astronomy, chemistry, mathematics, physics, or an allied science". It is named in honour of Henry Marshall Tory and is awarded bi-annually. The award consists of a gold plated silver medal. Recipients Source Royal Society of Canada See also * List of general science and technology awards * List of awards named after people This is a list of awards that are named after people. A B C D E F G H I J K L M N O P R S T U - V W Y Z See also * Lists of awards * List of eponyms * List of awards named after governors- ... References * {{Royal Society of Canada Canadian science and technology awards Royal Society of Canada Awards established in 1943 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Jeffery–Williams Prize
The Jeffery–Williams Prize is a mathematics award presented annually by the Canadian Mathematical Society. The award is presented to individuals in recognition of outstanding contributions to mathematical research. The first award was presented in 1968. The prize was named in honor of the mathematicians Ralph Lent Jeffery Ralph Lent Jeffery (3 October 1889 Overton, Yarmouth County, Nova Scotia, Canada – 1975 Wolfville, Nova Scotia) was a Canadian mathematician working on analysis. He taught at several institutions including Acadia University, the University of S ... and Lloyd Williams. Recipients of the Jeffery–Williams Prize SourceCanadian Mathematical Society See also * List of mathematics awards References External links Canadian Mathematical Society {{DEFAULTSORT:Jeffery-Williams Prize Awards of the Canadian Mathematical Society Awards established in 1968 1968 establishments in Canada ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tutte's Fragment
In mathematics, Tait's conjecture states that "Every 3-connected planar cubic graph has a Hamiltonian cycle (along the edges) through all its vertices". It was proposed by and disproved by , who constructed a counterexample with 25 faces, 69 edges and 46 vertices. Several smaller counterexamples, with 21 faces, 57 edges and 38 vertices, were later proved minimal by . The condition that the graph be 3-regular is necessary due to polyhedra such as the rhombic dodecahedron, which forms a bipartite graph with six degree-four vertices on one side and eight degree-three vertices on the other side; because any Hamiltonian cycle would have to alternate between the two sides of the bipartition, but they have unequal numbers of vertices, the rhombic dodecahedron is not Hamiltonian. The conjecture was significant, because if true, it would have implied the four color theorem: as Tait described, the four-color problem is equivalent to the problem of finding 3-edge-colorings of bridgeless ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
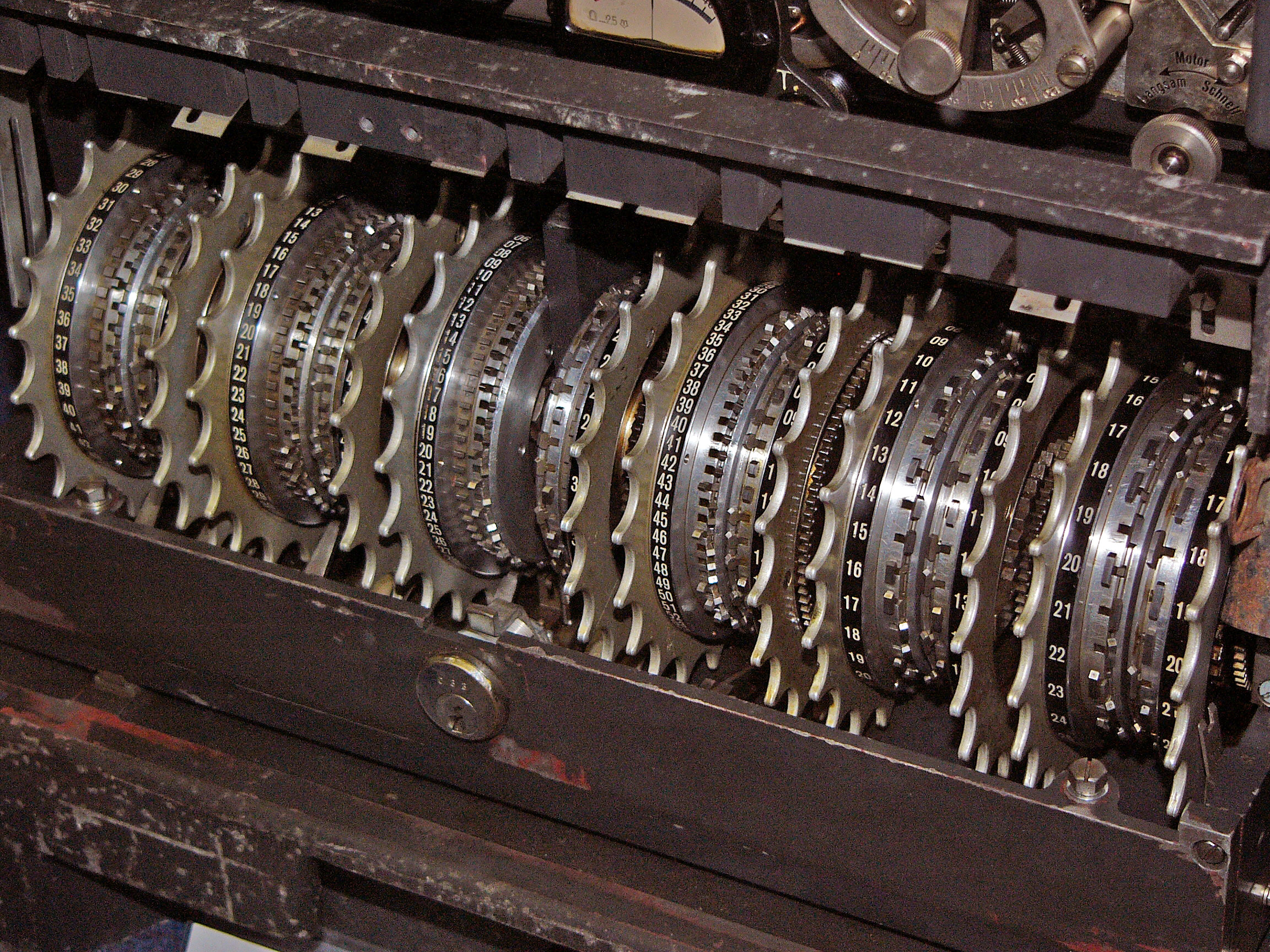
Cryptanalysis Of The Lorenz Cipher
Cryptanalysis of the Lorenz cipher was the process that enabled the British to read high-level German army messages during World War II. The British Government Code and Cypher School (GC&CS) at Bletchley Park decrypted many communications between the '' Oberkommando der Wehrmacht'' (OKW, German High Command) in Berlin and their army commands throughout occupied Europe, some of which were signed "Adolf Hitler, Führer". These were intercepted non-Morse radio transmissions that had been enciphered by the Lorenz SZ teleprinter rotor stream cipher attachments. Decrypts of this traffic became an important source of "Ultra" intelligence, which contributed significantly to Allied victory. For its high-level secret messages, the German armed services enciphered each character using various online ''Geheimschreiber'' (secret writer) stream cipher machines at both ends of a telegraph link using the 5-bit International Telegraphy Alphabet No. 2 (ITA2). These machines were subsequently di ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tutte–Grothendieck Invariant
In mathematics, a Tutte–Grothendieck (TG) invariant is a type of graph invariant that satisfies a generalized deletion–contraction formula. Any evaluation of the Tutte polynomial would be an example of a TG invariant. Definition A graph function ''f'' is TG-invariant if: f(G) = \begin c^ & \text \\ xf(G/e) & \text e \text \\ yf(G \backslash e) & \text e \text \\ af(G/e) + bf(G \backslash e) & \text \end Above ''G'' / ''e'' denotes edge contraction whereas ''G'' \ ''e'' denotes deletion. The numbers ''c'', ''x'', ''y'', ''a'', ''b'' are parameters. Generalization to matroids The matroid function ''f'' is TG if: : \begin &f(M_1\oplus M_2) = f(M_1)f(M_2) \\ &f(M) = af(M/e) + b f(M \backslash e) \ \ \ \text e \text \end It can be shown that ''f'' is given by: : f(M) = a^b^ T(M; x/a, y/b) where ''E'' is the edge set of ''M''; ''r'' is the rank function; and : T(M; x, y) = \sum_ (x-1)^ (y-1)^ is the generalization of the Tutte polynomial to matroids. Grothendieck group ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |